Big Ideas Math Green Answers and Solutions

In the world of educational resources, providing clear and accessible solutions to complex problems is essential for student success. This guide offers a valuable reference for those navigating through intricate mathematical concepts. Whether you’re tackling assignments, preparing for exams, or enhancing your understanding, having detailed explanations at your fingertips can make all the difference.

Effective learning involves breaking down difficult topics into manageable steps, allowing students to grasp challenging material with ease. With the right approach, each problem becomes an opportunity for growth. This resource is designed to support learners in achieving proficiency and confidence in their studies.

By exploring the various methods of solving mathematical tasks, readers will discover practical techniques that lead to better outcomes. With the proper tools, solving equations and understanding core principles becomes more intuitive, helping to strengthen critical thinking and problem-solving abilities in the process.

Big Ideas Math Green Answers Overview

This section provides an overview of the essential tools and resources aimed at simplifying complex concepts in mathematics. By offering comprehensive problem-solving strategies and step-by-step guides, it allows students to gain a deeper understanding and mastery of the subject. The materials are designed to assist learners at various levels, ensuring they can approach mathematical challenges with confidence and clarity.

The purpose of these resources is to support effective learning by offering clear explanations and structured methodologies. Whether tackling basic arithmetic or more advanced topics, the solutions provided aim to break down difficult problems into manageable parts. This approach fosters a stronger grasp of mathematical concepts and encourages independent problem-solving skills.

• Step-by-step problem breakdowns for easier comprehension
• Practical strategies for solving a variety of equations
• Solutions aimed at reinforcing key mathematical principles
• Examples designed to illustrate common challenges
• Support for both classroom learning and self-study

With these resources, learners can expect to not only find correct solutions but also develop a deeper understanding of the processes behind them. This comprehensive approach ensures that students are not just memorizing answers but actively engaging with the material, which is key to long-term success in any mathematical field.

Understanding Big Ideas Math Series

This educational program is designed to provide a comprehensive approach to learning through a structured sequence of topics. Its core objective is to guide students in mastering key principles by offering clear explanations, targeted exercises, and a logical progression from one concept to the next. Each section is carefully organized to help learners build their knowledge step by step, ensuring a deeper understanding of complex ideas.

The curriculum covers a wide range of concepts, from basic operations to more advanced theories, making it suitable for learners at different levels. It emphasizes conceptual understanding alongside practical problem-solving, allowing students to not only achieve correct solutions but also understand the reasoning behind them.

Topic	Description	Skills Developed
Basic Operations	Focuses on fundamental arithmetic concepts	Addition, subtraction, multiplication, division
Algebra	Introduction to variables and equations	Solving equations, simplifying expressions
Geometry	Explores shapes, sizes, and spatial understanding	Perimeter, area, volume, angles
Statistics	Analyzing data sets and interpreting results	Mean, median, mode, probability

By progressing through the various stages of the program, students are encouraged to think critically and solve problems with confidence. The series builds a solid foundation for future learning, ensuring that students are well-prepared for more advanced topics in their academic journey.

Key Concepts in Big Ideas Math

At the heart of any successful educational program is a set of fundamental principles that guide students through their learning journey. These core concepts form the foundation upon which advanced skills and techniques are built. Understanding these key ideas allows learners to progress with confidence, mastering more complex topics along the way. In this section, we explore some of the most important concepts that are essential to achieving proficiency in the subject.

Building a Strong Foundation

The first step in mastering any subject is developing a solid grasp of the basics. From arithmetic operations to the introduction of variables, understanding the foundational concepts enables students to approach more advanced topics with ease. By focusing on core principles, learners are equipped with the tools they need to solve problems and make logical connections between different ideas.

Applying Concepts to Real-World Problems

The ability to apply learned concepts to practical situations is another crucial aspect of the learning process. Through exercises and problem-solving activities, students can see how abstract ideas are used in real-world contexts. This application helps reinforce theoretical knowledge and encourages critical thinking, allowing students to understand the relevance and importance of what they are learning.

How to Use Big Ideas Math Answers

Effective use of solution guides is essential for students seeking to strengthen their understanding of mathematical concepts. These resources offer clear, step-by-step breakdowns that help learners not only find correct solutions but also comprehend the methods behind them. By following these solutions, students can enhance their problem-solving skills and gain deeper insights into complex topics.

To make the most of these resources, it’s important to approach them strategically. Start by attempting problems on your own before referring to the solution. This process helps develop critical thinking and problem-solving skills. When reviewing solutions, focus on understanding the methodology used and the reasoning behind each step, rather than just memorizing the final answer.

Step	Action	Benefit
1	Attempt problems independently	Enhances problem-solving skills and confidence
2	Review solutions step-by-step	Builds a deeper understanding of methods
3	Identify patterns in approaches	Improves efficiency in solving similar problems
4	Practice similar exercises	Reinforces concepts and strengthens knowledge

By following this approach, students can use solution guides not only as a reference but as a tool to improve their overall learning experience. With each problem solved, the understanding of the subject becomes more profound, preparing learners for more challenging material in the future.

Step-by-Step Solutions for Math Problems

Breaking down problems into clear, manageable steps is a key method for mastering complex concepts. Step-by-step solutions provide learners with a structured approach to problem-solving, making it easier to understand the logic behind each answer. By following a systematic process, students can learn to approach similar challenges with confidence and accuracy.

Each solution is carefully designed to guide the student through the problem, showing the reasoning behind each step. This approach not only helps with finding the correct solution but also reinforces the underlying principles, ensuring a deeper comprehension of the subject.

• Start by analyzing the given information and identifying key variables.
• Break down the problem into smaller, easier-to-understand parts.
• Apply appropriate methods and formulas step by step.
• Recheck calculations to ensure accuracy and consistency.
• Understand the reasoning behind each step to retain the concept.

Following this process allows students to develop critical thinking skills while solving problems, ensuring they gain a solid understanding of the principles at play. With continued practice, learners can confidently tackle more advanced challenges in the future.

Common Mistakes in Math Problems

In the process of solving mathematical problems, it’s easy to make errors that can lead to incorrect answers or misunderstandings of key concepts. Recognizing these common mistakes is crucial for improving problem-solving skills and achieving better results. By identifying where errors occur, students can take proactive steps to avoid them in the future, ultimately enhancing their learning experience.

Some errors are simple miscalculations, while others stem from misunderstanding the steps involved in a particular method. Regardless of the type, understanding these mistakes can help learners correct their approach and gain more confidence in their problem-solving abilities.

Mistake	Cause	Solution
Sign errors	Incorrectly adding or subtracting positive and negative numbers	Carefully review each operation, especially when dealing with negative values
Misinterpretation of the problem	Overlooking key details or instructions in the question	Read the problem multiple times and highlight important information
Skipping steps	Rushing through the process or missing intermediate steps	Write out each step methodically and check work at each stage
Incorrect order of operations	Not following the correct sequence of operations (PEMDAS)	Always follow the order of operations: parentheses, exponents, multiplication/division, addition/subtraction
Calculation errors	Simple mistakes when adding, subtracting, multiplying, or dividing	Double-check each calculation and use a calculator when necessary

By staying mindful of these common mistakes and consistently practicing careful attention to detail, students can improve their accuracy and efficiency when solving problems. Over time, they will develop a stronger grasp of the material and enhance their overall performance.

Tips for Mastering Math with Big Ideas

Achieving mastery in mathematics requires a combination of persistence, effective strategies, and a deep understanding of core principles. The key to success is not just practicing problems, but also developing a strong conceptual foundation and a structured approach to solving challenges. By employing proven strategies and focusing on continuous improvement, students can build confidence and skill over time.

Develop a Strong Foundation

Before tackling complex problems, it’s essential to grasp the basic concepts that form the building blocks of the subject. Understanding core principles allows learners to solve problems more effectively and with greater ease. Begin by focusing on foundational topics such as basic operations, number properties, and geometric concepts. Once these are well-understood, progressing to more advanced topics will feel more intuitive.

Practice Consistently and Strategically

Regular practice is one of the most effective ways to master any subject, especially mathematics. However, it’s not just about doing problems–it’s about practicing strategically. Work on a variety of problem types to ensure comprehensive understanding. Identify areas where mistakes frequently occur and focus on improving those weak points. It’s also important to revisit challenging problems after some time to see if new insights emerge.

Lastly, always approach each new concept with an open mind and a willingness to understand the “why” behind the solution methods. The more time spent understanding the rationale behind each step, the more confident and proficient you will become in solving future problems.

Structure of Big Ideas Math Curriculum

The curriculum is designed to guide learners through a progressive and structured path, starting with fundamental concepts and advancing to more complex topics. Each unit builds on the knowledge acquired in previous sections, ensuring that students develop a comprehensive understanding of the subject. The materials are organized to encourage critical thinking, problem-solving, and application of learned skills to real-world scenarios.

The structure incorporates a blend of theoretical lessons, practice exercises, and assessments that allow students to reinforce their knowledge and measure their progress. The pacing is carefully planned to ensure that learners can absorb new concepts without feeling overwhelmed, with regular opportunities for review and reinforcement.

Additionally, the curriculum emphasizes mastery through a combination of guided instruction and independent learning. This balance allows students to understand key principles in depth while also applying them in diverse contexts. Each topic is introduced with clear explanations, followed by examples that illustrate the methods and techniques used to solve problems. By the end of each section, students are encouraged to reflect on what they have learned and how it connects to the broader concepts within the subject.

Best Practices for Studying Math

Mastering the subject requires more than just solving problems; it involves adopting effective techniques that foster deep understanding and retention. With the right strategies, students can tackle challenging concepts, improve problem-solving abilities, and enhance their overall performance. Consistency, focus, and smart study habits are the keys to success in this area.

Active Learning and Practice

One of the most effective ways to learn is through active engagement with the material. Practice regularly by solving problems on your own, rather than simply reading or watching explanations. Working through different types of exercises allows you to identify patterns, reinforce concepts, and apply methods in various contexts. Make it a habit to solve problems without looking at the solutions first, and only refer to them when you’re stuck to understand where you went wrong.

Organize Your Study Sessions

Effective studying isn’t just about the time spent–it’s about how that time is spent. Organize your study sessions by breaking down larger topics into manageable sections. Focus on one concept at a time, and ensure that you fully understand it before moving on to the next. Don’t try to cram multiple topics into one session; instead, prioritize quality over quantity. Use tools like flashcards, summary notes, or diagrams to organize key points, making it easier to review and reinforce your understanding.

By adopting these practices, students can build a stronger foundation in the subject, feel more confident in their abilities, and improve their overall academic success.

Math Problem-Solving Strategies Explained

Approaching complex problems requires a set of strategies that help break down the task into manageable steps. By applying structured techniques, students can enhance their ability to tackle challenges systematically. These strategies not only guide the process of finding solutions but also help deepen understanding by encouraging logical thinking and the application of core concepts.

Effective problem-solving is about recognizing patterns, identifying key variables, and using appropriate methods to find solutions. Whether it’s simplifying the problem, making connections to previously learned material, or working backward from the desired outcome, these techniques can significantly improve a student’s ability to solve problems efficiently and accurately.

Step-by-Step Approach

One of the most reliable techniques is to break down the problem into smaller, easier-to-handle parts. Start by understanding the problem thoroughly, then divide it into logical steps. Address one piece at a time rather than trying to solve the entire problem at once. This can make even the most complex problems feel more manageable and less overwhelming.

Check and Reflect

After reaching a solution, it’s essential to review your work to ensure it’s correct. Check each step of your solution to identify any potential errors or missed details. Reflecting on the process also helps to reinforce your understanding and identify areas where improvement is needed for future problems.

By consistently applying these strategies, learners can develop a stronger grasp of problem-solving techniques, building both confidence and competence in solving challenging tasks.

How Big Ideas Math Prepares Students

The curriculum is designed to equip students with the essential skills and knowledge they need to succeed in their academic journey. By fostering a deep understanding of core concepts and emphasizing problem-solving abilities, the program ensures that learners are well-prepared for future challenges. It focuses on developing both critical thinking and practical application, making it easier for students to apply their knowledge in real-life situations.

Building a Strong Conceptual Foundation

One of the key strengths of the program is its ability to build a strong foundation in core principles. Through a gradual progression of topics, students start with basic concepts and move toward more complex material. This method helps to ensure that they fully understand each step before advancing, minimizing confusion and providing clarity throughout the learning process. By focusing on foundational skills, learners gain confidence and mastery in the subject, which supports their success in more advanced topics.

Encouraging Independent Problem-Solving

The curriculum also emphasizes the importance of independent thinking and problem-solving. Students are encouraged to approach challenges with a variety of strategies, developing the ability to think critically and adapt their methods to different situations. This fosters not only academic success but also essential life skills, such as decision-making and creative thinking. Through practice and reflection, learners build the resilience needed to tackle increasingly difficult problems on their own.

Overall, this approach ensures that students are not just memorizing formulas but are truly understanding and applying what they learn, preparing them for success in their future studies and careers.

Reviewing the Key Math Topics

Effective learning requires a comprehensive review of essential concepts to ensure understanding and retention. Regularly revisiting the core topics strengthens the foundation and prepares students for more advanced challenges. By identifying the most crucial areas and reinforcing them through practice, learners can build the confidence and skills needed to solve problems with ease.

Each topic plays a vital role in shaping a student’s overall proficiency. From foundational principles to more complex applications, it’s important to grasp the underlying theories before moving forward. Regularly reviewing these key concepts allows for greater retention and smoother progression to more intricate material.

Core Concepts and Applications

Understanding fundamental principles is critical for mastering the subject. These concepts are the building blocks that later lead to more advanced theories. Focusing on areas such as algebraic expressions, number theory, and geometric principles enables students to connect the dots between topics and apply their knowledge in various scenarios.

Techniques for Effective Review

To effectively review, it is important to approach each topic with a strategic mindset. Break down complex topics into smaller, manageable parts and revisit them regularly. Use practice exercises to reinforce learning and identify areas where further clarification may be needed. Reflecting on previous lessons and connecting them to new material is a proven strategy for improving long-term retention.

Math Help Resources for Students

When tackling complex problems, having access to reliable resources can make a significant difference in a student’s understanding and performance. There are many tools and platforms available to provide the necessary support for mastering various concepts. Whether seeking additional explanations, practice exercises, or interactive tutorials, these resources are invaluable for reinforcing learning and providing assistance outside the classroom.

These resources cater to different learning styles, ensuring that every student can find a method that suits their individual needs. From online platforms offering step-by-step guidance to interactive apps that enhance practice, the range of tools available today is diverse and accessible.

Online Platforms and Websites

• Khan Academy: Offers free tutorials and exercises on a wide range of topics, from basic operations to more advanced subjects. It provides step-by-step explanations and interactive exercises.
• Wolfram Alpha: A computational search engine that can solve complex equations and provide step-by-step solutions.
• IXL: A comprehensive platform that offers interactive questions and feedback for a variety of subjects.

Additional Support Options

• Tutoring Services: Local or online tutoring sessions provide personalized help in addressing specific challenges.
• Study Groups: Joining a study group allows students to share knowledge, ask questions, and collaborate on solving problems.
• Textbooks and Practice Guides: Physical or digital textbooks often include additional problems and solutions for extra practice.

Using these resources in combination can provide a well-rounded approach to mastering new concepts and enhancing problem-solving skills. Whether through interactive tools or one-on-one tutoring, the availability of support ensures that every student has the opportunity to succeed and grow academically.

Improving Math Skills with Practice

Regular practice is one of the most effective ways to enhance problem-solving abilities and develop a deeper understanding of key concepts. Repetition helps reinforce knowledge, making it easier to recall and apply when needed. By consistently working through problems, students can improve both their speed and accuracy, gradually mastering even the most complex challenges.

Practicing regularly not only strengthens existing knowledge but also builds confidence. The more students engage with problems, the more they begin to recognize patterns and develop strategies to tackle unfamiliar situations. As a result, consistent practice leads to greater retention and a more intuitive grasp of the subject matter.

Effective Practice Strategies

• Start with Simple Problems: Begin with basic problems to build confidence before tackling more challenging ones.
• Focus on Weak Areas: Identify topics or skills that need improvement and spend extra time working on them.
• Set Time Limits: Practice solving problems within a set time frame to improve speed and efficiency.
• Use Variety of Resources: Practice with textbooks, online quizzes, or interactive tools to keep learning fresh and engaging.

Tracking Progress and Staying Consistent

• Review Mistakes: After completing practice problems, go over any mistakes made to understand why the error occurred and learn from it.
• Track Improvement: Keep a record of progress by noting down the types of problems completed, the time spent, and areas where improvement is needed.
• Stay Consistent: Establish a regular study routine to ensure continuous improvement and avoid procrastination.

By making practice a regular habit, students can gradually build a strong foundation, develop effective problem-solving techniques, and increase their overall proficiency. With dedication and persistence, the skills acquired through consistent practice will translate into improved performance and deeper understanding.

How Big Ideas Math Helps Teachers

Effective teaching requires a well-structured approach, clear materials, and resources that support both instruction and student learning. A comprehensive system can provide educators with tools to enhance their lessons, monitor student progress, and adapt to individual needs. With the right support, teachers can create a more engaging and productive classroom environment.

This educational system offers a range of features designed to help teachers manage their lessons and guide students toward success. By streamlining lesson plans, offering clear explanations, and providing valuable feedback mechanisms, teachers can focus more on facilitating learning and less on administrative tasks.

Key Benefits for Teachers

• Structured Lesson Plans: Teachers can rely on well-organized lesson structures that save time and ensure that key concepts are effectively covered.
• Student Progress Tracking: Built-in tools allow teachers to easily monitor student performance and identify areas that need additional attention.
• Interactive Resources: Access to various digital tools, exercises, and activities engages students while reinforcing important skills.
• Personalized Instruction: Resources and assignments can be adapted to cater to the needs of individual students, ensuring personalized learning paths.

Supporting Professional Development

• Ongoing Training: Teachers have access to professional development resources to enhance their teaching strategies and stay updated on best practices.
• Collaborative Community: The platform often provides opportunities for educators to collaborate, share strategies, and learn from each other.
• Resource Access: Teachers can access a wide variety of teaching materials, including assessments, worksheets, and activities, all tailored to reinforce key concepts.

By offering these tools and resources, this system helps educators enhance their teaching methods and foster an environment where students can thrive. With efficient lesson planning, progress monitoring, and the ability to provide targeted support, teachers are better equipped to meet the diverse needs of their students.

Online Resources for Big Ideas Math

In today’s digital age, online tools and resources are integral to enhancing learning experiences. These platforms offer interactive exercises, instructional videos, and additional support materials that help both students and teachers deepen their understanding of core subjects. The right set of online resources can make a significant difference in mastering concepts and improving academic performance.

For educators and learners looking to strengthen their knowledge, there are a variety of web-based platforms and tools available. These resources often include engaging activities, step-by-step solutions, and detailed explanations to support classroom learning and independent study. By incorporating these digital aids, users can access personalized learning experiences that cater to individual needs.

Key Features of Online Tools

• Interactive Lessons: Many platforms offer dynamic lessons that include visual aids, animations, and simulations to clarify complex concepts.
• Practice Problems: Online exercises and quizzes provide ample opportunities for students to practice and solidify their understanding.
• Instant Feedback: Immediate feedback on assignments helps learners correct mistakes and improve their skills in real time.
• Progress Tracking: Many digital platforms allow both students and instructors to monitor progress and identify areas needing improvement.

Popular Online Platforms

• Online Tutorials and Videos: Websites like Khan Academy and YouTube offer detailed lessons and tutorials on a wide variety of topics, providing additional explanations and examples.
• Educational Games: Platforms like IXL and Mathletics turn learning into a fun and engaging experience, offering game-like exercises to build skills.
• Collaborative Tools: Websites such as Google Classroom enable students and teachers to share resources, assignments, and feedback seamlessly.
• Digital Textbooks: Many publishers now provide digital versions of textbooks that include interactive features, enabling deeper engagement with the material.

These online resources offer valuable support to learners and teachers alike. With access to rich multimedia content, personalized learning paths, and various practice opportunities, students can build a strong foundation in key concepts while educators can enhance their teaching strategies. By taking full advantage of these digital tools, learners are better prepared to achieve academic success.

Using Big Ideas Math for Test Prep

Preparing for exams requires a comprehensive approach that includes reviewing key concepts, practicing problems, and honing problem-solving techniques. One effective method for test preparation is utilizing structured resources that offer targeted exercises and explanations. These platforms provide students with the tools they need to tackle a variety of question types and understand underlying principles.

By leveraging a variety of practice materials, learners can not only test their knowledge but also improve their ability to approach different problem types with confidence. These resources offer numerous examples, detailed step-by-step solutions, and tailored exercises that help reinforce learning and fill any knowledge gaps.

Effective Strategies for Test Prep

• Focused Practice: Regular practice with a variety of question types ensures familiarity with exam formats and helps identify areas requiring additional review.
• Step-by-Step Solutions: Breaking down complex problems into smaller, manageable steps helps develop a clear understanding of how to approach different problems efficiently.
• Timed Practice Tests: Simulating real test conditions by completing practice exams within a set time limit improves time management skills and builds confidence under pressure.
• Review and Reinforce: Going over incorrect answers and understanding why mistakes were made ensures better retention of concepts and reduces the likelihood of repeating errors.

How the Platform Supports Test Preparation

• Interactive Lessons: Each lesson offers interactive tools that help clarify difficult concepts, making it easier to grasp complex material.
• Customized Learning Paths: With personalized practice sessions, learners can focus on specific areas of weakness, ensuring a more efficient review process.
• Instant Feedback: Real-time feedback helps students quickly correct mistakes, reinforcing correct methods and preventing bad habits from forming.
• Progress Monitoring: Tracking progress allows learners to see how much they’ve improved and what areas need more focus before the exam.

Using these resources, students can engage in focused, productive study sessions that build both knowledge and confidence. With a well-rounded preparation strategy, learners will be better equipped to perform well in exams and demonstrate a solid understanding of the material.

Advanced Features of Big Ideas Math

Modern educational platforms offer various advanced tools designed to enhance the learning experience and provide students with in-depth support. These features help learners engage with the material on a deeper level, offering personalized learning paths, interactive exercises, and immediate feedback. By incorporating advanced functionalities, these resources ensure a more effective, customized approach to mastering concepts.

In addition to standard lessons and practice problems, these platforms offer a range of specialized tools to assist students in understanding complex topics. These advanced features go beyond traditional methods, helping users apply what they’ve learned to real-world situations and various types of questions.

Key Advanced Features

• Personalized Learning Paths: This feature adapts to each learner’s needs by adjusting the difficulty and type of exercises based on their performance, ensuring they stay challenged yet not overwhelmed.
• Interactive Simulations: Hands-on, interactive tools allow students to explore and visualize mathematical concepts, making abstract topics more concrete and easier to understand.
• Progress Tracking: Real-time progress tracking enables learners to see how they are improving over time and pinpoint areas where additional practice may be necessary.
• Instant Feedback: Immediate corrections and explanations help learners understand their mistakes and reinforce correct methods, fostering continuous improvement.

How These Features Enhance Learning

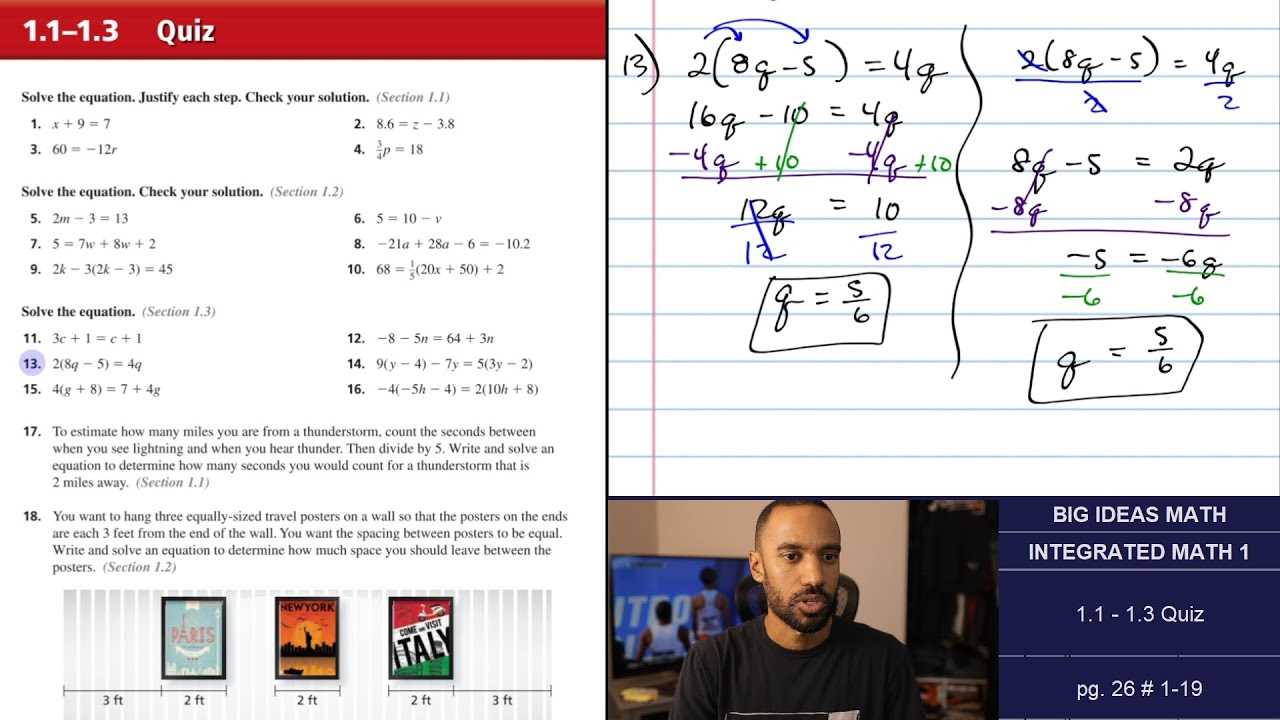
• Engagement: Interactive elements increase student engagement, making learning more dynamic and enjoyable.
• Retention: Personalized learning ensures that students spend more time on concepts they find challenging, improving retention and mastery of difficult topics.
• Efficient Learning: By focusing on the student’s individual progress, the platform ensures that learners do not waste time on concepts they already understand, allowing for more efficient use of study time.
• Confidence Building: Continuous feedback and progress reports provide reassurance to students, building confidence as they see their growth and improvement.

These advanced tools work together to create a more comprehensive and personalized learning experience. By integrating these features into their study routine, students can optimize their learning process and achieve a deeper understanding of complex concepts.