Big Ideas Math Red Accelerated Answers and Solutions
In this section, we explore essential methods and detailed explanations for tackling challenging concepts in advanced mathematics. This material aims to provide learners with structured approaches to problem-solving, helping them enhance their understanding of complex topics.
With a focus on building strong foundational skills, the content is designed to break down intricate problems into manageable steps. By following the outlined techniques, students can gain a deeper insight into the subject and improve their problem-solving abilities.
Mastery of these key ideas not only boosts performance but also fosters confidence in applying mathematical principles to real-world situations. This resource is tailored to guide learners through the process, offering both clarity and efficiency at every stage of learning.
Big Ideas Math Red Accelerated Answers
This section provides detailed solutions for complex topics in advanced coursework, focusing on a clear and systematic approach to tackling difficult problems. By offering step-by-step breakdowns, the content aims to guide students through the intricacies of each problem, allowing them to build confidence and mastery over the material.
Step-by-Step Problem Solving
To ensure a thorough understanding of the concepts, each problem is dissected into smaller, manageable parts. This method not only helps clarify the logic behind each solution but also ensures that learners can replicate the process independently. The explanations are designed to strengthen reasoning skills and enable students to apply similar techniques to future challenges.
Practical Application and Mastery
Focusing on real-world applications, this resource demonstrates how theoretical knowledge translates into practical solutions. By reinforcing core concepts and offering practical examples, students are better equipped to utilize these principles in various scenarios. The goal is to deepen their comprehension and enhance problem-solving abilities for long-term success.
Overview of Big Ideas Math Red
This section outlines the structure and core elements of the advanced learning curriculum designed for students tackling complex mathematical concepts. The program is tailored to build a strong foundation through comprehensive instruction and problem-solving techniques.
The curriculum focuses on providing clear explanations and well-structured exercises that gradually increase in difficulty. Students engage with a variety of topics, honing their skills to master both foundational and more intricate mathematical principles.
- Logical progression of concepts for steady learning
- Emphasis on hands-on practice and problem-solving
- Step-by-step guidance for tackling challenging problems
- Focus on real-world applications to reinforce learning
With this approach, students are not only prepared for exams but also equipped with the skills necessary for applying mathematical principles to everyday situations. This learning path encourages critical thinking, efficient problem-solving, and a deeper understanding of key concepts.
Key Concepts in Accelerated Math
This section highlights the core principles that underpin advanced coursework, providing a comprehensive understanding of essential topics. It focuses on strengthening key skills and deepening the learner’s ability to solve complex problems with precision.
Core Principles and Strategies
At the heart of this curriculum are foundational principles that are essential for tackling higher-level topics. Students are encouraged to develop a strong grasp of algebraic reasoning, geometric analysis, and functional relationships. These concepts are introduced progressively, ensuring that learners are well-equipped to build on them as they move forward.
Problem-Solving and Logical Thinking
Critical thinking plays a central role in the curriculum, helping students approach each challenge methodically. Problem-solving strategies are emphasized, with an emphasis on breaking down intricate questions into simpler, more manageable parts. By mastering these techniques, students are empowered to apply them to a variety of mathematical scenarios.
Understanding the Curriculum Structure
This section provides an in-depth look at the organization and framework of the learning program designed for advanced students. The structure is methodically arranged to ensure students gain a solid grasp of each topic before progressing to more complex material. With clear objectives at each stage, the curriculum promotes both conceptual understanding and practical application of skills.
Each unit is carefully structured, starting with fundamental concepts and gradually introducing more sophisticated ideas. Lessons build on prior knowledge, allowing learners to master key principles before moving on to higher-level topics. This logical flow ensures that no concept is overlooked, making the learning process seamless and coherent.
In addition, the curriculum integrates various types of exercises and assessments to reinforce learning. Students are encouraged to apply their knowledge in different contexts, enhancing their ability to solve real-world problems with the tools and techniques they’ve learned.
How to Approach Big Ideas Math
Approaching an advanced learning program requires careful planning and a strategic mindset. Success in tackling complex problems depends on the ability to break down concepts into manageable parts while maintaining a clear focus on the overall objective. A systematic approach ensures that each step builds on the last, reinforcing key principles and boosting confidence.
Start by understanding the foundation of each topic. Before diving into intricate problems, ensure a strong grasp of basic concepts. This will make more advanced material easier to digest. As you work through exercises, focus on developing logical reasoning and problem-solving strategies that can be applied to a variety of challenges.
Additionally, practice is crucial. Consistently working through problems, even if they seem repetitive, helps reinforce techniques and solidifies understanding. Don’t rush through exercises–take the time to carefully analyze each problem, apply the methods you’ve learned, and review your solutions for accuracy.
Solving Problems Step by Step
Approaching complex problems requires a structured method that breaks down each challenge into smaller, more manageable tasks. By following a systematic approach, students can tackle each part of the problem with confidence, ensuring that no detail is overlooked. This method not only simplifies the process but also enhances problem-solving skills that can be applied across various topics.
The first step in solving any problem is to thoroughly understand the question. Identify key elements and decide on the best approach before starting to solve. Once you have a plan, break the problem down into logical steps, focusing on one part at a time. This makes even the most difficult problems seem less intimidating.
Throughout the process, it’s important to check your work at each step to ensure accuracy. After completing each part, review it carefully before moving on to the next. This continuous evaluation helps to catch any mistakes early, saving time and effort in the long run. By staying organized and methodical, you can solve problems more effectively and efficiently.
Exploring Algebraic Foundations
Algebra forms the cornerstone of higher mathematics, providing the tools needed to solve a wide range of problems. Understanding its basic principles is essential for tackling more advanced topics. This section dives into the fundamental concepts that underpin algebra, allowing students to build a solid foundation for future learning.
- Variables and constants: Understanding their role in mathematical expressions
- Equations: Solving for unknowns through systematic methods
- Expressions: Simplifying and manipulating algebraic formulas
- Linear relationships: Interpreting and solving linear equations
By focusing on these key elements, learners can develop the skills necessary to approach more complex algebraic challenges with ease. Mastering these foundational concepts enables students to confidently move forward and apply algebra in real-world situations, making the process of learning both practical and rewarding.
Geometry Insights in Red Accelerated
Geometry plays a crucial role in developing spatial reasoning and understanding the properties of shapes and figures. This section focuses on key geometric concepts, exploring the relationships between points, lines, angles, and surfaces. A strong grasp of geometry is essential for solving real-world problems and advancing to more complex mathematical topics.
| Concept | Description |
|---|---|
| Angles | Understanding different types of angles and their properties |
| Triangles | Exploring the properties of various triangle types and their applications |
| Circles | Learning about the relationships between radii, diameters, and circumferences |
| Symmetry | Examining reflective and rotational symmetry in shapes |
By mastering these core principles, students develop a deeper understanding of how geometric figures relate to each other and to the world around them. The ability to visualize and solve geometric problems enhances overall mathematical proficiency and prepares learners for future challenges in the field.
Practice Questions for Mastery
To truly master any subject, consistent practice is essential. This section provides a range of questions designed to reinforce key concepts and sharpen problem-solving skills. Regularly working through these problems will not only strengthen understanding but also increase confidence in applying learned techniques to real-world scenarios.
Problem Sets for Skill Development
- Simple equations: Practice solving linear and quadratic expressions
- Word problems: Apply learned concepts to real-world situations
- Geometry challenges: Solve for angles, areas, and perimeters
- Advanced topics: Explore higher-level algebraic and geometric problems
Tips for Effective Practice
- Break down each problem into smaller steps to ensure clarity
- Review solutions carefully to understand the reasoning behind each step
- Practice regularly, focusing on areas of difficulty
- Challenge yourself with progressively harder problems to build mastery
By dedicating time to these exercises, students can improve their problem-solving speed and accuracy, leading to a deeper understanding and mastery of key concepts. Regular practice is the most effective way to build long-term skills and achieve academic success.
Tips for Effective Study Strategies
Developing a strong study routine is crucial for mastering complex subjects. Effective study strategies can help students retain information better, improve problem-solving skills, and make learning more enjoyable. The key is consistency and the application of various techniques that cater to different learning styles.
Organizing Your Study Time

- Create a study schedule: Plan your study sessions in advance and allocate specific times for each topic.
- Set realistic goals: Break down your learning into manageable tasks to avoid feeling overwhelmed.
- Use breaks wisely: Take short breaks to recharge and maintain focus during longer study periods.
- Review regularly: Spend a few minutes each day revisiting key concepts to strengthen retention.
Active Learning Techniques
- Practice regularly: Work through problems and exercises to reinforce what you’ve learned.
- Teach someone else: Explaining concepts to others helps solidify your understanding.
- Use mnemonic devices: Create memory aids for complex information to make it easier to recall.
- Stay organized: Keep notes and materials tidy so you can easily access them when needed.
By incorporating these strategies into your routine, you can maximize your study efficiency and boost your performance. Consistency, active engagement, and smart time management are essential components of a successful learning journey.
Common Mistakes to Avoid
While studying any subject, it’s important to be aware of common errors that can hinder progress. These mistakes often stem from misunderstandings or oversights, but they can be easily avoided with the right approach. Recognizing and addressing these issues early will help improve comprehension and performance.
Frequent Pitfalls
- Rushing through problems: Taking shortcuts can lead to careless mistakes and missed details.
- Skipping steps: Omitting steps in calculations or problem-solving can result in incorrect answers.
- Neglecting to review work: Failing to check for errors or recheck solutions can lead to avoidable mistakes.
- Not understanding the underlying concepts: Memorizing formulas without understanding their application leads to confusion in more complex problems.
How to Avoid These Errors
- Take your time: Carefully work through each problem and ensure you understand every step.
- Double-check your work: Review each calculation or answer before moving on to the next task.
- Focus on concepts: Spend time understanding the reasoning behind the formulas and procedures you use.
- Practice regularly: Reinforce your understanding through consistent practice to avoid misunderstandings.
By avoiding these common mistakes and implementing effective strategies, you can enhance your learning process and achieve better results. Developing a mindful approach to studying is key to mastering any subject.
Understanding Word Problems Efficiently
Word problems often pose a unique challenge as they require translating real-life situations into mathematical equations. The key to solving them lies in a clear understanding of the context and the ability to extract relevant information. By breaking down the problem into smaller, manageable parts, students can tackle each component systematically and arrive at the correct solution.
Steps for Solving Word Problems
- Read carefully: Take time to understand what the problem is asking. Identify important details such as numbers, units, and relationships.
- Identify the question: Focus on what you are being asked to find, whether it’s a total, a difference, or another value.
- Translate words into equations: Convert the word problem into mathematical expressions or equations using the given information.
- Work through systematically: Solve the equation step by step, ensuring each part is clear and logical.
- Double-check your answer: Once you find a solution, review the steps and check that it makes sense in the context of the problem.
Common Techniques to Apply
- Draw diagrams or models: Visual representations can help clarify the problem, especially in geometry or rate-related questions.
- Use estimation: Make rough calculations to check if the solution is reasonable.
- Look for patterns: Recognize patterns or relationships that can simplify the process of solving the problem.
By following these steps and applying these techniques, students can improve their ability to solve word problems efficiently and accurately. Consistent practice and a systematic approach will lead to greater confidence in tackling more complex problems over time.
Advanced Techniques for Problem Solving
As you progress in your studies, tackling more complex problems becomes a key aspect of mastering the subject. Advanced problem-solving requires not only a solid understanding of the fundamental principles but also the ability to apply creative and strategic approaches. Developing a toolkit of techniques allows you to tackle challenging problems with confidence and precision.
One such technique is the method of substitution, which can be extremely helpful when working with systems of equations. By solving one equation for a variable and substituting it into another, you can reduce the complexity of the system and isolate the unknowns more easily. This method is particularly useful when direct elimination seems cumbersome.
Another advanced approach is working backward, which is often employed in problems where the final answer is given, and the steps leading up to it must be discovered. This strategy allows you to reverse-engineer the problem, often revealing shortcuts and simplifying the solution process.
Graphical Methods
When algebraic techniques become cumbersome, graphical methods can provide a clear visualization of the problem. Plotting equations or inequalities on a coordinate plane allows you to see relationships between variables, identify intersections, and understand the problem more intuitively. This approach is especially helpful in solving optimization problems or when working with geometric figures.
Working with Approximations
Approximation techniques are another valuable tool, particularly when an exact solution is difficult or impossible to obtain. Rounding numbers or using estimation methods can provide sufficiently accurate results for many real-world problems, saving time and effort when precision is not a critical factor.
By expanding your problem-solving techniques and practicing them regularly, you will be equipped to handle a wider range of challenges with efficiency. Mastery of these advanced methods not only enhances your understanding but also prepares you for more complex academic and real-world applications.
Using Online Resources for Assistance
In today’s digital age, there is a wealth of online tools and platforms that can significantly enhance learning and problem-solving. These resources provide valuable support, whether you need extra practice, clarification on a difficult concept, or a different approach to a problem. Leveraging the power of the internet can help reinforce your understanding and improve your skills.
Interactive platforms and forums are excellent for engaging with both instructors and peers. Websites offering interactive lessons, quizzes, and exercises enable you to learn at your own pace while receiving instant feedback. Moreover, many forums provide a space for students to ask questions and discuss challenges, allowing for collaborative learning and the sharing of solutions.
Video tutorials are another great resource for visual learners. YouTube, educational websites, and online course platforms host an abundance of step-by-step guides that break down complex problems and walk you through the solution process. These videos can offer alternative explanations that might resonate better than traditional textbook methods.
Math Problem Solvers
For those struggling with specific questions, online problem solvers can be a game-changer. Many websites allow you to input a problem and receive a detailed solution, often with accompanying explanations of each step. While these tools are useful for verification and learning, it’s important to understand the underlying principles rather than relying solely on automated solutions.
Study Apps and Tools
Additionally, many mobile apps are designed to help with organization and study planning. These apps can help you track your progress, set goals, and remind you of key concepts, making it easier to stay on top of your learning. Some apps even use gamification techniques to keep you motivated while improving your problem-solving abilities.
By using these online resources wisely, students can access a vast range of support, deepen their understanding, and refine their problem-solving skills. However, it is crucial to use them as supplements to your core study efforts, ensuring that you remain engaged and actively involved in the learning process.
Real-World Applications of Concepts
The knowledge and techniques learned in the classroom have wide-reaching implications beyond academic settings. Understanding fundamental principles not only strengthens problem-solving abilities but also enhances practical decision-making in everyday life. These concepts can be applied across a variety of fields, from business to technology, engineering, and beyond.
In finance and budgeting, for example, understanding numerical relationships and proportional reasoning helps individuals manage personal finances, make investment decisions, and calculate interest rates. Whether it’s planning a household budget or analyzing the cost-effectiveness of an investment, these concepts play a crucial role in managing money wisely.
In architecture and design, geometric principles are essential for creating safe and aesthetically pleasing structures. Architects and engineers use mathematical concepts like angles, area, and volume to design buildings and bridges, ensuring stability and functionality. These same concepts are used in urban planning and construction projects to optimize space and resources.
Science and Technology
In scientific research, accurate measurements and data analysis are essential for drawing conclusions and making predictions. For example, in physics and chemistry, formulas and equations are used to calculate forces, chemical reactions, and energy transfer. Understanding these principles can lead to groundbreaking innovations and improvements in technology, from medical devices to renewable energy sources.
Technology and Computing
In the world of technology, algorithms and logic are applied every day. Software development relies heavily on algorithms, which are mathematical procedures used to solve problems and process information. Whether building an app or developing an operating system, the ability to apply logical reasoning and computational techniques is foundational in creating efficient solutions.
The ability to connect classroom concepts with real-world situations fosters a deeper appreciation for learning. It encourages students to see the value of what they are studying and motivates them to explore how these ideas can be used to solve practical problems in various industries.
Reviewing Key Answers for Success
To achieve mastery in any subject, reviewing key solutions and strategies is essential. By revisiting core concepts and practicing how to apply them effectively, students can solidify their understanding and approach complex problems with confidence. This section emphasizes the importance of carefully reviewing correct responses and identifying the thought processes behind them.
Regular practice and review of the correct methods and results help reinforce the learning process. Here are some essential strategies to follow when reviewing key solutions:
- Break down each step: Understand why each decision was made in solving a problem, not just the final answer. Identify the reasoning behind each calculation or logical step to ensure a deeper grasp of the material.
- Identify patterns: Review multiple problems with similar structures to recognize common techniques or formulas used to solve them. This will allow you to apply the same strategies to new problems in the future.
- Analyze mistakes: Mistakes are an inevitable part of the learning process. By reviewing errors, you can pinpoint where your understanding or approach went wrong and take corrective steps to avoid repeating those mistakes.
- Reinforce key concepts: Focus on the foundational principles that recur throughout the material. Understanding these core ideas will allow you to build more complex knowledge and improve problem-solving skills.
Active Learning and Reflection
Rather than passively reviewing solutions, active engagement with the material enhances retention and comprehension. Take the time to ask questions like: Why does this approach work? What if I tried a different method? Actively reflecting on these answers will foster critical thinking and a deeper understanding.
Using Resources to Support Review
Utilize available resources such as textbooks, online tutorials, and practice problems to revisit difficult concepts. These tools provide alternative explanations and different problem sets that can help clarify difficult material. By consistently practicing and reviewing key solutions, success will be more attainable and sustainable in the long run.
Preparing for Tests and Assessments
Effective preparation is key to performing well on any test or assessment. It goes beyond simply reviewing content; it involves developing a strategy to manage time, strengthen weak areas, and approach questions with confidence. This section outlines methods to help students approach exam preparation efficiently and maximize their performance.
Organizing study sessions is essential for effective test preparation. Planning ahead and breaking down the material into manageable sections allows for focused, organized review sessions. Additionally, incorporating different learning techniques, such as active recall and spaced repetition, can greatly improve retention.
Creating a Study Schedule

A well-structured study schedule ensures that you cover all the necessary material before the assessment. Here’s a simple plan to help you organize your time:
| Time Frame | Focus Areas | Action Steps |
|---|---|---|
| 1 Week Before | General review of key concepts | Identify weak areas and create study plan |
| 3-4 Days Before | Practice problems and sample tests | Focus on applying concepts to solve problems |
| 1 Day Before | Final review and relaxation | Go over notes, but avoid cramming |
Strategies for Test-Taking
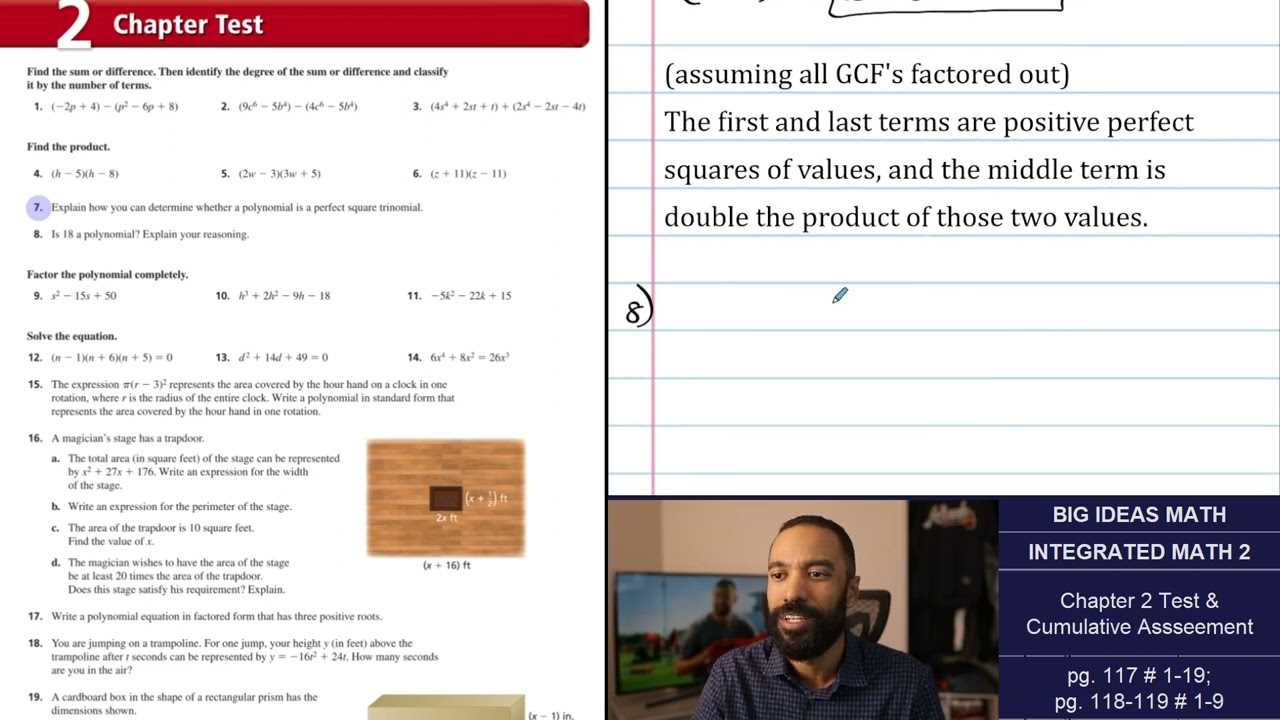
On the day of the assessment, employ techniques that help you stay calm and perform well:
- Read all instructions carefully: Make sure you understand the format of the questions and any special instructions before starting.
- Manage your time: Pace yourself throughout the test. Allocate time for each section and leave some buffer for reviewing your answers.
- Answer easy questions first: Quickly address questions you are confident in, then return to more challenging ones.
- Stay calm: If you feel stuck, take a deep breath and refocus. Clear thinking helps you recall information more effectively.
By planning ahead and following these strategies, you can approach tests and assessments with confidence and improve your chances of success.