Eureka Math Lesson 15 Homework Answers and Solutions

In this section, we will explore the critical principles and methods necessary to solve complex problems efficiently. By breaking down each task into manageable steps, the process becomes clearer, making it easier to apply these techniques to similar challenges.
Whether you’re struggling with specific problem types or just looking for a better understanding of the material, this guide will offer clear, step-by-step explanations. You’ll learn how to approach each problem with confidence, using proven strategies to reach the correct solutions.
By the end, you’ll have a better grasp of the concepts involved and be equipped to tackle any related exercises. The key to mastering these topics lies in practice and recognizing patterns, and this resource will help you achieve both.
Understanding Key Solutions and Approaches
This section is dedicated to providing a comprehensive breakdown of the strategies and methods needed to complete challenging problems. It aims to guide you through each step, ensuring clarity and precision in applying the concepts to similar tasks. By following these structured approaches, you will enhance your ability to solve a variety of problems with confidence.
Step-by-Step Breakdown
Each problem in this section is approached methodically, ensuring that each concept is fully explained. You will learn to identify key information, choose the correct methods, and apply them effectively. Clear examples and detailed explanations will support your understanding of how to handle similar problems in the future.
Common Pitfalls and How to Avoid Them
Many students encounter similar difficulties when facing these challenges. Recognizing common mistakes can significantly improve your accuracy and efficiency. This section highlights frequent errors and offers tips on how to avoid them, ensuring that your solutions are correct and well-structured.
Key Concepts for Lesson 15
This section focuses on the essential principles and skills necessary for mastering the material in this part of the curriculum. Understanding these core ideas is crucial for solving problems efficiently and accurately. By familiarizing yourself with the foundational concepts, you will be better prepared to tackle the exercises and apply these skills in future tasks.
Understanding Operations with Fractions
One of the main concepts in this section involves working with fractions. You will learn to perform various operations such as addition, subtraction, multiplication, and division. It’s important to recognize the relationship between the numerator and denominator and understand how to simplify fractions when necessary. Mastery of these operations is essential for solving more complex problems later on.
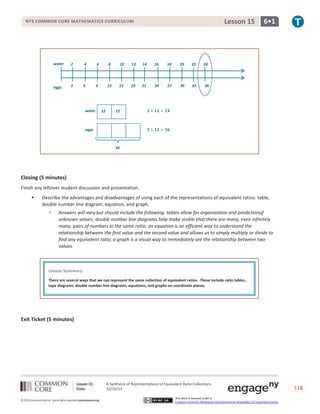
Visualizing Solutions with Models
Another key element in this section is using visual models to represent mathematical relationships. Whether through number lines, diagrams, or bar models, these tools help make abstract concepts more tangible. By visualizing solutions, you can gain a deeper understanding of the problem-solving process and ensure that you’re applying the correct strategies.
Step-by-Step Solutions
This section provides detailed, clear instructions on how to solve each problem from start to finish. By following the step-by-step process, you will be able to grasp the method behind each solution and gain the skills necessary to solve similar problems independently. These solutions are designed to make even the most complex tasks more manageable.
Solution Breakdown for Problem 1
In this example, we will break down the problem into smaller, more understandable parts. Follow each step carefully to see how the solution is formed.
| Step | Action | Explanation |
|---|---|---|
| 1 | Identify the key numbers | Look for the given values in the problem and write them down. |
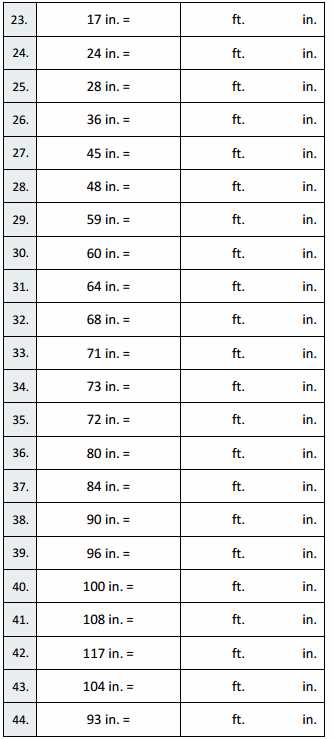
| 2 | Choose the correct operation | Decide whether you need to add, subtract, multiply, or divide based on the instructions. |
| 3 | Perform the calculation | Carry out the operation carefully, ensuring that all numbers are correctly placed. |
| 4 | Check the result | Verify that the outcome makes sense in the context of the problem. |
Solution Breakdown for Problem 2

This problem requires a different approach. The same process can be applied, but with adjustments based on the specific requirements of the task.
| Step | Action | Explanation |
|---|---|---|
| 1 | Read the problem carefully | Identify what is being asked and highlight important details. |
| 2 | Organize the information | Arrange the values and operations in a logical order to make the calculations easier. |
| 3 | Execute the calculation | Use the proper mathematical procedure to get the correct result. |
| 4 | Double-check your answer | Ensure all steps were followed accurately and the answer fits the context. |
Common Mistakes in Lesson 15
While working through the material, students often encounter similar challenges that can lead to mistakes. Recognizing and understanding these errors is key to improving your problem-solving skills. By learning from common pitfalls, you can avoid them in future tasks and approach similar exercises with more confidence.
One frequent mistake involves misinterpreting the instructions, especially when dealing with fraction operations or multi-step problems. It’s easy to overlook the importance of the order in which operations should be performed, leading to incorrect results. Another common error occurs when students forget to simplify fractions or apply improper techniques for combining values.
Another issue arises when numbers are mistakenly swapped or miscalculated during addition or subtraction. This often happens when the student rushes through the problem without carefully reviewing the process step-by-step. Taking time to double-check your work can help you catch these errors before finalizing your answer.
How to Approach Word Problems
Word problems can often seem intimidating due to the amount of information they contain. However, with a clear strategy, you can break down the problem into smaller, more manageable parts. The key is to approach them step-by-step, carefully analyzing the given information before jumping into calculations.
Here are some effective steps to follow when tackling word problems:
- Read the problem carefully: Make sure you understand the scenario. Identify important details like numbers, relationships, and the question being asked.
- Highlight key information: Underline or note the key values and terms that will help guide your solution.
- Identify the operations needed: Decide whether the problem involves addition, subtraction, multiplication, or division. Think about what the question is asking and choose the right approach.
- Set up the equation: Translate the information into a mathematical expression or equation that you can solve.
- Perform the calculations: Carefully work through the steps, ensuring each operation is executed correctly.
- Double-check your work: Go back over your steps to make sure everything makes sense and the calculations are correct.
By following these steps, you can gain more confidence in solving word problems and improve your overall problem-solving skills.
Understanding Fraction Operations
Working with fractions involves a set of fundamental operations that allow you to combine or compare parts of a whole. These operations–addition, subtraction, multiplication, and division–each have their own rules that are essential to mastering fraction-based problems. Knowing how to manipulate fractions correctly is crucial for solving many types of exercises.
When adding or subtracting fractions, it’s important to have a common denominator. Without it, you cannot combine the numerators directly. For multiplication, the process is simpler: multiply the numerators and denominators, then simplify the result if possible. Division of fractions requires flipping the second fraction (reciprocal) and then multiplying. Each operation follows a specific pattern, and mastering these patterns ensures accuracy in solving problems involving fractions.
By practicing these operations and understanding when and how to apply each rule, you will build confidence and be able to tackle more complex problems involving fractions with ease.
Helpful Tips for Solving Equations
Solving equations can seem challenging at first, but with the right approach, you can simplify the process. The key is to break down the equation into smaller, manageable steps and apply consistent methods. Understanding the rules and knowing when to apply different strategies will help you find the solution more efficiently.
Start by Isolating the Variable
One of the most important techniques in solving equations is isolating the variable. Begin by moving all terms involving the unknown to one side of the equation, and constants to the other. This simplifies the equation, making it easier to solve. Remember to perform the same operation on both sides to maintain balance.
Check Your Work
After solving, always substitute your solution back into the original equation to verify its accuracy. This ensures that the value you’ve found is correct. Double-checking your work helps catch small mistakes and confirms that the solution is indeed valid.
How to Use Models in Homework
Using visual representations or models can be an effective strategy when working through exercises. These tools help clarify complex concepts and make abstract ideas more tangible. By turning problems into visual forms, you can better understand relationships between numbers and solve tasks step-by-step.
Types of Models to Use

There are several types of models that can assist in solving problems. Each has its own advantages, depending on the type of problem you are working on:
- Bar Models: These are helpful for understanding part-to-whole relationships, particularly in addition, subtraction, and fraction problems.
- Number Lines: Great for visualizing operations involving whole numbers and fractions. They are especially useful for understanding the positioning of numbers and the difference between values.
- Area Models: Often used for multiplication and division, these models represent numbers in grid form to illustrate how numbers are split or combined.
Benefits of Using Models
Incorporating models into your process offers several benefits:
- Clarity: They provide a clear, visual way to break down and understand complex problems.
- Accuracy: Models reduce the risk of making errors by providing a structured method to follow.
- Confidence: By seeing the problem in a new light, you can feel more confident in your solutions and understanding.
Whether you’re solving a basic equation or tackling a more intricate problem, using models can greatly improve your problem-solving process and enhance your learning experience.
Guided Practice for Lesson 15
Guided practice is an essential part of the learning process, offering a structured way to tackle problems with expert support. This method allows students to approach challenging tasks with step-by-step guidance, helping them build confidence and solidify their understanding. By walking through problems together, students gain a deeper insight into concepts and techniques, ensuring they are well-prepared for independent work.
Step-by-Step Problem Solving
In guided practice, the instructor or a resource provides a clear, methodical approach to solving each problem. This allows students to understand the reasoning behind each step and how to apply the same methods to similar problems. It’s important to focus on both the process and the solution, as understanding the steps is just as critical as arriving at the correct answer.
Practice with Immediate Feedback
Another key benefit of guided practice is the opportunity to receive immediate feedback. If a student makes an error or misses a step, they can quickly correct it with the help of a mentor. This real-time assistance prevents misunderstandings from taking root and helps reinforce correct techniques, leading to better retention and improved problem-solving skills.
Mastering Number Lines in Math
Number lines are a powerful visual tool for understanding numerical relationships and operations. They provide a clear representation of numbers and their relative positions, making complex concepts easier to grasp. By mastering the use of number lines, students can improve their understanding of addition, subtraction, fractions, and even negative numbers.
Using Number Lines for Basic Operations
Number lines are particularly useful for visualizing simple operations such as addition and subtraction. By moving along the line, students can see how numbers increase or decrease. For example, to solve an addition problem, you can start at the first number and move forward by the second number of the equation, visually counting the steps.
Working with Fractions and Decimals
Number lines also help in understanding fractions and decimals. These lines can be divided into smaller intervals, allowing students to place fractions or decimals precisely between whole numbers. This method helps in comparing values and understanding their relationships in a concrete way, making abstract concepts like fraction addition or subtraction more tangible.
Clarifying Decimal Place Values
Understanding decimal place values is crucial for working with numbers that fall between whole units. The position of each digit in a decimal number determines its value, and knowing how to read and interpret these values can help in performing precise calculations. By mastering decimal place values, students can easily work with fractions, percentages, and operations involving decimals.
Identifying Decimal Places
Each digit to the right of the decimal point represents a specific fraction of a whole. The first digit after the decimal point is in the tenths place, the next is in the hundredths place, and so on. By understanding these place values, you can quickly determine the value of any decimal number. For instance, in the number 3.74, the 7 is in the tenths place, and the 4 is in the hundredths place.
Using Place Values for Calculation
Knowing the decimal place values is essential for performing accurate calculations, especially when adding, subtracting, multiplying, or dividing decimal numbers. When adding decimals, align the decimal points correctly to ensure that each digit is in the right place. This will help maintain the accuracy of the results and prevent errors in complex operations.
Problem-Solving Strategies Explained
Effective problem-solving requires more than just applying formulas; it involves using systematic approaches to break down and understand the task at hand. A clear strategy helps in organizing thoughts, identifying key elements of the problem, and finding the most efficient solution. Developing strong problem-solving skills empowers students to tackle complex challenges with confidence and clarity.
Common Problem-Solving Approaches
There are several strategies that can be applied to various types of problems. Below is a table outlining some of the most effective methods and how they can be used in different scenarios:
| Strategy | Description | Best for |
|---|---|---|
| Understanding the Problem | Carefully read and interpret the problem to make sure all aspects are clear before proceeding. | Any problem where the instructions or given information might be unclear. |
| Working Backwards | Start from the desired result and reverse the steps to reach the starting point. | Solving equations, puzzles, or tasks with multiple possible solutions. |
| Using Visuals | Create diagrams, graphs, or charts to help visualize the relationships between different elements of the problem. | Problems involving spatial relationships, comparisons, or multiple data points. |
| Breaking it Down | Divide a large problem into smaller, more manageable parts and tackle each part individually. | Complex word problems or tasks that involve multiple steps or concepts. |
By familiarizing oneself with these approaches and practicing them regularly, problem-solving becomes a more intuitive and effective process. Whether you’re working through a simple equation or solving a more intricate puzzle, having a clear strategy in mind can make all the difference in achieving the right solution.
Reviewing Key Terms in Lesson 15
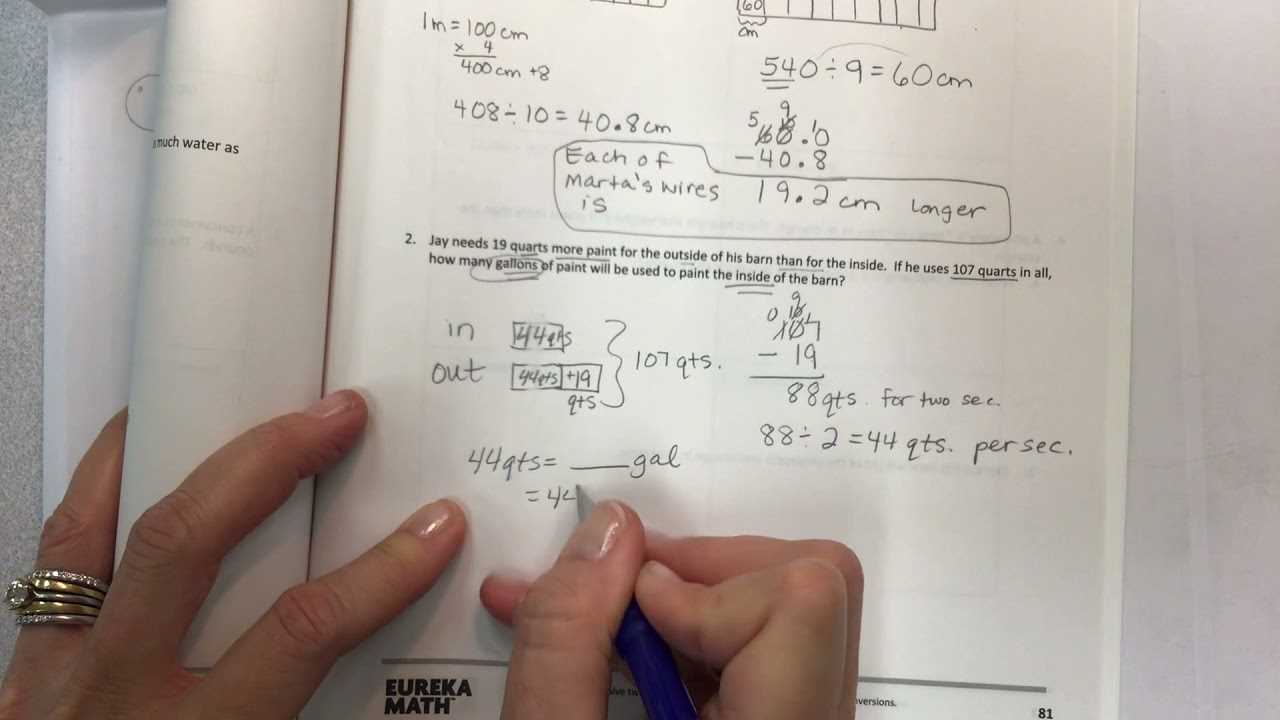
Understanding key terms is essential for mastering any subject, as they provide the foundation for more complex concepts. In this section, we will explore important vocabulary that plays a crucial role in grasping the material. By reviewing and familiarizing yourself with these terms, you will be better equipped to solve problems and apply the knowledge effectively.
Important Terms to Remember
Each topic introduces specific terminology that helps define the operations and relationships involved. Below is a list of key terms and their meanings:
- Variable: A symbol, often a letter, used to represent a number or value in an equation or expression.
- Equation: A mathematical statement that asserts the equality of two expressions, typically involving variables.
- Expression: A combination of numbers, variables, and operators (such as +, -, *, /) that represents a value.
- Solution: The value or set of values that satisfy an equation or inequality.
- Fraction: A way of expressing parts of a whole, typically written as a numerator over a denominator (e.g., 1/2, 3/4).
Using Key Terms Effectively
Mastering these terms allows you to approach problems with a clearer understanding. For example, recognizing when an expression should be simplified or when to find a solution to an equation makes it easier to solve problems step by step. As you progress, these terms will become second nature, helping you to communicate complex ideas with clarity and precision.
How to Check Your Answers
Verifying your results is an essential step in problem-solving. By checking your work, you ensure accuracy and identify any possible mistakes. This process involves using different strategies to confirm that your solution is correct and that all steps were followed properly.
Steps for Verifying Your Work
Here are some effective methods to check your results:
- Review Each Step: Go through the entire solution process again to make sure every operation was performed correctly and in the right order.
- Check for Consistency: Ensure that your final result makes sense with the context of the problem. If your solution is drastically different from your expectations, recheck your steps.
- Reverse Operations: If applicable, perform the inverse operation to see if it leads back to the original problem or equation.
- Estimate the Result: For certain problems, you can approximate your result and compare it to the exact solution. This is especially helpful in checking for any obvious errors.
- Use Different Methods: If possible, try solving the problem in a different way to see if you arrive at the same conclusion.
Tools for Double-Checking Solutions
Sometimes using additional tools or resources can help ensure accuracy:
- Calculators: Utilize a calculator for operations involving large numbers or complex calculations to verify your result.
- Online Resources: Websites, apps, or even peer assistance can be valuable in cross-referencing solutions and confirming your answer.
- Self-Assessment: Take a moment to reflect on whether the solution logically fits the problem and its conditions.
By implementing these strategies, you can confidently confirm the correctness of your work and improve your problem-solving skills.
Common Struggles in Lesson 15
Throughout the process of learning and solving various problems, there are often specific challenges that students encounter. These struggles can arise from a variety of factors, including confusion with concepts, difficulty in applying techniques, or simple oversight in calculations. Recognizing these common issues is key to addressing them effectively and improving overall understanding.
Some students may find it tricky to grasp certain foundational concepts that are critical for success in more complex problems. It is also common to encounter difficulties when trying to implement these ideas into practical solutions. The following are some of the typical challenges faced during this part of the curriculum.
1. Difficulty with Fraction Operations: Many students struggle with the manipulation of fractions, especially when adding, subtracting, or multiplying them. These operations require careful handling of numerators and denominators, which can lead to confusion if not understood properly.
2. Misunderstanding Word Problems: Interpreting word problems can often be a significant hurdle. Students may have trouble translating the information provided into mathematical expressions or equations, which can lead to mistakes or incomplete solutions.
3. Lack of Confidence with Negative Numbers: Negative values can create confusion, especially when they are involved in addition or subtraction. Understanding how negative numbers interact with others is essential to solving problems correctly.
4. Not Following the Order of Operations: The order in which mathematical operations must be performed can be tricky to remember, especially when dealing with multiple operations in one problem. Missing a step or performing operations in the wrong sequence can lead to incorrect results.
5. Overlooking Small Mistakes: In many cases, students overlook minor errors in calculation, such as misplacing a decimal point or incorrectly simplifying fractions. These mistakes can compound, leading to incorrect answers that seem difficult to identify and correct.
By identifying these common struggles, students can take proactive steps to overcome them, either through additional practice, seeking clarification, or applying helpful strategies. Understanding these typical issues will ultimately help build stronger problem-solving skills and ensure a deeper understanding of the material.
Using Resources for Extra Help
When tackling difficult topics, having access to additional resources can be a game-changer. These resources not only offer alternative explanations but also provide practice and support tailored to the specific challenges you may face. Whether you’re looking to clarify a concept or reinforce your understanding, the right tools can make all the difference in mastering the material.
There are several ways to seek help beyond classroom instruction, and it’s important to know where to look for the best assistance. By utilizing various resources, you can get the extra support needed to succeed and feel more confident with your work.
Online Platforms and Videos
Online tutorials and videos are invaluable for visual learners. They can offer step-by-step demonstrations, breakdowns of complicated concepts, and real-life examples that make understanding easier. Websites like Khan Academy, YouTube, and educational blogs provide free access to expert-led content that explains difficult topics in detail.
Peer Study Groups
Collaborating with classmates can also be an effective way to grasp complex material. Study groups allow for discussions, exchange of ideas, and the opportunity to learn from others’ perspectives. Sometimes, explaining a topic to someone else can help reinforce your own understanding.
Teacher and Tutor Support
If you find yourself struggling, don’t hesitate to reach out to your teacher or a tutor for additional guidance. Teachers often offer office hours or after-class support sessions where they can clarify difficult concepts. Tutors, whether in-person or online, can provide personalized attention and targeted help on areas where you need the most improvement.
Practice Worksheets and Apps
- Worksheets: These are great for reinforcing what you’ve learned by providing extra practice problems to solve.
- Apps: Educational apps, such as Quizlet or Photomath, offer practice problems, tutorials, and interactive tools to help visualize problems and solutions.
Utilizing a combination of these resources can greatly improve your grasp of challenging topics. By seeking help when needed and using all available tools, you’ll be better equipped to approach and solve problems confidently.