Eureka Math Lesson 17 Answers Explained

In this section, we will explore important concepts and provide detailed explanations for a set of challenging problems. With clear, structured steps, you will be able to understand the material more effectively and apply the knowledge to solve similar exercises.

By breaking down the problems into manageable parts, we aim to help you build a strong foundation. You will also find useful tips and methods that can assist in navigating through the more complex aspects of the exercises.

Through practical examples and insightful solutions, this guide is designed to enhance your comprehension and enable you to confidently tackle each problem. Mastering these steps is essential for progressing in your studies and achieving success.

Key Solutions and Explanations

This section provides detailed solutions for the set of problems presented in the previous topics. By breaking down each question into clear, actionable steps, you will gain a deeper understanding of the key principles and improve your problem-solving skills. The explanations are designed to guide you through complex ideas and provide you with practical approaches to tackle similar challenges in the future.

Step-by-Step Approach

To help you fully grasp the material, we will walk you through each problem, highlighting important steps along the way. Focus on understanding the process behind each solution, not just the final answer. This method will allow you to apply these techniques independently on other exercises.

• Start by identifying the main concept involved.
• Break the problem down into smaller parts for easier handling.
• Use logical reasoning and mathematical principles to connect the parts.
• Double-check your work to ensure accuracy before finalizing the solution.

Common Problem Types

Throughout this section, we will address some common types of problems that often arise. Understanding these patterns can help you recognize similar structures in future tasks and increase your efficiency in solving them.

1. Fraction and decimal operations.
2. Word problems requiring multiple steps to find the solution.
3. Equations that need simplification or substitution for easier solving.

Overview of Key Concepts in Lesson 17

This section introduces the fundamental ideas and objectives covered in the set of exercises. The goal is to provide a structured approach to understanding the key principles, allowing you to gain a solid grasp of the material before diving into more complex problems. The focus is on building a strong foundation that can be applied to future topics and challenges.

Concepts to Master

In this section, you will encounter various topics that challenge your ability to think critically and apply problem-solving techniques. The exercises require you to manipulate different types of data and practice essential skills that are crucial for progress in your studies.

• Identifying relationships between different values.
• Solving problems involving multi-step calculations.
• Applying strategies to simplify and understand complex questions.

Focus on Practical Application

Mastering the concepts presented here will enhance your ability to tackle both straightforward and advanced problems. By focusing on the practical application of the skills, you will be better prepared for more challenging topics down the line. Using these techniques will ultimately make you more efficient and confident in solving problems independently.

Key Concepts Covered in Lesson 17

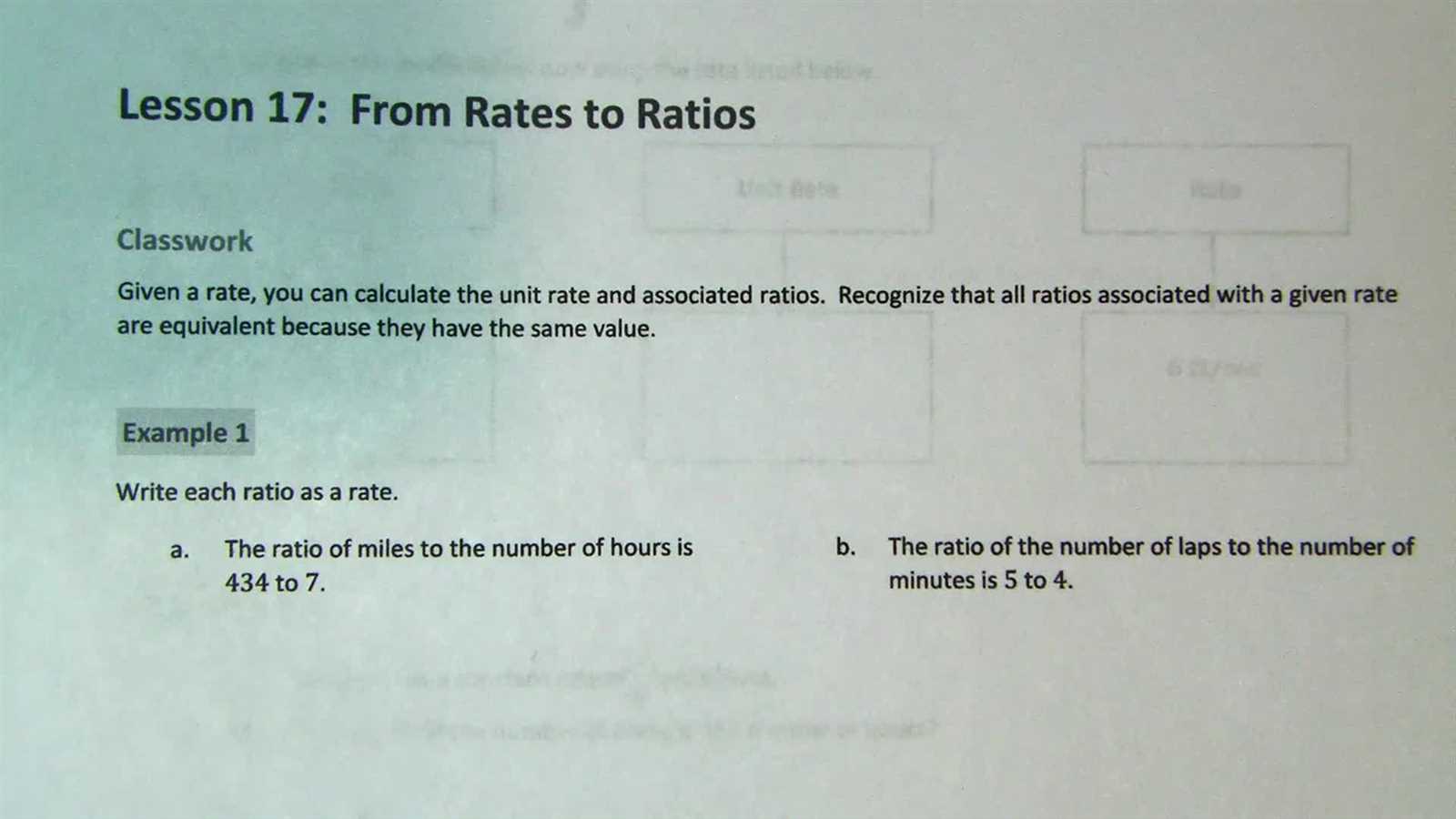
This section explores the core principles and topics addressed in the current set of exercises. Understanding these key ideas is essential for successfully working through the problems and applying them to more advanced concepts in future exercises. The goal is to build both foundational and practical knowledge that you can use effectively when solving similar tasks.

Important Topics to Understand

The exercises in this section focus on a range of interconnected concepts. Mastering these ideas will enhance your ability to approach complex problems with confidence and precision.

• Understanding the relationships between various mathematical elements.
• Working with fractions and decimals in different contexts.
• Breaking down word problems into smaller, manageable steps.
• Applying basic algebraic concepts to simplify and solve equations.
• Recognizing patterns and using them to predict solutions.

How These Concepts Build Upon Each Other

Each topic builds on the previous one, creating a logical progression of ideas. By mastering the foundational concepts first, you will be better prepared to tackle more complex problems as you move forward. These concepts are not isolated but are interrelated, meaning that strengthening one area will help improve your understanding of others.

1. Start with simple operations and gradually work towards multi-step problems.
2. Use visual aids such as diagrams and models to clarify concepts.
3. Practice applying these techniques to real-life scenarios for better understanding.

Step-by-Step Solution Guide for Lesson 17

This section provides a detailed, step-by-step guide to solving the key problems in the current set. By breaking down each problem into smaller, manageable steps, the aim is to help you understand the process and approach used to reach the correct solution. Following these steps will allow you to not only solve similar problems but also grasp the underlying concepts more effectively.

Step 1: Identify the Key Elements

Begin by carefully reading the problem and identifying the key values and variables. This is crucial for determining the operations and methods needed for solving it. Pay attention to units of measurement, relationships between numbers, and any specific instructions provided in the problem.

• Look for keywords or phrases that indicate the necessary operations, such as “total,” “difference,” or “product.”
• Highlight the numbers and variables that are directly involved in the solution.

Step 2: Break Down the Problem

Once the key elements are identified, break the problem into smaller, more manageable parts. This will allow you to approach it logically and avoid feeling overwhelmed. By simplifying the task into steps, it becomes easier to follow through and maintain accuracy throughout the process.

• Start with the most straightforward part of the problem.
• Solve step by step, checking each part as you go along.

Step 3: Apply the Appropriate Methods

Use the relevant formulas or techniques to solve each part of the problem. Depending on the type of task, you may need to use basic operations, simplify expressions, or apply algebraic rules. Always double-check your work to ensure no errors were made during calculations.

• Perform calculations carefully and in the correct order.
• Revisit any steps that seem unclear to ensure full understanding.

Step 4: Review the Solution

After arriving at a solution, take a moment to review the entire process. Ensure that every step makes sense and the final answer aligns with the problem’s requirements. This final review is essential for verifying your work and confirming the correctness of the result.

Understanding Core Problems in Lesson 17

This section focuses on the key problems that appear throughout the set of exercises. By analyzing the structure and types of these problems, you can develop strategies for tackling them effectively. Understanding these core problems is crucial for mastering the concepts and applying them successfully in similar situations.

Types of Problems You Will Encounter

The problems in this section come in various forms, each requiring different approaches. Identifying the type of problem you’re dealing with is the first step toward finding the most effective solution. Below is a table outlining common problem types and strategies for addressing them:

Problem Type	Key Strategy
Basic Calculations	Focus on performing each operation step by step. Double-check each calculation for accuracy.
Word Problems	Break the problem into smaller pieces, identify the unknowns, and set up equations or expressions based on the given information.
Algebraic Equations	Simplify the equation first and solve for the unknown variable using inverse operations.
Multi-Step Problems	Take a methodical approach by solving each step in order, ensuring accuracy in intermediate solutions.

Approach for Solving Complex Problems

For more complex problems, the key is breaking them down into smaller, more manageable parts. Approach each subtask systematically, and don’t hesitate to revisit earlier steps if needed. Using diagrams or visual aids can also help clarify the relationships between different components of the problem.

Helpful Tips for Lesson 17

This section provides valuable tips and strategies to help you navigate through the exercises more efficiently. Whether you’re struggling with a specific concept or simply looking for ways to improve your problem-solving skills, these suggestions will guide you toward mastering the material.

Key Strategies for Success

Applying the right techniques and methods is essential for solving problems accurately and efficiently. Below are some useful tips that will help you make the most out of each exercise:

• Read carefully: Take the time to fully understand each question before starting. Highlight important information and keywords.
• Break down the problem: Divide complex problems into smaller, manageable steps to simplify the process and avoid confusion.
• Check your work: After completing a problem, double-check your calculations and solutions to ensure accuracy.
• Use visual aids: Diagrams or number lines can help clarify relationships and simplify problem-solving.

Time Management Tips

Effective time management is crucial when working through a set of problems. To stay on track, try the following:

1. Start with easier problems: Tackle simpler exercises first to build confidence and gather momentum.
2. Work in intervals: Break your study time into focused intervals, with short breaks in between to maintain concentration.
3. Prioritize difficult tasks: Focus on the more challenging problems after you’ve completed the easier ones to avoid getting stuck early.

Common Mistakes in Lesson 17 and How to Avoid Them

As you work through the problems in this section, there are several common errors that students often make. Identifying these mistakes and understanding how to avoid them will help you improve your problem-solving accuracy and efficiency. This section outlines the most frequent issues and offers practical tips for overcoming them.

Typical Mistakes and Their Solutions

While solving problems, students frequently overlook key details or rush through calculations. Below is a table listing common errors along with strategies for avoiding them:

Mistake	How to Avoid It
Skipping important steps	Take the time to break each problem down into smaller steps. Always follow a clear process and check each step before moving forward.
Misinterpreting the question	Read the problem carefully and highlight key phrases. If necessary, rephrase the question to ensure understanding.
Incorrectly applying formulas	Familiarize yourself with the formulas before starting. Double-check each formula and its application in context.
Making calculation errors	Use a calculator for complex calculations and always review your math for mistakes. Take your time to ensure each operation is correct.

Strategies for Consistent Accuracy

To reduce errors and increase accuracy in your work, consider the following strategies:

• Work slowly and methodically: Rushing through problems often leads to mistakes. Ensure you understand each step before proceeding.
• Recheck your work: After completing a problem, review your solution to catch any potential errors.
• Use visual aids: Diagrams or drawings can help visualize relationships between values, preventing mistakes in interpretation.

How to Approach Word Problems in Lesson 17

Word problems can often seem intimidating, but with the right approach, they become much more manageable. The key is to break down the information presented and translate it into mathematical operations. By understanding the structure of the problem and following a clear process, you can solve them with confidence.

Step-by-Step Approach

Here is a systematic method to tackle word problems effectively:

• Read the problem carefully: Start by reading the entire question at least twice. Ensure that you understand the context and what is being asked. Look for keywords that indicate the operations needed, such as “total,” “difference,” or “product.”
• Identify the given information: Highlight or underline the numbers and important details that are given in the problem. These are the values you’ll use to form your equation.
• Translate into mathematical terms: Convert the verbal descriptions into mathematical expressions. For example, if a problem says “three times a number,” write it as 3x.
• Set up an equation: Using the information you have, set up an equation or a system of equations that reflects the relationships described in the problem.
• Solve the equation: Solve for the unknowns using the appropriate methods. Keep track of your work to avoid mistakes in the process.
• Check your solution: Once you have an answer, go back to the original problem and make sure the solution makes sense in the context. If it doesn’t, review your steps and look for errors.

Tips for Success

Here are a few additional tips to help you excel at solving word problems:

• Draw a diagram: For problems involving geometry or spatial relationships, drawing a picture can help visualize the scenario and simplify the problem.
• Use estimation: Before solving, estimate the possible answer. This will give you a rough idea of what to expect, helping you spot mistakes more easily.
• Practice regularly: The more word problems you solve, the more confident you will become in recognizing common patterns and applying the right strategies.

Strategies to Solve Complex Problems Efficiently

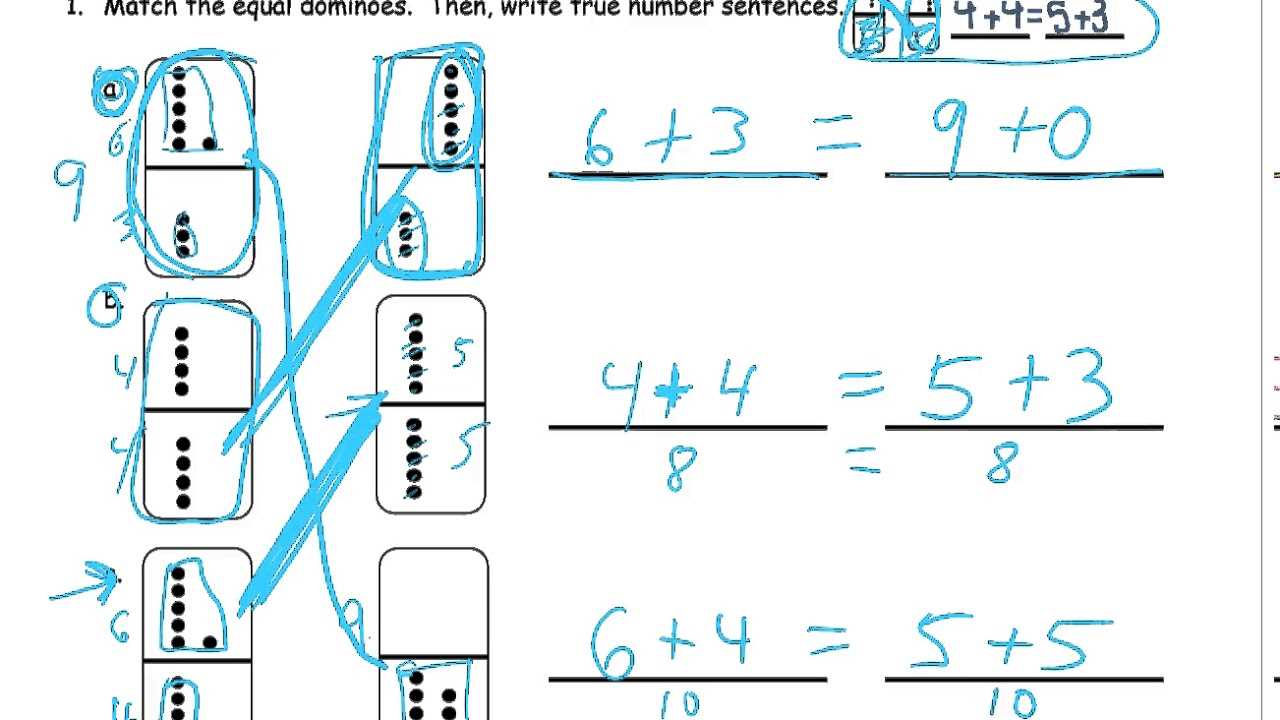
When facing challenging problems, having a clear and organized approach is essential. Complex problems often involve multiple steps, abstract concepts, or require the application of different techniques. By breaking down these problems into manageable parts and using strategic methods, you can solve them efficiently and accurately.

Breaking Down the Problem

One of the most effective ways to solve complex problems is by breaking them into smaller, simpler steps. This makes the task feel less overwhelming and helps you focus on one part at a time. Here are some strategies to break down the problem:

• Identify the goal: Start by clearly understanding what you’re trying to find. What is the final answer you’re looking for?
• Separate the information: Distinguish between the given information and the unknowns. Write down the facts that are provided in the problem.
• Organize your work: Lay out the steps you need to take to reach the solution. This could involve writing down intermediate equations, creating diagrams, or listing relevant data.

Using Logical Approaches and Estimation

In addition to breaking the problem down, using logical strategies can help ensure that you stay on track. Estimation can also be a valuable tool when solving complex problems, especially when accuracy isn’t critical at the initial stages.

• Start with what you know: Apply known formulas or methods to simplify the problem. This can give you a foundation on which to build your solution.
• Work backwards: If possible, try solving the problem starting from the end result and work backwards to identify the necessary steps.
• Estimate: Make a rough estimate of the final answer before diving into the exact calculations. This can give you a sense of whether your solution is reasonable.

Example Questions and Solutions from Lesson 17

To better understand the techniques and strategies discussed, it’s helpful to work through a few example problems. These examples demonstrate how to apply key concepts and provide step-by-step solutions to common types of problems found in this section. By reviewing these examples, you can gain a clearer understanding of the process and apply it to similar challenges.

Example 1: Solving for Unknowns in Word Problems

Problem: A store sells pencils at $1.50 each and erasers at $0.80 each. If a customer buys 4 pencils and 6 erasers, how much did they spend in total?

Solution: First, break the problem into two parts: one for the pencils and one for the erasers.

• Cost of pencils: 4 pencils × $1.50 = $6.00
• Cost of erasers: 6 erasers × $0.80 = $4.80

Now, add the two costs together: $6.00 + $4.80 = $10.80. Therefore, the total cost is $10.80.

Example 2: Solving an Equation with Variables

Problem: Solve for x in the equation: 3x + 5 = 20.

Solution: Begin by isolating the variable x.

• Subtract 5 from both sides: 3x = 20 – 5 → 3x = 15
• Divide both sides by 3: x = 15 ÷ 3 → x = 5

The solution to the equation is x = 5.

Practice Problems for Lesson 17

Practicing a variety of problems is one of the best ways to reinforce the concepts and methods introduced in this section. These exercises will help you apply what you’ve learned and test your understanding of the material. By working through different types of problems, you can build confidence and improve your problem-solving skills.

Practice Problems

Below are several practice problems designed to challenge your understanding and help you apply the strategies you’ve learned. Take your time to solve each one and refer back to the solution process if you get stuck.

Problem	Solution Steps
1. Find the value of x in the equation: 2x + 7 = 15	Subtract 7 from both sides: 2x = 15 – 7 → 2x = 8 Divide both sides by 2: x = 8 ÷ 2 → x = 4
2. A bag contains 3 red balls and 5 green balls. What is the total number of balls in the bag?	Red balls: 3 Green balls: 5 Total: 3 + 5 = 8 balls
3. Solve for y: 4y – 3 = 9	Add 3 to both sides: 4y = 9 + 3 → 4y = 12 Divide both sides by 4: y = 12 ÷ 4 → y = 3
4. If a car travels 60 miles in 2 hours, what is its speed?	Speed = Distance ÷ Time Speed = 60 miles ÷ 2 hours = 30 miles per hour
5. Solve for z: 5z + 3 = 18	Subtract 3 from both sides: 5z = 18 – 3 → 5z = 15 Divide both sides by 5: z = 15 ÷ 5 → z = 3

Challenge Problem

For those looking to push their skills further, here’s a more challenging problem:

Problem: A train travels at a speed of 45 miles per hour for 2.5 hours. Then, it slows down to 30 miles per hour for the next 3 hours. What is the total distance traveled by the train?

Solution:

• Distance = Speed × Time
• First part: 45 miles per hour × 2.5 hours = 112.5 miles
• Second part: 30 miles per hour × 3 hours = 90 miles
• Total distance = 112.5 miles + 90 miles = 202.5 miles

Explaining the Key Formulas in Lesson 17

Understanding the core formulas used in this section is essential for solving various types of problems effectively. These formulas serve as the foundation for finding solutions and offer a structured approach to tackling complex questions. In this part of the article, we will break down the key equations and explain how to apply them step-by-step for different scenarios.

Important Formulas to Remember

Here are some of the most important formulas covered in this section:

• Formula 1: Equation for Solving Linear Expressions
  • Used to solve for variables in linear equations
  • General form: Ax + B = C
  • To solve: isolate the variable (x) by performing inverse operations
• Formula 2: Area of a Triangle
  • Formula: A = ½ × base × height
  • Used to calculate the area of a triangle when the base and height are known
• Formula 3: Distance, Rate, Time Relationship
  • Formula: Distance = Rate × Time
  • Used to calculate distance traveled when speed (rate) and time are known

How to Apply These Formulas

Each formula serves a specific purpose, and it’s important to understand when and how to use them in different contexts. Let’s walk through a few examples:

• Example 1: Solving Linear Expressions
  • Given: 2x + 3 = 7
  • To solve, subtract 3 from both sides: 2x = 4
  • Then, divide both sides by 2: x = 2
• Example 2: Calculating the Area of a Triangle
  • Given: base = 6 units, height = 4 units
  • Formula: A = ½ × base × height = ½ × 6 × 4 = 12 square units
• Example 3: Using the Distance Formula
  • Given: Rate = 60 miles per hour, Time = 3 hours
  • Formula: Distance = Rate × Time = 60 × 3 = 180 miles

Mastering these formulas is key to progressing through the material and solving more advanced problems efficiently. Practice applying them regularly to strengthen your understanding and build confidence.

How to Check Your Answers in Lesson 17

Verifying your solutions is a critical step in ensuring accuracy and understanding the concepts better. Double-checking your results allows you to identify any potential errors early, providing an opportunity to correct them before moving on. In this section, we’ll explore different strategies to help you efficiently check your solutions and avoid common mistakes.

Steps to Review Your Work

Follow these steps to carefully verify your calculations and reasoning:

• Step 1: Revisit the Problem – Before checking your solution, make sure you understand the question thoroughly. Look for key information, such as the given values and what the problem is asking for.
• Step 2: Reverse Operations – For many types of problems, performing the opposite of your original steps can help you check your work. For example, if you added or subtracted, try doing the reverse operation to ensure the result is consistent.
• Step 3: Estimate the Answer – Quickly estimate what the solution should be. If your final answer seems drastically different from your estimation, it could indicate an error.
• Step 4: Check Units and Labels – Make sure that the units in your final answer match the expected units for the problem. A mismatch can be a sign of a miscalculation or misunderstanding of the question.

Additional Techniques for Verifying Solutions

Here are some more techniques to ensure your answers are correct:

• Use a Different Method – If possible, solve the problem using a different approach. Comparing the results from both methods will help you verify your answer.
• Consult Example Problems – Review examples that closely resemble the problem you’re solving. This will give you a clear benchmark to compare your work against.
• Ask for Feedback – If you’re still unsure about your solution, seek feedback from a teacher, peer, or tutor. A fresh perspective can often spot errors that you might have overlooked.

By following these strategies, you can increase your confidence in your answers and ensure that you fully grasp the material. Taking the time to review and check your work is an invaluable skill that will help you succeed in future problems and assessments.

Resources to Supplement Lesson 17 Learning

To deepen your understanding and reinforce the concepts introduced in this section, it’s helpful to explore additional materials. Whether you need more practice, visual aids, or expert explanations, there are several resources available that can enhance your learning experience. These tools can help clarify difficult topics, offer different perspectives, and provide more opportunities for practice.

Here are some valuable resources to consider as you work through the material:

• Online Educational Platforms – Websites like Khan Academy, Coursera, and edX offer free lessons, videos, and exercises that align with the topics covered. These platforms can provide a more interactive way to approach complex problems and concepts.
• Interactive Apps – Apps such as Photomath or Wolfram Alpha can help you step through problems and verify your solutions in real-time, offering detailed breakdowns of each step.
• Study Guides and Textbooks – Many textbooks and study guides offer comprehensive explanations of key concepts and practice problems. Reviewing these texts can offer deeper insights and alternative methods of solving problems.
• Workbooks – Dedicated workbooks focused on specific topics often feature practice exercises and explanations designed to help you master each concept. These can be particularly useful for practicing challenging problems at your own pace.
• YouTube Tutorials – Channels dedicated to educational content provide visual demonstrations of various concepts. Searching for tutorial videos can help you see how to approach different types of problems and offer alternative methods of explanation.

By utilizing these resources, you can supplement your learning and ensure that you fully understand the material. Whether through practice, visual aids, or expert guidance, these tools can help you strengthen your grasp on the concepts and improve your problem-solving skills.

How Lesson 17 Prepares for Future Lessons

The concepts introduced in this section are foundational for more advanced topics that will follow. By mastering the skills and strategies taught here, students can build a solid understanding that will be essential as they progress through more complex material. The techniques learned now act as stepping stones, ensuring that learners have the necessary tools and strategies to tackle future challenges with confidence.

This section focuses on reinforcing core problem-solving skills, which will be required in subsequent units. The ability to apply logical reasoning, break down multi-step problems, and use formulas effectively will become even more critical as the difficulty increases. By internalizing these strategies now, students will have an easier time understanding and mastering the more intricate concepts that await in future lessons.

Moreover, the exercises in this part help students develop a deeper understanding of the connections between different mathematical principles. This interconnectedness is key to solving more advanced problems down the road. Without a strong grasp of the material presented here, tackling future content would be much more challenging.

Ultimately, the knowledge gained in this unit sets the stage for greater success in upcoming topics. It equips students with the confidence and competence needed to approach more complex problems, ensuring they continue to progress smoothly throughout their studies.

Best Study Habits for Eureka Math Success

Developing effective study habits is essential for mastering complex material and achieving success in this curriculum. Consistency and focus are key to ensuring that the concepts are not only understood but also retained. By following a structured approach, students can make the learning process more manageable and improve their overall performance.

1. Break Down the Material
Rather than tackling large sections all at once, break the material into smaller, more digestible parts. Focusing on one concept at a time ensures that the information is absorbed fully, and it helps prevent overwhelm. It’s important to take time to understand each step before moving on to the next.

2. Practice Regularly
Consistent practice is one of the best ways to reinforce learning. Working through problems, especially those that challenge your understanding, helps to solidify the concepts. Repetition not only builds confidence but also improves problem-solving speed over time.

3. Seek Help When Needed
Don’t hesitate to ask for help when a concept feels unclear. Whether it’s from a teacher, tutor, or online resource, getting clarification early on prevents confusion from building up. Asking questions and seeking different perspectives can lead to deeper understanding.

4. Use Supplementary Resources
In addition to textbooks and class notes, leverage online tutorials, videos, and practice sets. These resources offer alternative explanations and methods that can aid in grasping difficult material. Visual aids and step-by-step walkthroughs can make complex topics more accessible.

5. Review and Reflect
After studying, take time to review what you’ve learned. Reflect on your understanding by explaining the material to someone else or summarizing it in your own words. Reviewing material regularly, even after it seems mastered, ensures long-term retention.

6. Stay Organized
Keeping track of notes, assignments, and important concepts is crucial for success. Use a dedicated notebook or digital tool to organize your study materials by topic. A clear organization system helps you find information quickly and ensures nothing important is overlooked.

By incorporating these habits into your study routine, you’ll be better equipped to grasp complex concepts, build a strong foundation, and succeed in future topics. The key to success lies in persistence, practice, and a proactive approach to learning.

Frequently Asked Questions About Lesson 17

Students often have similar questions when tackling this section of the curriculum. In this part, we address the most common queries to help you navigate through the material with greater ease and confidence. Understanding the key concepts and approaches will aid in resolving any confusion and set you up for success.

What is the main focus of this section?

This part of the course emphasizes building a strong foundation in essential problem-solving techniques. It focuses on helping students understand the underlying principles and strategies that will be essential for tackling more advanced topics later on.

How can I effectively solve the problems presented?

• Understand the concepts first: Before diving into the problems, ensure that you have a solid grasp of the core principles. Take time to read through the examples and study how the steps are applied.
• Work through smaller sections: Break down the problems into smaller steps. Focus on one aspect at a time to avoid becoming overwhelmed by more complex questions.
• Use practice problems: Practicing with different examples will help you familiarize yourself with various problem types and improve your problem-solving speed.

What are the most common mistakes to avoid?

• Rushing through the steps: Many students make the mistake of skipping important steps in an effort to finish quickly. Take your time to ensure accuracy at each stage.
• Misunderstanding key terms: Be careful not to overlook definitions and terminology, as misinterpreting these can lead to incorrect answers.
• Neglecting to review your work: Always check your calculations and reasoning before finalizing your answers. Reviewing your work can often catch small mistakes that might otherwise go unnoticed.

How can I improve my understanding of the topic?

• Utilize supplemental resources: Consider watching instructional videos or reading additional materials to reinforce what you have learned.
• Ask for help: If you’re stuck on a particular concept, don’t hesitate to reach out to your instructor or peers for clarification.
• Practice consistently: The more you practice, the more confident and proficient you will become. Consistent practice is key to mastering the material.

By addressing these frequently asked questions, we hope to clarify common concerns and provide the tools needed to successfully navigate this section. With dedication and the right approach, you’ll be able to overcome challenges and build a strong foundation for future learning.