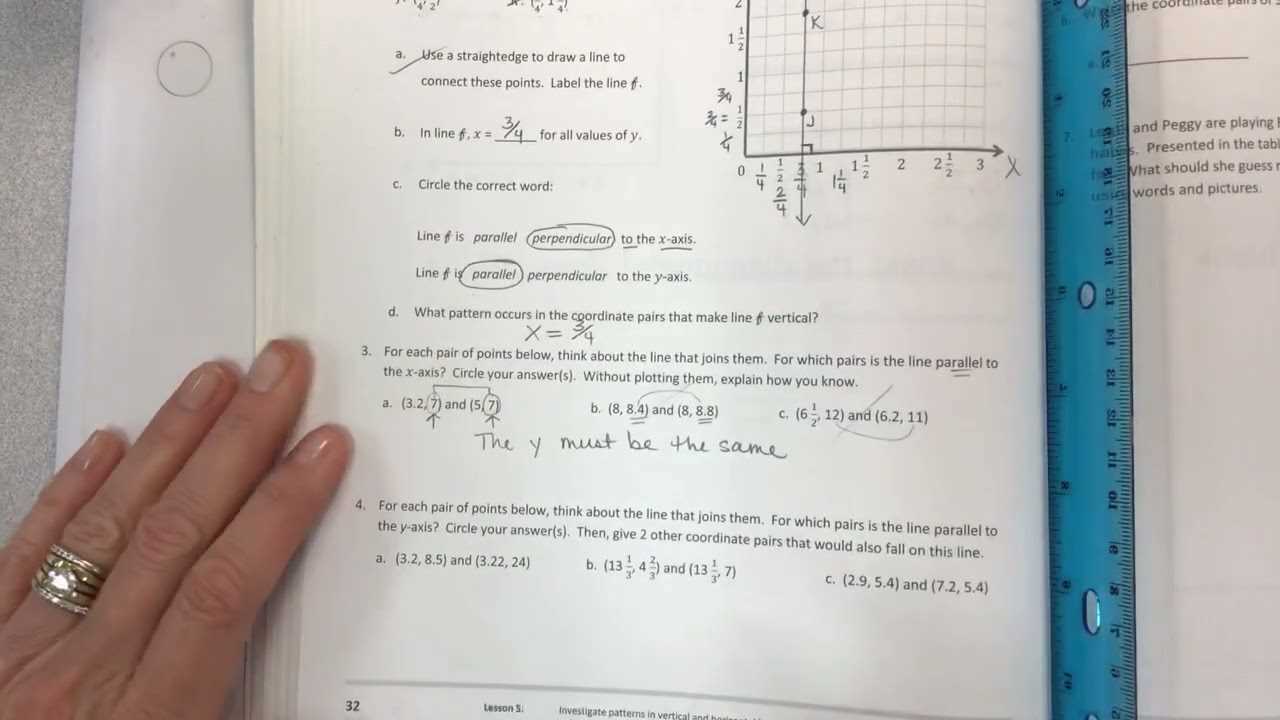
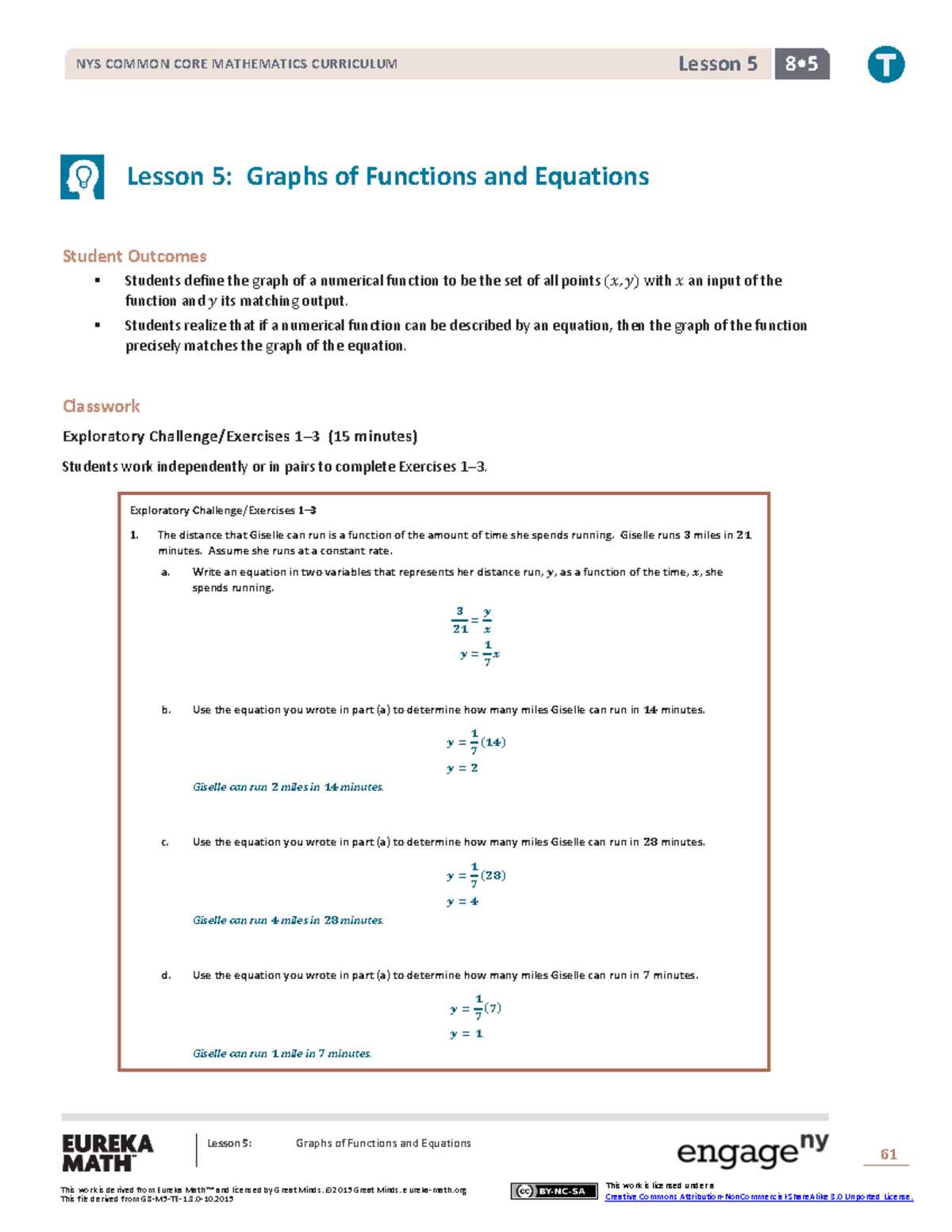
Eureka Math Lesson 5 Answers for Easy Understanding
Mastering the core principles of any subject requires practice and a clear understanding of the steps involved in solving complex problems. Whether you are a student or a tutor, knowing how to approach each challenge is essential for success. In this section, we will explore the best ways to tackle specific problems, ensuring a deeper grasp of the material and enhancing your problem-solving skills.
Throughout this guide, we will break down common obstacles and provide clear solutions, focusing on practical techniques that help you move through each problem with confidence. You will learn how to identify crucial concepts, apply appropriate strategies, and check your work for accuracy, all while developing a stronger foundation in the subject matter.
By following the steps outlined here, you’ll gain both the knowledge and the confidence to approach similar challenges in the future with ease. Understanding each concept thoroughly is the key to improving performance, and this approach will guide you toward mastering even the most difficult tasks.
Comprehensive Guide to Solving Key Problems
In any educational framework, mastering the process of solving problems is essential for gaining a deeper understanding of the material. This section will provide an organized approach to tackling challenging exercises, ensuring that students not only reach the correct solutions but also build the skills needed to solve similar problems independently. Each step is designed to enhance both comprehension and application of core principles.
Breaking Down the Process
The first step in solving any problem is understanding the structure of the question and identifying what is being asked. Whether the task involves fractions, equations, or word problems, breaking down the components helps in organizing thoughts and making sense of the required operations. Follow these steps for a more effective approach:
- Read Carefully: Take your time to understand the problem before jumping to conclusions.
- Highlight Key Information: Identify the numbers, operations, and conditions that are critical to solving the task.
- Choose the Right Strategy: Depending on the type of problem, select the method or formula that fits best.
Common Pitfalls and How to Avoid Them
Even experienced learners can face difficulties when working through complex tasks. Here are a few common mistakes and how to avoid them:
- Skipping Steps: It’s tempting to jump ahead, but skipping intermediate steps often leads to errors.
- Misinterpreting Instructions: Pay attention to units, signs, or specific conditions in the problem.
- Overcomplicating the Solution: Sometimes the simplest method is the best approach, so avoid overthinking the solution.
By following these guidelines and avoiding common mistakes, you’ll be able to approach any challenge with confidence, ensuring both accuracy and efficiency in your problem-solving approach.
Understanding Key Concepts in Lesson 5
Grasping the foundational ideas behind any educational content is crucial for applying them effectively to problem-solving. In this section, we’ll break down the core principles introduced in this particular segment, explaining the strategies and methods that will guide you through solving related exercises. By understanding these key elements, you will be better prepared to tackle the problems with confidence and clarity.
Core Principles of the Topic
This section introduces a series of essential concepts that build on previous knowledge. The focus is on mastering the steps required to handle the exercises with accuracy. Key techniques, such as breaking down complex problems into manageable parts, identifying patterns, and applying relevant formulas, are all integral to making sense of the material. Understanding these methods will allow you to see the connections between different types of problems and solutions.
Application of Strategies
Once you are familiar with the core principles, it’s time to apply them to real-world examples. Working through practice problems helps reinforce these techniques and highlights areas where further understanding may be needed. The goal is to create a solid foundation for solving similar tasks independently, applying the learned strategies with precision.
Step-by-Step Solutions for Lesson 5
Understanding how to approach each problem methodically is key to mastering the material. In this section, we will break down the process into clear steps, making it easier to follow and apply the techniques to similar exercises. By working through these examples, you will gain a deeper understanding of the necessary steps and how to execute them correctly for accurate results.
Breaking Down the Problem
The first step is to fully understand the task at hand. Start by identifying the critical components of the problem and the operations required. Below is a simplified step-by-step guide for solving a typical problem:
| Step | Action |
|---|---|
| 1 | Read the problem carefully and highlight key information |
| 2 | Determine the operations needed (addition, subtraction, multiplication, etc.) |
| 3 | Apply the correct formula or method for the task |
| 4 | Double-check each step for accuracy and consistency |
| 5 | Review the solution to ensure it aligns with the problem requirements |
Example Problem Breakdown
Now, let’s apply these steps to an example problem. For illustration, we will use a simple equation-solving task. By following the steps outlined above, you can approach each question systematically, ensuring that no crucial information is overlooked.
Key Concepts Covered in Lesson 5
In this section, we will explore the fundamental ideas and techniques introduced in this part of the curriculum. These concepts are crucial for building a deeper understanding of the material, as they form the foundation for solving more complex problems. By mastering these key points, you will be well-equipped to handle a variety of tasks and challenges moving forward.
The focus of this segment is on understanding core principles, such as problem decomposition, the application of relevant strategies, and the identification of patterns. Each of these elements plays an essential role in making sense of complex tasks and in ensuring that solutions are reached efficiently. Mastery of these ideas will significantly improve your ability to approach similar problems in the future.
Common Challenges in Understanding Key Concepts
While progressing through different exercises and problems, many learners encounter similar obstacles that can hinder their understanding and problem-solving efficiency. These challenges often stem from the complexity of the material or the way in which the concepts are presented. Identifying and addressing these issues early on is essential for ensuring a smoother learning experience.
Misunderstanding Problem Structure
One of the most common difficulties students face is misunderstanding the structure of the problems themselves. Complex word problems or multi-step tasks can be overwhelming, making it hard to determine what needs to be solved first. Students may struggle with identifying key information or applying the right operations to reach the solution. Focusing on breaking down the problem into smaller, more manageable parts can help alleviate this challenge.
Applying Strategies Inaccurately
Another frequent issue is the incorrect application of the appropriate solving strategies. Sometimes, students may attempt to use a method that is not suited for the task at hand, leading to confusion and errors. To overcome this, it is essential to practice recognizing which strategy works best for each type of problem and to become comfortable with various techniques. Over time, this will help in making more accurate choices when solving tasks.
Mastering Fraction Problems in Lesson 5
Fraction problems are a common challenge for many students, but once the core principles are understood, they become much easier to tackle. This section will guide you through the necessary steps and strategies to confidently solve fraction-related exercises. By mastering these techniques, you will be able to approach even the most complex fraction problems with ease and accuracy.
Key Concepts in Working with Fractions
Understanding how to handle fractions involves several critical concepts, including simplification, finding common denominators, and performing basic operations such as addition, subtraction, multiplication, and division. These steps are essential for solving fraction problems efficiently. It’s important to practice each of these techniques individually before combining them in more complex exercises.
Practical Strategies for Fraction Problems
To solve fraction problems effectively, start by identifying the type of operation required. Whether you’re adding or multiplying fractions, always ensure the fractions are in the simplest form possible before proceeding. For addition and subtraction, finding a common denominator is key. For multiplication and division, cross-multiplying or inverting fractions is often the solution. Consistent practice will build confidence and improve accuracy in solving fraction-related tasks.
How to Approach Problems Effectively
Approaching complex problems can be overwhelming, but breaking them down into smaller, manageable steps can make the process more straightforward. In this section, we will explore effective strategies for tackling challenging tasks, ensuring that each part of the problem is addressed properly. By following a structured approach, you will gain the confidence needed to solve problems accurately and efficiently.
Step-by-Step Approach to Problem Solving
When faced with a new problem, it’s essential to follow a clear process to ensure that no details are overlooked. Consider the following steps to help guide your approach:
- Read the Problem Carefully: Take your time to understand what is being asked before attempting to solve it.
- Highlight Key Information: Identify important numbers, units, and relationships that are essential for solving the task.
- Break It Down: Split the problem into smaller, more manageable parts to avoid feeling overwhelmed.
- Select the Appropriate Method: Choose the right strategy or formula based on the problem’s requirements.
- Check Your Work: After solving, go back and review each step to ensure accuracy.
Common Strategies for Different Types of Problems
Different types of problems require different approaches. Below are some common strategies to consider:
- For Word Problems: Focus on translating the words into mathematical expressions and identifying what is being asked.
- For Fraction Problems: Simplify fractions where possible and ensure common denominators for addition or subtraction.
- For Multi-Step Problems: Solve step by step, ensuring that each intermediate result is accurate before moving on to the next operation.
By following these steps and strategies, you will be able to approach each problem with clarity and confidence, increasing your ability to solve similar tasks in the future.
Tips for Solving Exercises Effectively
Solving complex exercises requires a strategic approach to ensure accuracy and efficiency. By following a set of useful techniques, you can simplify even the most challenging tasks. This section offers practical tips to help you navigate through problems with ease, saving time and minimizing errors along the way.
One key to success is to approach each problem with a clear plan. Start by fully understanding the question, identifying what is being asked, and then breaking the problem into smaller, manageable steps. Additionally, always double-check your work after each step to catch any mistakes early. Here are some more specific tips that can enhance your problem-solving skills:
- Work Step-by-Step: Avoid rushing through problems. Take the time to complete each step carefully to ensure accuracy.
- Use Visual Aids: Drawing diagrams, charts, or tables can help visualize the problem, making it easier to understand and solve.
- Simplify Whenever Possible: Look for opportunities to simplify fractions or expressions before proceeding to the next step. Simplifying early can save time later.
- Practice Regularly: The more you practice, the more comfortable you will become with applying different techniques. Consistent practice leads to improved skills and faster solutions.
- Review Your Work: Always take a moment to review each step before moving on. Small mistakes can often go unnoticed until you double-check.
By incorporating these tips into your approach, you’ll develop a more systematic and efficient method for solving problems. With continued practice, these strategies will become second nature, making even the most difficult tasks more manageable.
How to Check Your Answers Effectively
Ensuring the accuracy of your solutions is crucial in problem-solving. Even small errors can lead to incorrect results, so it’s essential to have a reliable method for reviewing your work. This section will guide you through practical steps to check your results and ensure they are correct before finalizing your work.
Step-by-Step Verification Process
Start by reviewing each step you took during the problem-solving process. Going back through the problem carefully allows you to spot any mistakes or miscalculations early. Follow these steps to verify your results:
- Revisit Each Step: Go through your work step-by-step and ensure every calculation is correct.
- Check for Consistency: Make sure that all numbers and operations are consistent with the original problem.
- Use Estimation: Before checking the exact value, estimate the result to ensure it falls within a reasonable range.
- Double-Check Units: Ensure that any units or labels are consistent throughout your work and that conversions (if any) are correctly applied.
Cross-Check with Alternative Methods

If possible, use a different method to solve the same problem and compare results. Sometimes, approaching the problem from a different angle can help confirm the correctness of your solution. Whether you’re checking your work with a calculator or using an alternate formula, this strategy can provide an additional layer of verification to ensure your solution is accurate.
By following these verification methods, you can be more confident that your solutions are correct, reducing the likelihood of errors and improving your overall problem-solving skills.
Why These Educational Lessons Matter
Strong foundational skills are essential for long-term academic success, and structured learning plays a crucial role in achieving this. The exercises and strategies introduced in these lessons are designed to build critical thinking and problem-solving abilities. Understanding why these approaches matter can help students not only succeed in their current coursework but also prepare them for more advanced challenges in the future.
Building a Strong Foundation
These lessons provide an essential framework that helps students develop core skills necessary for more complex topics. By tackling fundamental concepts in a clear, step-by-step manner, students can strengthen their ability to approach problems methodically. This structure makes learning easier and more effective, ensuring a solid foundation that will support further academic achievements.
Fostering Problem-Solving Skills
Through consistent practice and application, students enhance their ability to think critically and solve problems efficiently. The approach is not just about memorizing formulas or methods but understanding how and why specific techniques work. By mastering these concepts, learners gain the confidence to tackle challenges independently, an invaluable skill both in and out of the classroom.
In summary, the structured approach of these educational lessons is essential for building lasting skills, fostering critical thinking, and preparing students for future academic and real-world success.
Practical Applications of Lesson 5 Skills
The skills learned in this section have practical uses that extend beyond the classroom, impacting various aspects of daily life and professional work. Understanding how to apply these techniques in real-world scenarios helps students see the value of their education and boosts their problem-solving abilities. Whether it’s through analyzing data, managing finances, or making informed decisions, the knowledge gained can be directly applied to everyday challenges.
Everyday Applications
Students can apply the problem-solving techniques to a wide range of practical situations. Some examples include:
- Budgeting and Financial Planning: Understanding ratios, proportions, and basic calculations can help with managing personal finances, such as creating a budget or comparing prices.
- Time Management: The ability to break tasks into smaller steps and evaluate efficiency can enhance productivity and help prioritize responsibilities.
- Cooking and Recipe Scaling: Using fractions and proportions in cooking, such as adjusting ingredients for a different number of servings, is a direct application of these skills.
Professional Applications
In the professional world, the ability to solve complex problems is crucial. Here’s how the skills learned can be applied in various careers:
- Engineering: Whether it’s designing structures, circuits, or machinery, engineers rely on mathematical concepts to create precise and functional designs.
- Data Analysis: Analyzing trends, drawing conclusions from datasets, and making predictions are tasks that require the logical thinking taught in these lessons.
- Sales and Marketing: Understanding percentages and applying them to sales figures, discounts, and profit margins is key in many business roles.
These skills are essential for tackling problems in the real world, whether you are managing finances, working on a project, or making decisions in a professional setting. Mastering them opens doors to a variety of opportunities in life and work.
Visual Learning Techniques for Eureka Math
Visual learning techniques are powerful tools for understanding complex concepts, especially when working through problems that require a clear representation of relationships and structures. By utilizing images, diagrams, and other visual aids, students can gain a deeper understanding of abstract ideas and improve retention. This section will explore effective visual strategies to help reinforce the learning process and enhance comprehension.
Using Diagrams and Charts
Diagrams and charts can simplify complicated ideas by breaking them down into more manageable visual pieces. Here are some ways to use these tools:
- Flowcharts: Useful for outlining problem-solving steps, flowcharts guide students through a process, helping them see each stage clearly.
- Bar and Line Graphs: These charts are ideal for comparing data and identifying trends, making abstract numbers more tangible.
- Venn Diagrams: Effective for showing relationships between different sets, Venn diagrams can clarify concepts related to grouping and classification.
Color-Coding and Highlighting
Color-coding and highlighting key elements can also improve visual learning. By using different colors to represent different components of a problem, students can more easily differentiate between variables, constants, and operations. For instance:
- Highlighting Key Terms: Emphasize important terms or steps in a problem-solving process to ensure critical information stands out.
- Color-Coding Equations: Use different colors for coefficients, variables, and constants to make the structure of equations clearer.
Incorporating these visual techniques into problem-solving routines can lead to better understanding, quicker recall, and a more intuitive grasp of concepts.
Frequently Asked Questions About Lesson 5
When tackling complex concepts and exercises, it’s natural to have a range of questions. This section addresses some of the most common inquiries that students encounter while working through this particular topic. By understanding these frequently asked questions, learners can gain clarity on key points and avoid common pitfalls.
Common Inquiries
Below are some of the most common questions and answers related to the material covered in this section:
| Question | Answer |
|---|---|
| How do I know which method to use for solving a problem? | The key is to understand the type of problem you’re dealing with. Pay attention to the problem’s structure and use the method that best fits the situation, whether it’s a step-by-step breakdown, pattern recognition, or calculation. |
| What should I do if I don’t understand a specific concept? | If a concept feels unclear, try using visual aids like diagrams, and break down the problem into smaller, more manageable parts. Additionally, reviewing related examples can provide more context. |
| How can I check if my solution is correct? | Double-check your work by reversing the steps. If the result matches the original problem when you go back through it, your solution is likely correct. |
| Are there any shortcuts to solving these problems faster? | While there aren’t necessarily shortcuts, becoming familiar with common patterns and strategies can make the process quicker. Practice helps build familiarity and speed over time. |
Tips for Further Clarification
If you still have questions or need further explanations, consider asking for help from a teacher, tutor, or online learning resources. Don’t hesitate to revisit any sections that seem challenging, as reinforcement can improve understanding.
Improving Speed and Accuracy in Eureka Math
Achieving a balance between speed and accuracy is essential when solving problems. While it’s important to complete tasks efficiently, precision should never be sacrificed. This section explores strategies that can help you solve problems more quickly without compromising on correctness.
Techniques to Enhance Speed and Accuracy
By incorporating the following techniques into your practice, you can improve both the speed and accuracy of your problem-solving process:
- Practice regularly: The more you work with the material, the more familiar you become with the steps involved. This familiarity leads to quicker recognition of patterns and solutions.
- Break down complex problems: When faced with a challenging problem, divide it into smaller parts. Solving one step at a time helps maintain accuracy and prevents mistakes caused by rushing.
- Check your work: Always take a moment to review your solution after completing it. This double-checking process helps catch errors that may have been overlooked during the initial attempt.
- Work on mental calculations: Improving mental math skills allows for faster processing of numbers, which can significantly speed up the problem-solving process.
Practicing with Timed Challenges
To further develop both speed and accuracy, practice under timed conditions. Set a clock and attempt to solve problems within a set period. This type of focused practice simulates real-world scenarios where time constraints are common and helps you improve both speed and efficiency.
Benefits of Using Eureka Math Resources
Leveraging high-quality learning materials can make a significant difference in students’ understanding and retention of key concepts. These resources are designed to provide structured support, offer detailed explanations, and engage students with practical exercises that reinforce their learning. Utilizing such materials effectively can lead to improved comprehension, enhanced problem-solving skills, and increased academic confidence.
Some of the key advantages of using these educational resources include:
- Clear and structured guidance: Well-organized lessons break down complex topics into manageable sections, allowing students to build knowledge step-by-step.
- Comprehensive coverage: These resources cover a wide range of topics, ensuring that all foundational concepts are addressed thoroughly, helping students master each area before progressing.
- Interactive learning: Through various exercises, students can apply their knowledge in real-world scenarios, making learning more engaging and relevant to everyday life.
- Support for diverse learning styles: Whether students are visual, auditory, or kinesthetic learners, these resources offer multiple ways to absorb information, ensuring that every student can find a method that suits their learning style.
- Progress tracking: Many resources include tools for monitoring progress, enabling teachers and students to identify areas of improvement and adjust learning strategies accordingly.
By making use of these thoughtfully designed resources, students not only improve their academic performance but also develop a deeper understanding of the material that will serve them in future learning endeavors.
How to Track Your Progress in Math
Monitoring your advancement in any subject is essential for ensuring steady improvement and identifying areas that need attention. In the context of mathematical studies, tracking your progress allows you to measure your understanding of different concepts, evaluate your problem-solving abilities, and adjust your study approach accordingly. With a well-structured method for tracking, students can stay motivated and ensure they are continuously making progress towards mastering key topics.
There are various ways to track your progress, including self-assessment, periodic reviews, and feedback from teachers or tutors. A systematic approach can help highlight your strengths and weaknesses, providing clear insights into areas for improvement. Below are some strategies to effectively track your development:
1. Regular Practice and Self-Assessment
Consistent practice is one of the most effective ways to gauge your understanding. Regularly solving exercises and revisiting previous topics allows you to evaluate how well you grasp the material. Additionally, self-assessment through quizzes or review exercises can provide immediate feedback on your performance.
2. Tracking Improvement Over Time
One effective method is to track your performance on a set of exercises or problems over a period of time. This allows you to observe trends in your progress. A chart or table can help you see how your accuracy and speed improve with repeated practice.
| Week | Problems Attempted | Correct Answers | Score (%) |
|---|---|---|---|
| Week 1 | 20 | 15 | 75% |
| Week 2 | 25 | 20 | 80% |
| Week 3 | 30 | 27 | 90% |
As shown in the table, tracking performance over several weeks can highlight your progress and motivate you to continue improving.
By regularly checking your work and reviewing your results, you can make adjustments to your study routine, focus on challenging areas, and celebrate your accomplishments as you advance in your learning journey.