Math 2 Exam Review Answers Complete Guide

$math 2 exam review answers$

Mastering complex topics and applying mathematical reasoning is essential for excelling in higher-level assessments. Understanding the core principles and practicing effective techniques can significantly boost performance. This guide is designed to help you navigate through essential concepts and refine your problem-solving skills.

Whether tackling algebraic expressions, geometric proofs, or calculus, having a structured approach will help you approach each challenge with confidence. Focused preparation allows you to identify key patterns and improve your accuracy in solving problems, ensuring you make the most of your study sessions.

By breaking down difficult tasks into manageable steps and focusing on recurring themes, you can strengthen your grasp on core material and avoid common pitfalls. Consistent practice will enhance both speed and precision, ultimately leading to a deeper understanding and a higher level of readiness.

Math 2 Exam Review Answers

Preparing for advanced problem-solving challenges requires a structured approach. By focusing on key concepts and practicing regularly, you can improve both your speed and accuracy. A successful strategy involves reviewing various topics systematically and mastering the techniques needed to solve complex problems effectively.

To enhance your ability to tackle difficult questions, consider the following key strategies:

Understand core principles: Ensure that you have a solid grasp of foundational concepts, as these are essential for solving more advanced problems.
Practice different problem types: Regularly work through a variety of exercises to develop familiarity with different question formats.
Focus on time management: Practice solving problems under timed conditions to improve your ability to work efficiently.
Analyze mistakes: After solving a problem, review your solution to identify any errors and understand where you went wrong.

In addition to these strategies, reviewing common patterns and recurring themes across different questions will help you identify shortcuts and methods that can be applied in various scenarios. This preparation approach will not only build confidence but also ensure that you are ready for any challenge.

Finally, don’t forget to regularly test yourself with mock problems. Simulating the actual test environment allows you to evaluate your readiness and adjust your preparation accordingly.

Understanding Key Concepts for Success

To excel in any advanced problem-solving challenge, it is essential to build a strong foundation of key ideas and principles. Gaining a deep understanding of the underlying concepts allows you to approach each question with confidence and clarity. A solid grasp of these fundamentals will serve as a guide when tackling more complex scenarios and ensure accurate results.

Core Principles to Focus On

Start by identifying the fundamental theories and formulas that form the backbone of the subject. These include rules of algebra, geometric properties, and theorems in higher-level reasoning. Mastering these concepts provides the framework needed to solve a wide range of problems. Take the time to memorize and apply these core principles, as they will often reappear in various forms.

Building Logical Thinking Skills

Effective problem solving depends on the ability to think logically and break down complex tasks into manageable steps. Developing this skill allows you to approach challenges systematically, eliminating confusion and ensuring a clear path to the solution. Practice logical reasoning by working through examples and progressively increasing the difficulty level of the problems you tackle.

Common Topics in Math 2 Exams

When preparing for higher-level problem-solving challenges, it is crucial to familiarize yourself with the most frequently tested concepts. Understanding the typical themes and question types can help direct your focus and make your study sessions more effective. By concentrating on the core areas that are often emphasized, you can ensure that you are well-prepared for a range of potential questions.

Key Areas of Focus

Algebraic Expressions: Simplifying and solving equations, working with polynomials, and understanding functions.
Geometric Theorems: Analyzing shapes, calculating areas, volumes, and working with properties of triangles and circles.
Trigonometry: Understanding sine, cosine, and tangent functions, and solving related problems involving angles and triangles.
Calculus Basics: Differentiation, integration, and applying these techniques to solve real-world problems.

Additional Topics to Consider

Systems of Equations: Solving linear systems and understanding their graphical interpretations.
Sequences and Series: Recognizing arithmetic and geometric sequences, and applying summation formulas.
Probability and Statistics: Calculating probabilities, understanding distributions, and interpreting data.

These topics form the foundation for many questions in higher-level assessments, so prioritizing their study will ensure you are prepared for the most common challenges you will face.

Effective Study Techniques for Math 2

Developing a structured and focused approach to studying is essential for mastering complex concepts and performing well under pressure. Using the right study methods not only helps reinforce understanding but also enhances problem-solving speed and accuracy. By incorporating effective techniques, you can optimize your preparation and tackle any challenge with confidence.

Active Practice and Application

$math 2 exam review answers$

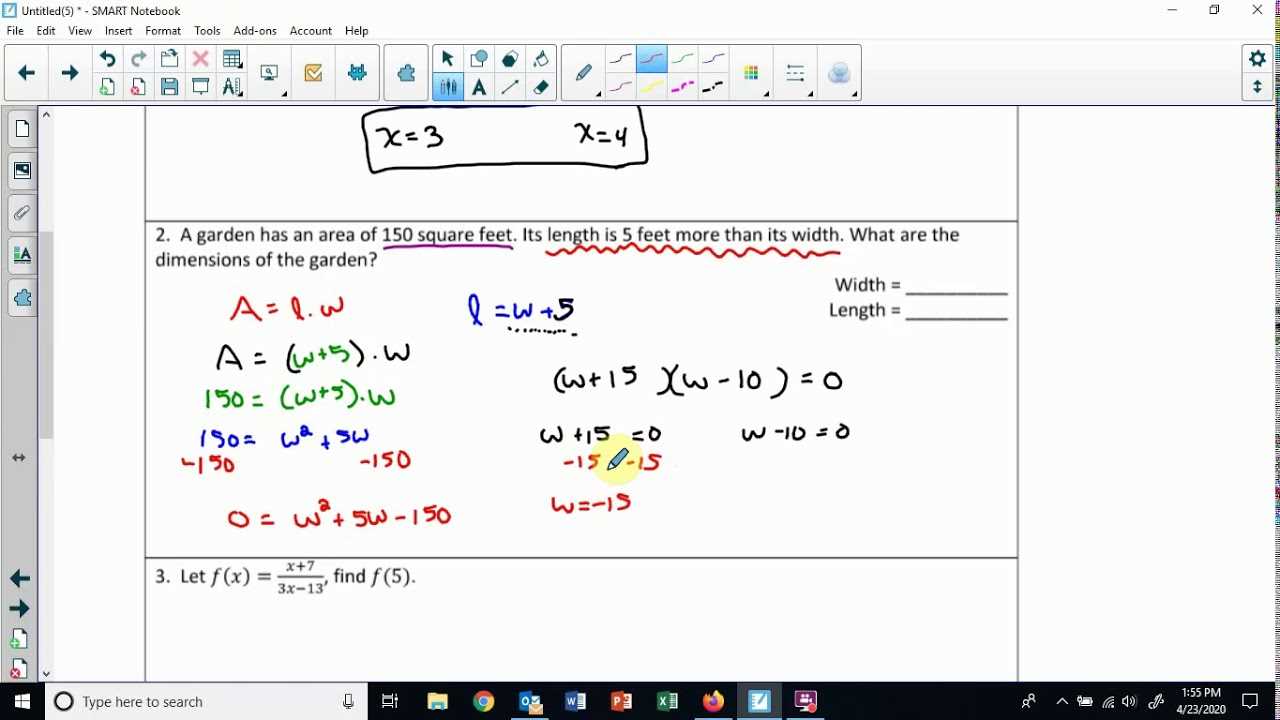
One of the most effective ways to retain information is through active problem-solving. Instead of passively reading through notes, actively engage with the material by working through examples and exercises. This hands-on approach helps solidify your understanding and improves your ability to apply concepts in different scenarios.

Solve diverse problems: Work on a variety of problems, from basic to advanced, to strengthen your grasp on different question types.
Simulate test conditions: Practice solving problems under timed conditions to build confidence and improve time management.

Review and Reinforce Key Concepts

Revisiting essential concepts regularly is vital for long-term retention. Instead of cramming all at once, break your study sessions into focused, manageable chunks. Regularly reviewing key principles, formulas, and techniques helps prevent knowledge gaps and reinforces your ability to solve problems accurately.

Create summary notes: Summarize key concepts in your own words to improve understanding and memory.
Teach someone else: Explaining concepts to a peer or study partner helps reinforce your own understanding.

How to Solve Common Math Problems

To tackle typical problems encountered in advanced assessments, it is essential to have a clear strategy for breaking down each question. By understanding the underlying principles and following a systematic approach, you can solve problems with greater efficiency and accuracy. This section provides practical techniques for approaching common question types and solving them step by step.

Step-by-Step Problem Solving Process

The key to solving complex problems is breaking them into manageable steps. Start by reading the question carefully, identifying what is being asked, and determining the known information. Then, use the appropriate methods and formulas to find the solution. Here’s a simple framework to follow:

Step	Action
1	Read the problem carefully and highlight key information.
2	Identify the unknowns and the formula or method needed to solve the problem.
3	Perform the necessary calculations or apply theorems to find the solution.
4	Review the solution to ensure it makes sense in the context of the problem.

Common Problem-Solving Techniques

Some problems can be efficiently solved using specific strategies. These include:

Substitution: Replacing variables with known values to simplify the problem.
Elimination: Removing variables or terms to make equations easier to solve.
Factorization: Breaking down expressions into simpler components to make solving easier.
Graphical methods: Using graphs to visualize and solve problems involving geometry or functions.

By mastering these techniques and practicing regularly, you can improve your ability to solve common problems efficiently and accurately.

Tips for Improving Your Math Skills

Enhancing your problem-solving abilities requires consistent practice and a proactive approach to learning. By focusing on strengthening key areas and developing effective strategies, you can significantly improve both your understanding and performance. These practical tips will help you build a solid foundation and tackle challenges with greater ease and confidence.

Key Strategies for Mastery

Practice regularly: The more problems you solve, the more familiar you will become with various techniques and methods. Consistent practice reinforces concepts and improves your problem-solving speed.
Review mistakes: When you make an error, take the time to understand where you went wrong. Learning from your mistakes helps you avoid repeating them and strengthens your overall comprehension.
Use different resources: Explore different learning materials such as online tutorials, books, and interactive tools. Each resource offers unique perspectives that can deepen your understanding.
Stay organized: Keep notes, formulas, and important concepts well-organized. Reviewing neatly written summaries and structured solutions helps reinforce what you’ve learned.

Building Confidence and Efficiency

Break problems into steps: Large problems can be overwhelming, so break them into smaller, manageable tasks. This approach reduces stress and makes it easier to find the solution.
Focus on understanding, not memorization: Aim to understand the principles behind the methods you use. This understanding will help you solve unfamiliar problems with greater ease.
Simulate real challenges: Practice solving problems under timed conditions to improve both accuracy and efficiency. This will help you feel more prepared for actual assessments.

By applying these tips and remaining dedicated to improvement, you’ll be well on your way to mastering complex problem-solving tasks.

Important Formulas to Remember

Mastering key formulas is essential for solving a wide range of problems efficiently. Having these formulas at your fingertips helps you apply the correct techniques and find solutions more quickly. This section covers some of the most important and commonly used formulas that will aid in your problem-solving process.

Algebraic Formulas

Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a – Used to solve quadratic equations.
Sum of a Geometric Series: S = a(1 – r^n) / (1 – r) – Formula for the sum of the first n terms of a geometric series, where a is the first term and r is the common ratio.
Factoring Difference of Squares: a² – b² = (a + b)(a – b) – Used to simplify expressions involving the difference of squares.
Binomial Expansion: (a + b)ⁿ = aⁿ + n * aⁿ⁻¹ * b + … + bⁿ – Expanding powers of a binomial expression.

Geometry and Trigonometry Formulas

Area of a Triangle: A = 1/2 * base * height – Basic formula for the area of a triangle.
Area of a Circle: A = π * r² – Used to calculate the area of a circle with radius r.
Sin, Cos, and Tan Relationships: sin(θ) = opposite/hypotenuse, cos(θ) = adjacent/hypotenuse, tan(θ) = opposite/adjacent – Fundamental trigonometric identities.
Pythagorean Theorem: a² + b² = c² – Used to find the length of sides in a right-angled triangle.

Keeping these formulas well-memorized and understanding when to apply them is critical for solving various problems efficiently and accurately. Regular practice and familiarity will help reinforce their use in different contexts.

How to Manage Your Time During the Exam

Effective time management is key to ensuring that you can complete all tasks within the allocated time while maintaining accuracy and focus. A well-organized approach to tackling questions allows you to prioritize and solve problems more efficiently, reducing stress and improving your performance. This section provides practical strategies for optimizing your time during assessments.

Plan Your Time Wisely

Start by reviewing the entire set of questions before you begin working. This gives you an overview of the task and helps you gauge how much time to allocate to each section. Prioritize questions based on their difficulty and point value, and ensure you spend more time on questions that are worth more.

Estimate the time for each question: Divide the total time by the number of questions to determine an average amount of time per question. Allocate more time for complex problems.
Skip difficult questions: If you encounter a question that is taking too long, move on and return to it later. This ensures you don’t lose valuable time.

Stay Focused and Keep Track of Time

$math 2 exam review answers$

During the assessment, it’s important to stay focused on the task at hand. Avoid distractions and maintain a steady pace. Regularly check the time to ensure you are on track and adjust your speed if necessary to avoid rushing at the end.

Use a watch or timer: Keep track of the time throughout the assessment. Set a timer for each section or question to remind you when to move on.
Practice time management: Before the assessment, practice with timed exercises to improve your ability to manage time effectively under pressure.

By following these strategies and practicing good time management, you will be better equipped to complete all tasks on time and with greater accuracy.

Breaking Down Complex Math Questions

When faced with a challenging problem, it’s important to break it down into smaller, more manageable parts. Tackling complex tasks step by step not only simplifies the process but also makes it easier to identify the right approach and solution. In this section, we’ll explore strategies for dissecting intricate problems and solving them systematically.

Step-by-Step Approach

Start by carefully reading the entire problem to understand what is being asked. Identify key information and highlight important data, such as variables, constants, and known values. Once the key elements are clear, organize them logically to form a plan of action. Breaking the question into smaller, more focused parts can help clarify the method to use and the steps to take.

Step	Action
Step 1	Read the problem carefully and identify key information.
Step 2	Break the problem into smaller parts, focusing on one at a time.
Step 3	Determine the method or formula needed for each part.
Step 4	Solve the parts individually, checking for accuracy as you go.
Step 5	Combine the results and review the final solution.

Common Techniques for Breaking Down Problems

Use diagrams: Drawing diagrams or visual aids can help make abstract concepts more concrete, especially for geometric or spatial problems.
Identify patterns: Look for repeating structures or patterns in the problem that can simplify the process, especially in algebraic or numerical tasks.
Work backward: For problems that involve finding unknowns, sometimes starting from the desired outcome and working backward can provide clarity.
Check intermediate steps: After solving each smaller part, review your calculations and reasoning to avoid errors before moving to the next part.

By breaking complex problems into smaller steps and applying systematic techniques, you can transform overwhelming tasks into solvable pieces. With practice, this approach will help you gain confidence in solving even the most intricate challenges.

Using Practice Tests for Better Results

$math 2 exam review answers$

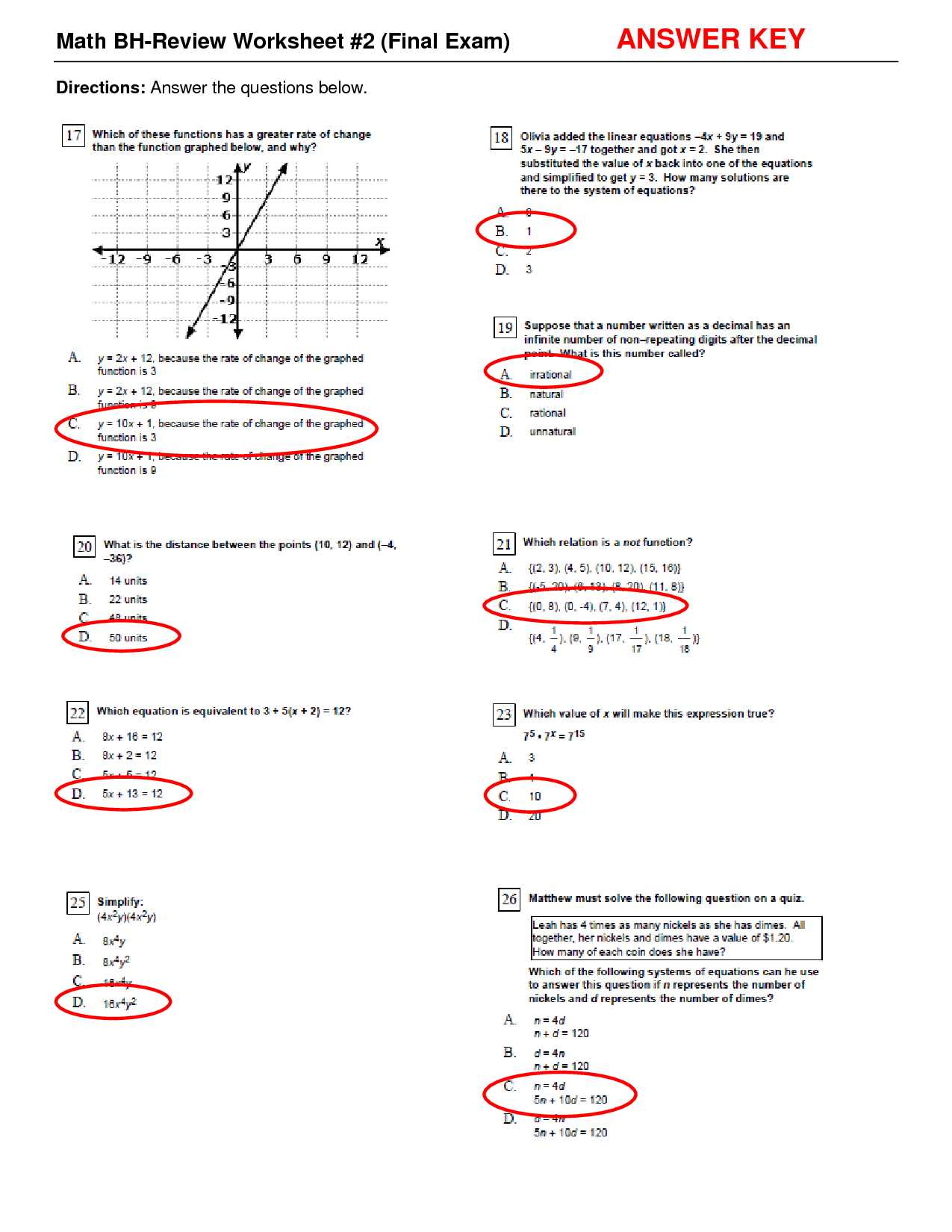
One of the most effective ways to prepare for any assessment is through consistent practice. Taking mock tests allows you to familiarize yourself with the format, manage your time more efficiently, and identify areas where further study is needed. This section explains the benefits of practice tests and how they can lead to better results when it matters most.

Benefits of Taking Practice Tests

Mock tests provide a controlled environment where you can simulate the real conditions of an assessment. This not only helps you become more comfortable with the structure but also boosts your confidence. The process of practicing under timed conditions helps reduce anxiety and prepares you to think quickly and efficiently.

Improves time management: Regularly practicing under time constraints helps you learn how to allocate your time more effectively during the actual assessment.
Identifies weak areas: By reviewing your performance on practice tests, you can pinpoint areas where you need further improvement or additional study.
Increases familiarity with question types: Practice tests expose you to various question formats, making it easier to recognize and tackle them during the actual assessment.

How to Use Practice Tests Effectively

To get the most out of your practice tests, it’s essential to approach them with a strategy. Don’t just take the test and forget about it–take time to analyze your mistakes and review the correct solutions. This process of reflection helps reinforce concepts and correct errors, improving your performance over time.

Simulate real conditions: Treat practice tests as if they were the actual assessment. This means following the same timing and environment as you would during the real test.
Review your mistakes: After completing a test, go over each incorrect answer to understand why it was wrong and how to approach similar problems in the future.
Track your progress: Keep track of your results from each practice test to monitor improvements and identify patterns in the areas where you are still struggling.

Using practice tests as part of your preparation will help you gain a deeper understanding of the material, sharpen your problem-solving skills, and improve your overall performance. The more you practice, the more confident and prepared you will be when the real test comes around.

Common Mistakes to Avoid in Math 2

When tackling complex problems, it’s easy to fall into common traps that can negatively impact your performance. These errors often arise from misunderstandings of concepts or careless mistakes during the process. Recognizing and avoiding these pitfalls can significantly improve your ability to solve problems accurately and efficiently. In this section, we will highlight some of the most frequent mistakes and provide tips on how to avoid them.

Misinterpreting the problem: One of the most common mistakes is failing to fully understand the question before attempting a solution. Always take a moment to read the problem carefully and identify the key elements before starting your calculations.
Rushing through calculations: Speed can be a factor in many mistakes. Taking the time to carefully perform each step is crucial. Skipping steps or rushing to the solution can result in avoidable errors.
Overlooking negative signs: Forgetting to account for negative numbers or signs in formulas can lead to incorrect answers. Always double-check that you’re considering all signs correctly, especially when working with equations or inequalities.
Not checking work: After solving a problem, it’s easy to move on without reviewing your solution. Taking the time to recheck each step can help catch any small mistakes that might have been overlooked initially.
Ignoring units: In problems that involve measurements or conversions, forgetting to include or convert units properly can result in incorrect answers. Always ensure that you’re using the right units and converting them as necessary.
Misapplying formulas: Using the wrong formula or applying it incorrectly is a common error. Be sure to understand which formula is applicable to the problem and how to use it correctly before proceeding.

By being aware of these common mistakes and taking proactive steps to avoid them, you can improve both your accuracy and confidence in solving problems. Focus on mastering the fundamentals, double-check your work, and approach each question with care to achieve the best results.

Reviewing Math 2 Exam Answers Effectively

After completing a test, it’s essential to take the time to carefully analyze your solutions. This process helps identify both strengths and weaknesses in your approach and ensures that you fully understand where any mistakes may have occurred. Reviewing your work is an invaluable tool for reinforcing your knowledge and improving your problem-solving skills for future assessments.

To conduct an effective review, focus on each question step-by-step. Examine both your correct and incorrect responses to identify patterns in your reasoning and calculation methods. By reflecting on your thought process, you can pinpoint specific areas that require further attention.

Analyze the steps: Go through each problem and rework the steps that led to your answer. If your solution was incorrect, determine where you deviated from the correct method and why.
Check for calculation errors: Small arithmetic mistakes are common and easy to overlook. Review your calculations thoroughly to ensure that every operation was performed accurately.
Verify your reasoning: Ensure that your reasoning aligns with the logic of the problem. If your solution doesn’t make sense, it may be helpful to approach the problem from a different angle.
Learn from mistakes: For each error, ask yourself what went wrong. Was it due to a misinterpretation of the question, a wrong formula, or an incorrect assumption? Use this insight to avoid similar mistakes in the future.

Revisiting your work and thoroughly analyzing each part will not only help you catch any overlooked mistakes but will also reinforce the concepts and methods that you applied correctly. This reflective practice is a powerful strategy for continuous improvement and long-term success.

Staying Calm During the Math 2 Test

During high-pressure assessments, maintaining composure is crucial for optimal performance. Anxiety and stress can cloud your thinking and lead to mistakes that you might otherwise avoid. It’s important to develop strategies that help you stay calm and focused throughout the test. By practicing mental relaxation techniques and approaching each problem methodically, you can manage any stress that arises and perform at your best.

One of the most effective ways to stay calm is by controlling your breathing. Deep, slow breaths can help reduce anxiety and improve your concentration. Additionally, pacing yourself and managing time wisely can prevent feelings of being overwhelmed. If you find yourself stuck on a question, it’s often better to move on and return to it later rather than getting frustrated.

Practice mindfulness: Take a moment before the test begins to focus on your breathing and clear your mind. This can help you approach the task with a calm and focused attitude.
Break the test into segments: Divide the time and questions into manageable sections. Focusing on one small part at a time will make the entire test feel less overwhelming.
Stay positive: Remind yourself that it’s okay not to know everything. Confidence in your abilities can help you stay relaxed and tackle each question with a clear mind.
Take short breaks: If allowed, use any brief moments to stand, stretch, or close your eyes. These quick breaks can help refresh your mind and reduce stress.

By cultivating these strategies and practicing them before the test, you can significantly reduce stress and increase your chances of success. Staying calm is not just about being relaxed–it’s about staying in control of your thoughts and actions, allowing you to tackle each challenge effectively.

Math 2 Exam Strategies for Faster Solutions

Speed and accuracy are key to performing well on time-sensitive assessments. The ability to solve problems quickly, without sacrificing correctness, can make a significant difference in achieving a high score. Implementing the right strategies allows you to approach questions with efficiency and confidence, ensuring that you maximize your time during the assessment.

One important aspect of improving speed is recognizing patterns and common techniques. Familiarizing yourself with frequently asked types of problems will allow you to instantly identify the most effective approach. Additionally, practicing shortcuts for common calculations or problem-solving methods can drastically reduce the time spent on each question.

Prioritize Simple Questions

Start by tackling the questions that appear the easiest or quickest to solve. This will not only help build momentum but will also give you more time to focus on the more complex problems later. Often, simpler questions are worth the same amount of points, so it’s essential to clear them quickly.

Master Time-Saving Techniques

Learning specific methods for reducing time on more complex problems is vital. For example, if a question involves a long equation, consider estimating values or using approximations to save time. If a geometric problem requires area calculations, use known formulas or shortcuts to avoid unnecessary steps.

Use elimination strategies: In multiple-choice questions, eliminate obviously incorrect answers to increase your chances of guessing the correct one if necessary.
Practice mental math: Work on quick mental calculations rather than relying on a calculator for every step. This reduces your dependence on external tools and speeds up your process.
Stay organized: Keep your work neat and structured so that you don’t waste time searching for the next step or revisiting past work.

By focusing on these strategies, you can effectively manage your time and tackle each problem with greater efficiency. Speed doesn’t mean rushing–it means being prepared and knowing when to apply the right method at the right time.

How to Check Your Answers Efficiently

Checking your work is a crucial step in ensuring accuracy during a timed assessment. However, this process should be done in a systematic and efficient way to avoid wasting precious time. The goal is not only to catch obvious mistakes but also to confirm that the method you applied is correct. Efficient checking techniques can help you review your responses without feeling rushed.

One effective approach is to check your calculations in reverse order. This allows you to quickly identify any errors by retracing your steps. Another strategy is to focus on the most significant parts of each problem, ensuring that you’ve addressed all necessary components before proceeding to the next task. Prioritize areas where mistakes are most likely to occur, such as complex formulas or multi-step problems.

Steps for an Efficient Review

To ensure that you check your work quickly and thoroughly, follow these steps:

Start with the most complex problems: Double-check your approach and solutions for complicated tasks first, as these are more likely to contain errors.
Use estimation: Before reviewing your detailed steps, make rough estimates to confirm that the general outcome seems reasonable.
Focus on units: Ensure that units and labels are consistent throughout the problem to avoid errors in interpretation.

Common Checking Techniques

There are several ways to double-check your work, and choosing the right method can save valuable time. Here are some effective techniques:

Technique	Description
Revisit Key Calculations	Review the major steps or calculations that were used to arrive at the final solution, looking for potential errors.
Check for Logical Consistency	Ensure that your answers are logical and consistent with the information provided in the problem.
Cross-Check with Other Problems	If possible, compare similar questions in the assessment to confirm if your approach and results align.

By using these techniques, you can efficiently check your work without feeling rushed. This process ensures that you minimize mistakes and maximize your performance in the assessment.

Building Confidence Before the Exam

Confidence plays a vital role in performing well under pressure. The way you prepare and approach the days leading up to a test can greatly impact your mindset and performance. Feeling confident isn’t just about knowing the material but also about trusting your abilities to apply what you’ve learned effectively. Building this self-assurance is crucial for success, especially when facing a challenging task.

To build confidence, it’s essential to focus on your strengths while actively addressing areas of improvement. Preparing in advance allows you to feel more in control and reduces feelings of anxiety. By practicing regularly and reinforcing positive thoughts, you can develop a mindset that encourages success and resilience, even when faced with difficulties.

Effective Ways to Boost Confidence

Here are a few strategies to help increase your confidence before the test:

Practice consistently: Regular practice helps reinforce concepts and boosts your sense of preparedness.
Review past successes: Remind yourself of past achievements to reinforce your capability and determination.
Stay positive: Visualize a successful outcome and remind yourself that challenges are opportunities to grow.

Importance of a Relaxed Mindset

Alongside solid preparation, having a calm and relaxed mindset is just as important. Stress and anxiety can hinder performance, so incorporating relaxation techniques such as deep breathing or light exercise can be helpful. Taking breaks during study sessions allows your mind to reset, ensuring that you stay sharp and focused when needed most.

Additional Resources for Math 2 Exam Preparation

Effective preparation for any test goes beyond just reviewing notes and practicing problems. Utilizing a variety of resources can help reinforce your understanding and provide different perspectives on the material. These resources not only offer additional practice but also give you access to tips, strategies, and explanations that can make complex topics easier to grasp. Exploring these tools can significantly improve your readiness and confidence before the test.

There are several types of resources available that cater to different learning styles. Whether you prefer video tutorials, interactive quizzes, or detailed textbooks, incorporating a mix of these tools into your study plan can enhance your preparation. Here are some popular resources to consider:

Online Platforms and Courses

Many online platforms offer structured courses and practice materials designed to help you master key concepts. These platforms often include video lectures, quizzes, and detailed solutions to problems, providing a comprehensive approach to studying.

Khan Academy: Offers free tutorials and practice exercises on a variety of topics with detailed explanations.
Coursera: Provides online courses from top universities with interactive components.
EdX: Features courses that range from beginner to advanced levels, with the option to earn certificates.

Books and Study Guides

Books and study guides remain a staple for any type of academic preparation. These resources provide in-depth coverage of topics and often include practice questions with answers and solutions.

Textbooks: Standard textbooks often provide clear explanations and examples to follow along with.
Study Guides: Specialized guides focus on specific tests and topics, summarizing essential concepts and offering tips for success.
Practice Problem Books: These books include a large number of practice questions with detailed solutions to help you practice under test-like conditions.

Interactive Tools

Interactive tools such as apps and websites allow you to practice in real-time, simulating actual test environments. These tools can help you track your progress and identify areas where further review is needed.

Quizlet: Allows you to create custom flashcards and quizzes for efficient learning and memorization.
Wolfram Alpha: A computational knowledge engine that can help solve problems and explain step-by-step solutions.
Brilliant: Offers interactive learning and problem-solving exercises for a deeper understanding of concepts.