Math Nation Algebra 2 Answers and Solutions

In this guide, we will explore effective methods to tackle complex mathematical challenges that often appear in higher-level courses. By focusing on clear explanations and step-by-step procedures, students can improve their problem-solving skills and gain a deeper understanding of the concepts involved.

Mastering key techniques is essential for tackling intricate problems. Whether you are grappling with equations, functions, or geometric concepts, understanding the fundamentals allows you to approach each task with confidence. This resource offers support for both practice and exam preparation, providing solutions that are easy to follow and applicable to real-world scenarios.

Improving your skills takes time and effort, but with the right strategies, any learner can succeed. In the sections ahead, we will dive into practical examples and helpful tips, designed to make even the most challenging topics more approachable.

Math Nation Algebra 2 Answers

In this section, we will explore effective solutions to challenging mathematical exercises commonly found in advanced courses. By breaking down problems into manageable steps, students can better understand the underlying principles and approach each problem with confidence. This guide offers clear solutions and useful strategies for mastering key concepts.

Problem	Solution	Explanation
Quadratic Equation	x = -b ± √(b² – 4ac) / 2a	Apply the quadratic formula to find the roots of the equation. Simplify to solve for x.
Rational Expression	Factor the numerator and denominator	Simplify the expression by factoring and canceling out common factors.
Linear System	x = (c – by) / a	Use substitution or elimination to solve for the unknown variables in a system of equations.

By following these examples and understanding the methods used, students can enhance their problem-solving skills and approach more complex topics with greater ease. Regular practice and a solid understanding of foundational concepts are key to mastering advanced mathematical topics.

Key Concepts in Algebra 2

Advanced mathematics requires a solid grasp of several essential principles that serve as the foundation for solving complex problems. These core concepts are crucial for understanding how different types of equations and functions behave and how they can be manipulated to find solutions. In this section, we will explore some of the most important ideas and techniques that students should master to succeed in this subject.

Understanding Functions and Graphs

One of the central concepts in advanced mathematics is the idea of a function. Functions describe the relationship between two sets of numbers, where each input corresponds to a single output. Understanding how to graph these relationships is crucial for visualizing the behavior of different mathematical models. Key points include recognizing function types such as linear, quadratic, and exponential, and knowing how to plot their graphs accurately.

Solving Systems of Equations

Another fundamental concept is solving systems of equations, which involves finding the values of variables that satisfy multiple equations at once. This can be done through methods like substitution, elimination, or graphing. The ability to solve these systems is vital for understanding how different variables interact and for finding solutions to real-world problems modeled by multiple equations.

Mastering these concepts enables students to approach more complex mathematical problems with confidence, laying the groundwork for success in further studies or applied fields.

How to Use Math Nation Effectively

To succeed in advanced mathematics, it’s essential to approach learning tools strategically. The platform provides a variety of resources designed to support students in mastering difficult topics. By understanding how to navigate and utilize these features, learners can maximize their study time and enhance their understanding of complex mathematical concepts.

Start by familiarizing yourself with the structure of the platform. It offers step-by-step solutions, practice exercises, and video tutorials that cater to different learning styles. Focus on the areas where you feel least confident and work through related exercises to reinforce your understanding. Regular practice is key to improving performance and developing a deeper comprehension of the material.

Additionally, take advantage of the progress tracking features to monitor your improvement over time. This can help identify areas that need more attention and ensure you’re on the right track. Consistent use of the platform, combined with a proactive approach, will greatly enhance your problem-solving skills and overall mathematical proficiency.

Common Algebra 2 Problems Solved

Advanced mathematics presents a variety of challenges, but many problems can be broken down into manageable steps. By recognizing the common patterns in equations and functions, students can apply consistent strategies to find solutions. In this section, we’ll address several typical problems and demonstrate how to solve them effectively.

Solving Quadratic Equations

Quadratic equations are among the most frequently encountered problems. These can typically be solved using the quadratic formula or by factoring. For example, given a standard equation of the form ax² + bx + c = 0, apply the formula x = (-b ± √(b² – 4ac)) / 2a to find the roots. Factoring, when applicable, involves rewriting the equation in terms of two binomials that multiply to equal the original expression.

Simplifying Rational Expressions

Rational expressions often require simplification to make them easier to work with. This can be done by factoring both the numerator and the denominator and canceling out any common factors. For instance, in the expression (x² – 9) / (x + 3), factoring the numerator as (x – 3)(x + 3) allows the (x + 3) terms to cancel, leaving (x – 3) as the simplified expression.

By following systematic approaches like these, students can tackle a wide range of problems and build a strong foundation for more complex topics in the future.

Step-by-Step Math Nation Solutions

Solving complex mathematical problems becomes much easier when broken down into clear, manageable steps. By following a structured approach, students can understand the logic behind each solution and apply the same methods to different types of problems. This section will provide detailed step-by-step instructions for solving some common challenges.

Solving Linear Equations

To solve a linear equation, start by isolating the variable. For example, in the equation 2x + 5 = 15, subtract 5 from both sides to get 2x = 10. Next, divide both sides by 2 to find x = 5. This process can be applied to any linear equation, making it a straightforward method for solving for unknown variables.

Working with Exponents

When working with exponents, it’s important to remember the basic rules: multiply exponents when multiplying like bases and subtract exponents when dividing. For example, for the expression x³ * x², apply the rule of adding exponents to get x⁵. Similarly, when dividing x⁶ / x², subtract the exponents to get x⁴. Mastering these exponent rules simplifies many problems involving powers.

By breaking down problems into these simple, logical steps, students can develop a stronger grasp of mathematical concepts and approach more difficult exercises with confidence.

Mastering Functions in Algebra 2

Understanding how functions work is fundamental to excelling in higher-level mathematics. Functions represent relationships between two sets of values, where each input corresponds to one unique output. Mastering this concept opens the door to solving more advanced problems and applying mathematical reasoning to real-world scenarios.

Key to success in working with functions is understanding their types and properties. Functions can take many forms, such as linear, quadratic, exponential, or logarithmic, and each type behaves differently. Recognizing these differences and knowing how to manipulate each function type is essential for solving related problems accurately and efficiently.

Graphing and transformations are also crucial elements when mastering functions. Understanding how to shift, stretch, or reflect the graph of a function allows for a deeper understanding of its behavior. By analyzing the graph, students can identify key features such as the domain, range, and intercepts, which are all important for solving function-based equations.

Algebra 2 Word Problems Explained

Word problems in advanced mathematics can be challenging, but they provide a valuable opportunity to apply theoretical concepts to real-world situations. The key to solving these problems is translating the words into mathematical expressions and equations that can be solved systematically. In this section, we will break down the process of tackling word problems step-by-step.

Breaking Down the Problem

Start by carefully reading the problem and identifying the key information provided. Often, word problems contain unnecessary details that can be ignored. Focus on the quantities, relationships, and conditions mentioned. For instance, if the problem involves the total cost of items, determine the variables that represent price, quantity, and any other factors involved. Once the important elements are identified, translate them into an equation or set of equations.

Solving and Interpreting the Results

After setting up the equation, solve for the unknowns using the appropriate methods. This may involve operations like substitution, elimination, or factoring. Once the solution is obtained, interpret the results in the context of the problem. Ensure that the answer makes sense logically and addresses the question being asked. If necessary, check the solution by substituting the values back into the original conditions of the problem.

By following a clear process and practicing regularly, students can build confidence in solving word problems and develop strong problem-solving skills that are applicable in a variety of scenarios.

Understanding Quadratic Equations

Quadratic equations are fundamental to many areas of advanced mathematics and are frequently encountered in various fields. These equations represent parabolic relationships and can be solved using several different methods. By grasping the underlying principles of quadratic equations, students can solve a wide variety of problems with confidence and accuracy.

A typical quadratic equation takes the form ax² + bx + c = 0, where a, b, and c are constants. The solutions to such equations, also known as the roots, can be found using the quadratic formula, factoring, or completing the square. Each of these methods offers a different approach, but they all ultimately lead to the same result.

Understanding how to identify and manipulate the components of quadratic equations is crucial for success in more advanced topics. By recognizing the standard form and being familiar with the various solving techniques, students can efficiently tackle problems that involve quadratic relationships and apply their knowledge to both theoretical and real-world applications.

Tips for Solving Polynomial Equations

Solve polynomial equations by carefully applying fundamental strategies. These equations often involve terms with multiple degrees, making them more complex than linear ones. However, by following systematic steps and using appropriate techniques, solving these equations becomes manageable and efficient.

Factoring the Polynomial

One of the most effective methods for solving polynomial equations is factoring. If the polynomial is factorable, you can break it down into simpler binomials or trinomials. For instance, a quadratic polynomial like x² – 5x + 6 can be factored into (x – 2)(x – 3). Once factored, set each factor equal to zero and solve for the variable.

Using the Rational Root Theorem

For higher-degree polynomials, the Rational Root Theorem can be helpful in identifying possible rational roots. This theorem suggests that any rational root of the polynomial equation must be a factor of the constant term divided by a factor of the leading coefficient. Testing these potential roots can help reduce the degree of the polynomial, simplifying the problem.

By systematically applying these methods and practicing different types of polynomials, you will develop a strong understanding and improve your ability to solve polynomial equations efficiently.

Equation	Method	Solution
x² – 5x + 6	Factoring	(x – 2)(x – 3)
x³ – 6x² + 11x – 6	Rational Root Theorem	(x – 1)(x – 2)(x – 3)

Graphing Techniques in Algebra 2

Graphing is a powerful tool for visualizing mathematical relationships and understanding how equations behave. Whether dealing with linear, quadratic, or higher-degree functions, knowing the right techniques to graph these equations will help you interpret their behavior and solve problems more effectively. This section covers essential graphing methods that are commonly used in more advanced mathematical studies.

One of the first things to understand when graphing any equation is its general shape. For example, linear functions create straight lines, while quadratic functions produce parabolas. Identifying the function type will guide you in applying the correct method for plotting points and understanding key features such as intercepts, vertices, and asymptotes.

Equation Type	Graph Shape	Key Features
Linear	Straight Line	Slope, Y-Intercept
Quadratic	Parabola	Vertex, Axis of Symmetry
Cubic	Curve	Inflection Point

Understanding the basic graphing techniques, such as plotting points, identifying intercepts, and recognizing symmetry, forms the foundation for analyzing more complex equations. Once you’ve mastered these, you can apply transformations such as translations, reflections, and stretches to manipulate the graph and solve related problems more efficiently.

Math Nation Algebra 2 Practice Tests

Practice tests are a valuable resource for reinforcing concepts and preparing for assessments. These tests provide an opportunity to apply what you’ve learned in a simulated exam environment, helping you identify areas of strength and pinpoint concepts that need further review. In this section, we will explore how to effectively use practice tests to improve problem-solving skills and test performance.

Benefits of Practice Tests

Taking practice tests allows students to familiarize themselves with the types of questions they may encounter in actual exams. It also helps to build test-taking strategies, such as time management and question prioritization. Regular practice not only boosts confidence but also provides immediate feedback, allowing you to assess your understanding of key topics and make necessary adjustments to your study plan.

Maximizing Your Practice Sessions

To get the most out of practice tests, it’s essential to approach them with a focused mindset. First, simulate real exam conditions by setting a time limit and eliminating distractions. After completing the test, thoroughly review both correct and incorrect answers. Take the time to understand why a particular answer is correct or incorrect, and rework the problem if needed. This reflective process helps reinforce your understanding and improves retention.

How to Approach Rational Expressions

Rational expressions are fractions where the numerator and denominator are both polynomials. Solving these expressions can be challenging, but with the right approach, it becomes manageable. The key to working with rational expressions is understanding their structure and applying systematic methods for simplifying, adding, subtracting, multiplying, and dividing them.

Simplifying Rational Expressions

The first step when working with rational expressions is often simplification. To simplify, identify and factor common terms in both the numerator and the denominator. Cancel out any common factors, keeping in mind that division by zero is not allowed.

• Factor both the numerator and denominator completely.
• Cancel out any common factors between the numerator and denominator.
• Ensure that no denominator contains zero after simplification.

Operations with Rational Expressions

Once simplified, performing operations with rational expressions follows similar rules to regular fractions. Each operation–whether it’s addition, subtraction, multiplication, or division–requires specific steps to ensure accuracy.

1. Addition/Subtraction: Find a common denominator before adding or subtracting the expressions.
2. Multiplication: Multiply the numerators and denominators directly, then simplify if possible.
3. Division: Multiply by the reciprocal of the divisor.

By carefully following these steps, rational expressions can be manipulated and solved accurately. Practice and familiarity with different forms of these expressions will build confidence and improve problem-solving skills.

Exponential and Logarithmic Functions

Exponential and logarithmic functions are closely related, yet they serve different purposes in mathematics. Exponential functions involve constants raised to a variable power, while logarithmic functions are the inverse of exponentiation. Understanding how these two types of functions work is crucial for solving complex equations and modeling real-world phenomena, such as population growth, radioactive decay, and financial interest calculations.

Exponential Functions

Exponential functions are characterized by having a constant base raised to a variable exponent. These functions are commonly used to describe growth or decay processes. The general form of an exponential function is:

• y = a * b^x
• where a is a constant, b is the base (a positive real number), and x is the exponent (a variable).

Exponential functions can exhibit rapid growth or decay depending on whether the base is greater than or less than 1. They are particularly useful in fields such as biology, economics, and physics.

Logarithmic Functions

Logarithmic functions are the inverse of exponential functions. They answer the question: “To what power must the base be raised to obtain a given number?” The general form of a logarithmic function is:

• y = log_b(x)
• where b is the base, and x is the number for which the logarithm is being calculated.

Logarithms are essential in solving equations where the unknown appears as an exponent, and they are frequently used in applications involving scale, such as in the Richter scale for measuring earthquakes or pH levels in chemistry.

Both exponential and logarithmic functions play vital roles in understanding and solving mathematical problems. Mastery of these functions allows for more efficient problem-solving and helps in interpreting various phenomena in science and engineering.

Solving Systems of Equations

When dealing with multiple equations that involve the same set of variables, finding the solution to these systems is a key mathematical skill. A system of equations represents several conditions that must be met simultaneously. Solving these systems requires identifying values for the variables that satisfy all the given equations at once. There are various methods to approach this, each suited to different types of problems.

Methods for Solving Systems

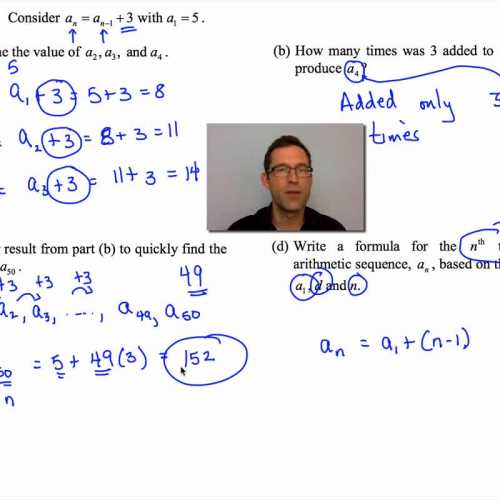
There are several strategies to solve a system of equations, including substitution, elimination, and graphing. Each method has its advantages depending on the structure of the system. Choosing the right approach can simplify the process and lead to a faster solution.

• Substitution Method: Solve one equation for one variable and substitute this expression into the other equation to solve for the second variable.
• Elimination Method: Add or subtract equations to eliminate one variable, allowing you to solve for the remaining variable.
• Graphing Method: Plot both equations on the coordinate plane and identify the point where the lines intersect, which represents the solution to the system.

Applications of Solving Systems

Systems of equations are not just theoretical problems; they have real-world applications in various fields such as economics, engineering, and computer science. For example, systems of equations can be used to model situations involving resource allocation, supply and demand, or electrical circuits. Understanding how to solve these systems allows for better decision-making and problem-solving in practical scenarios.

By mastering these techniques, you can efficiently solve for the unknowns in a variety of situations, both mathematical and practical. Whether working with linear or nonlinear systems, these methods are essential tools in your mathematical toolkit.

Algebra 2 Test Preparation Tips

Preparing for a test that involves advanced mathematical concepts requires a focused approach. Whether you are reviewing for a final exam or preparing for a smaller assessment, having the right strategies can make all the difference. Developing effective study habits, understanding the types of questions, and practicing problem-solving techniques are crucial steps in achieving success.

Study Strategies for Success

To ensure that you’re well-prepared for the test, consider these key strategies:

• Organize Your Study Sessions: Break your study time into manageable blocks with specific goals for each session. Focus on one concept at a time and ensure that you fully understand it before moving on.
• Review Key Concepts: Identify the main topics covered on the test, such as functions, equations, and graphing. Revisit these areas to ensure you have a strong grasp of the underlying principles.
• Practice with Sample Problems: The best way to prepare is through practice. Work through a variety of problems that reflect the format and difficulty level of the test questions.

Maximize Your Performance on Test Day

Once the test day arrives, there are additional tips that can help you perform your best:

• Read Each Question Carefully: Take your time to understand what each question is asking. Misunderstanding the question can lead to avoidable mistakes.
• Show Your Work: Writing out the steps of your calculations helps ensure that you don’t miss any key steps, and can help you spot errors along the way.
• Stay Calm and Confident: Test anxiety can impair performance, so take deep breaths and stay calm. Trust in your preparation and approach each problem with confidence.

By following these strategies and preparing thoroughly, you’ll be in a strong position to succeed on your test and deepen your understanding of the material. The more you practice and review, the more confident you’ll feel on exam day.

Common Mistakes to Avoid in Algebra 2

When working through advanced mathematical problems, it’s easy to make errors that can hinder your understanding and performance. Recognizing common pitfalls and learning how to avoid them can significantly improve your problem-solving skills. By addressing these mistakes early, you can strengthen your overall grasp of the concepts and approach each question with greater confidence.

Top Mistakes to Watch Out For

Here are some of the most frequent errors students make when tackling complex equations and functions:

• Misunderstanding Order of Operations: Always follow the proper order of operations (PEMDAS). Skipping steps or performing operations out of sequence can lead to incorrect results.
• Failing to Simplify Expressions: Many students forget to simplify expressions before moving on to the next step. This can lead to unnecessary complexity and mistakes in later stages of the problem.
• Overlooking Negative Signs: A simple mistake, like misplacing a negative sign, can drastically change the outcome. Be especially careful when dealing with subtraction or negative numbers.
• Incorrectly Applying Formulas: Using the wrong formula or misapplying a correct formula is a common error. Always double-check which formula you need and understand how it applies to the problem.

How to Avoid These Errors

Here are a few tips to prevent these mistakes from affecting your work:

• Take Your Time: Don’t rush through problems. It’s easy to make simple mistakes when you’re working too quickly. Take the time to check your work after each step.
• Break Down Complex Problems: When faced with complicated problems, break them down into smaller, more manageable parts. This will help you maintain clarity and ensure accuracy throughout the solution process.
• Double-Check Your Work: After solving a problem, take a moment to go over your work and verify that each step is correct. This can help catch any small mistakes that may have been overlooked.
• Practice Regularly: The more you practice, the more familiar you’ll become with common errors. Through repetition, you’ll develop a keen eye for spotting mistakes before they become an issue.

By being aware of these common mistakes and following these strategies, you can avoid unnecessary setbacks and improve your performance in advanced mathematics.

How Math Nation Helps Students Succeed

In today’s competitive academic environment, students often seek resources that can help them better understand complex concepts and achieve success. Educational platforms provide a wealth of tools, but some stand out for their ability to offer structured support, practice materials, and interactive learning experiences. One such platform aids students in mastering their coursework by breaking down challenging problems, offering guidance, and fostering a deeper understanding of critical subjects.

Key Benefits of Using the Platform

Here’s how the platform contributes to student success:

• Interactive Learning Modules: Engaging lessons allow students to work through problems step by step, reinforcing key concepts and promoting active participation in their learning process.
• Practice Opportunities: Regular practice is essential for mastering any subject. The platform provides a variety of exercises to strengthen knowledge and ensure retention of learned material.
• Instant Feedback: Immediate feedback on practice questions helps students identify mistakes and understand where they went wrong, enabling them to improve and correct misconceptions early.
• Detailed Explanations: In-depth explanations and tutorials break down difficult topics, providing students with a clearer understanding of how to approach problems effectively.

Additional Features to Enhance Learning

Aside from basic lessons and exercises, the platform offers several additional features that help students build confidence and stay motivated:

• Personalized Study Plans: Customizable study plans ensure that students focus on areas where they need the most improvement, tailoring the experience to individual learning needs.
• Peer Collaboration: The ability to collaborate with peers allows students to discuss concepts and share different approaches to problem-solving, creating a supportive learning environment.
• Progress Tracking: Tracking progress over time helps students see their improvements, boosting their confidence and encouraging continued effort.

By combining these features, the platform provides a comprehensive approach to mastering difficult topics, helping students gain the skills they need to succeed academically and beyond.