Algebra 2 Module 1 Answers and Solutions
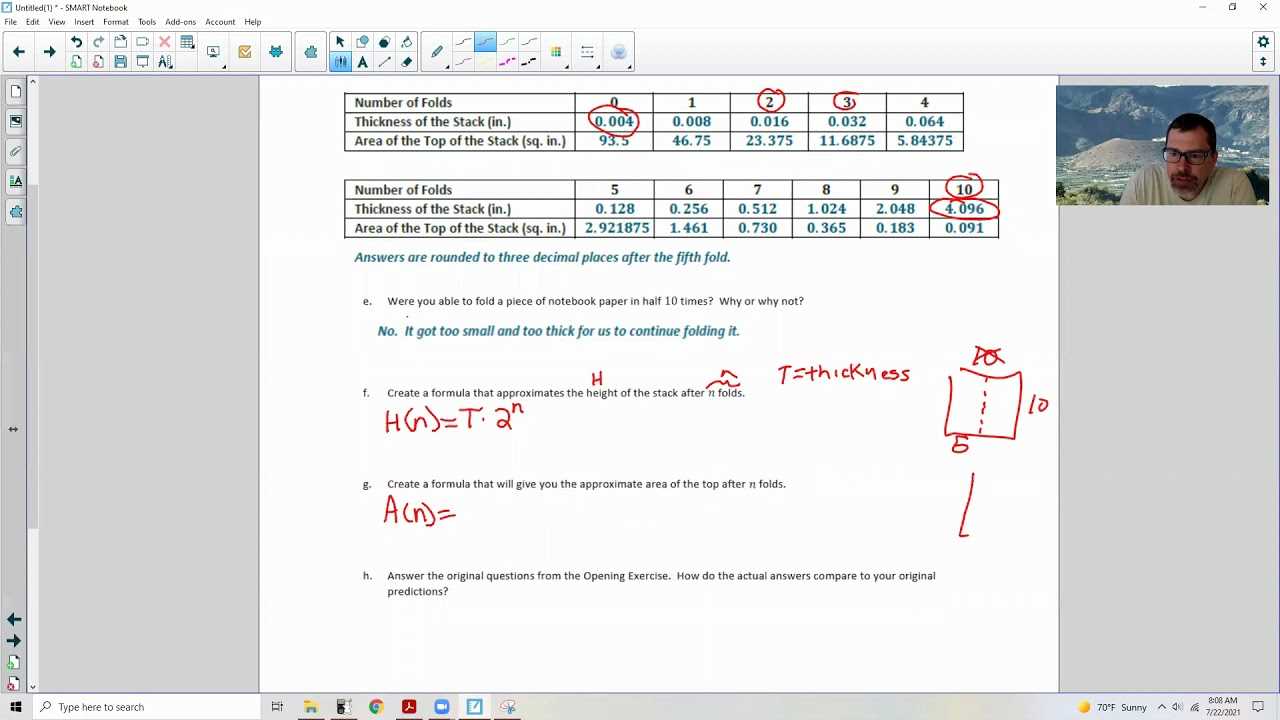
In this section, we explore the foundational topics of higher-level mathematics that provide essential skills for solving complex problems. The content focuses on strengthening your problem-solving abilities through various techniques and strategies that apply to different mathematical challenges. Mastery of these concepts is crucial for progressing through more advanced levels of study.
Clear step-by-step instructions will guide you through each concept, ensuring that you not only learn the procedures but also understand the reasoning behind them. Whether dealing with equations, functions, or inequalities, this material helps build a strong mathematical foundation that you can apply to various situations, both in academic settings and in real-world scenarios.
Algebra 2 Module 1 Answers
This section focuses on providing solutions to key mathematical problems, offering a deeper understanding of complex concepts. By working through each problem, you will learn the techniques needed to approach similar challenges with confidence. The goal is to help you grasp the methods that underlie various mathematical operations, making it easier to solve future problems independently.
Each solution is broken down into clear steps to highlight the logical flow of problem-solving. From working with variables to solving equations, these explanations aim to build a comprehensive understanding that supports your progress in higher-level mathematics. Mastering these techniques will not only help with coursework but also provide practical skills for real-world applications.
Understanding Key Concepts in Algebra 2
Mastering the core principles of advanced mathematics is essential for solving a wide range of problems. By focusing on the fundamental techniques, students can build a solid foundation that will support more complex topics in the future. Whether dealing with equations, inequalities, or functions, understanding these core concepts is critical to progressing in this subject.
Key Mathematical Operations
Before diving into more complicated exercises, it’s important to first familiarize yourself with the basic operations that form the basis of more advanced work. These foundational skills include simplifying expressions, solving linear and quadratic equations, and understanding the properties of functions.
| Operation | Example | Explanation |
|---|---|---|
| Simplification | 3x + 5x | Combine like terms to get 8x |
| Solving Equations | x + 4 = 10 | Subtract 4 from both sides to find x = 6 |
| Factoring | x² – 9 | Factor into (x – 3)(x + 3) |
Advanced Techniques for Problem Solving
Once the basics are in place, it’s time to explore more advanced methods for solving problems. These include working with complex numbers, exploring logarithmic functions, and understanding polynomial division. These techniques are often used to solve real-world problems and provide a deeper understanding of the subject.
How to Solve Polynomial Equations
Solving polynomial equations involves finding the values of variables that satisfy the equation. These equations can vary in complexity, but the core methods for solving them remain consistent. Whether the polynomial is quadratic, cubic, or of higher degree, the process generally involves simplifying the equation and applying various techniques such as factoring, using the quadratic formula, or graphing.
To begin solving these equations, it’s important to recognize the structure of the polynomial and determine which method will be most effective. Factoring is often the first step when the equation is factorable, but other methods may be necessary depending on the complexity of the terms involved.
| Method | Example | Explanation |
|---|---|---|
| Factoring | x² – 5x + 6 = 0 | Factor into (x – 2)(x – 3) = 0, then solve for x = 2, 3 |
| Quadratic Formula | x² + 4x + 3 = 0 | Use the formula x = [-b ± √(b² – 4ac)] / 2a to find x = -1, -3 |
| Graphing | y = x³ – 6x² + 11x – 6 | Plot the equation on a graph and find the x-intercepts, which are the solutions |
Factoring Strategies for Algebra 2
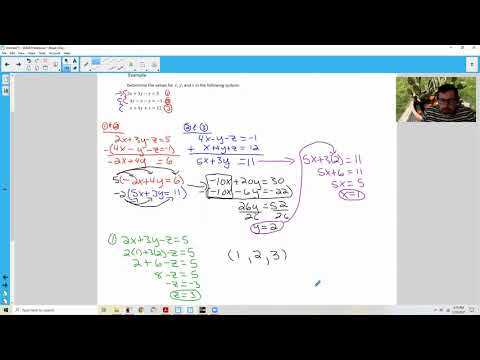
Factoring is a crucial technique for simplifying expressions and solving equations. It involves rewriting a polynomial as the product of simpler polynomials, making it easier to find solutions. Understanding various factoring methods is essential for tackling more advanced problems efficiently. This section will cover key strategies that can be applied to different types of polynomials.
Common Factoring Methods

There are several methods for factoring polynomials, each suitable for different types of expressions. The most common methods include factoring by grouping, using the difference of squares, and factoring trinomials. Knowing when to apply each method can make the process more straightforward and help you solve equations more quickly.
| Method | Example | Explanation |
|---|---|---|
| Factoring by Grouping | x² + 5x + 6x + 30 | Group terms: (x² + 5x) + (6x + 30), then factor out common terms: x(x + 5) + 6(x + 5) to get (x + 5)(x + 6) |
| Difference of Squares | x² – 9 | Rewrite as (x – 3)(x + 3) |
| Factoring Trinomials | x² + 7x + 12 | Look for two numbers that multiply to 12 and add up to 7, such as 3 and 4. Factor as (x + 3)(x + 4) |
Advanced Factoring Techniques
In addition to the basic methods, there are also more advanced techniques for factoring higher-degree polynomials or expressions with more complex structures. These include the use of synthetic division, the rational root theorem, and factoring by substitution. Mastering these techniques allows for handling more challenging problems with greater ease.
Working with Rational Expressions
Rational expressions involve fractions where the numerator and denominator are both polynomials. Simplifying, multiplying, dividing, and adding these expressions require a solid understanding of how to manipulate polynomials and fractions. Mastery of these skills is essential for solving a variety of mathematical problems, from basic to advanced levels.
Simplifying Rational Expressions
Simplification of rational expressions often begins by factoring both the numerator and the denominator. This process eliminates common factors, reducing the expression to its simplest form.
- Factor the numerator and denominator separately.
- Cancel any common factors between the numerator and denominator.
- Rewrite the expression with the remaining terms.
Operations with Rational Expressions
When working with these expressions, it’s important to apply the correct operations–addition, subtraction, multiplication, or division–while carefully considering the restrictions placed by the denominators.
- Multiplying: Multiply the numerators together and the denominators together.
- Dividing: Invert the second fraction and multiply.
- Adding and Subtracting: Find a common denominator, then add or subtract the numerators.
| Operation | Example | Explanation |
|---|---|---|
| Simplifying | (x² – 4) / (x² – 2x) | Factor both: (x – 2)(x + 2) / x(x – 2), cancel the common factor (x – 2), leaving (x + 2) / x |
| Multiplying | (x + 1) / (x – 3) * (x – 3) / (x + 2) | Multiply the numerators and denominators: (x + 1)(x – 3) / (x – 3)(x + 2), cancel (x – 3), leaving (x + 1) / (x + 2) |
| Adding | 1 / (x + 1) + 2 / (x + 1) | Both fractions have the same denominator, so add the numerators: (1 + 2) / (x + 1) = 3 / (x + 1) |
Solving Linear Systems in Algebra 2
Solving systems of linear equations is an essential skill in advanced mathematics, as it allows for the determination of multiple variables that satisfy several equations simultaneously. The process involves finding the values of the unknowns that make all the equations in the system true at the same time. There are various methods to solve these systems, each suited to different types of problems.
Among the most common methods are substitution, elimination, and graphing. Each method offers unique advantages depending on the structure of the equations involved. Substitution is ideal when one equation is easy to solve for a single variable, while elimination is effective when adding or subtracting equations leads to cancellation of a variable. Graphing can provide a visual representation of the solutions, which is particularly useful for understanding the relationships between the equations.
By mastering these techniques, students can solve a wide range of problems, from basic to more complex systems with multiple variables. Understanding how to efficiently choose and apply the right method will save time and improve accuracy when working with linear systems.
Graphing Functions and Their Properties
Graphing is a powerful tool for understanding mathematical relationships and visualizing the behavior of different equations. By plotting functions on a coordinate plane, we can observe key features such as intercepts, symmetry, and the overall shape of the graph. Recognizing these properties helps in analyzing and solving problems more effectively.
When graphing a function, it’s important to identify several key characteristics that reveal important information about its behavior. These properties include:
- Intercepts: Points where the graph crosses the x-axis (roots) and y-axis (y-intercept).
- Domain and Range: The set of possible input values (domain) and output values (range) for the function.
- Symmetry: Identifying whether the graph is symmetric with respect to the y-axis (even functions) or the origin (odd functions).
- Asymptotes: Lines that the graph approaches but never actually reaches.
Types of Functions and Their Graphs
Different types of functions have distinct graph shapes and behaviors. Some common types include linear, quadratic, cubic, and exponential functions. Understanding the basic form of each function can help predict its graph and properties.
- Linear Functions: Graphs are straight lines with constant slope.
- Quadratic Functions: Parabolas that open upward or downward depending on the coefficient of the x² term.
- Exponential Functions: Curves that increase or decrease rapidly based on the base of the exponent.
By recognizing these patterns and understanding how the equations relate to their graphs, solving problems and predicting behavior becomes much easier.
Quadratic Equations and Their Solutions
Quadratic equations are a fundamental type of polynomial equation where the highest power of the variable is squared. These equations often arise in various real-world applications, including physics, engineering, and finance. The process of solving these equations is essential for finding the values of the unknowns that satisfy the given relationship.
There are several methods to solve quadratic equations, depending on the specific form of the equation. Common techniques include factoring, completing the square, and using the quadratic formula. Each method has its advantages based on the complexity and the given equation. For example, factoring is quick when the equation can be easily factored, while the quadratic formula is a reliable approach when the equation is difficult to factor or when exact solutions are needed.
Solving by Factoring: This method is useful when the equation can be rewritten as the product of two binomials. After setting each factor equal to zero, the solutions are found by solving for the variable.
Completing the Square: This method involves rearranging the equation so that the left-hand side becomes a perfect square trinomial. From there, you can solve for the variable by taking the square root of both sides.
Using the Quadratic Formula: The quadratic formula is a universal method for solving any quadratic equation. Given a standard equation of the form ax² + bx + c = 0, the solutions can be found using the formula:
x = (-b ± √(b² – 4ac)) / 2a
This formula will always provide the correct solutions, whether they are real or complex, and can be applied to all quadratic equations.
Solving Inequalities in Algebra 2
Solving inequalities involves finding the range of values that satisfy a given condition, rather than a single solution. These problems are crucial in various fields such as economics, physics, and engineering, where constraints or limits are often involved. Inequalities can be solved similarly to equations, but with additional considerations when manipulating them, particularly with negative numbers.
The process typically involves isolating the variable on one side of the inequality, much like solving an equation. However, there are some key differences, such as flipping the inequality sign when multiplying or dividing by a negative number. Understanding these rules is essential for obtaining accurate solutions.
To solve linear inequalities, the following steps are often applied:
- Step 1: Simplify both sides of the inequality, if needed.
- Step 2: Isolate the variable on one side by performing algebraic operations.
- Step 3: If multiplying or dividing by a negative number, remember to reverse the inequality sign.
- Step 4: Express the solution as an interval or graph it on a number line.
When dealing with quadratic or higher-order inequalities, the process may involve factoring or using a sign chart to determine the intervals where the inequality holds true. These methods allow for a comprehensive understanding of where the solutions lie within a given range.
Exploring Exponential Functions
Exponential functions are mathematical expressions where a variable appears in the exponent. These functions model a wide range of real-world phenomena, such as population growth, radioactive decay, and interest calculations. The key feature of an exponential function is its constant rate of growth or decay, which makes it distinct from linear functions that increase or decrease at a steady rate.
In general, an exponential function can be represented as:
f(x) = a * b^x
Where “a” is a constant that determines the initial value, “b” is the base that determines the growth or decay rate, and “x” is the exponent. If the base “b” is greater than 1, the function shows exponential growth; if the base is between 0 and 1, it shows exponential decay.
Key properties of exponential functions include:
- Initial Value: The value of the function when x = 0, typically represented by “a.”
- Growth or Decay Rate: The value of “b” determines whether the function grows or decays.
- Horizontal Asymptote: The function approaches a specific value as x increases or decreases, but never actually reaches it.
- Domain and Range: The domain of an exponential function is all real numbers, while the range is positive real numbers (if b > 0).
Applications of Exponential Functions
Exponential functions are used to model various real-life situations. Some common applications include:
- Population Growth: When a population increases at a constant percentage rate over time.
- Compound Interest: Used in finance to calculate the growth of investments over time.
- Radioactive Decay: Describes the decrease in the quantity of a substance over time due to radioactive decay.
By understanding the behavior and applications of exponential functions, we can better model and solve real-world problems involving growth and decay.
Systems of Equations and Word Problems
Systems of equations are sets of two or more equations that share common variables. Solving these systems allows us to find the values of the unknowns that satisfy all the equations simultaneously. These problems are common in various fields, such as economics, engineering, and physics, where multiple relationships need to be understood and solved at the same time.
There are different methods for solving systems of equations, including substitution, elimination, and graphing. Each method offers a different approach to finding the solution, depending on the structure of the equations. In many cases, solving these systems is essential for addressing real-life scenarios, where multiple conditions must be met simultaneously.
Solving by Substitution: This method involves solving one equation for one variable and substituting that expression into the other equation. This approach works best when one of the equations is easy to manipulate.
Solving by Elimination: In this method, the goal is to eliminate one variable by adding or subtracting the equations. This approach is especially useful when the equations are aligned in such a way that one variable can be canceled out.
Graphing: Graphing involves plotting the equations on a coordinate plane and finding the point where the graphs intersect. This method provides a visual representation of the solution, which can be especially helpful for understanding the relationship between the variables.
Word Problems Involving Systems
Word problems that involve systems of equations require translating a real-world situation into mathematical equations. These types of problems often involve scenarios where two or more conditions must be satisfied simultaneously, such as determining the number of items sold, the cost of materials, or the distribution of resources.
For example, a typical word problem could involve two people selling tickets for a fundraiser. One person sells tickets at one price, while the other sells them at a different price. The goal would be to determine how many tickets each person sold to achieve a specific total amount raised. Solving the system of equations derived from this scenario provides the necessary answers to the problem.
Working with Complex Numbers
Complex numbers extend the concept of numbers to include solutions that do not exist within the real number system. They consist of two parts: a real part and an imaginary part. These numbers are essential for solving problems in various fields, particularly in engineering, physics, and signal processing, where real solutions are not always sufficient.
The standard form of a complex number is written as a + bi, where a represents the real part and bi represents the imaginary part. The symbol i is used to denote the square root of -1, which is the foundation of complex numbers. Understanding how to manipulate and perform operations with these numbers is vital for tackling problems that involve non-real solutions.
There are several key operations when working with complex numbers:
- Addition: To add two complex numbers, simply add their real and imaginary parts separately. For example, (3 + 2i) + (1 + 4i) = (3 + 1) + (2i + 4i) = 4 + 6i.
- Subtraction: Subtraction follows the same rule as addition, subtracting the real parts and the imaginary parts individually.
- Multiplication: To multiply complex numbers, apply the distributive property and use the fact that i^2 = -1 to simplify the result.
- Division: Dividing complex numbers involves multiplying both the numerator and the denominator by the conjugate of the denominator to eliminate the imaginary part from the denominator.
Complex numbers are also commonly represented on the complex plane, where the x-axis represents the real part and the y-axis represents the imaginary part. This graphical representation helps visualize the addition, subtraction, and multiplication of complex numbers, making it easier to understand their properties and operations.
Understanding Logarithmic Functions
Logarithmic functions are an essential class of functions that are the inverse of exponential functions. These functions are widely used in solving equations involving exponential growth or decay, such as in finance, biology, and physics. Understanding how these functions behave and how to manipulate them is crucial for solving various real-world problems.
In its general form, a logarithmic function is written as f(x) = logb(x), where b is the base of the logarithm, and x is the argument. The base b represents the number that is raised to a power to produce x. For example, in the function log10(1000), the base is 10, and the question is asking: “What power should 10 be raised to in order to get 1000?” The answer, in this case, is 3, since 103 = 1000.
There are a few important properties of logarithms that are commonly used in solving equations:
- Product Property: logb(x * y) = logb(x) + logb(y)
- Quotient Property: logb(x / y) = logb(x) – logb(y)
- Power Property: logb(xn) = n * logb(x)
Logarithmic functions also have several key applications in different fields. In finance, for example, logarithms are used to calculate compound interest and model investment growth. In biology, they can help describe population growth or the decay of substances. In physics, logarithmic functions appear in formulas that model sound intensity, earthquake magnitude, and radioactive decay.
Graphing Logarithmic Functions
Graphing logarithmic functions involves understanding their basic shape and transformations. A typical logarithmic function with base greater than 1 will have a curve that increases slowly at first and then steepens as x increases. The function has a vertical asymptote at x = 0 and passes through the point (1,0), since any logarithm of 1 is always 0.
In the case of base b where b > 1, the graph will show increasing values from left to right, whereas for b , the graph will show decreasing values.
Solving Logarithmic Equations
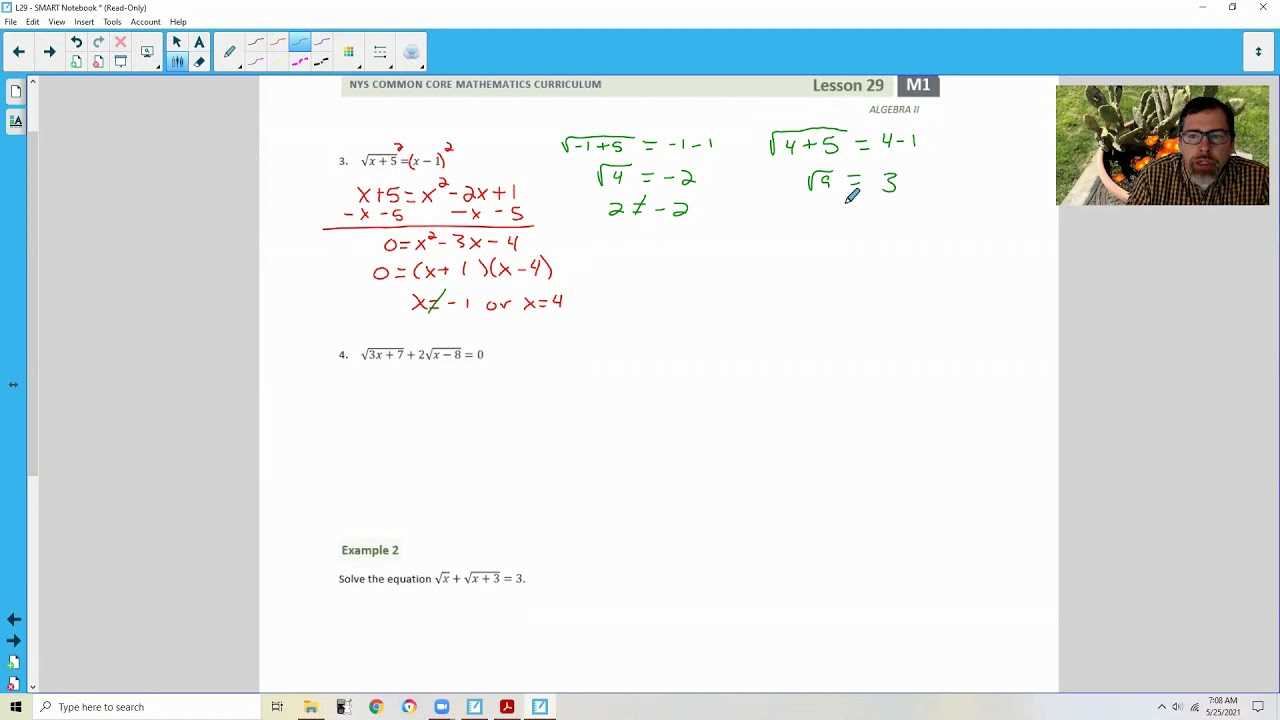
Solving logarithmic equations often involves rewriting the equation in its exponential form. For example, to solve logb(x) = y, we can rewrite it as by = x. By understanding this fundamental property, we can solve logarithmic equations by applying the inverse relationship between logarithms and exponentials.
| Logarithmic Form | Exponential Form |
|---|---|
| logb(x) = y | by = x |
| log10(x) = y | 10y = x |
Real-World Applications of Algebra 2
Mathematical concepts learned in advanced courses are not just theoretical; they play a crucial role in solving real-world problems across various industries. From economics to engineering, understanding complex functions, equations, and models is key to making informed decisions and creating innovative solutions. This section will explore how the skills gained in advanced math can be applied to everyday challenges.
One of the main areas where these skills are used is in the field of finance. For instance, solving equations related to growth models helps determine the future value of investments, while understanding logarithms can assist in calculating compound interest. These mathematical tools are used by financial analysts and investors to make predictions and assess risks.
Another important application is in engineering, where mathematical models are used to design structures, predict behaviors, and optimize systems. Engineers use advanced functions to analyze stress on materials, model fluid dynamics, and improve the efficiency of machines. Without these tools, many of the technologies and infrastructures we rely on today would not be possible.
Applications in Science and Medicine
In science, advanced mathematics is used to model everything from population growth to chemical reactions. Logarithmic and exponential functions help scientists predict how populations will change over time or how the concentration of substances will change in a reaction. Medical researchers use similar models to track the spread of diseases and determine the most effective treatment plans.
Applications in Computer Science
Computer science also relies heavily on advanced mathematical techniques. Algorithms used in data analysis, cryptography, and artificial intelligence are built on mathematical models. For example, sorting and searching algorithms use functions to optimize the speed and efficiency of computer programs. Mathematical knowledge is also essential in cybersecurity, where encryption methods ensure data privacy and protection.
- Finance: Investment growth, risk assessment, compound interest.
- Engineering: Structural design, fluid dynamics, system optimization.
- Science: Population modeling, chemical reactions, disease spread analysis.
- Computer Science: Algorithms, encryption, data analysis.
Ultimately, the applications of advanced mathematics are vast and varied, influencing nearly every aspect of our modern world. Whether improving technology, advancing scientific research, or optimizing financial strategies, these mathematical concepts help drive progress in countless fields.
Practice Problems for Module 1
To fully grasp the concepts learned, it’s essential to put theory into practice. By solving problems, students can strengthen their understanding and improve their problem-solving skills. This section presents a variety of exercises designed to challenge your knowledge and help you apply what you’ve learned. Whether you’re reviewing key ideas or tackling new ones, these problems will test your ability to work through different mathematical scenarios.
Start by solving simple equations and gradually move on to more complex problems. Each problem provides an opportunity to refine your skills, making sure you are prepared for more advanced topics. Practice is the key to mastering mathematical techniques, and this section aims to build your confidence through repetition and problem-solving.
Example Problems
- Problem 1: Solve for x: 3x + 5 = 20.
- Problem 2: Simplify: (2x + 3)(x – 1).
- Problem 3: Solve the inequality: 4x – 7 > 9.
- Problem 4: Factor: x² + 5x + 6.
- Problem 5: Solve for x: x² – 4x = 0.
Working through these examples will help solidify your understanding of important mathematical methods. Once you have completed them, try more challenging variations to push your problem-solving abilities further. With enough practice, these concepts will become second nature, preparing you for future coursework and real-life applications.
Common Mistakes and How to Avoid Them
When working through mathematical problems, it’s easy to make small errors that can lead to incorrect results. These mistakes often arise from misunderstandings of key concepts or simple calculation errors. By recognizing common pitfalls and learning how to avoid them, you can improve both your accuracy and confidence in solving problems.
It’s important to develop a methodical approach to solving equations and performing operations. Taking the time to review each step can help you catch errors before they impact your final answer. This section highlights the most frequent mistakes and provides tips on how to avoid them, ensuring that you approach each problem with a clear and focused mindset.
Common Mistakes
- Incorrect Distribution: Forgetting to apply the distributive property correctly can lead to errors. Always double-check your work when multiplying expressions.
- Sign Errors: One of the most common mistakes is flipping signs during addition or subtraction. Pay close attention to negative and positive signs.
- Misunderstanding Exponents: Remember that when raising a number to a power, both the base and the exponent must be correctly handled. Confusing rules for exponents can lead to inaccurate results.
- Forgetting to Check Solutions: Once you solve an equation, it’s essential to substitute your solution back into the original equation to verify your result.
- Overlooking Restrictions: Sometimes, certain values may make an equation undefined (e.g., dividing by zero). Always check for restrictions before finalizing your solution.
How to Avoid These Mistakes
- Review Key Concepts: Take the time to revisit fundamental rules and properties. A solid understanding of the basics will help you avoid common pitfalls.
- Work Step-by-Step: Break down complex problems into smaller, manageable steps. This allows you to focus on each part of the solution, minimizing the risk of overlooking something important.
- Double-Check Your Work: After completing a problem, always go back and review your calculations. A quick review can help you spot mistakes before finalizing your answer.
- Use Tools for Practice: Utilize online calculators, practice problems, and worksheets to reinforce your skills and identify areas where you might be prone to making errors.
By keeping these tips in mind and practicing regularly, you can avoid the most common mistakes and improve your problem-solving abilities. The key to success is staying vigilant, reviewing your work, and continuing to refine your understanding of mathematical principles.