1988 AP Calculus AB Multiple Choice Answer Key

Preparing for advanced mathematical assessments requires a thorough understanding of both the questions and the reasoning behind each solution. By analyzing past tests, students can gain insight into the patterns and problem types that appear most frequently. This method not only helps with time management but also improves problem-solving skills by identifying areas of strength and weakness.

In this section, we will explore a comprehensive guide to tackling questions from previous exams, focusing on critical thinking and strategic approaches. By reviewing specific examples and breaking down solutions, learners can better prepare for future assessments. The goal is to enhance both understanding and performance through detailed explanations and targeted practice.

Throughout this guide, we will cover strategies for interpreting problems, recognizing common misconceptions, and mastering key concepts. Whether you’re revising or approaching the material for the first time, the following tips and solutions will help sharpen your skills and boost your confidence.

1988 AP Calculus AB Multiple Choice Answers

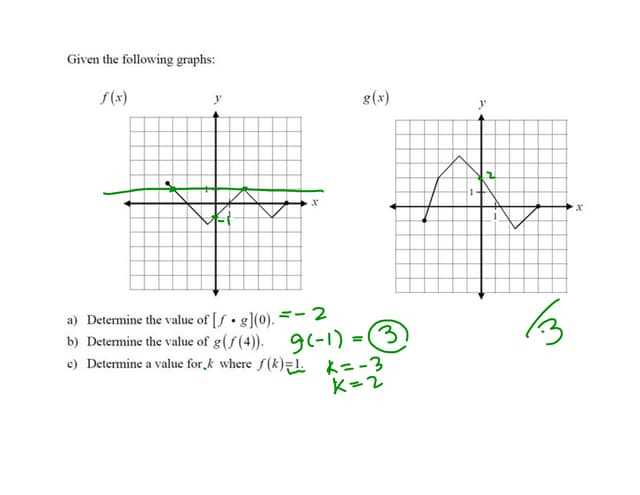
Examining the solutions from previous assessment papers offers valuable insights into how to approach and solve complex mathematical problems efficiently. These questions test a range of topics, from fundamental concepts to advanced techniques, and provide a clear picture of the key areas students need to focus on. Understanding the structure and logic behind the solutions can help enhance both performance and comprehension for future exams.

Key Concepts Tested

The questions in this set cover various mathematical concepts that are fundamental to succeeding in higher-level assessments. Some of the most important topics include:

• Limits and continuity
• Derivatives and their applications
• Integrals and integration techniques
• Sequences and series
• Functions and their properties

Approach to Problem Solving

When tackling questions from past tests, it’s essential to develop a strategy that allows for careful analysis and efficient problem-solving. Here are some effective methods:

1. Break down complex problems into smaller, more manageable steps.
2. Identify key variables and relationships in the problem statement.
3. Consider alternate methods of solution, such as using symmetry or simplifying expressions.
4. Double-check all calculations to avoid simple errors.
5. Review each answer option carefully to eliminate any unlikely choices.

By practicing with these past questions and applying these strategies, students can improve their chances of success in future assessments and gain a deeper understanding of the material. Regular review and targeted practice are key to mastering the subject and building confidence in solving complex problems.

Overview of AP Calculus AB Exam

The AP exam in advanced mathematics tests students’ understanding of essential mathematical principles and their ability to apply these concepts to solve complex problems. It is designed to evaluate both theoretical knowledge and practical skills, providing a measure of a student’s readiness for college-level coursework. The exam covers a wide range of topics, from functions and derivatives to integrals and applications of calculus, all within a structured testing format.

The assessment is divided into two main sections: one focuses on solving problems without a calculator, while the other allows the use of a calculator for more complex calculations. This structure challenges students to demonstrate their ability to think critically and apply mathematical techniques effectively in different contexts. By mastering the material and practicing problem-solving strategies, students can perform well and gain college credit for their efforts.

Key Topics Covered in 1988 Exam

The exam assesses a variety of mathematical concepts that are essential for understanding higher-level mathematics. These topics range from foundational principles to more complex theories, all designed to challenge students and test their ability to apply learned concepts in practical scenarios. By mastering these areas, students can better prepare for future academic endeavors in the field of mathematics.

Core Concepts in the Exam

Several key concepts are tested throughout the exam, each focusing on different aspects of mathematics. The most important areas include:

• Limits and their applications
• Derivatives and differentiation techniques
• Integration and related problems
• Applications of integrals in real-world problems
• Analyzing and understanding functions

Problem-Solving Strategies

Effective problem-solving is at the core of the exam, requiring students to apply their knowledge to both familiar and unfamiliar questions. Some of the strategies to approach these problems include:

• Carefully breaking down complex problems into simpler steps
• Identifying key relationships between variables
• Choosing appropriate methods for calculation
• Rechecking results to ensure accuracy

Focusing on these core areas and honing problem-solving skills will help students achieve success in the exam while reinforcing their understanding of key mathematical principles.

How to Use Answer Keys Effectively

Using solution guides from past assessments can be an invaluable tool for improving understanding and performance. By reviewing the explanations behind each solution, students can gain deeper insights into problem-solving techniques and learn from any mistakes they may have made. However, it is essential to approach these guides in a structured manner to maximize their educational value.

Step-by-Step Review

The most effective way to use a solution guide is to go through each problem methodically. Start by attempting to solve the problems on your own before consulting the key. This approach helps reinforce your problem-solving abilities and highlights areas where you need more practice. Once you’ve worked through the problem, compare your solution with the provided explanation.

• Identify discrepancies: If your solution differs from the key, carefully examine the steps to understand where your approach went wrong.
• Learn the method: Focus not just on the final result but on the techniques used to reach the answer. This will help you apply the same methods to similar questions.

Avoid Over-Reliance on Solution Keys

While solution guides are useful, it is important not to rely on them too heavily. Instead of memorizing the answers, use them to understand the logic behind each step. Strive to internalize the methods and apply them to new problems. This approach encourages independent problem-solving and strengthens your mathematical intuition.

Incorporating solution keys into your study routine with these strategies will lead to a more comprehensive understanding of the material and improve your overall exam performance.

Understanding Multiple Choice Format

When approaching exams with questions that offer several possible responses, it’s essential to understand the structure of such assessments. This format tests not only knowledge but also the ability to quickly analyze each option and select the correct one. Familiarity with the setup can help you improve your decision-making process and avoid common mistakes.

The key to excelling in this format lies in strategic thinking and careful review of each question. Often, these questions include distractors–incorrect options that are designed to test your understanding of the material. Recognizing patterns in these options can help you eliminate the wrong choices and focus on the most accurate answer.

Key Features of the Format

• Clarity of Instructions: Each question is typically accompanied by clear instructions on how to answer, allowing you to focus on the content itself.
• Answer Options: There are usually several possible responses, one of which is correct. Being familiar with the structure of these options can help you quickly identify the right one.
• Time Management: Because of the limited time, it’s important to read each question thoroughly, but also move efficiently through the test.

Strategies for Success

1. Eliminate Incorrect Choices: Start by crossing out answers that are clearly wrong. This narrows down your options and improves your chances of selecting the correct one.
2. Focus on Keywords: Pay attention to critical terms in the question, as they often reveal the best approach to solving the problem.
3. Double-Check Calculations: When the question involves mathematical problems, ensure that your calculations align with the given options.
4. Trust Your First Instinct: Often, your initial answer choice is the correct one. If you have a strong feeling about an answer, trust it unless you find clear evidence to the contrary.

By understanding the structure of these types of questions and employing effective strategies, you can navigate them more confidently and improve your exam performance.

Tips for Preparing for AP Calculus

Effective preparation for advanced mathematical exams requires a focused and organized approach. To achieve success, students should not only review key concepts but also develop problem-solving strategies and manage their study time efficiently. The right preparation techniques can make a significant difference in performance, helping you tackle even the most challenging questions with confidence.

Master Key Concepts and Techniques

Familiarity with the core topics is essential for doing well in any advanced mathematics exam. To prepare effectively:

• Review fundamental concepts such as limits, differentiation, and integration.
• Understand the applications of these concepts in real-world problems.
• Practice different methods of solving problems, including both analytical and numerical techniques.
• Work on mastering key formulas and theorems that often appear in exams.

Practice Problem Solving

Solving problems regularly is one of the most effective ways to prepare for an exam. Here are some tips:

• Work through past exam papers and practice problems to become familiar with the types of questions that might appear.
• Start by solving problems without the aid of a calculator to strengthen your manual calculation skills.
• Focus on understanding the logic behind each solution, not just memorizing steps.
• Time yourself to practice completing problems within the exam’s time limits.

By mastering key concepts and practicing regularly, you can improve both your confidence and performance on the exam.

Common Pitfalls in Multiple Choice

When faced with a set of possible answers, students often make mistakes that could easily be avoided with a little more attention. These common errors can stem from misreading questions, rushing through the options, or failing to carefully evaluate each answer. Understanding these pitfalls and learning how to avoid them can greatly improve exam performance.

Misinterpreting the Question

One of the most frequent mistakes students make is misunderstanding what the question is asking. To avoid this:

• Carefully read each question and make sure you understand the context before moving on to the options.
• Look for keywords that signal the main focus of the question (e.g., “increase,” “decrease,” “exact,” “approximate”).
• Pay attention to negative words such as “not” or “except,” which can change the entire meaning of the question.

Rushing Through Answer Options

Another common mistake is rushing through the possible answers without carefully considering each one. Here’s how to avoid this error:

• Take the time to review all options before selecting your answer, even if the correct one seems obvious at first glance.
• Eliminate obviously incorrect answers first, narrowing your choices to make a more informed decision.
• Double-check your calculations and reasoning before finalizing your answer, especially for complex problems.

By being aware of these pitfalls and practicing careful reading and thoughtful analysis, you can significantly improve your chances of selecting the correct option and perform better on exams.

How Scoring Works for AP Calculus AB

Understanding the scoring system of any exam is crucial for students as it helps them approach their study and test-taking strategies more effectively. The scoring for this particular exam combines multiple sections with different types of questions, each contributing to the final score. The total score is based on both accuracy and the application of concepts across a range of topics.

Score Breakdown

The exam is divided into two main sections: one focusing on multiple-choice questions and the other on free-response problems. Each section is weighted differently, and the scores from both are combined to calculate the final score. Below is a summary of how the scoring is distributed:

Section	Weight	Content
Multiple-Choice	50%	Various topics covered through a set of questions with pre-determined responses.
Free-Response	50%	Open-ended questions where students must show their work and reasoning.

Scoring Scale

After the exam is completed, both sections are scored, and the combined raw score is then converted into a final score. The final score is scaled to range from 1 to 5, where:

• 5: Extremely well qualified
• 4: Well qualified
• 3: Qualified
• 2: Possibly qualified
• 1: No recommendation

This system helps provide a comprehensive view of a student’s performance, accounting for both the correctness of the answers and the quality of problem-solving skills demonstrated throughout the exam.

1988 Exam Question Breakdown

The structure of any exam is designed to assess various levels of understanding and problem-solving ability. In this case, the questions are strategically organized to cover a broad range of topics, each with a focus on different skills and concepts. Understanding the breakdown of the questions can help you identify key areas to focus on during your preparation and improve your performance.

Topic Distribution

The questions in this exam span several key areas of mathematics, each designed to test specific skills. Here’s a breakdown of the major topics covered:

• Limits and Continuity: Questions often explore the fundamental principles of limits, continuity, and the behavior of functions at boundaries.
• Derivatives: A significant portion of the exam assesses knowledge and application of differentiation techniques, including rules and applications of derivatives.
• Integrals: Expect questions on both definite and indefinite integrals, as well as their applications in areas such as area under curves and accumulation functions.
• Applications of Derivatives and Integrals: Some questions require applying calculus concepts to real-world problems, such as optimization and rate of change.
• Fundamental Theorem of Calculus: This is a core concept tested through a range of problems, often linking the concept of integration to differentiation.

Question Types and Difficulty Levels

The questions vary in difficulty, with some focusing on basic conceptual knowledge, while others test more advanced problem-solving skills. Here’s how the questions are typically structured:

1. Basic Conceptual Questions: These questions are typically straightforward and test the understanding of core principles.
2. Intermediate Application Problems: These questions require you to apply your knowledge to solve more complex problems, often involving multiple steps.
3. Advanced Problem-Solving: These problems are designed to challenge your ability to synthesize various concepts and techniques, often requiring deeper analysis and critical thinking.

By understanding the distribution of topics and the varying levels of difficulty, you can approach each section with a clearer sense of what to expect and focus your study efforts on the areas that require the most attention.

Mathematical Concepts to Focus On

To excel in any advanced mathematical exam, it’s essential to have a strong grasp of the fundamental concepts that are frequently tested. These core ideas form the foundation for most problems you will encounter and will be crucial in both solving individual questions and applying mathematical reasoning. By focusing your study efforts on these key areas, you can improve both your speed and accuracy during the exam.

Some of the most important mathematical principles to concentrate on include:

• Limits and Continuity: Understanding the behavior of functions as they approach specific points is critical. Key concepts include determining limits, analyzing continuity, and identifying discontinuities.
• Differentiation: Mastering derivative rules, including product and quotient rules, chain rule, and implicit differentiation, is essential for solving many types of problems.
• Integration Techniques: Be proficient with both basic integration methods and more complex strategies, such as integration by parts, substitution, and partial fractions.
• Fundamental Theorem of Calculus: This theorem bridges the concepts of differentiation and integration, and understanding how to apply it is vital for solving many problems.
• Optimization and Related Rates: Many problems require applying differentiation to solve real-world problems involving rates of change, maximizing or minimizing quantities, and finding tangents to curves.
• Area and Volume: Familiarize yourself with concepts involving areas under curves, surface areas, and volumes of solids of revolution using integration techniques.

By reinforcing your understanding of these concepts and regularly practicing related problems, you’ll be better equipped to handle the challenges presented in this type of exam and apply your knowledge effectively to achieve a strong performance.

Interpreting Answer Explanations

Understanding the rationale behind the solutions to problems is just as important as solving the problems themselves. By examining the step-by-step explanations, you can uncover the underlying principles and strategies used to arrive at the correct answer. This not only helps reinforce your knowledge but also improves your problem-solving skills for future questions.

When interpreting answer explanations, focus on the following key aspects:

• Identify the Key Concepts: Look for the core mathematical principles being applied in each step. This could be a rule of differentiation, an integration technique, or a method for solving equations. Recognizing these concepts will help you understand how to approach similar problems in the future.
• Understand the Reasoning Process: Break down each explanation to understand why specific steps are taken. For example, why was a certain rule or formula used? Why was one approach chosen over another? Understanding the reasoning behind each step enhances your ability to make decisions during the exam.
• Examine Alternative Approaches: Some explanations may offer multiple methods for solving a problem. Comparing these methods can deepen your understanding and show you different ways of tackling similar questions.
• Learn from Mistakes: If an explanation reveals a mistake, carefully analyze what went wrong. Understanding common errors, such as misapplying a formula or making a calculation mistake, will help you avoid making the same mistakes in the future.

By carefully studying and interpreting answer explanations, you can transform mistakes into valuable learning opportunities and deepen your understanding of the material. This approach will ultimately enhance both your exam performance and your overall mathematical comprehension.

Analyzing Patterns in 1988 Questions

Recognizing recurring themes and trends in test questions can provide valuable insights into how to approach the exam. By analyzing past problems, you can identify which concepts are frequently tested, and adapt your study strategies accordingly. This pattern recognition allows you to predict the types of problems that may appear, and prepare more effectively for the exam.

To effectively analyze patterns, consider the following approaches:

Focus on Repeated Concepts

Review the types of mathematical concepts that appear multiple times across different problems. Whether it’s certain equations, methods of integration, or specific theorems, understanding these frequently tested areas will give you a focused set of topics to prioritize during your preparation.

Examine the Problem Structure

In addition to the content, look at how the problems are structured. Are there specific formats that reappear? For instance, do questions often involve applying the same set of steps, or solving within certain contexts? Recognizing these patterns in the structure will help you understand what is expected in terms of problem-solving approach.

By consistently reviewing past questions and identifying patterns, you can gain a clearer picture of the types of challenges you are likely to encounter. This targeted preparation strategy allows you to sharpen your skills and improve your performance in future assessments.

How to Tackle Difficult Questions

When faced with challenging problems, it’s important to remain calm and methodical in your approach. Difficult questions often test your understanding of complex concepts and your ability to think critically. By breaking down the problem into manageable parts and applying a logical problem-solving strategy, you can increase your chances of success.

Here are some strategies to effectively handle tough questions:

• Stay Calm and Focused: First, take a deep breath and don’t rush. Panicking can cloud your judgment and hinder your ability to think clearly. Remind yourself that every problem has a solution, and it’s simply a matter of finding the right approach.
• Break the Problem Down: Start by dissecting the question into smaller, more manageable components. Identify the given information, what is being asked, and any constraints or conditions that apply. This will help you focus on the key elements necessary to solve the problem.
• Eliminate Obvious Mistakes: Review the problem carefully and rule out any obvious errors or misinterpretations. Sometimes, questions are designed to mislead, so being aware of this can help you avoid common traps.
• Use Logical Reasoning: Even if a problem seems complicated, try to approach it logically. Apply known methods or principles step by step and check if the results make sense as you go along. If you’re stuck, think about similar problems you’ve solved before and how those methods might apply here.
• Don’t Get Stuck on One Question: If a question is taking too long to solve, it’s okay to move on and come back to it later. Spending too much time on one problem can jeopardize your ability to complete the rest of the exam. Time management is key.

With practice and patience, tackling tough questions becomes easier. By staying organized, calm, and persistent, you will develop the confidence needed to approach even the most difficult problems with ease.

Importance of Practice for Success

Consistent practice is the cornerstone of mastering any subject, especially when preparing for complex exams. The more you engage with problems and concepts, the better you become at recognizing patterns, applying techniques, and solving problems efficiently. Through regular practice, you solidify your understanding and build the skills necessary for success on challenging assessments.

Developing Problem-Solving Skills

Repetition allows you to refine your problem-solving strategies. By encountering a variety of questions, you become adept at choosing the most appropriate methods for different types of problems. This flexibility is crucial, as exams often present questions in ways you might not expect. The more problems you solve, the faster and more accurately you can approach new challenges.

Boosting Confidence and Reducing Stress

As you practice, your confidence grows. Familiarity with the material reduces anxiety and helps you stay calm during the exam. Knowing that you have tackled similar problems before allows you to approach even the toughest questions with a sense of preparedness, giving you a clear edge on test day.

Ultimately, practice is the key to transforming theoretical knowledge into practical skill. By continuously challenging yourself with a wide range of problems, you lay the foundation for long-term success and mastery in any subject area.

Reviewing the 1988 AP Solutions

After taking an exam, reviewing the solutions to each question is essential for deepening your understanding of the material. It’s not just about knowing the correct response, but also understanding the reasoning behind it. Going through the solutions step-by-step helps identify areas where you may have made errors and learn how to approach similar problems more effectively in the future.

Benefits of Solution Review

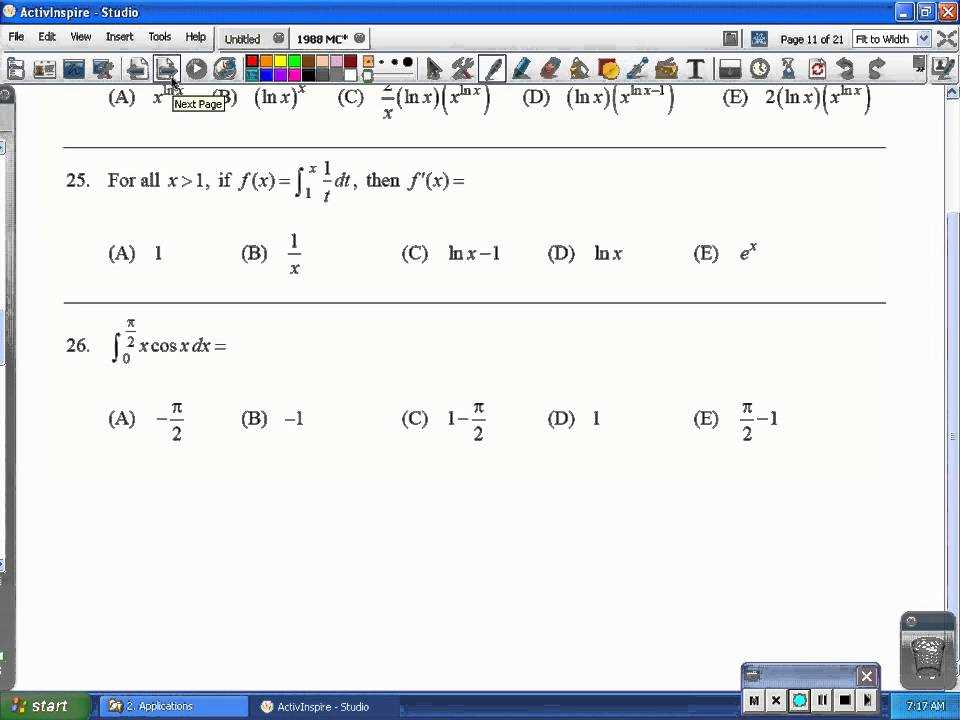
By analyzing the provided solutions, you can improve your problem-solving techniques. You’ll learn how to apply various strategies more efficiently and spot common pitfalls. Furthermore, reviewing solutions allows you to gain insight into the thought process of the exam designers, which can be invaluable for future test preparation.

Key Aspects to Focus On

When reviewing the solutions, pay attention to the following:

• Conceptual understanding: Make sure you grasp the underlying principles behind the solution, not just the final result.
• Methodology: Focus on the methods used to solve the problem and consider whether there are alternative approaches.
• Common errors: Identify any mistakes you made and understand why they occurred.

Example Solution Breakdown

Below is a breakdown of a typical solution for a problem from the exam:

Step	Description
Step 1	Read the question carefully to identify what is being asked.
Step 2	Use the relevant formula or theorem to begin solving the problem.
Step 3	Perform calculations or logical steps, checking work along the way.
Step 4	Review the final answer to ensure it makes sense in the context of the problem.

By carefully going over each solution, you gain more than just the right answers–you enhance your ability to approach future questions with confidence and precision.

How to Avoid Common Mistakes

In any examination, it’s easy to fall into common traps that can lead to mistakes, even when you know the material. The key to minimizing errors lies in preparation, attention to detail, and recognizing patterns in the types of problems you may encounter. By understanding the most frequent pitfalls, you can develop strategies to avoid them and improve your performance.

Common Errors to Watch For

Being aware of typical mistakes can help you prevent them in the first place. Here are some of the most frequent errors that test-takers make:

• Misreading the question: Always take the time to carefully read each question. Skimming can lead to missing key details.
• Overlooking units: Many problems involve specific units (e.g., time, distance, etc.), and failing to convert or interpret them correctly can lead to incorrect answers.
• Rushing through the problem: Speeding through a question increases the likelihood of making simple arithmetic mistakes or missing important steps in the solution.
• Not showing work: While some problems may be solvable mentally, writing down each step helps ensure accuracy and helps identify errors when reviewing solutions.
• Forgetting to check answers: After solving a problem, always take a few extra moments to review your work. Sometimes a quick recheck can reveal simple errors.

Strategies for Avoiding Mistakes

Here are a few strategies that can help you avoid common errors during your exam:

• Practice time management: Allocate enough time for each question, and don’t rush. If you get stuck, move on and come back to the question later.
• Break down problems: Take each question step-by-step to avoid overlooking key details or rushing through the solution.
• Use elimination techniques: If you are unsure about an answer, try to eliminate the obviously incorrect options to increase your chances of choosing the correct one.
• Double-check your calculations: Whether using a calculator or solving by hand, always check your arithmetic twice to catch any simple errors.

By being mindful of these common mistakes and employing these strategies, you can improve your chances of success and approach each problem with more confidence and precision.

Using Past Exams for Future Prep

One of the most effective ways to prepare for any exam is to review previous test papers. By analyzing past exams, you can familiarize yourself with the format, the types of questions commonly asked, and the level of difficulty you can expect. This practice not only helps build confidence but also allows you to identify areas where you may need further improvement. Using past exams as a study tool enables you to gain insight into both the content and the testing strategy, making your preparation more efficient.

Benefits of Practicing with Past Tests

Practicing with previous exams provides several advantages that can enhance your readiness:

• Familiarity with exam structure: By working through past tests, you get accustomed to the format, helping reduce anxiety on exam day.
• Understanding question patterns: Many exams reuse certain types of questions or concepts, so reviewing old tests can help you recognize these patterns.
• Improved time management: Practicing under timed conditions can help you develop the ability to manage your time effectively during the actual exam.
• Better identification of weaknesses: Regular review of old exams allows you to pinpoint topics or concepts that require more focus in your study sessions.

How to Use Past Exams Effectively

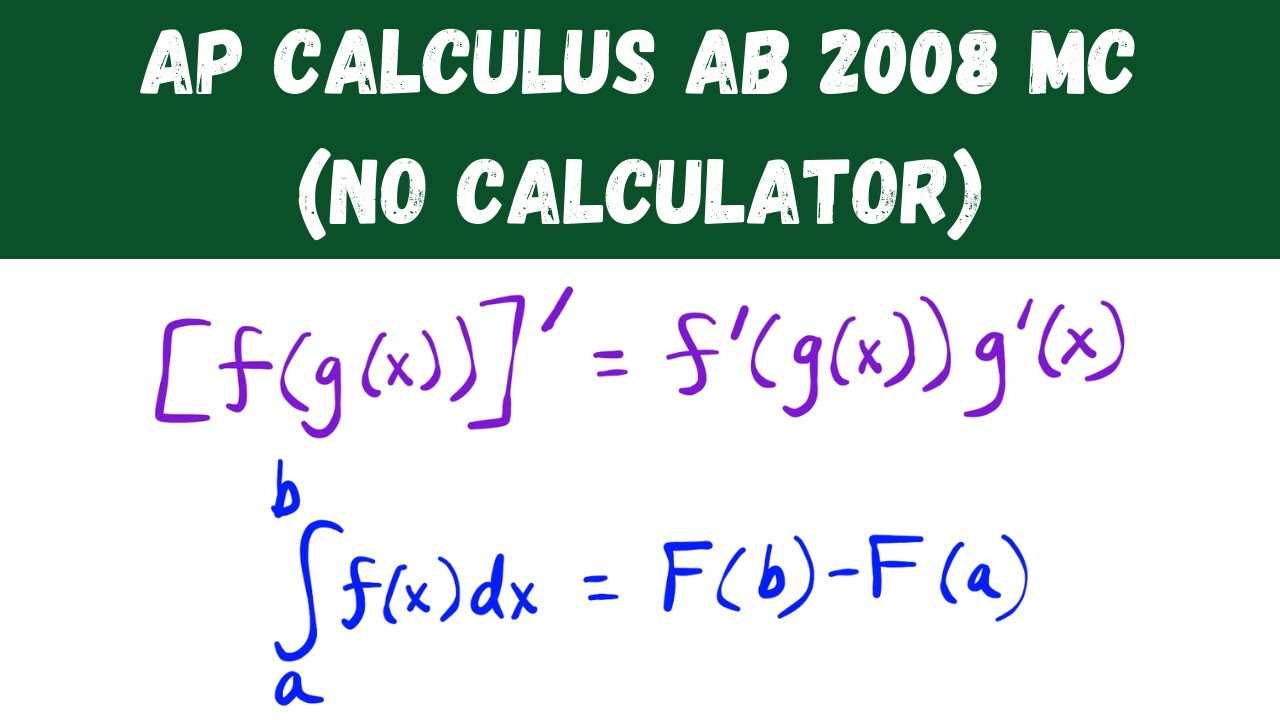
To get the most out of past tests, follow these strategies:

• Simulate exam conditions: Set aside dedicated time and simulate exam conditions as closely as possible. This means no interruptions, no notes, and strictly adhering to time limits.
• Analyze your mistakes: After completing each practice exam, carefully review any incorrect answers to understand your mistakes and learn from them.
• Focus on weak areas: Use the mistakes from past exams as a guide to identify your weaker areas and focus your study efforts on those topics.
• Use them as a review tool: Instead of simply attempting the questions, use the past exams to revise and reinforce key concepts and strategies you’ve already learned.

By incorporating past exams into your study plan, you can approach the exam with a more strategic mindset, reducing uncertainty and maximizing your chances for success.