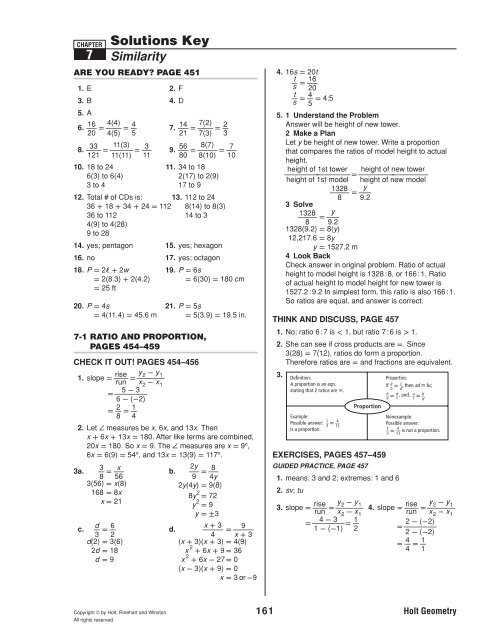
Lesson 14 Multistep Ratio Problems Answers
In this section, we dive into the intricacies of solving advanced mathematical questions that require multiple steps to reach a solution. These types of exercises are designed to challenge your understanding of core mathematical concepts and help you develop problem-solving strategies that can be applied across various situations.
Through clear explanations and detailed examples, we will explore how to break down complex calculations into manageable parts. You’ll learn how to approach each question systematically, ensuring accuracy and efficiency in your solutions. By the end of this section, you will have the tools needed to confidently tackle even the most challenging exercises.
Solving Advanced Arithmetic Challenges

This section focuses on tackling complex mathematical exercises that require several steps to reach the correct conclusion. These tasks often involve a combination of operations and require careful analysis to ensure all steps are performed correctly. Mastering this approach is key to improving your mathematical skills and enhancing your problem-solving ability.
In the following examples, we will guide you through each stage of the calculation process, demonstrating how to break down intricate questions into smaller, more manageable parts. With each exercise, you will gain a clearer understanding of how to approach such tasks efficiently and accurately.
By practicing these techniques, you will develop a strong foundation in handling advanced calculations and gain confidence in solving similar questions in future challenges.
Understanding Complex Mathematical Exercises
Complex arithmetic questions often require multiple calculations and careful reasoning. These tasks test your ability to analyze different elements and apply appropriate mathematical operations to find the correct solution. By mastering this approach, you’ll gain the skills needed to solve more challenging exercises effectively.
Breaking Down Each Step
To solve these types of exercises, it’s crucial to approach them step by step. Each stage of the calculation builds on the previous one, and understanding the logical sequence is essential for reaching the final result. Start by identifying the key components and then focus on how each part relates to the overall solution.
Common Techniques for Effective Solutions
When faced with complex calculations, using a methodical approach can make the process smoother. Techniques such as simplifying intermediate steps, identifying patterns, and double-checking your work will ensure accuracy. With practice, you’ll become more comfortable tackling intricate tasks and solving them with confidence.
Key Concepts in Ratio Calculations
To effectively solve complex calculations, it is essential to understand the foundational principles that guide each operation. These concepts provide the framework for approaching a variety of questions that involve relationships between quantities. By grasping these key ideas, you can tackle even the most intricate tasks with confidence and accuracy.
- Proportional Relationships: Understanding how different quantities relate to each other is the basis of solving many mathematical exercises. Recognizing proportionality helps in setting up equations that simplify complex tasks.
- Scaling: Often, problems involve adjusting one value in proportion to another. This skill is crucial in manipulating quantities to find unknown values and ensuring consistent results.
- Unit Conversion: In many cases, converting units of measurement is necessary to ensure all values align. This requires a solid understanding of different measurement systems and the ability to switch between them efficiently.
By internalizing these concepts, you can confidently approach any challenge that involves finding relationships between multiple quantities. These principles serve as the building blocks for more advanced problem-solving strategies.
How to Approach Complex Calculations
When faced with intricate mathematical tasks, breaking them down into smaller, manageable steps is key to reaching the correct solution. This approach helps organize your thoughts and ensures that no essential part of the process is overlooked. By carefully analyzing each stage, you can solve even the most challenging exercises with confidence.
Start with Understanding the Question: Begin by fully grasping what is being asked. Identify the known values and what needs to be found. This step sets the foundation for how you will approach the calculations ahead.
Break It Down into Steps: Rather than attempting to solve everything at once, break the exercise into smaller, logical steps. Focus on solving one part at a time, building on each completed stage.
Check Your Work: After completing each step, take a moment to review your calculations. Verifying your work helps catch any errors early and ensures that each step is on track before moving forward.
By following these guidelines, you will not only improve your accuracy but also gain the ability to approach difficult tasks with a clear and systematic mindset.
Breaking Down Complex Questions
When dealing with intricate exercises, the key to finding the correct solution lies in breaking down the task into smaller, simpler parts. This process allows you to approach the question logically, step by step, and reduces the chances of overlooking crucial details. By focusing on one aspect of the calculation at a time, you can prevent confusion and ensure accuracy throughout.
Start by identifying the most straightforward pieces of information. This often includes the quantities that are given and the relationships between them. Once you have a clear understanding of the basic components, the next step is to organize the data and structure the solution in a way that makes sense.
As you proceed, remember to handle one step at a time, solving intermediate equations before moving on to the next. This methodical approach not only ensures accuracy but also helps you track your progress as you work through the problem.
Step-by-Step Guide to Solving Calculations
Solving complex mathematical exercises requires a systematic approach that ensures clarity and accuracy. By breaking down the task into distinct, manageable stages, you can efficiently work through each step and reach the correct result. Here’s a step-by-step guide to help you solve these types of questions with confidence.
- Understand the Problem: Carefully read the question to determine what is being asked and identify the given values. Highlight the key pieces of information that are essential for solving the exercise.
- Set Up the Equation: Based on the information provided, write down the mathematical expression that reflects the relationships between the values. Ensure that the equation accurately represents the situation.
- Solve Step-by-Step: Focus on solving the equation one step at a time. Begin with the simplest calculations and gradually work towards the final solution. If necessary, simplify intermediate steps for clarity.
- Verify Your Work: After completing the calculation, check each step to ensure there are no errors. Cross-reference your work with the original question to confirm that your solution makes sense and meets the requirements.
By following these steps, you can confidently tackle even the most challenging exercises and develop a strong understanding of the processes involved.
Common Mistakes in Complex Calculations
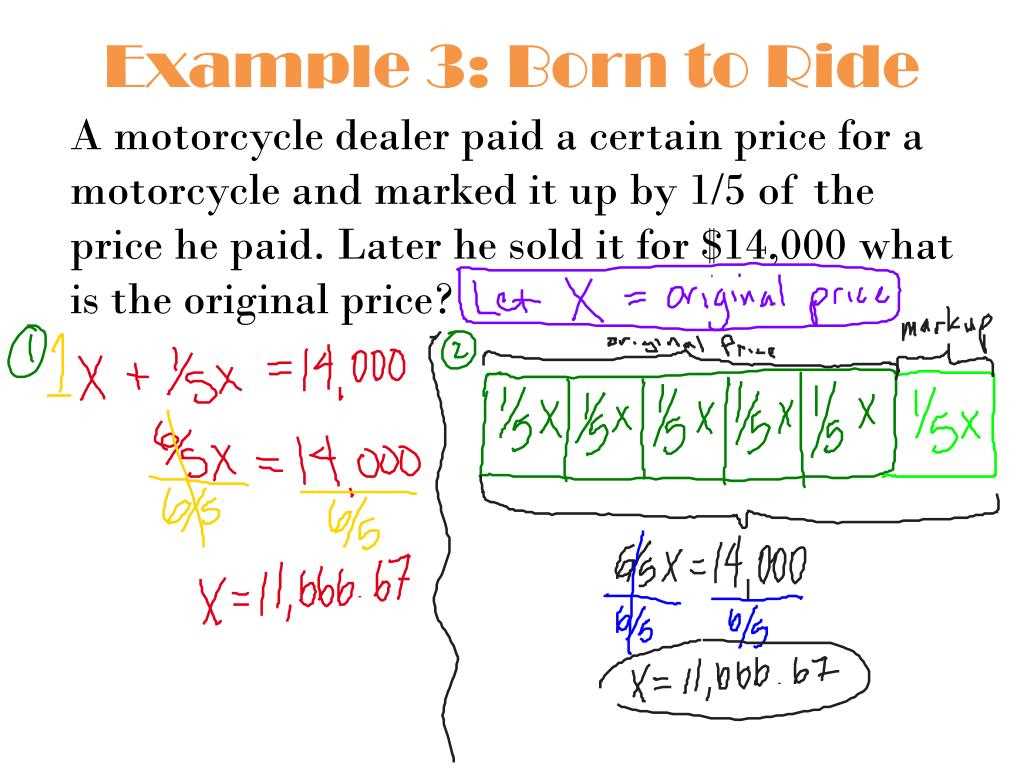
When solving intricate mathematical tasks, it’s easy to make mistakes, especially when multiple steps are involved. These errors often occur during the process of setting up equations, performing calculations, or interpreting results. Being aware of common mistakes can help you avoid them and improve the accuracy of your solutions.
Overlooking Key Details

One of the most frequent errors is missing crucial information. Whether it’s a missing unit, a key number, or an important relationship between values, overlooking any part of the question can lead to incorrect results. Always ensure you read the entire problem carefully and highlight the essential data before starting the calculations.
Skipping Intermediate Steps
Another common mistake is attempting to skip over intermediate steps, thinking they are unnecessary. However, skipping steps can cause confusion and lead to mistakes. It’s important to solve the problem gradually and verify each calculation before proceeding to the next stage.
Strategies for Efficient Problem Solving
When faced with challenging exercises that require multiple steps, using effective strategies can significantly improve the speed and accuracy of your solutions. The key is to approach the task systematically, break it down into smaller parts, and stay organized throughout the process. By adopting a structured method, you can reduce errors and make the problem-solving process more efficient.
Organize Your Work
One of the most important strategies is to keep your work well-organized. Writing out each step clearly, using symbols or variables where necessary, and keeping calculations neat can help you stay focused and avoid making mistakes. Here’s a simple table format you can use to keep track of intermediate steps:
| Step | Action | Result |
|---|---|---|
| 1 | Identify given values and relationships | Record key information clearly |
| 2 | Set up the equation | Write the correct expression |
| 3 | Perform calculations | Break into smaller tasks |
| 4 | Verify the result | Double-check for accuracy |
Use Simplification Techniques
Simplifying each part of the task as much as possible can reduce complexity and help you avoid getting stuck. Look for opportunities to simplify fractions, reduce terms, or factor expressions before proceeding with the next steps. This approach can help you reach a solution more quickly and with fewer chances for error.
Using Proportions in Complex Calculations

Proportions are a powerful tool for solving tasks that involve relationships between different quantities. By setting up a proportion, you can express the relationship between two sets of values and use it to find missing information. This method is especially helpful when dealing with questions that require comparing or scaling quantities in multiple stages.
Understanding Proportional Relationships: The foundation of using proportions lies in understanding how quantities are related. Once you identify the values that correspond to each other, setting up a proportion allows you to establish an equation that can be solved systematically.
Solving with Proportions: To solve using proportions, follow these basic steps:
- Identify the known values: Look for pairs of quantities that are related.
- Set up the proportion: Write the relationship between the known values and the unknown quantities in the form of a fraction.
- Solve the proportion: Cross-multiply if necessary and solve for the unknown variable.
By using proportions, you can simplify many complex tasks and find missing values by comparing known quantities efficiently. This approach is a fundamental strategy for solving a variety of mathematical exercises with clarity and precision.
Understanding Unit Rates in Relationships
Unit rates are a crucial concept when working with comparisons of different quantities. They allow you to express one quantity in terms of another, which helps to simplify the understanding of relationships between variables. A unit rate tells you how much of one thing there is for a specific amount of another thing, often making it easier to interpret data and make comparisons.
For example, if you know how much time it takes to travel a certain distance, you can express the relationship as a unit rate, such as “miles per hour.” This unit rate gives you a clear understanding of the speed, making it easier to compare with other travel scenarios or calculate the time needed for different distances.
To calculate unit rates, divide one quantity by another. In cases where the comparison involves multiple items or different units, it’s important to standardize the units so that the relationship is expressed in a consistent and comparable manner.
Applying Ratios to Real-Life Scenarios
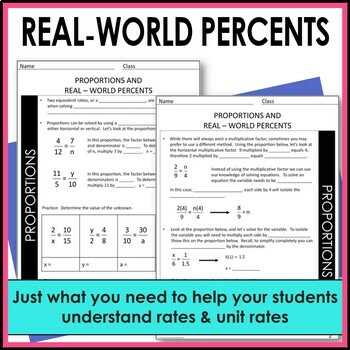
The ability to use mathematical relationships to solve real-world issues is a valuable skill. Many everyday situations involve comparing quantities, and understanding these relationships can help make informed decisions. By applying mathematical concepts, such as understanding proportions, we can solve practical problems more effectively.
For instance, when cooking, you often need to adjust ingredient amounts based on the number of servings. This is a practical example of applying proportional relationships. Similarly, when shopping, comparing prices per unit allows you to determine the best deal. Here are some examples of how ratios are used in daily life:
- Cooking: Adjusting ingredient quantities based on serving size.
- Travel: Comparing fuel efficiency to calculate fuel consumption over a given distance.
- Shopping: Calculating price per unit (e.g., cost per ounce or liter) to determine the best value.
- Finance: Comparing interest rates for savings or loans to make informed decisions.
By applying these concepts, we can make more accurate calculations, save time, and make better choices in various aspects of our daily lives. Understanding these relationships not only helps in problem-solving but also sharpens critical thinking and analytical skills.
How to Verify Your Solutions

Verifying the correctness of your solutions is an essential step in ensuring that the calculations you have made are accurate. It helps to confirm that all steps were followed correctly and that the final result is consistent with the problem requirements. This process provides confidence that you have arrived at the right conclusion, reducing the likelihood of errors.
Here are some effective ways to verify your calculations:
- Check the initial setup: Review the values and relationships you’ve used to ensure they correctly reflect the given information.
- Reverse calculations: In some cases, you can reverse the process to check if the result holds true when applied in the opposite direction.
- Use estimation: Estimate what the result should be. If your final answer is significantly different from your estimate, recheck your steps.
- Cross-check with a different method: If possible, use an alternative approach to solve the same problem and see if you get the same result.
- Check for consistency: Ensure that the units of measurement are consistent throughout the process and that the answer makes logical sense in the context of the question.
By applying these techniques, you can ensure that your solutions are not only accurate but also reliable. This careful checking process is a crucial habit for anyone working with mathematical relationships or complex calculations.
Examples of Multistep Ratio Problems
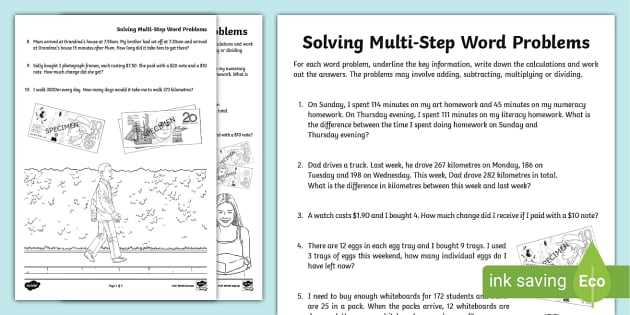
When faced with real-life situations that require comparing quantities, multiple steps are often involved in reaching a solution. These tasks require careful analysis and the ability to break down the problem into manageable parts. Below are some examples that illustrate how such challenges can be approached and solved using logical steps and mathematical principles.
Let’s consider a scenario involving a recipe that needs to be adjusted for different quantities. In this case, we need to scale the ingredients accordingly and follow the necessary steps to find the required amounts.
| Step | Description |
|---|---|
| Step 1 | Identify the original recipe’s proportions for each ingredient. |
| Step 2 | Determine how many servings you need, compared to the original recipe. |
| Step 3 | Multiply each ingredient amount by the factor that corresponds to the new number of servings. |
| Step 4 | Check the adjusted amounts to ensure they match the proportions of the original recipe. |
This approach can be applied to many other situations, such as adjusting speed, distance, or currency conversions. By breaking down each problem into smaller steps and applying consistent logic, it becomes easier to reach an accurate solution.
Calculating with Equivalent Ratios
When working with relationships between quantities, it’s often necessary to scale or adjust the proportions of different items while maintaining consistency between them. Equivalent ratios help in such situations, as they allow us to express different values that represent the same proportional relationship. Understanding how to calculate using equivalent ratios is essential in many fields, from cooking to construction and beyond.
To calculate with equivalent proportions, one method is to multiply or divide both parts of the ratio by the same number, thereby keeping the proportionality intact. This principle is used to adjust the quantities without altering the fundamental relationship between them.
Here’s an example of how to apply this principle:
| Original Proportion | Equivalent Proportion |
|---|---|
| 2:5 | 4:10 |
| 3:7 | 6:14 |
In this case, we multiplied both parts of the original ratio by 2 to generate equivalent proportions. The ratio remains the same even though the individual quantities have increased.
This method can be applied in various contexts, such as scaling recipes, adjusting quantities for different measurements, or converting units while maintaining consistent relationships. Mastering the process of calculating with equivalent ratios ensures that you can maintain accuracy even when working with large sets of data or varying measurements.
Advanced Techniques for Ratio Problems
As you delve deeper into solving complex relationships between quantities, it becomes essential to employ advanced strategies that simplify calculations and ensure greater accuracy. These techniques involve using algebraic methods, cross-multiplication, and understanding how to handle different types of conversions and unit changes in proportional situations.
Using Algebraic Methods
Algebra is an extremely useful tool when it comes to solving for unknowns in proportional relationships. By setting up equations, you can isolate variables and solve for the missing parts in a proportion. This method becomes particularly valuable when dealing with more complicated scenarios where a direct calculation isn’t possible.
For example, consider the following setup where you need to find the unknown quantity:
| Known Relationship | Equation |
|---|---|
| 4:5 = x:20 | x = (4 * 20) / 5 |
Solving this equation gives us the value of x, which is 16. Using algebraic methods, we can solve for unknown quantities even when they’re part of a more complex equation.
Cross-Multiplication for Precision
Cross-multiplication is a key technique for solving proportional relationships quickly. This method involves multiplying the terms diagonally across an equation to eliminate fractions and simplify the calculation. Cross-multiplication is especially effective when working with ratios involving fractions.
Consider this example:
| Proportion | Cross-Multiplication |
|---|---|
| 3/5 = x/10 | 3 * 10 = 5 * x |
By solving for x, we find that x = 6. This technique not only speeds up the process but also ensures greater accuracy when working with ratios that contain fractions or decimals.
In summary, advanced techniques like algebraic manipulation and cross-multiplication offer powerful ways to solve complex proportional relationships efficiently. These methods allow for more flexibility and precision, making them invaluable tools in higher-level ratio calculations.
Practical Tips for Multistep Problem Solving
Solving complex tasks involving multiple steps can often seem overwhelming, but with the right approach, you can break down the process into manageable pieces. By applying a few simple strategies, you can simplify each phase of the calculation and reach a solution efficiently. Below are some tips that can help you approach these types of tasks with confidence and accuracy.
Organize Your Approach
One of the first things to remember is that organization is key. Before you start, make sure to outline the steps and understand the structure of the task. This helps you avoid skipping over any important information and ensures that you are working logically. Consider the following tips:
- Read the entire task carefully to identify the key elements.
- Write down the given values and what needs to be calculated.
- Break down the task into smaller, simpler steps to avoid confusion.
Work Step by Step

Another important tip is to approach each phase methodically, focusing on one part at a time. Trying to solve the entire task in one go can lead to mistakes and confusion. By working through each step, you ensure that you are on track and that each calculation is accurate.
- Start with the first known value or equation.
- Use the appropriate mathematical operation (addition, subtraction, multiplication, or division) to simplify the next step.
- Once you have completed one step, move on to the next one, ensuring that the previous step’s result is correct.
- Double-check each step to avoid small errors that can accumulate over time.
These tips will not only help you handle complex tasks more effectively but also build confidence as you progress. By staying organized and taking it one step at a time, you’ll be able to navigate through any challenging scenario.