Physics Chapter 10 Answers Explained

The topics covered in this section are crucial for building a deeper understanding of core principles that form the foundation of advanced studies in science. With a focus on practical problem-solving techniques, this part provides essential tools to tackle challenging concepts effectively. Whether you’re studying for exams or aiming to enhance your grasp of theoretical material, mastering these areas is key to success.
Key ideas presented here highlight the importance of both conceptual clarity and mathematical precision. By reviewing these topics, you’ll be better equipped to approach various types of questions with confidence. The step-by-step breakdowns and practical examples provided will help solidify your understanding and prepare you for any test scenarios.
Learning how to apply these principles in real-world situations not only improves academic performance but also encourages critical thinking. Through thorough practice and careful analysis, you’ll gain the skills necessary to excel in this area and apply your knowledge to solve complex problems.
Chapter 10 Solutions and Insights
This section focuses on providing clear explanations and detailed steps to tackle the challenges presented in the current topic. By breaking down each problem into smaller, manageable parts, it allows for a deeper understanding of the underlying principles and methods. Whether solving for variables, applying formulas, or interpreting results, this approach ensures a thorough grasp of the material.
Key Steps to Follow
To successfully navigate through complex questions, follow these essential steps:
- Identify the given information and what needs to be determined.
- Choose the appropriate formulas or laws to apply.
- Break the problem into smaller, solvable parts.
- Perform calculations systematically and check the units.
- Review the solution to ensure all steps are logically followed.
Common Challenges and How to Overcome Them
Several issues may arise when working through these types of problems, but they can be easily managed with practice and proper techniques:
- Misunderstanding the problem’s context: Always ensure you fully comprehend what the question is asking before proceeding.
- Forgetting key formulas: Regularly review essential equations to stay familiar with the tools you’ll need.
- Skipping steps in the process: Avoid rushing; take your time to carefully follow each stage of the solution.
Understanding Key Concepts in Chapter 10
This section covers the foundational ideas that are essential for mastering the material in the current chapter. By focusing on the main principles, we can build a solid framework that will allow for easier problem-solving and deeper comprehension. Understanding these core concepts is crucial for tackling the more complex problems that follow.
Core Principles to Grasp
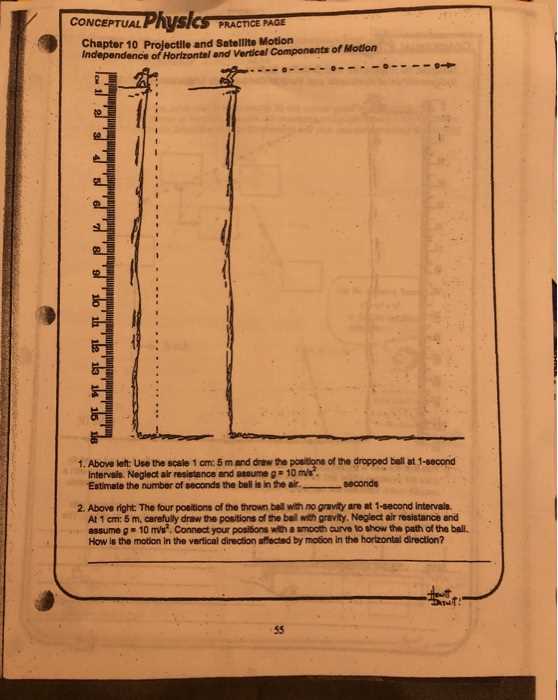
The following concepts are fundamental to success in this chapter:
- Basic Theorems: Ensure you have a clear understanding of the fundamental laws that govern the processes in this topic.
- Key Variables: Identifying and understanding the significance of the different variables used in calculations is critical.
- Relationship Between Concepts: Recognize how the concepts relate to one another and how one idea may influence another.
Common Misunderstandings
While these concepts are central, there are a few common areas where students may face difficulties:
- Confusing similar terms: Pay close attention to the differences between closely related terms and their specific applications.
- Overlooking assumptions: Be aware of any assumptions or approximations made during problem-solving and their impact on the outcome.
- Misapplying formulas: Review the correct formulas for each scenario to avoid incorrect results.
How to Approach Problems in Chapter 10
When faced with complex challenges in this chapter, it’s essential to approach each problem systematically. Breaking down the process into clear, manageable steps ensures a more efficient solution and a better understanding of the underlying concepts. With the right strategy, even the most difficult questions can become solvable.
Start by analyzing the question: Identify what is being asked and what information is provided. Often, the key to solving a problem lies in understanding exactly what is needed, so don’t rush through this step.
Next, choose the appropriate method: Determine which principles or equations are most relevant to the situation. Depending on the problem, you may need to apply one or more concepts to find the solution.
Organize your work: Write down all given data clearly, and define the variables. This helps in visualizing the problem and prevents confusion later on. Proper organization also helps keep track of units and conversions.
Finally, solve and verify: Carefully calculate the result and double-check your steps to avoid errors. Reviewing your solution will ensure that you’ve followed the correct method and arrived at a reasonable answer.
Step-by-Step Solutions to Chapter 10
This section provides a detailed, systematic approach to solving problems from Chapter 10. By breaking down each solution into clear steps, we can ensure a thorough understanding of the methods involved and how they apply to different types of questions. Following this process will help reinforce key concepts and improve problem-solving skills.
Solution 1: Example Problem Breakdown
Let’s work through an example problem step-by-step:
| Step | Action | Explanation |
|---|---|---|
| 1 | Identify known values | Write down all given information, such as measurements, constants, and variables. |
| 2 | Select relevant formula | Choose the equation that best fits the problem, based on the concepts involved. |
| 3 | Substitute values into the formula | Plug in the known values and solve for the unknown variable. |
| 4 | Perform calculations | Complete the necessary mathematical steps to find the solution. |
| 5 | Verify the result | Check if the answer makes sense within the context of the problem. |
Solution 2: Another Example
Now, let’s apply the same steps to another problem to see how they work in different contexts.
| Step | Action | Explanation |
|---|---|---|
| 1 | List all known quantities | Ensure all values are accounted for before starting the solution. |
| 2 | Choose the correct equation | Identify which formula will help solve the problem based on the situation. |
| 3 | Insert values into the formula | Substitute the known values into the equation to isolate the unknown. |
| 4 | Calculate and simplify | Perform any necessary calculations and simplify the result for clarity. |
| 5 | Double-check your solution | Verify that the outcome is logical and corresponds to the expected result. |
Common Mistakes to Avoid in Chapter 10
When tackling problems in this chapter, students often make certain errors that can hinder their understanding and lead to incorrect solutions. Recognizing and avoiding these mistakes is crucial for improving accuracy and efficiency. By addressing these common pitfalls, you can streamline your problem-solving approach and enhance your comprehension of key concepts.
One frequent mistake is neglecting to carefully read and understand the problem before starting calculations. Jumping to conclusions without fully analyzing the given information can lead to using the wrong approach. Additionally, misapplying formulas is another common issue. Each equation is specific to certain conditions, and using an incorrect one can result in errors in the final solution.
Another common mistake is ignoring units of measurement. Conversions are essential in many problems, and failing to check or correctly convert units can lead to significant inaccuracies. It’s also important not to skip intermediate steps during the solution process. Rushing through calculations without showing each step can result in overlooking small but critical details that affect the final result.
Finally, students often overlook the importance of verifying their final answers. It’s crucial to check the logic and consistency of the results to ensure they make sense in the context of the problem. Taking the time to review your work can prevent simple mistakes from impacting the outcome.
Practical Applications of Scientific Principles in Real Life
The concepts covered in this chapter are not just theoretical; they have numerous practical applications in our daily lives. Understanding how these principles work helps us make sense of the world around us and enables us to apply them to solve real-world problems. From technology to engineering, the principles explored here influence a wide range of fields.
Everyday Examples of Scientific Concepts
Here are a few areas where these concepts are applied regularly:
- Transportation: The mechanics of vehicles, from cars to airplanes, rely on key principles like motion, forces, and energy transfer.
- Medicine: Technologies such as X-rays, MRIs, and ultrasound use various scientific principles to help diagnose and treat medical conditions.
- Energy Production: The generation of electricity from fossil fuels, nuclear plants, and renewable sources such as wind and solar power depends on understanding energy transformations.
- Building and Construction: Engineers use the concepts of forces, stress, and materials science to design and construct safe and durable buildings and infrastructure.
Impact on Technology and Innovation
The application of these concepts is also at the heart of technological innovation. For instance:
- Electronics: Smartphones, computers, and other devices rely on the principles of electricity, magnetism, and materials science to function efficiently.
- Space Exploration: The study of forces, motion, and thermodynamics plays a crucial role in the development of spacecraft and missions to outer space.
- Environmental Science: Understanding energy, heat transfer, and fluid dynamics is key to addressing climate change and developing sustainable technologies.
Tips for Solving Complex Questions
Tackling challenging problems requires a methodical approach and careful attention to detail. By following a structured strategy, you can break down even the most complicated questions into simpler, manageable parts. These tips are designed to help you build confidence and improve your problem-solving skills.
Key Strategies for Success
- Understand the Problem: Read the question carefully and identify the given information and what you are required to find. Clarifying the objective is the first step to a successful solution.
- Draw a Diagram: Visualizing the problem through diagrams or sketches can make complex situations easier to understand. Label all important quantities clearly.
- List Known Variables: Write down all the known values and their units. This will keep the important data organized and prevent confusion during the solution process.
- Break Down the Problem: Split the question into smaller, more manageable sub-problems. Solving these individually will help piece together the overall solution.
Avoiding Common Pitfalls
- Check Units: Always verify that your units are consistent throughout the calculations. Incorrect units can lead to errors in the final result.
- Avoid Rushing: Take your time with each step. Rushing through can cause careless mistakes or lead to misapplying concepts.
- Double-Check Calculations: After solving, review your work to ensure each step follows logically and that no mistakes were made in your calculations.
Essential Formulas for Chapter 10
In this section, we will review the most important equations that are critical for solving the problems presented in this chapter. Mastering these formulas is key to understanding the concepts and efficiently finding solutions. By applying the right formulas, you can tackle various types of questions with confidence and precision.
Key Equations and Their Applications
The following table lists the essential formulas that you will need to understand and apply in this chapter. Each formula is accompanied by a brief description of its use:
| Formula | Description |
|---|---|
| F = ma | Newton’s second law of motion, used to calculate force (F) given mass (m) and acceleration (a). |
| v = u + at | Equation for velocity, where v is final velocity, u is initial velocity, a is acceleration, and t is time. |
| d = ut + ½ at² | Formula for displacement (d) in uniformly accelerated motion, where u is initial velocity, a is acceleration, and t is time. |
| W = Fd cos(θ) | Work formula, used to calculate work done (W) by a force (F) over a distance (d), where θ is the angle between the force and displacement direction. |
| KE = ½ mv² | Kinetic energy equation, used to calculate the energy (KE) of an object in motion, where m is mass and v is velocity. |
Important Considerations

When applying these formulas, always make sure to keep track of the units, as incorrect units can lead to errors. Additionally, it’s important to understand the context in which each formula applies–knowing when and how to use each equation is just as important as knowing its structure. Practice is key to becoming familiar with these essential equations and using them effectively in problem-solving.
Breaking Down Difficult Problems in Chapter 10
When faced with complex problems, it can often feel overwhelming. However, by systematically breaking them into smaller, more manageable parts, you can approach each step with clarity and precision. The key to solving difficult questions lies in analyzing the problem carefully and applying the appropriate methods in a structured way.
Here are the general steps you can follow to simplify even the most challenging problems:
| Step | Action |
|---|---|
| 1. Understand the Problem | Read the problem statement carefully to identify the known values, the unknowns, and what the question is asking. Highlight key details to avoid missing crucial information. |
| 2. Organize the Information | Write down the given values and variables. Draw diagrams or sketches to visualize the situation, making it easier to comprehend the relationships between different elements. |
| 3. Identify Relevant Equations | Based on the problem’s context, determine which formulas or principles apply. Make sure you understand the conditions under which each equation is valid. |
| 4. Solve Step by Step | Work through the problem incrementally. Start with simpler calculations or smaller sub-problems, then build towards the full solution. |
| 5. Double-Check Your Work | After completing your calculations, review each step to ensure accuracy. Verify that the solution makes sense in the context of the question. |
By following this process, you can avoid feeling overwhelmed and ensure that you address each part of the problem thoroughly. Taking a step-by-step approach allows you to stay organized and focused, leading to more accurate and reliable solutions.
Chapter 10 Practice Questions
Practicing problems is essential for reinforcing concepts and developing problem-solving skills. By working through a variety of questions, you can deepen your understanding and improve your ability to apply what you’ve learned. This section provides a series of practice questions designed to challenge your knowledge and help you prepare for more complex scenarios.
Types of Questions

Below are different types of questions you may encounter, each focusing on a specific aspect of the chapter. These questions are designed to test both theoretical knowledge and practical application.
- Conceptual Questions: These questions assess your understanding of core principles and theories. They often require you to explain or describe relationships between variables.
- Calculation-Based Problems: These questions require you to apply formulas and perform calculations. They test your ability to manipulate equations and solve for unknown values.
- Real-World Scenarios: These questions place theoretical knowledge in a practical context, asking you to analyze situations or make predictions based on what you have learned.
Sample Practice Questions
Try solving the following practice questions to test your knowledge:
- Question 1: An object with a mass of 5 kg is moving with an initial velocity of 10 m/s. Calculate its final velocity after 4 seconds if the acceleration is 2 m/s².
- Question 2: A force of 15 N is applied to an object, causing it to move a distance of 3 meters. Calculate the work done by the force.
- Question 3: A 10 kg object is dropped from a height of 20 meters. What is its velocity just before it hits the ground, assuming no air resistance?
These questions are designed to challenge your ability to apply formulas, understand physical concepts, and think critically about real-world applications. Take your time and review each solution thoroughly to gain the most benefit from the practice session.
How to Improve Your Skills in Chapter 10
Enhancing your problem-solving abilities in this subject requires a combination of practice, understanding core principles, and developing a systematic approach. The more you familiarize yourself with key concepts and equations, the easier it becomes to apply them effectively. By following certain strategies, you can improve your overall grasp of the material and excel in tackling difficult problems.
Strategies for Improvement
Here are several techniques you can use to strengthen your skills and achieve better results:
- Understand the Fundamentals: Before diving into complex problems, ensure you have a solid grasp of basic principles. Understanding the theory behind the equations and laws will make it easier to apply them correctly in various situations.
- Practice Regularly: The more problems you solve, the more familiar you become with different problem types and the methods used to solve them. Consistent practice is key to building confidence and efficiency.
- Break Down Complex Problems: If a problem seems overwhelming, break it down into smaller, more manageable parts. Address each part step by step, which will help you stay focused and organized.
- Use Visual Aids: Diagrams, graphs, and charts are incredibly useful tools for understanding complex scenarios. Sketching out a situation can help you visualize relationships and better understand the problem.
- Review Mistakes: After attempting a problem, always go back and analyze your errors. This process helps identify where you went wrong and ensures you don’t repeat the same mistakes in the future.
- Seek Additional Resources: If a concept or problem type is unclear, don’t hesitate to seek extra help. Use online tutorials, textbooks, or ask peers and instructors for clarification.
Focus on Real-World Applications
Linking theory to real-life situations makes learning more engaging and helps solidify your understanding. Look for examples in everyday life where the concepts you’re studying apply. This practical approach will not only make learning more interesting but also deepen your conceptual understanding.
By applying these strategies and staying committed to consistent practice, you’ll find that solving problems in this subject becomes more intuitive and manageable over time.
Concepts You Need to Master for Chapter 10
To excel in this chapter, it is crucial to develop a strong understanding of several key concepts. These foundational ideas will not only help you solve complex problems but will also enable you to apply your knowledge effectively in various contexts. Mastering these concepts ensures that you can approach problems systematically and with confidence.
Key Concepts to Focus On
Below are some of the most important concepts you should fully understand in order to succeed in this chapter:
- Force and Motion: The relationship between force, mass, and acceleration is central to understanding many problems. Mastering Newton’s laws will help you analyze various physical scenarios.
- Work and Energy: Grasping the concepts of work, energy, and power is crucial. Understanding the conservation of energy and the ability to calculate work done in different situations is essential for problem-solving.
- Momentum: The concept of momentum and its conservation in isolated systems is a critical area. Be sure to understand how momentum applies to both collisions and explosions.
- Gravitational Forces: The study of gravitational interactions, including the concepts of gravitational force and potential energy, is foundational for various applications in this chapter.
- Waves and Oscillations: Waves, wave properties, and harmonic motion are common themes in many problems. Mastering the behavior of waves and their interactions is essential for tackling related questions.
Application of These Concepts

Mastering the above concepts will not only help you solve textbook problems but will also allow you to connect theoretical knowledge with practical applications. From engineering to natural phenomena, the principles you’ve learned can be applied in a variety of real-world scenarios. Understanding these key ideas ensures that you are fully prepared for both exams and real-life problem-solving situations.
Reviewing Important Theories and Laws
To successfully tackle complex problems, it is essential to have a solid understanding of the core theories and laws that govern the topics in this chapter. These foundational principles not only provide the framework for solving problems but also help explain the underlying mechanisms behind physical phenomena. Reviewing these laws regularly ensures that you can apply them accurately and effectively in various scenarios.
Key theories and laws act as the building blocks of problem-solving. They offer a structured way of approaching challenges and understanding the behavior of objects in different conditions. Mastery of these concepts will make the process of solving problems more intuitive and less daunting.
Common Questions in Chapter 10
Throughout this chapter, certain questions often arise that challenge students’ understanding of key concepts. Addressing these questions can clarify common misconceptions and reinforce your grasp of the material. By reviewing these frequent queries, you can strengthen your problem-solving skills and prepare more effectively for assessments.
Frequently Asked Questions

Here are some of the most common questions encountered in this chapter:
- How do I apply the laws of motion to complex systems? Understanding the basic principles of force and acceleration is essential. Breaking down a system into simpler parts and analyzing their interactions is a good approach.
- What is the difference between potential and kinetic energy? Energy can be classified into these two types depending on the state of the object. Kinetic energy relates to motion, while potential energy is stored energy based on position.
- How can I determine the velocity of an object in free fall? Use the equations of motion, considering gravitational acceleration and initial velocity. This helps calculate the velocity at any given moment.
- What is the significance of momentum conservation in collisions? Momentum is always conserved in an isolated system, meaning that the total momentum before a collision is equal to the total momentum after it, regardless of the type of collision.
Clarifying the Concepts
By understanding the answers to these common questions, you can resolve areas of confusion and deepen your comprehension of the material. These topics are frequently tested and revisiting them will enhance your confidence in applying theoretical knowledge to practical problems.
Explaining the Most Challenging Topics
Some concepts in this chapter can be particularly difficult to grasp, often requiring deeper analysis and careful consideration. These topics may seem abstract or counterintuitive at first, but with a structured approach and thorough understanding, they become more manageable. Breaking down these concepts into smaller, more digestible parts can help you make sense of them and improve your problem-solving ability.
Addressing these challenging areas with clear explanations and examples is crucial for mastering them. Whether it’s understanding complex relationships between variables or applying multiple principles simultaneously, a methodical approach can unlock greater clarity.
How to Use Resources for Better Understanding

To truly grasp the concepts in this chapter, it’s essential to take advantage of a variety of learning resources. Textbooks, online tutorials, practice problems, and study guides can all offer unique perspectives and explanations. When used strategically, these tools can help clarify difficult topics and provide additional insight beyond the classroom material.
Making the Most of Available Materials
Here are some ways to effectively utilize resources:
- Textbooks: Review chapter summaries and practice questions to reinforce your understanding of key points. Look for detailed explanations of formulas and concepts.
- Online Tutorials: Videos and interactive lessons can provide visual explanations that make abstract concepts easier to grasp. Platforms like Khan Academy and YouTube can offer step-by-step guides.
- Study Groups: Collaborating with peers allows for the exchange of ideas and solutions, helping you see problems from different angles and gaining a deeper understanding.
- Practice Problems: Working through problems is one of the most effective ways to learn. Start with simpler questions and gradually tackle more complex scenarios.
Utilizing Online Tools and Forums
In addition to traditional materials, online forums and educational platforms can provide community support. Websites like Stack Exchange or subject-specific forums allow you to ask questions and get answers from fellow learners and experts. Don’t hesitate to explore different approaches to problem-solving that others have shared.
Effective Study Strategies for Physics
Mastering complex concepts and problem-solving techniques requires more than just reading the material. A structured and focused approach to studying can significantly enhance understanding and retention. By breaking down the study process into manageable parts, you can better organize your learning, target weak areas, and build a solid foundation in the subject.
Key Techniques for Effective Studying
To optimize your study sessions, try implementing these strategies:
- Active Learning: Engage with the material actively by taking notes, summarizing key points, and discussing concepts with peers. This method helps reinforce the material and improves long-term retention.
- Regular Practice: Consistent practice is essential, particularly with problem-solving. Work through a variety of problems to reinforce concepts and identify areas where you need improvement.
- Time Management: Break study sessions into focused blocks of time with short breaks in between. This approach prevents burnout and helps maintain concentration.
- Understand the Basics: Focus on understanding fundamental principles before tackling advanced problems. Building a strong foundation will make it easier to grasp more complex topics.
Creating a Study Schedule
Developing a study schedule tailored to your needs will help you stay organized and manage your time efficiently. Below is a sample schedule for a week of focused study:
| Day | Study Focus | Time Allocated |
|---|---|---|
| Monday | Review core concepts and formulas | 1.5 hours |
| Tuesday | Work through practice problems | 2 hours |
| Wednesday | Watch tutorial videos and summarize | 1 hour |
| Thursday | Group study session and discussion | 1 hour |
| Friday | Review notes and practice new problems | 1.5 hours |
| Saturday | Take a practice test | 2 hours |
| Sunday | Rest and light review | 1 hour |
By following a structured plan, staying consistent, and actively engaging with the material, you’ll increase your understanding and improve your problem-solving skills over time.
What to Expect in Chapter 10 Exams
When preparing for exams in this chapter, you should be ready to tackle a variety of questions that test your understanding of the key concepts, formulas, and problem-solving techniques. The exams are designed to assess not only your knowledge but also your ability to apply what you’ve learned to real-world situations. Expect a mix of theoretical questions and practical problems that challenge you to think critically and systematically.
Types of Questions
Exams in this chapter typically consist of the following types of questions:
- Multiple-Choice Questions: These questions assess your understanding of core concepts and principles. You’ll be asked to choose the correct answer from a set of options, often requiring quick recall and application of knowledge.
- Short Answer Questions: These require concise explanations of key ideas or the steps involved in solving a problem. Be prepared to show your thought process and explain the reasoning behind your answers.
- Problem-Solving Questions: These are designed to test your ability to apply formulas and concepts to solve complex problems. You may need to perform calculations and demonstrate your understanding of the material in a step-by-step format.
How to Prepare
To do well in the exam, it’s important to focus on both understanding the theoretical aspects of the chapter and honing your problem-solving skills. Here are some tips:
- Review Key Formulas: Make sure you’re familiar with all the essential formulas and know when and how to apply them.
- Practice Problems: Regularly work through problems similar to those you might encounter in the exam. This will help you become more efficient at solving them under time pressure.
- Understand Concepts: Ensure that you understand the fundamental principles behind each topic. This will help you approach questions from different angles and apply your knowledge in various contexts.
- Time Management: During the exam, allocate time wisely for each section and question. Don’t spend too long on any one problem; move on and come back to it later if needed.
By understanding the format of the exam and focusing your preparation on both theoretical knowledge and practical skills, you’ll be well-equipped to perform confidently and successfully.