How to Round Answer to One Decimal Place
When working with numbers, it is often necessary to simplify them to a more manageable format, especially in everyday calculations. This process helps in presenting values that are easier to interpret without losing essential accuracy. Whether dealing with measurements, financial data, or mathematical solutions, adjusting the level of detail is a common practice.
For many situations, reducing the complexity of a figure involves limiting its detail to just one digit beyond the main whole number. This is useful when the extra precision does not significantly impact the overall interpretation of the result, but it helps in presenting a cleaner and more understandable figure.
This approach is frequently employed across various fields such as science, finance, and engineering, where precision is important, but too much detail may cause confusion or unnecessary complexity. By simplifying results in this way, professionals can communicate findings more effectively, ensuring clarity without compromising on essential accuracy.
Understanding Decimal Place Rounding
In many cases, numbers need to be simplified to make them more practical for everyday use. This process involves adjusting figures to a level of precision that is easy to work with, while still retaining their general accuracy. The goal is to make calculations or results more comprehensible without overwhelming the reader with excessive detail.
When performing this adjustment, the decision is made based on how much precision is necessary for the given context. For example, when dealing with measurements, financial figures, or other practical data, reducing the number of digits beyond the whole number is common practice. This helps in presenting the most relevant information in a clear and concise manner.
Such modifications are essential in various fields, including science, engineering, and finance, where clarity is paramount. By simplifying the representation of numbers, professionals can ensure that the most important aspects of a calculation are emphasized, while unnecessary complexity is avoided.
Why Round to One Decimal Place
In many scenarios, simplifying a number to a specific level of precision can make it more practical for use. By limiting the amount of detail in a figure, we can present it in a way that is easier to understand and work with, without sacrificing essential information. This process ensures that the final result is both accurate and manageable.
Reducing the number of digits beyond the whole number is particularly useful when the extra precision is not necessary for the task at hand. For instance, in daily life or business, figures with excessive detail can be cumbersome and may lead to confusion. Using fewer digits makes it easier for people to interpret the data quickly and accurately.
In fields like finance, engineering, or science, this level of simplification strikes a balance between accuracy and clarity. By cutting down on unnecessary precision, we ensure that the outcome remains meaningful while being accessible and simple to communicate. This approach is especially valuable when dealing with large datasets or calculations that don’t require extreme detail to make informed decisions.
Basic Rounding Rules Explained
When simplifying numerical results, there are a few fundamental guidelines to follow. These rules help ensure that the final number is both manageable and accurate enough for its intended purpose. While the specifics may vary slightly depending on the context, the core principles remain consistent across different types of calculations.
General Guidelines
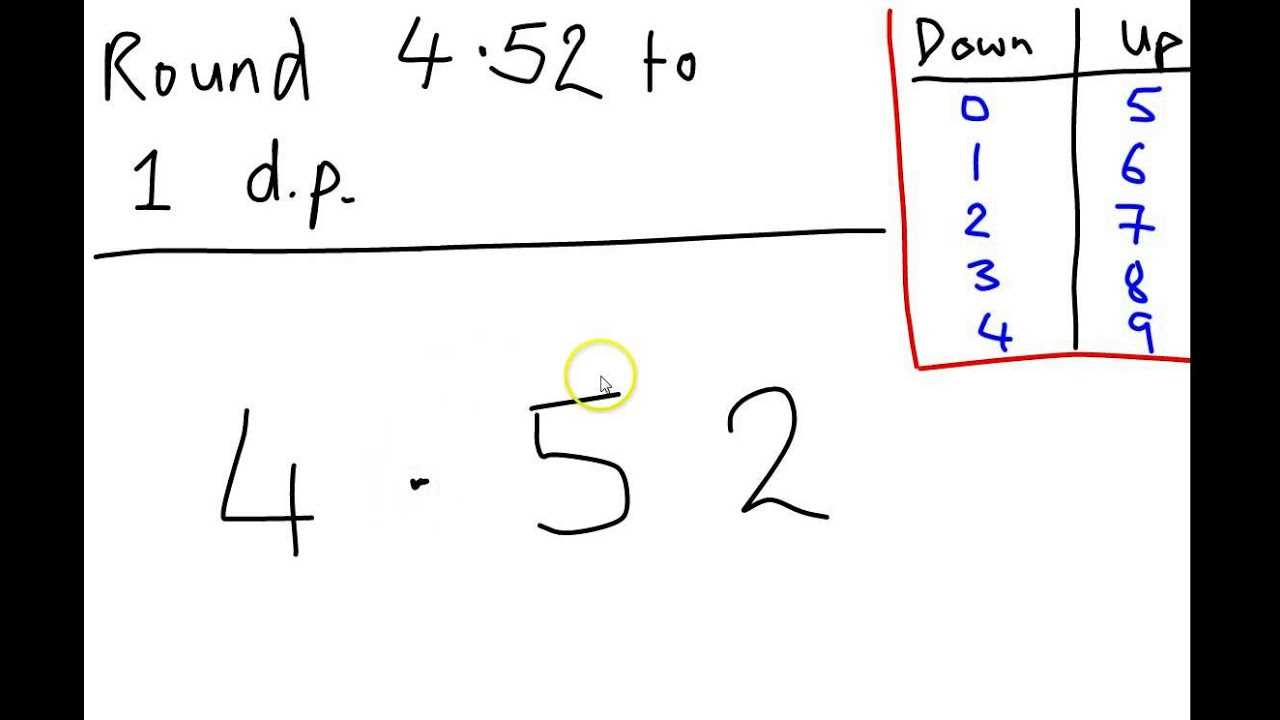
The process of reducing the precision of a number typically involves examining the digit right after the level you want to keep. If this digit is 5 or higher, the preceding number is increased by one. If it’s less than 5, the number remains unchanged. This simple method helps maintain the most relevant information while simplifying the figure.
Exceptions and Special Cases
There are instances when standard rules may need to be adjusted. For example, in certain scientific calculations, specific rounding conventions might be followed to ensure consistency in reporting results. Additionally, when working with financial data, some regulations or practices may require rounding to a specific threshold for tax purposes or pricing structures.
How to Simplify Numbers Efficiently
Efficiently adjusting the precision of a number is a key skill in many fields, from finance to engineering. The goal is to achieve a balance between accuracy and ease of use, ensuring that the result is both manageable and informative. By following a few simple techniques, you can simplify numerical results without losing essential information.
Step-by-Step Process
To adjust the precision of a number, follow these steps:
- Identify the position at which to stop the detail.
- Examine the next digit in the sequence.
- If the digit is 5 or greater, increase the preceding number by one.
- If the digit is less than 5, keep the preceding number as is.
Tips for Quick Calculation
For faster and more consistent results, consider these tips:
- Use a calculator or spreadsheet software to handle larger numbers quickly.
- Familiarize yourself with rounding rules to speed up manual calculations.
- Always double-check results to ensure no mistakes in the simplification process.
Examples of Simplifying to One Digit
When adjusting a number to a more practical level, it is helpful to look at examples that illustrate the process. These examples show how to remove unnecessary digits without affecting the overall value too much, making the result easier to work with while still maintaining its core meaning.
Simple Numerical Examples
Here are a few examples of how this simplification works:
- 3.74 becomes 3.7, as the second digit is less than 5.
- 5.86 becomes 5.9, as the second digit is greater than or equal to 5.
- 12.34 becomes 12.3, as the second digit is less than 5.
- 7.95 becomes 8.0, as the second digit is greater than or equal to 5.
Real-World Applications
Here are some contexts where this approach is commonly used:
- Financial calculations: When working with prices or budgets, simplifying values ensures clarity without overcomplicating the details.
- Scientific measurements: In experiments, simplifying readings to a practical level helps focus on the significant results.
- Everyday usage: In daily tasks such as cooking or shopping, this method ensures figures are easy to understand and apply.
Common Mistakes in Rounding
While simplifying numbers is a helpful technique, it’s easy to make mistakes in the process. These errors can lead to incorrect results, causing confusion or miscalculations. Understanding the most common mistakes is essential to avoid misrepresenting data and ensure accuracy in your calculations.
Overlooking the Rounding Rules
One common mistake is failing to apply the basic guidelines correctly. For example, sometimes people mistakenly increase the digit before the target position when the following digit is less than 5. This leads to an inflated value that doesn’t reflect the true intent of the simplification.
Misinterpreting Precision Requirements
Another error is not considering the context in which the simplification is being made. In certain situations, reducing precision too much can result in loss of important information. For instance, in scientific experiments, removing too many digits can alter the validity of the findings. It’s important to assess whether a specific level of precision is needed for the task at hand.
When to Use One Decimal Precision
Determining the right level of detail in numerical values is crucial in many fields. While it’s often tempting to use full precision, simplifying a number to a certain level can enhance clarity and utility without sacrificing accuracy. Deciding when to limit the precision depends on the context in which the number is being used.
In many practical applications, reducing the number of digits beyond the main figure makes the result easier to understand and apply. For instance, in everyday tasks like budgeting or shopping, using fewer digits helps to avoid unnecessary complexity and makes figures more accessible. Similarly, in fields like finance or engineering, this approach ensures that the data is presented clearly, without overloading the reader with excessive information.
In other situations, such as scientific measurements or highly technical calculations, it’s important to evaluate whether this level of simplification is appropriate. While simplification improves readability, it can sometimes compromise the accuracy needed for precise work. Therefore, assessing the requirements of the task at hand is key to determining the correct approach.
Rounding vs Truncating Decimal Values
When simplifying numerical results, there are two main techniques often used: adjusting the value to a specific level of precision or cutting off extra digits beyond a certain point. While both methods aim to make numbers easier to handle, they follow different principles and can produce distinct outcomes. Understanding the difference between these approaches is key to choosing the right method for a given situation.
Understanding the Difference
Adjusting the number typically involves looking at the digit immediately after the desired precision level. If that digit is 5 or higher, the preceding number is increased by one. In contrast, truncating involves simply removing all digits beyond the set point without any further changes, regardless of the value of the next digit.
When to Use Each Method
Choosing between these techniques depends on the context. In most cases, adjusting the number is preferred when you want the result to reflect a more accurate approximation. Truncating, however, may be used when a strict cutoff is needed, such as in certain financial or statistical calculations where rounding could introduce bias. The decision should be made based on the level of precision required and the purpose of the calculation.
Rounding in Different Mathematical Operations
In various mathematical operations, adjusting the precision of numbers is essential to ensure clarity and avoid overcomplicating calculations. Depending on the type of operation being performed, the method of simplifying values may vary. Each operation has its own approach to handling numbers with excessive detail, especially when the result needs to be presented in a more manageable form.
Operations Involving Addition and Subtraction
When adding or subtracting numbers, it’s important to consider the level of precision in each value. In most cases, the final result should reflect the same level of precision as the least precise number used in the operation. This means that after performing the calculation, the resulting value should be adjusted to match the required level of detail.
- If adding 3.74 and 2.3, the result should be simplified to 6.0 to match the precision of the least precise number.
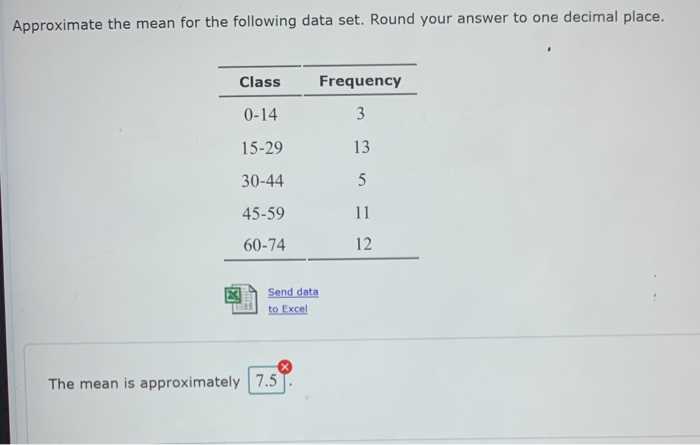
- When subtracting 7.9 from 15.37, the result would be simplified to 7.5.
Operations Involving Multiplication and Division
For multiplication and division, the general rule is to round the result to the appropriate number of significant figures. However, the final simplification often depends on the precision of the initial numbers involved. It is crucial to assess the desired level of precision and adjust the result accordingly.
- Multiplying 2.56 by 3.7 results in 9.5 when simplified.
- Dividing 16.85 by 4.2 yields 4.0 after applying the necessary simplification.
Practical Applications of One Decimal Rounding
Simplifying numerical results to a specific level of precision is an essential tool in various real-world situations. Whether it’s for ease of communication, clarity in reporting, or simply reducing complexity, this approach helps to make data more accessible and practical. Here are some common areas where this technique is applied to improve understanding and usability.
Finance and Budgeting
In financial calculations, it is often necessary to simplify values to a single digit after the decimal point. This makes transactions easier to handle, especially when dealing with currencies or expenses. Simplified numbers also enhance clarity in reports and budgets.
- When calculating prices, a value like $19.87 may be simplified to $19.9 for easier transactions.
- In budgeting, a sum like $15.43 might be adjusted to $15.4 to maintain consistency in spending categories.
Scientific and Engineering Measurements

In fields like science and engineering, precision is important, but simplifying measurements to a practical level helps in presenting results clearly. While some experiments require high precision, many calculations can benefit from limiting the detail without compromising the integrity of the findings.
- Measurements like 9.678 grams could be simplified to 9.7 grams for easier interpretation.
- In engineering designs, a calculation result of 37.129 meters might be rounded to 37.1 meters for simplicity in communication.
Rounding in Financial Calculations
In financial calculations, simplifying numerical values is often necessary to ensure consistency and clarity in reporting. By adjusting numbers to a more manageable level, financial professionals can make calculations easier to understand, while maintaining the integrity of the data. Whether for budgeting, tax computations, or price adjustments, this practice plays a key role in daily financial tasks.
Tax Calculations and Pricing
In the world of taxes and pricing, slight adjustments are commonly made to avoid dealing with long, complex figures. This simplification process helps both businesses and customers handle payments more efficiently, ensuring that the financial results remain clear and easy to follow.
| Original Price | Adjusted Price |
|---|---|
| $199.999 | $200.0 |
| $15.498 | $15.5 |
Banking and Loans
In banking, especially with loan calculations and interest rates, it’s crucial to manage the precision of values in order to simplify financial transactions. For example, loan interest rates or repayment amounts may be adjusted to a specific level, ensuring clarity for customers and preventing confusion due to excessive precision.
| Interest Rate | Adjusted Interest Rate |
|---|---|
| 5.875% | 5.9% |
| 3.278% | 3.3% |
Impact of Rounding on Data Accuracy
Adjusting numerical results to a simpler form is a common practice in many fields, but it can impact the precision of the data. While this simplification makes figures more manageable and easier to interpret, it can also introduce small discrepancies that affect the overall accuracy of the information. Understanding the trade-off between clarity and precision is essential when deciding how to handle complex calculations.
When numbers are simplified, they may no longer reflect the exact value of the original data. This can be particularly significant in situations where small differences are crucial, such as in scientific research, financial forecasting, or medical calculations. However, for most everyday applications, this level of adjustment does not lead to any major issues.
| Original Value | Simplified Value | Impact on Accuracy |
|---|---|---|
| 5.389 | 5.4 | Minimal Impact |
| 12.895 | 12.9 | Minimal Impact |
| 56.231 | 56.2 | Potential Impact |
In some cases, simplifying numbers may lead to a small loss of information, which can affect decision-making in sensitive contexts. The impact on accuracy largely depends on the degree of simplification and the importance of exact values in the specific task at hand.
How Computers Handle Numerical Simplification
Computers follow specific methods to adjust numerical values when they need to be expressed in a simpler form. These methods are designed to manage the limitations of digital systems, where numbers must often be approximated due to finite memory and processing capabilities. The process involves altering numbers to a more manageable level while attempting to minimize the loss of precision.
Most computer systems use a combination of algorithms and predefined rules to handle these adjustments. Depending on the programming language and computational environment, the approach may vary slightly, but the overall goal remains the same: to ensure that numbers are represented as accurately as possible without exceeding the system’s precision limits.
For example, when a program encounters a number with more digits than it can store, it may choose to truncate or adjust the value based on a set of predefined rules. This ensures that the result remains within the computational limits while still being sufficiently accurate for the task at hand.
Common Techniques Used:
- Truncation: The digits beyond a certain point are simply discarded without consideration of their value.
- Rounding Up: Numbers are increased to the next possible value when the next digit is 5 or greater.
- Rounding Down: Numbers are reduced to the nearest value when the next digit is less than 5.
- Bankers’ Rounding: A more balanced approach that rounds halfway cases to the nearest even number.
It’s important to note that while these methods help to maintain computational efficiency, they may introduce small discrepancies when working with extremely precise data.
Advanced Rounding Techniques
In situations requiring greater precision, there are advanced methods available for simplifying numerical values without compromising too much on accuracy. These techniques are designed to handle specific challenges that arise when working with large datasets, high-precision calculations, or complex mathematical models. By using sophisticated approaches, it is possible to reduce errors and improve the overall reliability of the results.
Advanced techniques go beyond basic adjustments and take into account specific rules and conditions that help maintain consistency and fairness across calculations. For instance, some methods attempt to minimize the cumulative error that may occur when rounding multiple values in a series. Others focus on achieving the most statistically representative outcome by applying different strategies depending on the context.
Common Advanced Methods
- Stochastic Rounding: This method introduces randomness by rounding each value based on probability, aiming to distribute rounding errors more evenly across a dataset.
- Chop and Adjust: A hybrid approach that combines truncation with correction techniques to compensate for errors in subsequent calculations.
- Gaussian Rounding: This technique adjusts values using a bell-curve distribution, allowing for a more statistically balanced representation of data.
Examples of Advanced Rounding Techniques in Practice
| Method | Description | Use Case |
|---|---|---|
| Stochastic Rounding | Uses probability to round each value based on its nearest neighbor. | Large datasets, data science applications |
| Chop and Adjust | Truncates values and then applies correction factors to minimize bias. | Financial calculations, engineering models |
| Gaussian Rounding | Applies a Gaussian distribution to adjust values based on statistical principles. | Scientific simulations, statistical analysis |
These advanced methods allow for more nuanced adjustments and are particularly useful when the highest level of accuracy is required. While they may be more complex to implement, they provide better control over how numerical data is simplified, ensuring minimal distortion and optimal results.
Rounding in Scientific Measurements
In scientific fields, numerical values often represent measured quantities that cannot be expressed with absolute precision. The process of simplifying these values helps maintain clarity while keeping the measurements accurate enough for practical use. In many cases, scientists and researchers must decide how to represent their results in a way that balances readability and correctness, particularly when dealing with significant figures or large datasets.
The goal is to reduce the complexity of numbers without introducing significant errors that could skew the outcome of experiments or calculations. This simplification process plays an essential role in fields such as physics, chemistry, and engineering, where precise data is necessary for analysis, but minor inaccuracies are often acceptable within certain limits.
When to Simplify Values in Science
- Significant Figures: Scientific measurements are often simplified based on the precision of the instruments used. For example, if a measurement tool can only provide accuracy to the nearest tenth, the result should reflect that limitation.
- Data Analysis: When analyzing large datasets, some values may be simplified to prevent overwhelming details that may not add significant value to the interpretation.
- Experimental Precision: In cases where experimental conditions limit precision, reducing the number of digits can help highlight trends or patterns without misleading the analysis.
Impact of Simplification on Results
- Minimizing Errors: Properly simplifying values ensures that rounding errors do not accumulate, which could lead to incorrect conclusions.
- Standardization: In scientific research, consistency in how measurements are reported allows for easier comparison across different studies and datasets.
- Practical Applications: For many practical purposes, highly precise values are unnecessary, and simplifying numbers makes communication of results more efficient.
While scientific measurements require careful handling of numbers, the goal is to strike a balance between precision and practicality. The ability to effectively simplify values ensures that conclusions remain valid and relevant in the broader context of research and analysis.