SOA Exam FM Syllabus Overview and Study Guide

Financial mathematics plays a critical role in understanding and managing risks, investments, and various financial instruments. Those preparing for a rigorous evaluation in this field need to be well-versed in a broad range of mathematical concepts and techniques. This section provides an overview of the key topics and skills required to succeed in the evaluation process, which is essential for anyone pursuing a career in finance or actuarial science.

Successful candidates must demonstrate proficiency in areas such as probability theory, time value of money, and the application of mathematical models to real-world financial problems. The material covered is both theoretical and practical, ensuring that individuals are well-equipped to apply these concepts to real-life situations. A structured approach to studying these topics, along with thorough preparation, is essential for achieving success.

Understanding the core areas is the first step towards mastery. This guide outlines the most important subjects, recommended study resources, and tips for efficient preparation. By familiarizing oneself with the requirements and expectations of the assessment, candidates can approach their studies with confidence and clarity.

SOA Exam FM Syllabus Breakdown

Understanding the structure and content of a financial mathematics evaluation is crucial for effective preparation. This section will guide you through the primary topics and key areas of focus that will be assessed. Mastery of these subjects is essential for passing and excelling in the evaluation.

Key Areas of Focus

The evaluation primarily covers fundamental topics such as probability theory, risk management, interest theory, and the time value of money. Candidates must be proficient in applying these concepts to solve practical financial problems. Understanding how to model financial scenarios and interpret their results is essential for success.

Preparation Strategy

Effective preparation involves not only reviewing the theoretical concepts but also practicing problem-solving skills through past questions and sample problems. Allocating time to study each topic in depth, while also focusing on weak areas, will improve the chances of performing well. It’s also beneficial to use a variety of resources, from textbooks to online courses, to ensure a comprehensive understanding of the material.

Understanding the Financial Mathematics Assessment Structure

The structure of the financial mathematics assessment is designed to evaluate candidates’ ability to apply mathematical principles to solve practical financial problems. This section breaks down the key components of the evaluation and outlines what to expect during the process. Familiarity with the structure allows for better preparation and more focused study efforts.

Format and Duration

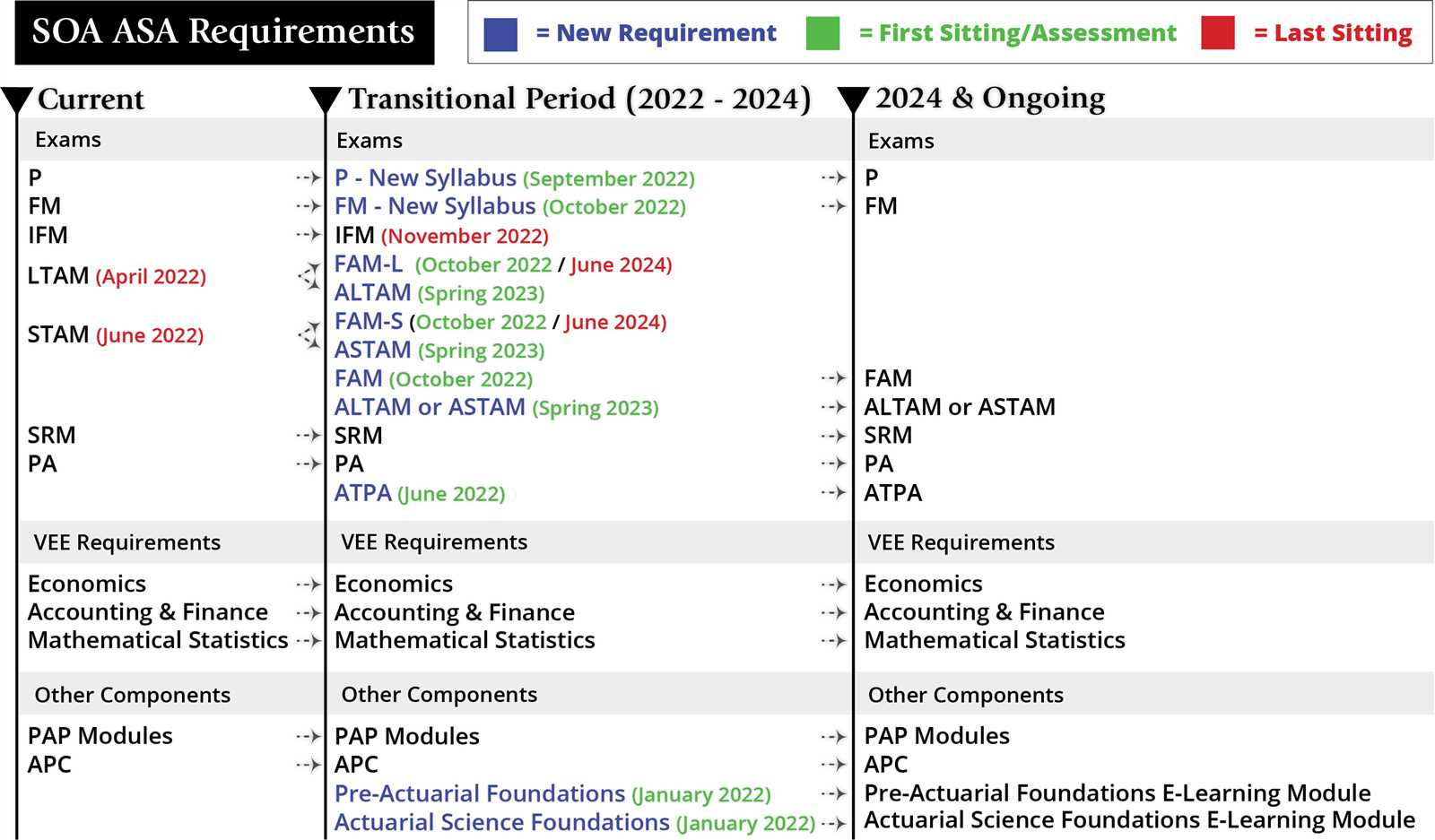
The assessment typically consists of a series of multiple-choice questions that test your understanding of various mathematical and financial concepts. It is important to manage your time efficiently, as the questions are designed to challenge both your speed and accuracy in solving problems.

• Multiple-choice format
• Time-limited, typically 3-4 hours
• Covers a wide range of topics

Topic Distribution and Weighting

Each topic covered in the assessment carries a specific weight based on its importance and complexity. The following is a general overview of how topics are distributed:

1. Probability and Statistics: 30-40%
2. Financial Mathematics and Time Value of Money: 20-30%
3. Risk Management and Financial Instruments: 20-30%
4. Interest Theory and Discounting: 10-15%

Being aware of the distribution helps prioritize your study efforts according to the weight of each subject. Focus on mastering the core concepts of higher-weighted topics for a better chance of success.

Key Topics Covered in the Assessment

The financial mathematics assessment evaluates a wide range of core concepts that are fundamental to understanding and applying mathematical techniques in financial contexts. These topics are crucial for anyone looking to pursue a career in finance or actuarial science. Below are the primary areas of focus that will be tested during the evaluation.

Core Areas of Focus

The assessment covers a variety of subjects, each integral to solving financial problems. From probability to risk management and interest theory, each area builds on the foundation needed to make sound financial decisions. A solid grasp of these topics is essential for success.

Topic	Focus Area	Percentage Weight
Probability and Statistics	Understanding distributions, risk models, and statistical methods	30%
Time Value of Money	Discounting, compounding, and financial decision-making principles	25%
Risk Management	Identifying and mitigating financial risks using mathematical models	20%
Interest Theory	Applying principles of interest and discounting to real-world problems	15%
Financial Instruments	Understanding bonds, options, and other financial products	10%

Application of Concepts

Each topic is not only theoretical but also practical, requiring candidates to apply the concepts to solve real-world financial problems. Understanding the mathematical models and their application in various financial instruments and markets is essential for performing well in the evaluation.

Mathematical Foundations for Financial Mathematics Assessment

To succeed in the financial mathematics assessment, candidates must possess a strong understanding of several fundamental mathematical principles. These core concepts form the foundation for solving complex financial problems and applying mathematical models to real-world scenarios. Mastery of these topics is essential for success in the evaluation process.

Key Mathematical Concepts

The evaluation covers a variety of mathematical topics that are critical for understanding and analyzing financial systems. These include, but are not limited to, the following areas:

• Probability Theory: Understanding distributions, expected values, and statistical measures.
• Calculus: Application of differentiation and integration in financial models.
• Linear Algebra: Use of matrices and vectors in modeling financial systems.
• Financial Ratios and Metrics: Analyzing investment performance using ratios and returns.
• Time Value of Money: Concepts of discounting, compounding, and cash flow analysis.

Preparation Strategies for Mathematical Concepts

To build a solid foundation, it’s crucial to practice these mathematical techniques regularly. Using problem sets, online courses, and textbooks can help reinforce these concepts. Focus should be placed on understanding the practical applications of each mathematical area, particularly in the context of finance.

1. Start by reviewing core probability and statistical methods.
2. Work through problems involving time value of money calculations.
3. Strengthen your calculus and algebra skills by solving finance-based problems.
4. Regularly test your understanding using mock questions and practice exams.

Risk Management in Financial Mathematics

Risk management is a crucial aspect of financial mathematics, as it involves identifying, assessing, and mitigating potential risks that can affect financial outcomes. A deep understanding of risk management principles allows professionals to make informed decisions that minimize losses and maximize returns. This section explores the key concepts and tools used in managing risk within the financial sector.

Key Concepts in Risk Management

Risk management in finance relies on a range of mathematical models and statistical techniques to evaluate and control financial uncertainties. Some of the key areas include:

• Probability Distributions: Used to model the likelihood of different outcomes and assess the potential for risk.
• VaR (Value at Risk): A method used to measure the potential loss in value of a portfolio over a defined period.
• Risk Adjusted Return: Evaluating investment performance considering both risk and return.
• Hedging Strategies: Techniques used to offset potential losses by taking opposite positions in related assets.
• Scenario Analysis: Assessing how different economic scenarios could impact a financial portfolio.

Mathematical Models for Risk Assessment

Several mathematical models are employed to analyze and predict risk in financial markets. These models often rely on historical data and advanced statistical techniques to forecast potential outcomes. Key models include:

1. Black-Scholes Model: Used for pricing options and derivatives.
2. Monte Carlo Simulation: A computational technique used to model the probability of different outcomes based on random variables.
3. Stochastic Processes: Mathematical models that describe systems evolving over time under uncertainty.

By mastering these models, candidates can develop effective risk management strategies and apply them to real-world financial situations, ensuring they are well-prepared for any financial challenges they may face.

Probability and Statistics for Financial Mathematics

Probability and statistics are fundamental pillars of financial mathematics, as they provide the tools needed to analyze uncertainty and make informed decisions in uncertain environments. These mathematical concepts help model and predict the behavior of financial markets, assess risks, and evaluate the likelihood of different financial outcomes. A solid understanding of these topics is essential for anyone aiming to apply mathematical methods to financial problems.

Key Probability Concepts

Probability theory helps to quantify uncertainty and evaluate the likelihood of various events. In the context of financial mathematics, it is essential for assessing risks and returns. Some key concepts include:

• Probability Distributions: Used to describe the behavior of random variables, such as asset prices or interest rates.
• Expected Value: The average or mean value of a random variable, important for evaluating the average outcome of financial investments.
• Conditional Probability: The probability of an event occurring given that another event has already occurred, often used in financial models to predict future outcomes based on available information.

Statistical Tools for Financial Analysis

In addition to probability, statistical methods are crucial for analyzing historical data and making predictions about future financial trends. Key statistical tools include:

• Regression Analysis: A technique used to understand relationships between variables and predict future trends based on past data.
• Variance and Standard Deviation: Measures of the spread or volatility of a data set, essential for understanding risk in financial markets.
• Correlation and Covariance: Statistical measures that assess the relationship between two variables, important for portfolio diversification and risk management.

Mastery of these concepts allows financial professionals to develop models that assess the likelihood of various financial outcomes and manage uncertainty effectively. These skills are indispensable for tackling the complex challenges posed by modern financial systems.

Interest Theory and Discounting Methods

Interest theory and discounting methods are essential concepts in financial mathematics, focusing on the time value of money and how it influences financial decision-making. Understanding how to calculate and apply interest, as well as how to discount future cash flows to present values, is vital for making informed financial and investment decisions. These principles help evaluate the worth of money over time, accounting for factors like inflation and interest rates.

Understanding Interest Calculation

Interest calculations form the foundation of many financial models, determining how much money grows over time when invested or borrowed. Two main types of interest are commonly used in financial mathematics:

• Simple Interest: Calculated on the initial principal only, simple interest is often used for short-term investments or loans.
• Compound Interest: Calculated on the principal plus any accumulated interest, compound interest is commonly used for longer-term investments, leading to exponential growth.

Discounting Future Cash Flows

Discounting is the process of determining the present value of a future sum of money. Since money in the future is less valuable than money today, understanding how to discount future cash flows is essential for valuing investments, bonds, and other financial instruments. The key methods include:

• Present Value (PV): The current value of a sum of money to be received in the future, discounted at a specific interest rate.
• Net Present Value (NPV): The difference between the present value of cash inflows and outflows, used to assess the profitability of investments.

Mastering interest theory and discounting techniques allows professionals to assess and compare the value of different financial options and make decisions based on sound mathematical principles.

Understanding Financial Products and Markets

In the world of finance, understanding the variety of financial products and the markets in which they operate is crucial for effective decision-making and risk management. Financial products range from simple instruments like bonds to complex derivatives that require advanced mathematical models for evaluation. The markets where these products are traded, including equity, debt, and commodities markets, play a critical role in determining their value and liquidity.

Types of Financial Products

Financial products come in many forms, each serving different purposes depending on the investor’s goals and risk tolerance. Some of the most common types include:

• Stocks: Equities that represent ownership in a company, offering potential dividends and capital appreciation.
• Bonds: Debt instruments issued by governments or corporations, providing regular interest payments and principal repayment at maturity.
• Derivatives: Financial contracts whose value is derived from an underlying asset, such as options and futures contracts.
• Mutual Funds: Pooled investment vehicles that allow investors to diversify across a range of securities.
• Exchange-Traded Funds (ETFs): Investment funds that trade on stock exchanges, offering exposure to indices, commodities, or sectors.

Financial Markets Overview

Financial markets provide the platform for buying and selling financial products, and they are essential for price discovery and liquidity. The main types of financial markets include:

• Equity Markets: Where stocks are bought and sold, providing companies with capital and investors with ownership in those companies.
• Bond Markets: Where debt securities are issued and traded, typically used by governments and corporations to raise funds.
• Commodity Markets: Markets where raw materials and primary agricultural products are traded, influencing global supply and demand.
• Derivative Markets: Where contracts based on the value of underlying assets like commodities, currencies, and interest rates are bought and sold.

Understanding how these financial products and markets operate enables investors, analysts, and financial professionals to assess risk, forecast returns, and make informed investment decisions in a constantly evolving financial environment.

Time Value of Money Concepts

The time value of money is a core principle in finance that emphasizes the idea that the value of money changes over time. A dollar today is worth more than a dollar tomorrow, due to the potential for earning interest or returns on investment. Understanding this concept is essential for evaluating investments, loans, and any financial decisions that involve cash flows over time. The time value of money helps in comparing financial options and determining the present or future value of money in various scenarios.

The key concepts related to time value of money include calculating present value, future value, and determining the interest rate or time period that equates different cash flows. These calculations help investors and financial analysts assess the value of future earnings and make informed decisions about their investments.

Core Time Value of Money Formulas

There are several important formulas used to calculate time value of money, each suited for different scenarios:

Concept	Formula	Description
Present Value (PV)	PV = FV / (1 + r)^t	Calculates the current value of a future sum of money.
Future Value (FV)	FV = PV * (1 + r)^t	Calculates the value of a present sum at a future date based on a given interest rate.
Interest Rate (r)	r = (FV / PV)^(1/t) – 1	Determines the interest rate that will convert a present value to a future value over a specific period.
Number of Periods (t)	t = log(FV / PV) / log(1 + r)	Finds the number of periods needed for an investment to grow from present value to future value at a given rate.

Mastering these formulas and understanding the time value of money is crucial for making sound financial decisions, whether it’s valuing an investment, comparing loan options, or analyzing cash flows over time.

Financial Models and Their Applications

Financial models are essential tools used to represent the performance and value of financial assets, companies, or projects over time. These models rely on various mathematical and statistical techniques to simulate real-world financial scenarios. They allow analysts and decision-makers to forecast potential outcomes, assess risks, and evaluate investment opportunities. Financial modeling plays a crucial role in budgeting, financial analysis, and strategic planning, helping professionals make informed choices based on quantitative data.

These models are applied in different areas of finance, such as investment analysis, risk management, pricing of financial products, and capital budgeting. By utilizing various assumptions, inputs, and formulas, financial models help determine the possible future performance of a financial asset or investment strategy.

Common Financial Models

There are several widely used financial models, each tailored to address specific needs and challenges in financial analysis:

Model	Purpose	Application
Discounted Cash Flow (DCF) Model	Valuates the present value of future cash flows based on a specified discount rate.	Used for determining the intrinsic value of a company or investment.
Capital Asset Pricing Model (CAPM)	Estimates the expected return on an asset based on its risk relative to the market.	Applied in portfolio management and investment analysis to assess the risk-return trade-off.
Black-Scholes Model	Calculates the theoretical price of options using variables such as stock price, volatility, and time to maturity.	Used in pricing options and derivatives in the financial markets.
Monte Carlo Simulation	Uses random sampling and statistical modeling to predict the likelihood of different outcomes in uncertain environments.	Applied in risk management, forecasting, and complex financial simulations.

Each of these models plays a pivotal role in various financial decision-making processes. By providing a structured approach to evaluating financial scenarios, they help professionals mitigate risk, optimize returns, and make more accurate predictions in an uncertain economic landscape.

Study Resources for Financial Mathematics Preparation

Preparing for financial mathematics assessments requires access to the right study materials and resources. These resources help candidates develop a deep understanding of the mathematical concepts and practical applications required for the test. Using a combination of textbooks, online courses, practice exams, and interactive tools ensures a well-rounded preparation strategy. The following study materials are designed to cover all aspects of the financial mathematics curriculum, helping learners strengthen their knowledge and improve their problem-solving skills.

Recommended Books and Textbooks

Books are foundational resources that provide detailed explanations, examples, and exercises related to financial mathematics. The following titles are often recommended for those looking to build a solid understanding of the subject:

• “Financial Mathematics: A Comprehensive Guide for Students” – A detailed textbook covering all key mathematical concepts in finance.
• “Fundamentals of Financial Mathematics” – Focuses on essential formulas and theories, with numerous examples and practice problems.
• “The Theory of Interest” – An in-depth guide on interest rates, time value of money, and related financial models.

Online Learning Platforms

Online platforms offer courses that provide structured lessons and interactive quizzes. They allow for flexible, self-paced learning. Popular platforms include:

• Coursera – Offers courses from top universities that cover essential financial concepts and mathematical techniques.
• Udemy – Features affordable courses with video tutorials and practice problems tailored for financial mathematics learners.
• Khan Academy – Provides free, beginner-friendly lessons on topics like probability, statistics, and financial calculations.

Combining textbooks with online resources helps ensure a comprehensive and effective study experience, preparing you for the challenges ahead. These tools can significantly improve understanding and retention, ensuring success in financial mathematics assessments.

Recommended Textbooks for Exam Preparation

When preparing for financial mathematics assessments, textbooks play an essential role in providing comprehensive coverage of the key concepts, formulas, and problem-solving strategies. These books are designed to guide students through complex topics, offering in-depth explanations and practice exercises to reinforce understanding. Selecting the right textbooks ensures that learners are well-prepared to tackle the challenges of the subject and succeed in their assessments.

The following textbooks are highly recommended for students who want to build a strong foundation in financial mathematics and related fields:

• “Mathematics of Finance” – A complete guide to financial mathematics, this textbook covers interest theory, risk management, and the time value of money with practical examples and exercises.
• “Fundamentals of Financial Mathematics” – An excellent resource for beginners, this book offers a clear introduction to the core concepts of financial modeling, with simple explanations and a variety of problems to practice.
• “The Theory of Interest” – A comprehensive text that explores the mathematical foundations of interest rates, compounding, and discounting methods. It is ideal for students looking to understand the theoretical aspects of finance.
• “Financial Mathematics: A Practical Guide for Actuaries” – Specifically tailored for actuarial students, this book provides practical examples, exercises, and case studies to help students develop the skills needed for real-world financial applications.

By working through these recommended textbooks, students can ensure that they have a strong grasp of the fundamental principles and techniques needed to excel in financial mathematics. These resources also help prepare for practical applications, enabling learners to approach any financial problem with confidence and precision.

Effective Time Management for Exam Prep

One of the most critical factors in preparing for any assessment is managing your time efficiently. Effective time management allows you to allocate enough hours to cover all necessary topics, practice problem-solving, and review key concepts, all while maintaining a healthy balance. By organizing your study schedule and prioritizing tasks, you can reduce stress and increase your chances of success.

Here are some strategies to help you manage your time effectively while preparing for your assessment:

1. Create a Detailed Study Plan

Having a structured study plan ensures you stay focused and organized throughout your preparation. Break down the material into manageable chunks and assign specific topics to each study session. This way, you can track your progress and cover all necessary areas without feeling overwhelmed.

• Set clear goals – Define what you need to accomplish each day and week.
• Be realistic – Don’t overburden yourself with too many topics at once. Allow time for review and practice.
• Allocate time for breaks – Taking short breaks between study sessions improves focus and productivity.

2. Prioritize High-Impact Areas

Some topics may carry more weight in the assessment, or you may find them more difficult to grasp. Prioritizing these areas ensures that you spend more time on the subjects that matter most.

• Identify difficult topics – Spend extra time on areas where you struggle the most.
• Focus on frequently tested areas – Review past tests to identify which topics are most commonly assessed.
• Practice regularly – Focus on practicing problems related to high-priority topics.

3. Avoid Procrastination

Procrastination is a common obstacle during exam preparation. Staying disciplined and following your study plan will help you avoid last-minute cramming.

• Set deadlines – Create small, time-bound tasks to stay on track.
• Eliminate distractions – Create a quiet study environment to maximize concentration.
• Stay accountable – Share your study goals with a friend or study partner for added motivation.

By following these time management strategies, you can maximize your study efficiency, reduce stress, and ensure you’re fully prepared for your assessment. Proper planning and disciplined execution are key to success.

Practice Questions for SOA Exam FM

One of the most effective ways to prepare for any assessment is through practicing sample problems. Working through questions allows you to apply the knowledge you’ve gained, identify areas of weakness, and improve problem-solving speed. In this section, we will provide you with a selection of practice questions designed to help you familiarize yourself with the types of problems you may encounter.

These practice questions are structured to simulate the actual assessment experience, covering a range of topics from probability to financial mathematics, ensuring you get a comprehensive review of key concepts.

Sample Practice Questions

Question	Topic	Answer
Calculate the present value of a payment of $500 due in 3 years, with an annual interest rate of 5% compounded annually.	Time Value of Money	$432.95
If a bond pays an annual coupon of $100 and is priced at $950, calculate its yield to maturity.	Bond Pricing	6.65%
What is the probability of a company’s stock price increasing by more than 10% next year, given a normal distribution with a mean of 5% and a standard deviation of 8%?	Probability	0.3085
Determine the duration of a bond with a 5% coupon, 10 years to maturity, and a yield to maturity of 6%.	Financial Models	8.12 years
What is the future value of a $1,000 investment after 5 years if it earns 4% annual interest, compounded quarterly?	Compound Interest	$1,221.99

By regularly practicing questions like these, you will not only reinforce your understanding of financial mathematics but also increase your confidence and readiness for the real test. Make sure to check your answers and understand the reasoning behind each solution to solidify your knowledge.

SOA FM Exam Grading and Scoring

Understanding how the scoring system works is essential for any assessment. Knowing how your performance will be evaluated allows you to focus on the most important areas during preparation. In this section, we will break down how the scoring is conducted, what constitutes a passing score, and how the results are calculated.

Typically, the grading system for this type of assessment is designed to fairly evaluate your understanding of the material. The scoring involves a combination of correct answers across multiple sections, with different types of questions contributing to the final score. There may be multiple-choice questions, quantitative problems, and theoretical concepts, all tested in a structured way.

After completing the assessment, your performance will be reviewed, and a score will be assigned based on the number of correct answers you provided. This score is then compared to the passing threshold set for that particular evaluation period. It is important to note that scores are often scaled to account for any difficulty variations across different test versions.

While it’s essential to aim for the highest score possible, understanding the weight of each section and how it impacts your total score can guide your study approach. Make sure to prioritize areas with higher point allocations while also ensuring a well-rounded understanding of all topics.

Common Mistakes to Avoid in Exam FM

As you prepare for the assessment, it is important to be aware of common pitfalls that can hinder your performance. These mistakes are often subtle but can significantly impact your score if not addressed properly. Being mindful of these errors will help you approach the material with a more strategic mindset and increase your chances of success.

Rushing Through Questions: One of the most frequent errors is rushing through questions without thoroughly understanding them. It’s easy to get caught up in time constraints, but taking a moment to read the problem carefully can prevent costly mistakes. Always ensure that you grasp the question’s core before attempting an answer.

Neglecting to Practice Time Management: Proper time allocation is critical. Some candidates tend to spend too much time on difficult problems, leaving too little for others. Practicing under timed conditions can help you gauge how long to spend on each section and prevent time-related stress during the actual assessment.

Overlooking Key Formulas and Theorems: Many individuals underestimate the importance of memorizing and understanding core mathematical formulas and theorems. These are the building blocks for solving problems efficiently, and not having them at your fingertips can slow you down or cause errors in calculations.

Failing to Review Your Answers: Another common mistake is not leaving enough time to review your responses. Even if you are confident about your answers, a quick second look can help catch any simple mistakes, such as miscalculations or misread questions, that could affect your final score.

Focusing Too Much on One Topic: While it is important to be strong in key areas, focusing too heavily on one topic at the expense of others can be detrimental. Ensure that you allocate study time across all topics and understand the full scope of the material, rather than just excelling in a single area.

Strategies for Passing FM Assessment

Successfully navigating the assessment requires a combination of preparation, strategy, and focus. By understanding the structure of the material and employing effective study techniques, you can improve your chances of achieving a favorable outcome. The following strategies are designed to help you streamline your preparation and approach the assessment with confidence.

Master the Core Concepts

Start by thoroughly mastering the foundational topics. Understanding key concepts, such as interest theory, probability, and risk management, is essential for solving complex problems efficiently. Spend time on the basics before advancing to more difficult material. This strong foundation will help you tackle more challenging questions with ease and accuracy.

Utilize Practice Tests and Mock Questions

Regularly practicing with mock questions and sample tests will allow you to familiarize yourself with the format and timing of the assessment. It also gives you the opportunity to identify areas of weakness that need further attention. Practicing under exam conditions can also help reduce anxiety and improve your time management during the actual assessment.

Break Down Study Sessions: Avoid cramming by breaking your study sessions into manageable chunks. Focus on specific topics during each session and allow adequate time for review. Spacing out your study sessions over a period of weeks or months will help reinforce your learning and prevent burnout.

Stay Calm and Focused: Maintaining a calm and focused mindset during your preparation and on the day of the assessment is crucial. Practice stress-reduction techniques, such as deep breathing, to help stay composed when faced with difficult questions. Confidence and mental clarity play a significant role in successfully completing the assessment.

Post-Assessment Tips and Career Guidance

Once you have completed the assessment, it is important to reflect on your performance, whether successful or not, and plan your next steps accordingly. The period after the assessment can be a time of both evaluation and future planning. By following certain strategies, you can make the most of your results and leverage them to advance your career in the financial field.

Evaluate Your Performance

Regardless of the outcome, it is essential to take time to review how you approached the assessment. This will provide insight into your strengths and areas for improvement. Some useful steps include:

• Review your mistakes: Identify which questions caused the most difficulty and determine why.
• Assess time management: Reflect on whether you spent enough time on each section and adjust your approach for future assessments.
• Seek feedback: If possible, discuss your performance with a mentor or colleague to gain perspective on where improvements can be made.

Planning Your Career Path

The completion of the assessment is just one step in your professional journey. Whether you passed or need to retake, this is an opportunity to plan your next moves. Consider these tips:

• Network: Build connections with professionals in the industry to expand your opportunities for future growth.
• Seek relevant internships: Internships or entry-level positions in finance or actuarial sciences can help you apply what you’ve learned and build practical experience.
• Continue education: Consider pursuing additional certifications or coursework to enhance your skills and knowledge base.
• Stay updated: Follow industry trends and keep learning to remain competitive in the job market.

Use this time wisely to reflect on your goals and develop a plan for the future. With the right strategy and dedication, you can turn the results of this assessment into a stepping stone toward a successful career.