Stat Textbook Answers for Better Understanding
Many students face challenges when working through complex mathematical exercises involving data analysis and probability. Understanding these concepts is essential for success, yet the path to mastering them can often feel overwhelming. With the right approach and resources, however, anyone can improve their problem-solving skills and gain clarity in the subject.
Breaking down difficult tasks and systematically addressing each component can make seemingly impossible questions more manageable. By focusing on key principles and applying a structured method, learners can tackle even the most intricate problems with confidence. Whether it’s basic calculations or advanced statistical analysis, consistent practice and the right techniques are key to mastering the material.
Developing a deep understanding of the subject helps build a solid foundation, enabling students to progress with ease. This guide will explore practical strategies for approaching various exercises, highlight common pitfalls, and offer tips to enhance your learning experience. The aim is to equip you with the tools you need to excel in your studies and achieve better results.
Stat Textbook Answers: A Comprehensive Guide
Solving complex mathematical exercises requires a clear strategy and a solid understanding of underlying principles. It’s important to approach problems systematically, breaking them down into manageable steps. The key to mastering this subject lies in knowing how to apply different techniques effectively, whether you’re dealing with data analysis, probability, or hypothesis testing.
To navigate through challenging questions, it’s essential to focus on core methods and learn how to identify patterns within problems. Once the basic concepts are understood, applying them becomes much easier. Consistent practice, along with careful review of previous solutions, can help reinforce knowledge and improve problem-solving abilities.
This guide aims to provide you with a comprehensive approach to tackling exercises efficiently. It covers strategies for each type of problem, from simple calculations to more advanced analysis, and offers practical tips for verifying your work and ensuring accuracy. By following these principles, you can boost your confidence and achieve better results in your studies.
How to Solve Stat Problems Effectively
Mastering complex mathematical problems requires more than just knowing formulas–it’s about understanding how to approach each question methodically. A structured approach can help break down even the most complicated tasks into simpler steps. By focusing on key techniques and practicing regularly, anyone can improve their ability to solve these problems with greater accuracy and efficiency.
One effective strategy is to start by carefully reading the question, identifying the given information, and recognizing the type of problem you are dealing with. This initial step ensures that you are on the right path before diving into calculations. Next, organize the data and establish a clear plan for solving the problem.
| Step | Action | Purpose |
|---|---|---|
| 1 | Read the problem carefully | Understand the question and identify given data |
| 2 | Organize the data | Set up the information in a way that makes it easier to work with |
| 3 | Choose the right method | Determine which technique or formula is needed for solving |
| 4 | Perform calculations | Follow the steps systematically to get the solution |
| 5 | Verify the result | Check for errors and ensure the answer makes sense |
By following these steps, you can approach each problem with confidence. Remember, consistent practice and a methodical approach are key to success. Over time, this will not only help you complete exercises more efficiently but also improve your overall understanding of the material.
Key Concepts to Understand in Statistics
To solve problems in data analysis, it’s essential to grasp the fundamental principles that govern the subject. These core concepts form the foundation for more advanced techniques and are crucial for interpreting results accurately. A clear understanding of these principles allows for better decision-making and improves overall problem-solving skills.
One of the first things to focus on is the nature of data itself. Understanding different types of data and how to categorize them is key. Once data is properly classified, it becomes easier to choose the right methods for analysis.
- Variables: The factors that are measured or observed, which can be classified as categorical or numerical.
- Probability: The study of likelihoods and how events occur under different conditions.
- Distributions: Understanding how data points are spread out or arranged, including normal and skewed distributions.
- Sampling: Techniques for selecting a representative sample from a population to draw conclusions about the entire group.
- Hypothesis Testing: A method for making inferences or decisions based on sample data and statistical tests.
These key concepts serve as the building blocks for all further study. By mastering them, students can tackle a wide variety of problems and interpret statistical results more effectively. Understanding how to apply these concepts ensures that data is analyzed correctly and that conclusions are valid and reliable.
Step-by-Step Approach to Textbook Questions

Solving problems efficiently requires a systematic method that ensures no detail is overlooked. Breaking down complex tasks into manageable steps allows for better focus and more accurate results. By following a clear, structured process, anyone can approach each question with confidence and precision, making difficult problems much more approachable.
Understanding the Problem
The first step is to carefully read the problem to ensure you understand what is being asked. Identify the key information and any constraints. Pay attention to the specific terms and figures provided in the question. Organize the data in a clear format, which helps in visualizing the solution process.
Applying the Appropriate Method
Once you understand the problem, select the right approach to solve it. This may involve choosing the correct formula, method, or concept based on the data at hand. Apply the chosen technique step-by-step, ensuring each part of the process is completed before moving to the next. By doing so, you’ll reduce the likelihood of errors and improve your problem-solving efficiency.
Following these steps not only helps in achieving the correct result but also builds a strong foundation for tackling more advanced exercises. By practicing this approach, you’ll gradually enhance both your understanding and your ability to handle increasingly complex tasks.
Common Mistakes in Stat Homework
When working through mathematical exercises, it’s easy to make errors that can lead to incorrect results. Many of these mistakes stem from misunderstandings or oversights in the problem-solving process. Recognizing these common pitfalls is the first step toward avoiding them and improving overall accuracy.
One frequent error is misinterpreting the question. Often, students overlook key details or fail to fully understand what is being asked, leading them down the wrong path. It’s crucial to read the problem carefully and identify all relevant information before attempting a solution.
Another mistake involves applying the wrong method or formula. Without a solid understanding of the concepts, it’s easy to select an inappropriate technique. To avoid this, ensure you are familiar with the proper approaches for different types of problems and practice applying them regularly.
Calculation errors are also quite common. In the rush to complete assignments, students sometimes make simple arithmetic mistakes or forget to carry out intermediate steps. Double-checking all calculations and taking time to verify results can significantly reduce these errors.
Finally, failing to review the solution is another mistake that can lead to oversights. After completing the problem, take the time to review your work, check for consistency, and ensure that your answer makes sense in the context of the question.
By being aware of these common mistakes and actively working to avoid them, you can improve both the accuracy of your results and your overall performance in solving exercises.
Using Online Resources for Stat Answers
In today’s digital age, numerous online tools and resources are available to help students solve complex mathematical problems. These resources can provide step-by-step solutions, explanations, and additional practice, making them valuable assets in understanding difficult concepts and improving problem-solving skills.
Finding Reliable Websites
It’s important to use trustworthy websites that provide accurate and detailed solutions. Many educational platforms offer free resources, including solution guides, video tutorials, and interactive exercises. When selecting a site, check for credibility and look for platforms that are widely recognized by students and educators.
Using Online Calculators and Tools
Online calculators and specialized tools can assist with calculations, visualizations, and graphing. These resources are especially helpful for more advanced tasks, where manual computation can be time-consuming or error-prone. While they can simplify complex problems, it’s still essential to understand the underlying principles in order to interpret the results correctly.
By leveraging online resources, you can enhance your learning experience, clarify difficult topics, and gain deeper insights into problem-solving techniques. However, it’s important to ensure that you are using these tools responsibly, complementing them with your own practice and understanding.
How to Verify Your Stat Solutions
Ensuring the accuracy of your results is an essential part of solving mathematical problems. Verification involves reviewing your steps, double-checking calculations, and validating your answers against expected outcomes. Taking the time to verify your solutions helps catch errors and enhances your understanding of the material.
Check Each Step Carefully

Start by reviewing each part of the solution process. Make sure you followed the correct method and applied the right formulas at each step. It’s easy to overlook small mistakes, especially when working through complex calculations, so a thorough review is crucial.
Compare with Known Results
If possible, compare your solution with known benchmarks or use different methods to solve the same problem. Consistency between different approaches is a strong indicator that your solution is correct. Additionally, many problems have answers available in textbooks or online resources that can serve as a reference for your work.
| Verification Step | Action | Purpose |
|---|---|---|
| Step 1 | Review the method used | Ensure the correct technique was applied to solve the problem |
| Step 2 | Check calculations | Double-check numbers and operations to identify possible errors |
| Step 3 | Cross-check with resources | Compare the result with reliable references or tools for consistency |
| Step 4 | Consider logical consistency | Ensure the result makes sense within the context of the problem |
By following these steps, you can ensure the accuracy and reliability of your solutions. Verification is a crucial skill that helps reinforce your understanding and builds confidence in your problem-solving abilities.
Helpful Tips for Stat Textbook Exercises
When tackling exercises in mathematical subjects, it’s important to approach each problem strategically. By adopting effective methods and staying organized, you can improve both your understanding of the material and your ability to solve problems quickly and accurately. Here are some tips that can help you navigate exercises more effectively.
Break Down Complex Problems
Many problems may seem overwhelming at first glance, but breaking them down into smaller, manageable parts can make them more approachable. Start by identifying the given information and what you need to find. Then, divide the problem into sequential steps, addressing one part at a time. This methodical approach helps avoid confusion and ensures you’re tackling each aspect of the question accurately.
Use Visual Aids and Tools

Sometimes, visualizing the problem can make a significant difference in understanding. Drawing diagrams, charts, or graphs can help you organize your thoughts and see relationships between data points more clearly. Additionally, make use of online tools or calculators when appropriate to help you perform calculations quickly and double-check your results.
By using these strategies, you can not only solve problems more efficiently but also gain a deeper understanding of the concepts. Consistent practice and following a structured approach will lead to improved performance in solving complex exercises.
Statistics Formulas Every Student Should Know
Mastering fundamental mathematical formulas is key to solving problems effectively. Whether you’re analyzing data, determining probabilities, or working with distributions, certain formulas are indispensable tools for success. These formulas provide a foundation for understanding more advanced concepts and ensure accurate results when applied correctly.
Basic Descriptive Statistics
One of the first areas of study involves measures that summarize data sets. Here are some key formulas:
- Mean (Average): ( bar{x} = frac{sum x_i}{n} )
- Median: The middle value in a sorted data set.
- Mode: The value that appears most frequently in a data set.
- Variance: ( sigma^2 = frac{sum (x_i – bar{x})^2}{n} )
- Standard Deviation: ( sigma = sqrt{frac{sum (x_i – bar{x})^2}{n}} )
Probability and Distribution Formulas
Understanding probabilities and distributions is essential in statistical analysis. Key formulas include:
- Binomial Probability Formula: ( P(X = k) = binom{n}{k} p^k (1 – p)^{n-k} )
- Normal Distribution Formula: ( f(x) = frac{1}{sigma sqrt{2pi}} e^{-frac{(x – mu)^2}{2sigma^2}} )
- Z-Score Formula: ( Z = frac{X – mu}{sigma} )
By familiarizing yourself with these essential formulas, you’ll be better equipped to handle various problems and deepen your understanding of statistical concepts. Mastery of these formulas will help you analyze data more efficiently and accurately.
Practical Applications of Stat in Real Life
Understanding the principles of data analysis and probability can greatly enhance decision-making in many real-world situations. From business to healthcare, these concepts are used to interpret trends, predict future outcomes, and optimize processes. Whether it’s in evaluating risks, conducting market research, or analyzing medical data, mathematical techniques are integral to shaping informed choices across various fields.
One of the most common applications is in business, where organizations use data analysis to improve marketing strategies, optimize operations, and forecast sales. For instance, companies analyze consumer behavior, track purchasing patterns, and adjust their strategies based on statistical models that predict demand and identify profitable opportunities.
In the field of healthcare, data plays a critical role in understanding disease patterns, treatment efficacy, and patient outcomes. Statistical analysis helps doctors and researchers identify risk factors, develop treatments, and make evidence-based decisions that improve patient care and public health.
Another example is in sports, where teams use data to analyze performance metrics, develop strategies, and assess player statistics. This data-driven approach has revolutionized sports management, with coaches and analysts relying on statistical models to gain a competitive edge.
These are just a few examples of how data analysis influences decisions in everyday life. By applying these methods, individuals and organizations can make better, more informed decisions that lead to greater efficiency and success.
Breaking Down Complex Stat Problems

Approaching complex problems in mathematics or data analysis can be intimidating at first. However, breaking them down into smaller, more manageable components can simplify the process and make the solution much more approachable. By following a structured method, you can identify key elements, apply appropriate techniques, and solve even the most difficult questions with confidence.
Here’s how you can break down a challenging problem step by step:
- Understand the Problem: Carefully read through the problem statement and highlight important details such as given data, what needs to be solved, and any conditions or constraints.
- Identify the Key Variables: Determine the variables involved and how they relate to one another. Write down any known values and define unknowns clearly.
- Choose the Right Approach: Decide on the appropriate methods or formulas to apply based on the type of problem. Whether it’s a probability, regression, or hypothesis testing problem, use the right tools for the job.
- Break the Problem into Parts: If the problem involves multiple steps, divide it into smaller sections. Solve each part individually and then combine the results for the final solution.
- Check for Consistency: After obtaining the solution, review it to ensure it makes sense in the context of the problem. Cross-check your steps to avoid any potential errors.
By following these steps, you can tackle any complex problem with greater clarity and precision. A systematic approach not only makes the process easier but also ensures that your solutions are accurate and reliable.
Improving Your Stat Problem-Solving Skills
Enhancing your ability to solve complex problems involves more than just knowing the right formulas. It requires developing a deep understanding of the underlying concepts, recognizing patterns, and refining your approach to each type of challenge. By consistently practicing and applying strategic methods, you can improve both the speed and accuracy of your problem-solving skills.
Master the Fundamentals

Start by ensuring that you have a solid grasp of the basic principles. Understanding core concepts such as probability, data distribution, and hypothesis testing forms the foundation for tackling more complex problems. Regularly reviewing and revisiting these fundamental topics will help reinforce your knowledge and increase your confidence in applying them.
Practice with a Variety of Problems

One of the most effective ways to improve your problem-solving abilities is through consistent practice. Work through a wide range of problems, from easy to difficult, to become comfortable with various types of questions. As you tackle different scenarios, you’ll start recognizing common techniques and patterns that can simplify the problem-solving process.
Additionally, take time to reflect on mistakes and challenges faced during problem-solving. Identifying where errors occurred and understanding the reasoning behind your mistakes will help you avoid similar pitfalls in the future. The key is persistence and continuous improvement through experience.
Best Practices for Studying Stat Textbooks
Effective studying involves more than simply reading through chapters. To truly understand the material and retain knowledge, it’s essential to adopt structured and strategic study habits. This approach allows you to not only master the content but also apply it successfully in practical situations.
Organize Your Study Sessions
Creating a well-organized study plan is key to mastering the material. Break down your study sessions into smaller, manageable chunks to avoid feeling overwhelmed. Here are a few tips for structuring your study time:
- Set Clear Goals: Define what you aim to achieve during each study session, whether it’s mastering a specific concept or completing a set of exercises.
- Prioritize Difficult Topics: Identify areas where you’re struggling and focus more time on those topics to ensure a deeper understanding.
- Review Regularly: Consistent review of previously studied material helps reinforce your learning and improve retention.
Active Learning Techniques
Active engagement with the material leads to better comprehension and long-term retention. Instead of passively reading through content, try the following techniques:
- Practice Problems: Solve problems related to the concepts you’ve studied. This helps reinforce your understanding and improves your problem-solving abilities.
- Summarize Key Concepts: After studying a section, summarize the key points in your own words. This will help solidify your understanding and make it easier to review later.
- Teach What You Learn: Explaining complex ideas to someone else, or even to yourself, forces you to internalize the material more deeply.
By adopting these study habits and actively engaging with the material, you’ll develop a stronger foundation and be better equipped to tackle complex problems effectively.
Understanding Data Interpretation in Stat

Interpreting data correctly is a critical skill in any field that relies on statistical analysis. It goes beyond simply looking at numbers; it involves understanding the meaning behind the data, recognizing patterns, and drawing conclusions that are both valid and meaningful. Developing strong data interpretation skills enables you to make informed decisions based on evidence rather than assumptions.
Key Elements of Data Interpretation
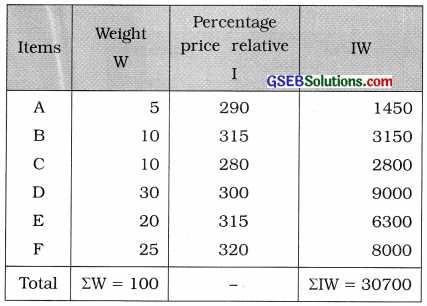
When analyzing data, it’s important to keep several key elements in mind. These components form the foundation for a clear and accurate interpretation of statistical information:
- Context: Understand the context in which the data was collected. This helps ensure the data is being interpreted correctly and relevant conclusions are drawn.
- Trends and Patterns: Look for trends, relationships, or patterns within the data. Identifying these can provide insights into underlying causes or predict future outcomes.
- Outliers: Be aware of outliers or anomalies in the data, as they can significantly affect the overall interpretation. Determining whether to include or exclude outliers is a crucial part of the analysis process.
Effective Techniques for Data Interpretation
To interpret data effectively, it’s helpful to use various analytical techniques that allow for deeper insights. Below are some approaches you can use when analyzing and interpreting statistical information:
- Visualization: Create graphs, charts, or plots to visually represent the data. This makes it easier to spot trends, outliers, and correlations.
- Descriptive Statistics: Use measures such as the mean, median, mode, and standard deviation to summarize key aspects of the data.
- Hypothesis Testing: Perform hypothesis tests to confirm whether your interpretations are supported by the data or if further analysis is needed.
By focusing on these key elements and techniques, you can improve your ability to interpret data accurately and make more reliable conclusions from your analysis.
Commonly Asked Questions About Stat Textbooks
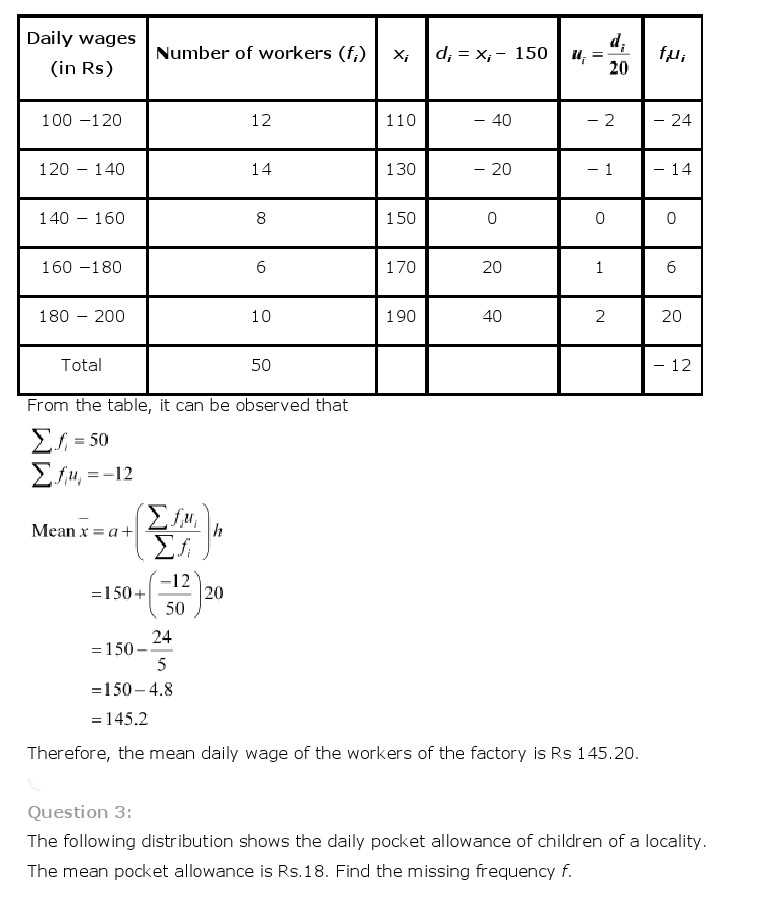
When it comes to understanding statistical concepts and solving related exercises, many learners have similar questions. These queries often revolve around how to effectively use learning materials, how to approach complex problems, and what resources can further aid in mastering the subject. This section addresses some of the most frequently asked questions to provide clarity and guidance to students.
How can I improve my understanding of statistical concepts?
To strengthen your understanding, focus on breaking down concepts into smaller, manageable sections. Use real-world examples to apply theoretical knowledge and practice regularly by solving exercises. It also helps to review the material multiple times and seek out additional explanations or tutorials if needed.
What are the best strategies for tackling challenging problems?
When facing difficult problems, start by reading the question carefully and identifying the key information. Break the problem down step by step and use diagrams or visual aids when appropriate. If a problem involves formulas, ensure you understand each component and its relationship before applying it to the question.
How important are practice exercises in mastering statistical methods?
Practice exercises are essential for reinforcing concepts and techniques. They allow you to apply theoretical knowledge to solve real-world problems and improve problem-solving skills. The more problems you solve, the more comfortable you will become with the material.
Should I rely on external resources or solve problems independently?
While external resources such as online tutorials, forums, and study guides can provide valuable insights, it’s crucial to first attempt solving problems on your own. Independent practice will help you develop critical thinking skills and gain confidence in your ability to tackle new challenges.
By addressing these common concerns, students can feel more confident in navigating statistical learning materials and continue to improve their proficiency in the subject.
How to Approach Stat Textbook Practice Tests
Practice tests are a crucial tool in mastering statistical concepts. They provide an opportunity to assess your understanding and identify areas that need improvement. When preparing for these assessments, it is essential to have a structured approach to ensure that you get the most out of the practice test experience. This section offers practical tips for tackling these exercises effectively.
Prepare in Advance
Before you begin the practice test, review the relevant concepts and formulas. Ensure that you understand the methods required to solve typical problems, such as data analysis, hypothesis testing, or probability calculations. It is helpful to go over previous lessons and solve example problems to refresh your knowledge and boost confidence.
Approach Each Question Methodically
When working through each question, read it carefully and highlight key pieces of information. Identify the question type–whether it involves calculation, interpretation, or analysis–and consider the most appropriate method to use. Break down complex problems into smaller, manageable steps. Don’t rush; ensure that each step is clear and logical before moving on to the next.
Time Management is Key
Practice tests are often timed, so managing your time efficiently is essential. Set a specific amount of time for each section or problem and stick to it. If you get stuck on a particular question, move on to the next one and come back to it later. This ensures that you complete the test in the allotted time and gives you a chance to revisit more difficult questions with a fresh perspective.
Review Your Solutions
Once you complete the test, take the time to review your answers. Look for mistakes or areas where you made incorrect assumptions. Understanding where you went wrong will help reinforce your knowledge and prevent similar errors in future assessments. It is also helpful to look at the correct solutions and compare them to your approach to learn from your mistakes.
By following a thoughtful and organized strategy, practice tests can become an effective tool for reinforcing your learning and improving your performance in statistical assessments.