Work Energy Calculations Answers for Physics Classroom

When studying physical forces and their effects, it’s crucial to grasp how different forms of motion and interaction influence objects. This section will explore the fundamental principles that explain these phenomena, offering practical approaches to solve related challenges. Mastery of these concepts is essential for any student seeking to improve their problem-solving skills in this field.

By breaking down complex situations into simpler components, we can effectively understand the underlying mechanics that govern movement and force. With the right strategies, anyone can tackle even the most challenging problems in this area. Key methods and examples will guide you through the process of making precise predictions and solving for unknown variables.

Understanding Work and Energy Concepts

In any study of mechanics, it’s important to recognize how forces influence the movement and behavior of objects. The relationship between force, motion, and how energy is transferred or transformed plays a central role in solving related problems. By grasping these core ideas, you gain the tools to predict outcomes in a wide range of physical situations, from simple motions to more complex systems.

To better understand how these forces interact, it helps to consider different types of motion and the ways in which they can affect an object’s state. Both potential and kinetic variations contribute to changes in an object’s position or speed, offering key insights into how external forces can cause movement or stop it altogether.

Type of Motion	Effect on Object	Common Example
Constant Velocity	No change in speed or direction	Car cruising on a highway
Acceleration	Increase in speed	Object falling under gravity
Deceleration	Decrease in speed	Car braking to stop

Key Principles of Work in Physics

Understanding how forces lead to changes in an object’s motion is fundamental to solving physical problems. When a force acts on an object, it can cause it to move or change its velocity. The relationship between the applied force, displacement, and the object’s resulting motion is central to many concepts in mechanics.

There are a few core ideas that help explain how energy is transferred when forces act on objects. These include the magnitude of the applied force, the direction of movement, and how far the object moves in the direction of the force. Grasping these factors is key to accurately solving problems that involve any kind of force-induced movement.

Key Factor	Effect on Object	Example
Force Magnitude	Larger forces cause greater movement	Pushing a car versus pushing a bicycle
Direction of Force	Movement occurs in the direction of the force	Applying force to lift an object upwards
Displacement	Movement in the same direction as the force	Object sliding across a flat surface

Energy Types and Their Importance

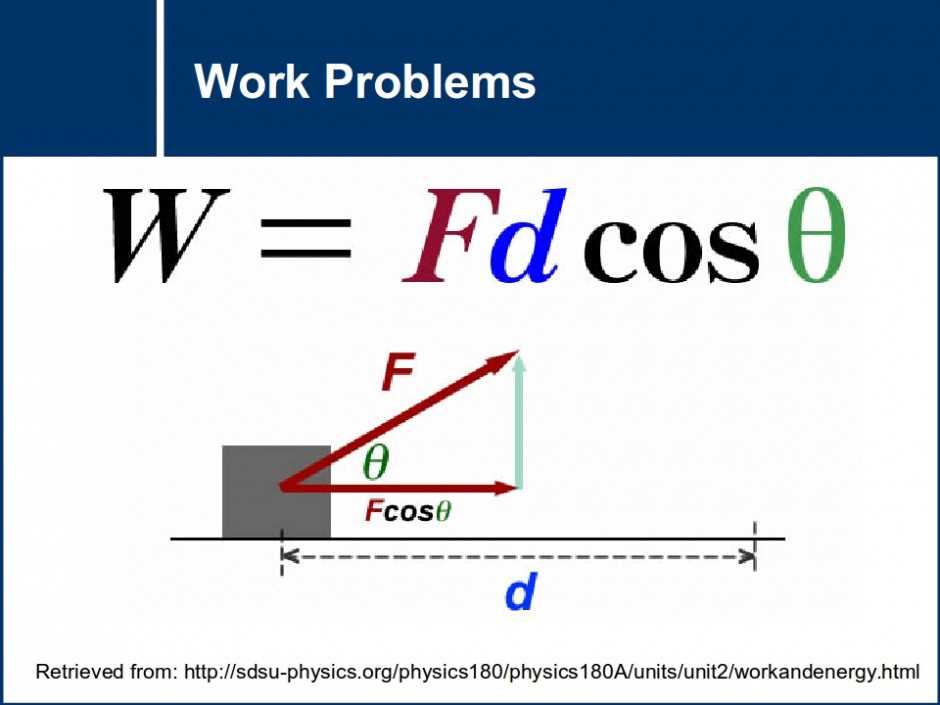
In any system, different forms of stored or transferred power play crucial roles in determining how objects move or change their state. Understanding how each type influences the system’s behavior helps in predicting and controlling outcomes in various real-world applications. Whether it’s through motion, position, or even changes in state, these forms are essential for solving practical problems and understanding natural processes.

Each form has its own unique characteristics and can be converted from one type to another under certain conditions. For example, energy can shift from being stored within an object’s position to being active in its motion, or even be transformed into heat under specific circumstances. Understanding these types and their transitions is vital for a complete understanding of mechanical systems.

Calculating Work in Different Scenarios

When forces act on objects, the effects vary depending on the situation. The amount of movement produced and how much of it is in line with the applied force can be assessed through specific methods. These approaches are useful for determining how forces contribute to changes in motion across various contexts, from simple tasks to complex mechanical systems.

In different scenarios, factors like the direction of movement, force magnitude, and distance covered all play a part in determining the outcome. By applying fundamental principles, we can evaluate how these factors interact and calculate the results for each unique situation. Understanding how to approach each case is essential for accurate problem-solving in mechanics.

Understanding Potential Energy Calculations

When objects are positioned at certain heights or within specific fields, they possess a form of stored power due to their position. This stored power can be transformed into motion or other types of activity when released. To assess the amount of potential stored within a system, we need to understand how certain factors like height, mass, and gravitational pull contribute to its value.

By analyzing the relationship between these factors, we can quantify the amount of potential within an object and predict how much will be converted into other forms of motion when conditions change. This process is vital for solving many real-world problems where changes in position or height affect the overall system behavior.

Kinetic Energy and Its Role in Work

When objects are in motion, they possess a specific form of power that is directly related to their speed and mass. This power plays a crucial role in determining how objects interact with their environment, especially when it comes to affecting other objects or systems. Understanding this form of power is key to explaining how movement influences changes in mechanical systems.

Factors Affecting Motion and Power

The amount of power an object holds while moving depends on two main factors: its mass and its speed. A heavier object moving at high speeds will possess more power than a lighter object moving at the same velocity. This relationship helps explain why larger objects require more force to accelerate or decelerate, and why high-speed collisions can cause significant effects.

Applications of Kinetic Energy in Real Life

In everyday situations, this type of power is crucial for understanding how vehicles move, how objects collide, and how systems are designed to either absorb or harness this power. From designing safer vehicles to calculating the force needed to stop a moving object, the concept plays a fundamental role in many engineering and technological applications.

The Work-Energy Theorem Explained

The relationship between force applied to an object and the resulting change in its motion is fundamental in understanding how physical systems behave. This principle explains how applying a force over a distance leads to a change in an object’s velocity. By connecting the applied force to the resulting motion, this concept allows us to calculate how much movement is produced under certain conditions.

At its core, this theorem shows that the force applied to an object is directly proportional to the change in its kinetic state. It helps in understanding how a system moves and reacts when influenced by external forces, and it can be used to predict the outcome of various interactions. Here’s how it breaks down:

• Force applied to an object over a distance causes a change in velocity.
• Acceleration results from the application of a force over time.
• Resulting Motion depends on the magnitude of the applied force and the object’s mass.

This principle is not limited to simple scenarios but extends to complex systems where multiple forces interact. By applying this theorem, we can determine how forces will affect objects, whether they are in motion or at rest.

Energy Conservation in Physics Problems

In many physical scenarios, certain quantities remain constant despite changes in other variables. This principle is crucial for solving problems where the total amount of motion or stored power within a system is conserved. By understanding how different forms of power are interrelated and how they can transform, we can predict the behavior of systems without needing to track every individual factor.

In these situations, the key idea is that although power may shift from one form to another, the overall amount remains unchanged. Whether it is transferred between objects or transformed from potential to motion, the total sum stays constant. This concept is useful for solving problems where objects undergo changes but the overall “balance” remains intact.

Units of Measurement in Work Calculations

When assessing how forces impact objects or systems, understanding the units used to measure the quantities involved is essential. These units help quantify the magnitude of applied forces, distances traveled, and the changes in motion or position. Accurate measurements ensure that results are consistent and meaningful, allowing for comparisons across different systems and problems.

In most physical situations, the units used for distance, force, and resulting motion follow standard conventions. These units make it easier to calculate and interpret the impact of various forces on a system. Here are the key units used in such evaluations:

• Force: Measured in newtons (N), which quantifies the push or pull applied to an object.
• Distance: Measured in meters (m), representing how far an object moves in a given direction.
• Resulting Motion: The resulting change is often measured in joules (J), representing the amount of power transferred.

Using these standard units makes it possible to work with various physical systems in a consistent way, helping to apply concepts across a wide range of real-world problems.

How Force Affects Work and Energy

When a force is applied to an object, it can lead to a change in the object’s motion or position. This change is directly influenced by the amount and direction of the applied force. The greater the force and the longer it acts, the more significant the change in the system, which can affect the overall state of motion or stored power within that system.

Force can transform stored power into motion or cause an object to stop moving. The amount of change depends on how much force is applied and how long it is exerted over a given distance. This interaction between applied forces and resulting motion is crucial for understanding how systems behave under different conditions.

For example, when you push an object, the force you apply influences how quickly the object accelerates. The more force you use, the faster it moves. Similarly, if you stop applying force, the object’s movement will gradually slow down or come to a stop.

Work Done by Variable Forces

In many physical scenarios, the force acting on an object is not constant but changes over time or with position. This variation complicates the direct calculation of the impact of the force on the object. Instead of a simple multiplication of force and distance, it becomes necessary to integrate the changing force over the object’s path to determine the overall effect. This concept is key in understanding how dynamic systems behave when forces are not constant.

Understanding Variable Forces

Variable forces change in magnitude and direction during the course of their application. Examples of such forces include spring forces, forces due to friction, and gravitational forces acting on objects with varying mass or distance from a source. In these cases, determining the effect of the force requires considering how it behaves at each point along the path.

Mathematical Approach to Variable Forces

To calculate the overall result of a variable force, we use integration to sum up the contributions of the force at each infinitesimal point along the object’s motion. This allows us to account for the changes in magnitude and direction over time or space, providing a more accurate representation of the object’s movement or potential transformation.

Force Type	Example	Equation
Spring Force	Force exerted by a spring	F = -kx
Gravitational Force	Force between two masses	F = G(m1m2)/r²
Frictional Force	Force opposing motion between surfaces	F = μN

Power and Its Relation to Work

Power is a key concept that describes how quickly a force causes a change in an object’s state. It is closely related to the amount of motion or transformation an object undergoes over a period of time. While force and distance are important, the rate at which these changes occur–how fast or slow–can be just as crucial in understanding the dynamics of a system.

Understanding the Concept of Power

Power measures how rapidly a system’s state is altered when a force is applied. It is often associated with machines, engines, and human performance, where tasks are completed at different rates. The faster the rate of change, the more power is involved. For instance, if two people lift the same object, the one doing so more quickly is exerting more power.

Mathematical Formula for Power

To quantify power, we use a simple equation that relates the total force applied and the time over which it acts. This allows us to calculate how much force is exerted in a given time frame, providing a clearer picture of system efficiency and performance.

Quantity	Unit	Formula
Power	Watts (W)	P = W / t
Force	Newtons (N)	F = ma
Distance	Meters (m)	d = vt

Solving Work-Energy Problems Step by Step

When tackling problems that involve forces and motion, it’s essential to break the process into manageable steps. By approaching each scenario methodically, you can systematically determine how different factors interact and ultimately find the solution. A clear step-by-step approach ensures that all elements are considered and that the solution is accurate and complete.

Step 1: Identify Known Values

Before beginning any problem, start by identifying all the given information. This can include values for mass, velocity, distance, and force. Understanding the problem’s specifics allows you to know which variables you need to calculate and how to proceed.

• Mass of the object (m)
• Initial and final velocities (v₁, v₂)
• Distance moved (d)
• Applied force (F)

Step 2: Choose the Right Formula

Select the appropriate equations based on the problem’s requirements. Different scenarios will involve different formulas, such as those related to motion, forces, or displacement. Make sure to choose the right one to reflect the given situation accurately.

• For constant force: F = ma
• For kinetic situations: K = ½mv²
• For potential energy changes: P = mgh

Step 3: Perform the Necessary Calculations

After selecting the right equations, plug in the known values to perform the calculations. Ensure that units are consistent throughout, as this is critical for accurate results. Often, you may need to rearrange equations to solve for the unknown variable.

Step 4: Check the Solution

Once the problem is solved, review the final answer to ensure it makes sense in the context of the question. Does the magnitude seem reasonable? Are the units correct? Checking your result helps avoid errors and ensures the solution aligns with physical expectations.

Examples of Work Energy Calculations

Understanding how to apply various formulas and principles to real-world situations is essential for mastering force and motion-related problems. By working through examples, you can see how different variables come into play and how to calculate the desired values in a structured manner. Below are some practical examples to illustrate these concepts in action.

Example 1: Kinetic Energy of a Moving Car

Suppose you need to find the kinetic energy of a car with a mass of 1,500 kg moving at a speed of 20 m/s. Using the formula for kinetic energy:

• K = ½mv²

Substitute the given values into the equation:

• K = ½ × 1500 kg × (20 m/s)²
• K = ½ × 1500 × 400
• K = 300,000 J

The kinetic energy of the car is 300,000 joules.

Example 2: Gravitational Potential Energy of an Object

Next, let’s calculate the gravitational potential energy of a 10 kg object lifted 5 meters above the ground. The formula for gravitational potential energy is:

• P = mgh

Substitute the known values:

• P = 10 kg × 9.8 m/s² × 5 m
• P = 490 J

The gravitational potential energy of the object is 490 joules.

Example 3: Force Applied to Move an Object

Finally, consider a situation where a force of 100 N is applied to move an object 3 meters in the direction of the applied force. To calculate the work done, use the formula:

• W = F × d

Substitute the given values:

• W = 100 N × 3 m
• W = 300 J

The work done in moving the object is 300 joules.

Common Mistakes in Work Calculations

When dealing with force and motion problems, several common errors often arise, leading to incorrect results. These mistakes typically involve misinterpretations of formulas, incorrect assumptions, or errors in unit conversions. Understanding and avoiding these mistakes can help ensure more accurate solutions and a deeper comprehension of the concepts involved.

1. Ignoring Direction of Force

A frequent mistake occurs when the direction of the applied force is not properly considered. If the force is not aligned with the direction of motion, it can lead to incorrect results. For example, in scenarios where force is applied at an angle, the horizontal and vertical components must be accounted for separately. Failing to break the force into these components can lead to inaccurate calculations.

2. Incorrect Unit Conversion

Another common issue is failing to convert units properly. Whether it’s converting mass from kilograms to grams, distance from meters to kilometers, or time from seconds to hours, inconsistent units can throw off the entire calculation. Always double-check that the units match those required in the formula and convert them accordingly to avoid errors.

3. Using Wrong Formula for Specific Situations

Different problems require specific formulas. Sometimes, using a general formula without considering the exact nature of the problem can lead to mistakes. For example, when dealing with potential or kinetic scenarios, one formula may not apply if friction or other forces are involved. Ensure the appropriate equation is used based on the given conditions.

4. Failing to Account for External Forces

Many problems involve additional forces, such as friction or air resistance, that must be taken into account when solving for movement or energy. Ignoring these external factors can lead to overly simplified solutions that don’t reflect the actual scenario. Always consider the complete system and all forces acting on it to avoid incomplete or incorrect results.

Work-Energy Calculations in Real Life

Understanding how force and movement interact is not only important in academic contexts but also plays a significant role in many practical, everyday situations. From designing efficient vehicles to understanding how machines work, these principles have a wide array of applications. By applying these concepts, we can make more informed decisions, whether in engineering, sports, or even daily activities like lifting objects.

1. Automotive Design and Fuel Efficiency

In the automotive industry, the efficiency of a vehicle is often measured by how effectively it converts fuel into motion. Engineers calculate the forces acting on a vehicle, considering factors like air resistance, road friction, and the vehicle’s weight. By understanding these factors, they can design engines that maximize output while minimizing energy loss.

2. Sports and Athletic Performance

Athletes use principles of force and motion to enhance their performance. For example, sprinters must understand how to apply maximum force in the shortest time, while weightlifters focus on generating the most power to lift heavy loads. Coaches and trainers rely on these calculations to improve athletes’ techniques and training regimens.

3. Construction and Lifting Equipment

In construction, heavy machinery like cranes, forklifts, and pulleys rely on force and movement calculations to safely lift and move objects. Engineers and operators must ensure that the machinery’s lifting capacity matches the load being carried, preventing accidents and ensuring the efficiency of operations.

4. Roller Coasters and Amusement Rides

Amusement parks use force and motion calculations to design thrilling yet safe rides. Engineers calculate the forces experienced by riders, ensuring that acceleration, deceleration, and turns are safe while providing an exciting experience. These calculations help determine the optimal speed, angle, and height for the ride’s structure.

5. Renewable Energy Generation

Wind turbines, water wheels, and other renewable energy sources operate based on principles of motion and force. By calculating how much movement is converted into usable power, engineers can improve efficiency and make these energy sources more effective. These calculations are key in harnessing the natural forces of wind and water to produce electricity.

Additional Resources for Learning Work Energy

For those looking to deepen their understanding of how forces and movement interact, there are many helpful materials available. These resources can guide you through both basic principles and more advanced topics, whether you’re a beginner or an experienced learner. Exploring various formats such as books, online platforms, or interactive tools can provide a well-rounded approach to mastering these concepts.

1. Online Learning Platforms

Websites and apps offer structured courses and tutorials that break down key concepts into manageable lessons. Platforms like Khan Academy, Coursera, and edX provide free courses that cover topics related to motion, force, and mechanical systems. Many of these courses include video lessons, quizzes, and exercises to reinforce learning.

2. Interactive Simulations and Tools

Simulations are an excellent way to visualize abstract concepts. Websites like PhET Interactive Simulations and Wolfram Alpha allow users to experiment with virtual models of force, motion, and machines. These interactive tools let learners adjust variables and see how changes impact outcomes in real-time, providing valuable hands-on experience.

3. Textbooks and Reference Materials

For a more in-depth study, textbooks on mechanics and motion provide a thorough understanding of underlying principles. Popular books like “Fundamentals of Physics” by Halliday and Resnick and “Classical Mechanics” by Herbert Goldstein are excellent resources. These books offer both theoretical explanations and practical examples for solving complex problems.

4. Educational Videos and Documentaries

Video tutorials and documentaries can offer a visual and often entertaining approach to complex topics. YouTube channels like MinutePhysics, Veritasium, and Physics Girl feature videos that explain fundamental principles in accessible and engaging ways. Documentaries on engineering and technology also provide real-world examples of how force and motion are applied in everyday life.