Calculus 1 Practice Exam with Answers

Achieving proficiency in fundamental mathematical concepts is crucial for success in higher education and various professional fields. Developing a strong foundation allows you to solve complex problems with ease and confidence. This section aims to guide you through key topics that will help enhance your understanding and boost your performance.
By engaging with a variety of problems and reviewing detailed solutions, you can sharpen your problem-solving abilities. It’s not just about memorizing formulas but also about building a deeper comprehension of how different principles work together to form the bigger picture.
In this guide, you’ll explore strategies to tackle a wide range of questions, review key techniques, and develop the necessary skills to excel. Regular practice and thorough analysis will ultimately lead you to greater mastery and success.
Understanding the Basics of Calculus
To build a strong foundation in mathematics, it is essential to understand the fundamental concepts that govern the way we analyze and solve problems involving change and motion. Mastery of these foundational principles is crucial for tackling more advanced topics and for applying mathematical reasoning to real-world situations.
Key Concepts to Focus On
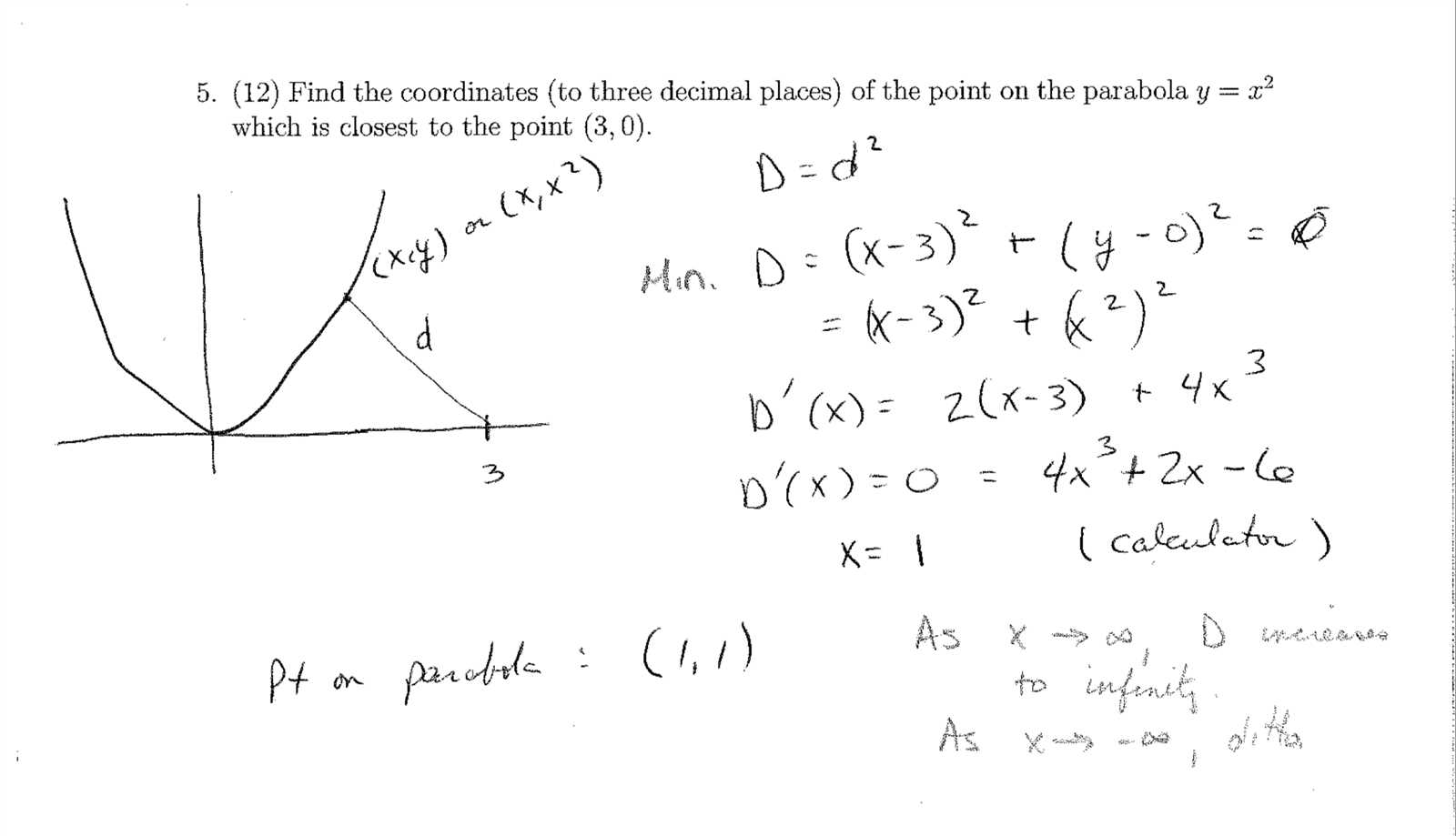
- Limits: The idea of approaching a value and understanding behavior near specific points.
- Functions: The relationship between variables and how one affects the other.
- Derivatives: Techniques for understanding how one quantity changes in relation to another.
- Integrals: Methods for calculating the area under curves or the accumulation of quantities.
Each of these principles forms the core of understanding how mathematical models are developed and applied. By focusing on these essential elements, you can begin to see the connections between various concepts and their practical implications.
Approaching Problem-Solving

When solving mathematical problems, it is important to break down complex situations into simpler parts. Understanding each step of the process helps in grasping the larger concept at hand. Using the right techniques and recognizing patterns in problems can make challenging questions more manageable.
- Start by clearly defining the problem.
- Identify key variables and their relationships.
- Apply appropriate methods and check your work carefully.
By thoroughly understanding the basics, you will be better prepared to tackle more advanced topics and gain greater confidence in your mathematical abilities.
Why Practice Exams Are Essential
Engaging in mock tests and simulated challenges is a crucial step toward improving your ability to solve mathematical problems efficiently. These tools not only help you identify your strengths but also reveal areas where improvement is needed. By actively working through problems similar to those on actual assessments, you gain the confidence and skills necessary to perform well under pressure.
Boosting Confidence and Reducing Anxiety

One of the primary benefits of mock assessments is the boost in confidence they provide. Familiarity with the format and types of questions allows you to approach the real test with less stress. Knowing that you’ve encountered similar problems before helps reduce anxiety and improve focus during actual evaluations.
- Develop a sense of familiarity with the problem-solving process.
- Gain confidence in your ability to manage time effectively.
- Identify areas that require additional focus or review.
Improving Time Management and Efficiency

Time management is a critical skill that can be honed through repeated practice. By timing yourself while solving problems, you learn how to pace yourself and avoid rushing through key steps. This helps you allocate your time wisely during the real test, ensuring that you complete each section with attention to detail and accuracy.
- Learn to prioritize questions based on difficulty.
- Practice pacing to ensure enough time for each section.
- Refine strategies for tackling time-consuming problems.
Regular engagement with these activities allows you to refine your problem-solving techniques and sharpen your skills, ultimately leading to better performance when it counts the most.
Key Concepts in Calculus 1
Understanding the foundational principles of mathematics is essential for solving complex problems involving rates of change, accumulation, and motion. These core concepts form the building blocks of more advanced topics and have wide applications in various fields such as physics, engineering, and economics. Mastering these key ideas will allow you to approach challenges with greater confidence and precision.
The main areas to focus on include the concept of limits, which helps describe behavior as values approach certain points, and the derivative, which measures how quantities change relative to one another. Additionally, integrals are used to calculate accumulated quantities, such as areas under curves or the total of some continuous value. These fundamental ideas serve as tools for breaking down real-world problems into manageable solutions.
By mastering these essential topics, you’ll be better prepared to tackle more complex problems and apply mathematical thinking to practical situations.
How to Approach Your Practice Test

Approaching a simulated assessment requires careful planning and a strategic mindset. It’s not just about solving problems, but about practicing effective techniques that will help you refine your skills and boost your performance. By following a methodical approach, you can make the most out of each question and enhance your understanding of key concepts.
Start with a Clear Strategy: Before you begin, take a moment to familiarize yourself with the layout of the test. Understanding the format and the types of questions will help you manage your time effectively. Break down the test into sections and prioritize areas where you feel least confident.
Manage Your Time Wisely: Allocate time for each section based on its difficulty and the number of questions. Don’t spend too much time on one problem–move forward and return to it later if necessary. This helps ensure that you have time to address every question, increasing your chances of success.
Stay Calm and Focused: Stress can cloud your judgment, so it’s important to stay calm throughout the test. Read each question carefully, take a moment to think through your approach, and tackle each one systematically. The more you practice this, the easier it becomes to maintain focus when it matters most.
Common Mistakes to Avoid
When solving mathematical problems, it’s easy to fall into certain traps that can lead to errors. Recognizing and avoiding these common mistakes is crucial for improving your performance and ensuring that you arrive at the correct solution. By being aware of these pitfalls, you can develop better problem-solving habits and avoid unnecessary setbacks.
Overlooking Key Details
One of the most frequent mistakes is not paying attention to the finer details of the problem. It’s easy to rush through a question, missing important information such as signs, coefficients, or constraints. Always read each question carefully and take the time to underline or highlight critical details before proceeding with your solution.
- Check for negative signs, fractions, and constants.
- Ensure all variables are clearly defined.
- Note any specific instructions or conditions mentioned in the problem.
Relying Too Much on Memorization
While memorizing formulas and methods can be helpful, it’s more important to understand the underlying principles behind them. Relying too much on rote memorization can lead to mistakes, especially when the problem is presented in a different format or requires a new approach. Aim to grasp the core concepts and logic, as this will help you adapt to various types of problems.
- Focus on understanding the ‘why’ behind formulas.
- Practice applying concepts to a variety of problems.
- Don’t hesitate to break down complex problems into smaller, more manageable parts.
Avoiding these common mistakes will improve your problem-solving skills and help you approach challenges more effectively.
Breaking Down the Test Questions

When facing a set of problems, it’s important to approach each one systematically rather than trying to solve them all at once. By breaking down each question into smaller, more manageable parts, you can better understand what is being asked and how to tackle it effectively. This method helps you stay organized and focused, ultimately leading to more accurate solutions.
Step 1: Understand the Question
Before jumping into solving a problem, make sure you fully understand what the question is asking. Read it carefully and identify key elements such as given values, what you are asked to find, and any conditions or restrictions provided. Clarifying these aspects early on will set you up for success.
- Underline or highlight important terms and numbers.
- Write down any conditions or restrictions explicitly mentioned in the problem.
- Ensure you know what is being asked before attempting a solution.
Step 2: Break Down the Process
Once you understand the problem, break it into smaller steps. Identify what methods or techniques are needed to solve the problem, and outline a clear plan before proceeding. This structured approach will help keep you on track and prevent missing critical steps.
- Identify the main concept or technique required.
- Write out the formula or equation that might be helpful.
- Work through the problem step-by-step, checking your progress as you go.
By breaking down each problem into smaller tasks, you ensure that no details are overlooked and that you are solving the problem in a logical, efficient manner.
Improving Problem-Solving Skills
Sharpening your ability to tackle complex questions is essential for success in any mathematical discipline. The more you practice approaching problems systematically and logically, the more confident and efficient you will become. Developing strong problem-solving skills not only enhances your ability to perform well in assessments but also improves your analytical thinking in real-world scenarios.
Developing a Systematic Approach

One of the most effective ways to improve problem-solving skills is by adopting a structured approach. Begin by analyzing the question to fully understand what is being asked, then break the problem into smaller, more manageable parts. This will help you identify the best methods to apply and prevent you from getting overwhelmed.
- Start with identifying the key variables and relationships.
- Break the problem into smaller sections that are easier to handle.
- Choose the appropriate methods based on the problem type.
Practice Consistently
Regular practice is essential for honing problem-solving techniques. The more problems you solve, the more patterns and strategies you will recognize. Over time, you’ll be able to approach even the most complex questions with greater ease and speed.
- Set aside time for focused problem-solving sessions.
- Try solving problems from different topics to broaden your skills.
- Review your solutions and learn from any mistakes.
By practicing consistently and following a methodical approach, you can significantly enhance your problem-solving abilities, ultimately leading to better results in assessments and real-life applications.
Reviewing Solutions Step by Step
Carefully reviewing your solutions is a crucial part of the learning process. It allows you to identify any mistakes or oversights, reinforce your understanding, and ensure that your approach is both accurate and efficient. By breaking down each solution and examining the steps in detail, you can gain valuable insights into areas where you may need improvement.
The process of reviewing should not be rushed. Instead, take your time to revisit each step, check the logic, and ensure that every calculation or assumption is correct. This approach will help you spot errors, deepen your understanding, and improve your problem-solving strategies over time.
Step 1: Revisit the Problem Statement
Before diving into the solution, go back to the original question. Ensure you fully understand the problem and the required outcome. This step ensures that you are addressing the correct issue and that your method aligns with what’s being asked.
- Read the problem carefully and identify the key details.
- Check if you’ve missed any important constraints or values.
- Make sure your solution focuses on answering the right question.
Step 2: Break Down Each Step
After revisiting the problem, go through each step of your solution one by one. Examine your reasoning, formulas, and calculations to verify that each part of the solution is logical and correctly executed. This step-by-step review helps you identify where errors may have occurred and strengthens your understanding of the material.
- Recheck each formula and calculation for accuracy.
- Verify that your logic aligns with the principles you’ve learned.
- Look for any skipped steps or misapplied methods.
By breaking down your solutions and reviewing them thoroughly, you’ll not only correct any errors but also improve your problem-solving approach for future challenges.
Techniques for Time Management
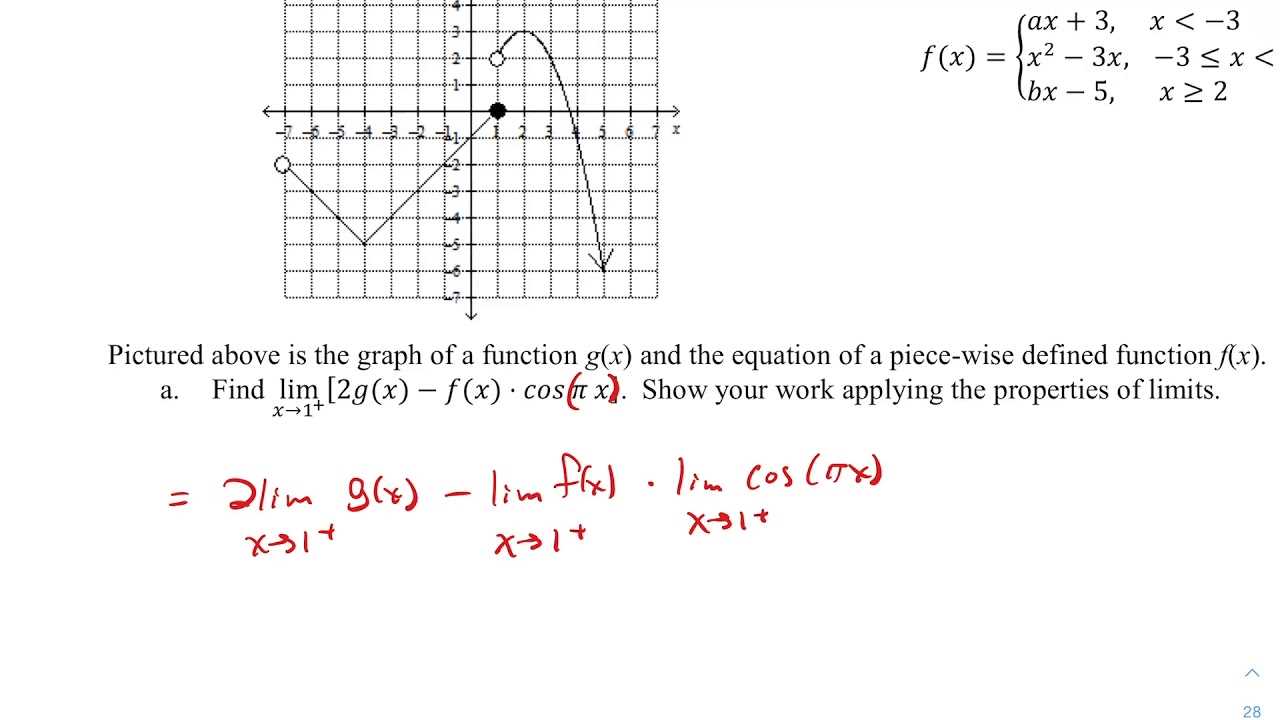
Effective time management is essential for maximizing productivity and ensuring that you can complete tasks within the given timeframe. By applying certain strategies, you can stay organized, prioritize your work, and avoid unnecessary stress. Proper time management allows you to approach challenges calmly and efficiently, leading to better performance and results.
Whether preparing for a task, solving a set of problems, or working through complex questions, planning your time is key. You can allocate specific blocks of time for each task, minimize distractions, and focus on one thing at a time. The right techniques will help you make the most of the time available, ensuring that you complete your work to the best of your ability.
Plan Your Tasks Ahead of Time
One of the best ways to manage time effectively is to plan in advance. Creating a schedule or outline for your tasks helps you stay on track and prevents you from wasting time figuring out what to do next.
- Make a to-do list and prioritize the most important tasks.
- Break larger tasks into smaller, more manageable parts.
- Set specific time limits for each task to stay focused.
Avoid Multitasking
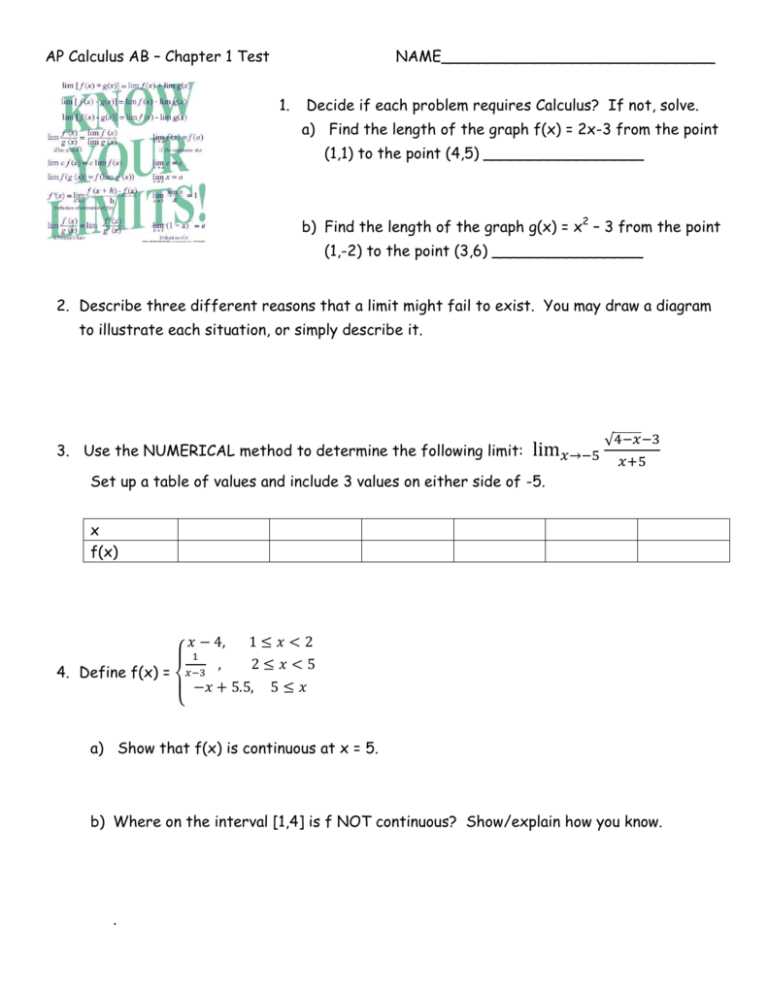
While it may seem efficient to juggle multiple tasks at once, multitasking often leads to mistakes and wasted time. Instead, focus on one task at a time, complete it, and then move on to the next. This will improve the quality of your work and speed up the process.
- Concentrate on the current task without distractions.
- Finish one task before starting another.
- Allow time for short breaks to maintain focus.
By following these strategies, you can effectively manage your time, reduce stress, and achieve better results in less time.
Analyzing Commonly Tested Topics
In any rigorous assessment, certain subjects tend to appear more frequently than others. Identifying and focusing on these recurring topics can greatly enhance your ability to perform well. By understanding which concepts are commonly tested, you can direct your study efforts more efficiently and increase your chances of success. Analyzing these areas helps you prioritize the most relevant material and approach your preparation with a strategic mindset.
Breaking down commonly tested areas allows you to focus your attention on the concepts that matter most. This focused approach not only saves time but also ensures that you’re thoroughly prepared for a variety of challenges.
Key Areas to Focus On
While the content may vary, there are specific concepts that tend to be highlighted in most assessments. By understanding these key topics, you can anticipate the types of problems you are most likely to encounter.
- Understanding the fundamentals of functions and their properties.
- Mastering derivatives and how they relate to rates of change.
- Solving problems involving integration and area under curves.
- Identifying limits and their importance in determining continuity.
- Working with optimization problems and real-world applications.
Strategies for Mastering Common Topics
To fully prepare for these commonly tested topics, it’s crucial to not only understand the theory but also practice applying the concepts through various problems. Familiarity with the types of questions typically asked can make a significant difference in your performance.
- Review the fundamental principles behind each topic before attempting problems.
- Practice solving problems from past assessments to understand the format and structure.
- Work on progressively challenging problems to build confidence and skill.
By focusing on these commonly tested areas and employing targeted strategies, you can significantly boost your chances of success in any assessment.
Tips for Mastering Derivatives

Understanding how to calculate the rate of change of a function is a fundamental skill in many mathematical fields. Mastering this concept requires both a solid grasp of the rules and a lot of practice applying them to different types of problems. By focusing on key strategies, you can develop a deeper understanding of derivatives and improve your problem-solving abilities.
To effectively work with derivatives, it’s essential to approach each problem systematically. This means recognizing the function type, selecting the appropriate rule, and carrying out the necessary calculations carefully. The more familiar you become with the rules and methods, the quicker and more accurately you’ll be able to solve derivative problems.
Understand the Core Rules
There are several key rules that form the foundation of differentiating functions. Knowing these rules inside and out will make it easier to tackle even the most complex problems.
- Power Rule: For any function in the form of ( f(x) = x^n ), the derivative is ( f'(x) = n cdot x^{n-1} ).
- Product Rule: When differentiating the product of two functions, use the formula ( (f cdot g)’ = f’ cdot g + f cdot g’ ).
- Quotient Rule: For a division of functions, apply ( frac{d}{dx} left(frac{f(x)}{g(x)}right) = frac{f’ cdot g – f cdot g’}{g^2} ).
- Chain Rule: Use this rule for composite functions: ( frac{d}{dx} [f(g(x))] = f'(g(x)) cdot g'(x) ).
Practice with Different Function Types
The key to mastering derivatives lies in consistent practice. While the core rules are universal, the type of function you’re working with may require slight adjustments in how you apply the rules. Different types of functions such as polynomials, trigonometric, exponential, and logarithmic functions all come with their own unique challenges.
- Polynomials: Apply the power rule to differentiate terms like ( ax^n ).
- Trigonometric Functions: Familiarize yourself with the derivatives of sine, cosine, and other trig functions.
- Exponential and Logarithmic Functions: Understand how to differentiate functions involving ( e^x ) and ( ln(x) ).
By focusing on these key techniques and practicing regularly, you’ll significantly improve your ability to master derivatives and handle any related problem with confidence.
Understanding Integrals and Their Applications
Integrals are a core concept in mathematical analysis, representing a way to calculate the accumulated value of a function over a specific range. This concept is crucial not only in mathematics but also in various real-world applications, such as physics, engineering, economics, and even biology. The process of finding the integral allows us to understand areas, volumes, and rates of change in a broader context.
At its core, the integral is used to determine quantities that cannot be easily calculated through simple arithmetic. For example, it can help find the area under a curve or the total distance traveled by an object when velocity is known. Understanding how to compute integrals and applying them to solve practical problems is an essential skill for anyone studying advanced mathematics or working in related fields.
Key Types of Integrals
There are two main types of integrals, each serving different purposes in both theoretical and applied mathematics.
- Indefinite Integrals: These integrals represent a family of functions and are used to find the general form of a function given its rate of change. They do not have specified limits and result in an expression that includes a constant of integration.
- Definite Integrals: These integrals calculate the accumulated value over a specific interval. They are used to find quantities such as areas under curves, volumes of solids, and work done in physics.
Practical Applications of Integrals
Integrals are more than just theoretical constructs; they have numerous practical applications that make them essential tools in many fields.
- Area Calculation: Integrals allow us to calculate the area under a curve, which is useful in fields such as economics for determining total profit or in biology for analyzing growth patterns.
- Physics and Engineering: In these fields, integrals are used to compute work, energy, and force, as well as to solve problems involving motion, electric fields, and fluid dynamics.
- Population Models: In biology and environmental science, integrals are applied to model population growth, decay, and the spread of diseases, helping to predict future trends and outcomes.
By understanding integrals and practicing their applications, one can gain valuable insight into the fundamental processes governing both theoretical and real-world systems.
Strategies for Solving Limits
Solving limits is a fundamental skill in mathematical analysis, helping to understand the behavior of functions as they approach certain points or infinity. Approaching limit problems systematically can lead to efficient solutions and a deeper understanding of the function’s behavior in various scenarios. Whether dealing with indeterminate forms or evaluating limits at infinity, there are several techniques that can be applied to simplify and solve these problems accurately.
Here are some key strategies to consider when solving limit problems:
| Technique | Description |
|---|---|
| Substitution | Direct substitution is often the simplest method. If the function is continuous at the point of interest, substitute the value directly to find the limit. |
| Factoring | When the limit results in an indeterminate form like 0/0, factoring the numerator and denominator can often eliminate the indeterminate form and simplify the expression. |
| Rationalizing | Rationalizing the numerator or denominator can help to resolve square roots or other irrational expressions, allowing for easier simplification of the limit. |
| L’Hôpital’s Rule | When the limit results in an indeterminate form such as 0/0 or ∞/∞, L’Hôpital’s Rule can be applied by differentiating the numerator and denominator separately until a solvable limit is reached. |
| Using Squeeze Theorem | For functions that are difficult to evaluate directly, the Squeeze Theorem can be used by comparing the function to two other functions whose limits are easier to calculate. |
By mastering these techniques, you can effectively solve a wide range of limit problems, ensuring a strong foundation in understanding how functions behave near specific points or at infinity.
Making the Most of Practice Answers

When preparing for assessments, reviewing solutions to problems is essential for improving understanding and reinforcing key concepts. Simply having the solutions is not enough; it’s important to analyze each step carefully to ensure that you not only arrive at the correct answer but also understand the process behind it. By focusing on the reasoning, methods, and techniques used in each solution, you can turn every review session into a learning opportunity that strengthens your skills and deepens your comprehension.
Understand the Steps Involved
It’s crucial to break down each step of the solution to grasp how it was derived. Don’t just focus on the final result. By reviewing each action taken–such as simplifying an expression, applying a rule, or manipulating terms–you can identify areas where you might need further clarification. Understanding these small steps will help you apply similar techniques in future problems.
Identify Common Mistakes and Patterns
Practice solutions often reveal common mistakes that students make. By identifying these errors in your solutions, you can prevent them from reoccurring. Additionally, analyzing the pattern of correct steps helps to internalize the strategies that work best, enabling you to approach new problems more efficiently.
To maximize the value of reviewing solutions, take the time to work through similar problems on your own before checking the solution. This will help reinforce your problem-solving abilities and ensure that you’re not just memorizing steps but developing the analytical skills needed to succeed independently.
Preparing for the Real Exam

Effective preparation for any major test involves more than just reviewing material–it requires a strategic approach to ensure that you’re ready to tackle challenges under time pressure. It’s essential to review all key concepts, practice problem-solving techniques, and hone your ability to recall important formulas and methods quickly. By simulating the conditions of the real test, you can reduce anxiety and improve your confidence when it’s time to sit down for the actual assessment.
Effective Study Plan
One of the most critical components of preparation is creating a focused study schedule. Prioritize areas where you feel less confident and allocate extra time to those topics. Break your study sessions into manageable chunks to avoid burnout, and be sure to incorporate breaks to maintain your focus. Consistency is key–regular, structured practice helps to reinforce concepts and enhance retention.
Simulating Test Conditions
To improve performance under timed conditions, it’s helpful to simulate the actual testing environment. Set aside dedicated time to work through practice problems without distractions, just as you would during the real assessment. This will help you get accustomed to managing your time effectively and applying your knowledge efficiently under pressure.
| Study Tip | Description |
|---|---|
| Review Key Concepts | Focus on core principles and methods that are often tested. |
| Time Management | Practice solving problems within a set time frame to simulate real test conditions. |
| Identify Weak Areas | Dedicate extra time to the topics that you find most challenging. |
| Stay Organized | Keep your study materials and notes organized for easy reference. |
By focusing on these strategies and sticking to a well-organized study routine, you’ll be better prepared to approach the test with confidence. Remember, consistent practice and strategic planning are key to performing well when the time comes.
Building Confidence Through Practice

Achieving a high level of confidence in any subject comes from consistent effort and focused repetition. The more you engage with the material and work through challenges, the more comfortable you become with the content and the test-taking process. This approach helps reduce uncertainty and allows you to approach each problem with clarity and assurance. Confidence isn’t built overnight, but through continuous, deliberate effort over time.
Focused Repetition
One of the best ways to build confidence is through focused repetition. The more you solve problems, the more you begin to recognize patterns and techniques that are commonly used in solutions. This repeated exposure helps reinforce your knowledge and strengthens your problem-solving skills. As you see progress, you become more confident in your abilities, which translates to better performance when faced with new challenges.
Tracking Progress and Celebrating Milestones

It’s essential to track your progress along the way. Keep a record of the areas you have mastered and the ones that need more attention. Celebrating small wins along the way boosts morale and provides motivation to continue. Every time you successfully work through a problem or a concept, take a moment to acknowledge the improvement, no matter how small it may seem.
By consistently putting in the effort, you’ll build not only your knowledge but also your confidence. With time and dedication, what once seemed difficult will become more manageable, and your assurance will continue to grow.