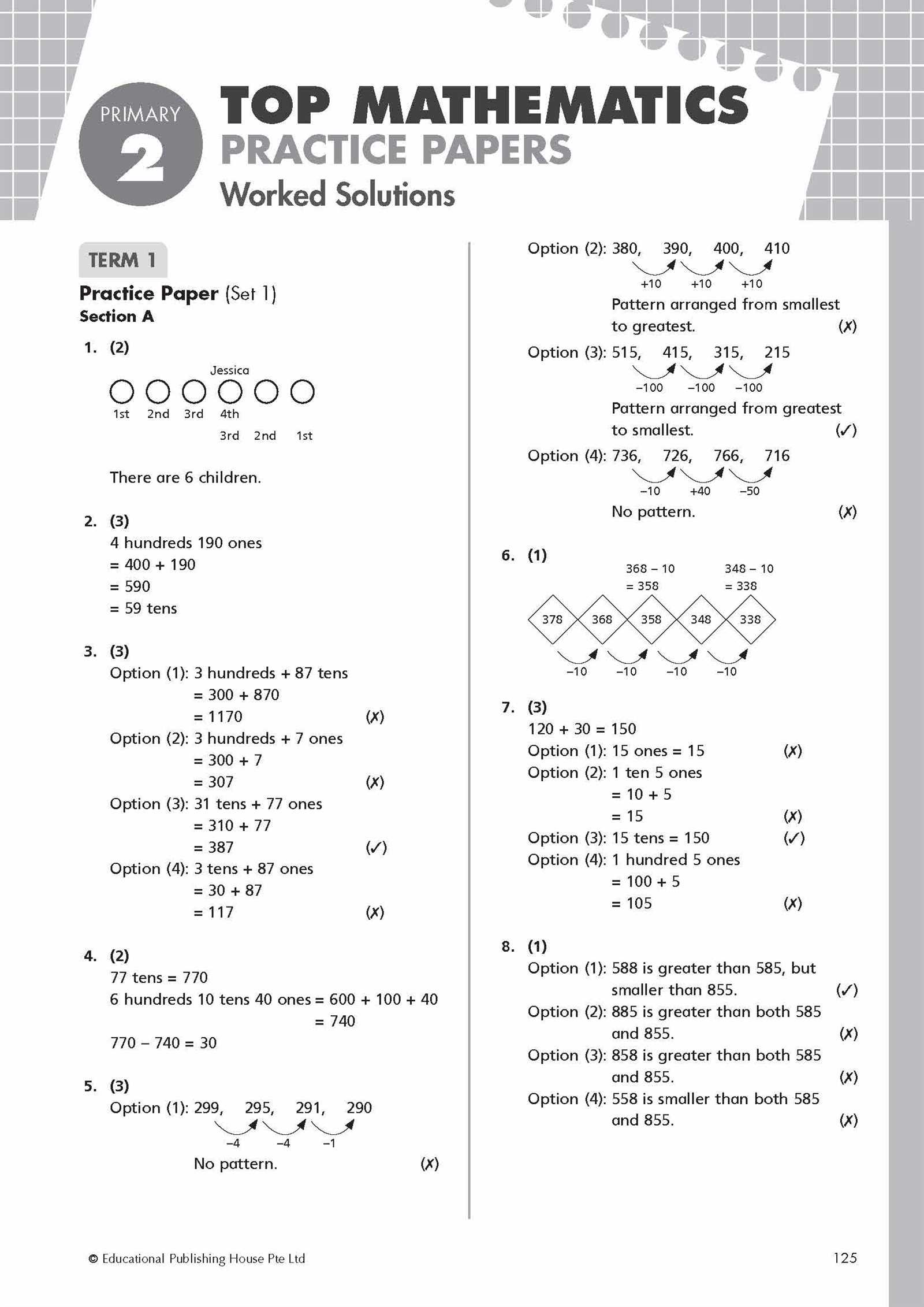
Glencoe Algebra 1 Chapter 8 Practice Test Answers

Understanding fundamental mathematical principles is essential for success in solving complex problems. This section focuses on reinforcing core skills through comprehensive exercises designed to enhance comprehension and retention of critical material.

With carefully crafted problems, learners can build their confidence and improve their ability to apply concepts in real-world situations. Each challenge is intended to help strengthen reasoning and problem-solving techniques that are crucial for mastering advanced topics.

Clear explanations and step-by-step solutions provide a solid foundation for improving mathematical abilities. By consistently practicing these exercises, students can achieve greater proficiency and gain a deeper understanding of the subject.

Glencoe Algebra 1 Chapter 8 Overview

This section introduces essential mathematical techniques that lay the groundwork for tackling more advanced topics. Focus is placed on honing critical skills that help students develop a deeper understanding of problem-solving methods. By the end of this unit, learners will gain the confidence to approach more complex equations with ease.

Key Concepts and Skills

Throughout this unit, the focus is on mastering the basics of equation manipulation and interpretation. It covers core strategies that involve simplifying expressions, working with inequalities, and applying formulas in various problem scenarios. These fundamental skills form the basis for more complex tasks encountered later in mathematics.

Building Proficiency and Confidence

By engaging with carefully structured exercises, students can reinforce their understanding of core principles. The goal is not just to solve problems but to cultivate a strategic mindset that allows learners to approach mathematical challenges effectively. With continued practice, the skills developed in this section will provide the foundation for tackling more advanced topics with confidence.

Key Concepts in Algebra 1

This section focuses on the fundamental principles that serve as the building blocks for solving mathematical problems. A solid understanding of these core concepts is essential for progressing in more advanced studies and applying mathematical thinking in everyday situations.

• Equation Solving – Understanding how to isolate variables and manipulate expressions is crucial for solving equations.
• Expressions and Simplification – Mastering the ability to simplify complex expressions and identify like terms is a key skill.
• Inequalities – Learning how to solve and graph inequalities helps develop a deeper understanding of relationships between variables.
• Functions and Graphing – Gaining proficiency in plotting graphs and understanding how functions represent real-world scenarios is essential for advanced topics.
• Factoring – Factoring polynomials is an important technique for simplifying and solving expressions efficiently.

These foundational concepts are critical for progressing through higher levels of mathematics. Each skill reinforces the others, ensuring that students build a well-rounded understanding of the subject. Mastering these ideas provides the tools necessary to approach increasingly complex problems with confidence.

Understanding Chapter 8 Material

Mastering the material in this section requires a thorough understanding of key mathematical principles and methods. By breaking down each concept into manageable parts, learners can build a strong foundation that supports more complex problem-solving techniques. This approach allows for a clearer grasp of the relationships between variables and how to manipulate them effectively.

As you progress through the exercises, focus on fully grasping the underlying logic behind each problem. Pay attention to the steps involved in finding solutions and familiarize yourself with common patterns. With consistent practice and careful review, the material will become more intuitive, and students will be able to apply these skills in various scenarios with greater ease.

How to Approach Practice Tests

Successfully tackling exercises designed to evaluate your understanding requires a strategic approach. Instead of simply focusing on finding solutions, it is crucial to understand the steps involved in solving each problem. A methodical and thoughtful approach will help you develop deeper insights and reinforce essential skills.

Start with Familiar Problems

Begin by reviewing questions that are similar to the ones you have already practiced. This will help you build confidence and identify the techniques that work best for solving specific types of problems. Starting with easier questions can also warm up your mind and prepare you for more challenging tasks.

Time Management and Focus

Set a clear time limit for each problem to simulate exam conditions. Focus on maintaining accuracy rather than rushing through tasks. Taking regular breaks between sections can also help maintain concentration and prevent fatigue, ensuring a more effective study session.

Step-by-Step Problem Solving Guide

To effectively solve mathematical challenges, it is important to follow a structured approach that breaks down each problem into manageable steps. By addressing each part methodically, you can simplify complex tasks and ensure accuracy in your solutions. This guide will walk you through the general steps to help you approach and solve problems with confidence.

1. Understand the Problem

The first step is to carefully read the problem and identify what is being asked. Pay attention to key details and make sure you understand the given information. Highlight or underline important data, and think about the relationship between the variables involved.

2. Plan Your Approach

Once you have a clear understanding, decide on the method you will use to solve the problem. This could involve applying a formula, isolating a variable, or working through a series of steps. Outline your approach before you begin, and make sure you know what tools and techniques will be necessary for solving the problem.

Common Mistakes in Algebra 1

When working through mathematical exercises, it’s easy to make small errors that can lead to incorrect answers. These mistakes often stem from misunderstandings or overlooked details. Being aware of common pitfalls can help you avoid these issues and improve accuracy in your work.

Misunderstanding Order of Operations

One of the most frequent errors involves misapplying the order of operations. It’s crucial to remember the sequence: parentheses first, followed by exponents, multiplication and division, and then addition and subtraction. Skipping steps or performing operations out of order can lead to incorrect results.

Neglecting to Simplify Expressions

Another common mistake is failing to fully simplify expressions before moving to the next step. Whether it’s combining like terms or factoring an expression, skipping this important step can make solving the problem much harder and may lead to errors in later stages.

Effective Test-Taking Strategies

To succeed in assessments, it’s important to approach each problem with a clear strategy. A calm and organized mindset can make a significant difference in both speed and accuracy. By implementing specific techniques, you can increase your chances of performing well and reduce the likelihood of errors under pressure.

First, read all instructions carefully and understand the format of the questions. This will help you navigate through the exam efficiently. Additionally, manage your time wisely by allocating appropriate periods for each section, ensuring that no question is left unanswered due to time constraints.

Finally, review your work if time permits. Often, a second look can help spot small mistakes that may have been overlooked during the first attempt. Even if you’re unsure about an answer, providing a reasonable attempt is better than leaving it blank.

Solving Linear Equations with Ease

Mastering the art of solving linear equations is a crucial skill in mathematics. By following a clear, systematic approach, even the most complex equations can be solved quickly and accurately. The key is to isolate the variable and simplify the equation step by step until the solution becomes clear.

Start by eliminating any constants or terms that do not involve the variable. Move all terms with the variable to one side and constants to the other. Then, use basic arithmetic operations, such as addition, subtraction, multiplication, and division, to simplify the equation further. With consistent practice, this process will become more intuitive.

Remember, patience and precision are essential. Take your time to work through each step carefully and double-check your calculations to ensure accuracy. Over time, you’ll find that solving linear equations becomes a straightforward and efficient task.

Graphing Techniques for Algebra 1

Graphing is a powerful tool that helps visualize relationships between variables. Understanding how to accurately plot points and interpret graphs allows you to better analyze and solve equations. By mastering key graphing techniques, you’ll gain the ability to quickly assess solutions and identify patterns.

• Plotting Points – Start by identifying the coordinates of each point, ensuring they are plotted accurately on the grid.
• Understanding the Slope – The slope represents the rate of change between two points on a graph. Learn to calculate the slope by determining the rise over the run.
• Drawing Lines – Once you have plotted the points and calculated the slope, draw a straight line through the points to represent the equation.
• Interpreting Intercepts – The point where the line crosses the vertical and horizontal axes is crucial. These intercepts provide important information about the equation.
• Using Graphing Calculators – Graphing calculators can be a helpful tool for quickly plotting more complex equations. Learn how to use them effectively to save time.

By consistently practicing these techniques, you will become more comfortable with graphing and develop a deeper understanding of how equations are represented visually.

Practice Test Solutions Explained

Understanding the process behind each solution is essential for reinforcing key concepts and improving problem-solving skills. Rather than simply reviewing answers, focusing on the steps that lead to the solution helps clarify your approach and strengthens your grasp of the material. This section breaks down the methodology used to solve common types of problems, providing a clear explanation of each step.

Step-by-Step Breakdown

For each problem, start by carefully identifying the given information and what is being asked. Organize the data logically, and choose the most efficient method to find the solution. Then, execute each step methodically. Check your work as you go to catch potential errors before moving forward.

Common Techniques and Strategies

Different problems may require different strategies. For instance, solving equations often involves isolating the variable, while word problems may require translating the scenario into mathematical expressions. Mastering these techniques will ensure you can approach a wide range of problems with confidence.

Important Formulas to Remember

Having a solid grasp of key mathematical formulas is essential for solving problems efficiently and accurately. These formulas serve as tools to simplify complex equations and enable quick calculations. Below are some of the most important formulas that are frequently used and should be memorized for success in mathematical tasks.

Formula	Explanation
Linear Equation: y = mx + b	This formula represents a straight line, where m is the slope and b is the y-intercept.
Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a	Used to solve quadratic equations in the form ax² + bx + c = 0.
Slope Formula: m = (y₂ – y₁) / (x₂ – x₁)	Determines the slope of a line given two points (x₁, y₁) and (x₂, y₂).
Pythagorean Theorem: a² + b² = c²	Relates the lengths of the sides of a right triangle, where c is the hypotenuse.
Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²)	Calculates the distance between two points in a coordinate plane.

By committing these essential formulas to memory, you will be able to quickly approach and solve a variety of mathematical problems, boosting both speed and accuracy.

Helpful Study Resources for Chapter 8

To master the material covered in this section, it is essential to utilize a variety of study tools and resources. Whether you prefer interactive activities, detailed explanations, or additional practice problems, there are plenty of options to enhance your learning experience. Below are some valuable resources that can help reinforce concepts and improve problem-solving skills.

Online Platforms and Tools

• Khan Academy – Offers video tutorials and practice exercises on key topics, providing step-by-step explanations for difficult concepts.
• Wolfram Alpha – A powerful tool for solving equations and checking your work. It also provides step-by-step solutions for many problems.
• Desmos – An interactive graphing tool that allows you to visualize equations and explore relationships between variables.

Printed Resources and Books

• Study Guides – Comprehensive study guides offer summary notes, example problems, and tips to better understand the material.
• Textbooks – Your textbook can be an invaluable resource for review. Revisit sections you find difficult and work through additional examples provided.
• Workbooks – Practice workbooks often include extra exercises that can help reinforce key concepts and improve retention.

Using a combination of these resources will allow you to approach the material from multiple angles, giving you a well-rounded understanding and boosting your confidence when solving problems.

How to Improve Your Scores

Improving your performance requires a strategic approach that blends consistent effort, focused practice, and effective study techniques. Whether you’re struggling with certain topics or simply aiming to boost your results, applying the right methods can make a significant difference. Here are some practical tips to help enhance your scores and build confidence.

• Focus on Weak Areas – Identify topics where you struggle the most and dedicate extra time to understanding them. Working through specific challenges will help you gain clarity and reduce mistakes.
• Practice Regularly – Consistent practice is key. The more problems you solve, the better you’ll understand the concepts and improve your problem-solving speed.
• Review Mistakes – After completing exercises or quizzes, carefully review your errors. Understand why you made each mistake and work on correcting it to prevent repeating it in the future.
• Stay Organized – Keep a study schedule and break down your work into manageable sections. Being organized helps you stay on track and reduces the chances of feeling overwhelmed.
• Ask for Help – Don’t hesitate to seek assistance when needed. Whether it’s a teacher, tutor, or study group, getting support can provide additional perspectives on difficult material.

By following these strategies, you’ll not only improve your understanding of the material but also build the skills needed to perform better in assessments. With perseverance and the right tools, you can see tangible improvements in your results.

Frequently Asked Questions About Algebra 1

As students work through various mathematical concepts, it’s common to encounter questions and uncertainties. This section addresses some of the most frequently asked questions related to foundational math courses. Here, we clarify key points that can help improve understanding and ease the learning process.

General Questions

• What are the most important concepts to focus on? Understanding operations with variables, solving linear equations, and mastering graphing techniques are fundamental. These concepts form the building blocks for more complex topics.
• How can I remember formulas more effectively? Practice is crucial. Create flashcards, use mnemonic devices, and repeatedly apply formulas to different problems to reinforce them in memory.
• Is it okay to ask for help when I don’t understand something? Absolutely. Seeking help from a teacher, tutor, or even classmates can provide valuable insights and offer different perspectives on challenging material.

Common Concerns

• How do I improve my problem-solving speed? Work through problems consistently and focus on recognizing patterns. Over time, you’ll become more efficient at solving them quickly.
• What should I do if I get stuck on a problem? Take a step back, break the problem down into smaller steps, and look for similar examples you’ve already worked through. If necessary, ask for clarification or additional help.
• How do I prepare for exams effectively? Start studying well in advance. Focus on reviewing key concepts, solve practice problems, and take mock exams to simulate the test environment.

By addressing these common concerns and questions, you can better navigate the material and approach the subject with confidence. Remember, persistence and consistent practice are key to mastering the concepts.

Tips for Memorizing Algebra 1 Concepts

Mastering mathematical ideas and formulas requires effective memorization techniques. It’s not just about remembering facts but understanding how and when to apply them. In this section, we will explore several strategies that can help you internalize key concepts and improve recall when solving problems.

Techniques for Memorization

• Practice Regularly: Consistent practice helps reinforce new concepts. The more you apply what you’ve learned, the more likely you are to retain the information.
• Create Visual Aids: Diagrams, charts, and color-coded notes can make abstract concepts easier to understand and remember.
• Break Down Complex Ideas: Divide complicated ideas into smaller, manageable parts. Focus on mastering one section before moving on to the next.
• Use Mnemonics: Develop mnemonic devices to remember key formulas or steps in problem-solving. Creating a catchy phrase can make recalling information easier.

Helpful Memorization Techniques

Method	How It Helps
Repetition	Constantly reviewing material helps solidify concepts in memory.
Chunking	Breaking information into smaller, related “chunks” makes it easier to absorb and retain.
Application	Applying concepts in various problems helps reinforce memory through practice.
Visualization	Creating mental images or diagrams allows you to connect abstract ideas with real-world examples.

By utilizing these memorization techniques, you can effectively internalize mathematical concepts and apply them with confidence. The key to success is persistence and consistent practice–these methods will gradually improve your ability to recall and utilize the material accurately.

Using Online Tools for Practice

In today’s digital age, a variety of online platforms offer an excellent opportunity to enhance your skills and reinforce your understanding of key concepts. These tools provide interactive exercises, step-by-step solutions, and immediate feedback, which can significantly improve your ability to solve problems efficiently and accurately. Incorporating online resources into your study routine can help you gain confidence and sharpen your problem-solving abilities.

Many websites and apps are designed to cater to different learning styles. Whether you prefer guided lessons, quizzes, or visual aids, you can find resources that match your needs. These tools not only offer practice problems but also allow you to track your progress and identify areas that need improvement. They can be an invaluable supplement to your traditional study methods.

Some platforms even allow for personalized learning, adjusting the difficulty of exercises based on your performance, ensuring you’re always working at the right level of challenge. By regularly using these online tools, you can reinforce your learning and boost your ability to tackle problems with ease.

Preparing for the Next Algebra Chapter

As you move forward in your studies, it’s essential to build a strong foundation that will support your learning in upcoming lessons. Understanding the concepts from the current section is crucial, but you must also begin to anticipate the material that will follow. By preparing ahead, you ensure that you’re not only reviewing past topics but also setting yourself up for success in the next set of challenges.

One of the best ways to get ready for the next section is to preview the upcoming topics. This might include familiarizing yourself with key terms, theorems, or types of problems that will be covered. Taking the time to look through the material briefly before it’s taught in class can give you a head start and make it easier to grasp the concepts when they are introduced.

Stay organized by keeping notes and reviewing them regularly. This will help you reinforce what you’ve learned while also making it easier to relate new material to what you already know. Consistent review will allow you to identify areas where you might need more focus, and actively engaging with the material will strengthen your understanding over time.

Reviewing Key Algebra 1 Topics

Revisiting fundamental concepts regularly is essential for mastering any subject, especially as the material becomes more complex. Focusing on core principles helps to solidify your understanding and improves your ability to tackle more advanced problems. By reviewing key topics frequently, you ensure that you retain vital information and develop a deeper understanding of how different concepts connect.

One effective strategy for reviewing is to break down the material into smaller sections, focusing on each individual concept. For example, start by revisiting basic operations with variables, then move on to more complex equations or functions. Reinforcing these topics consistently will build confidence and help you apply them with ease when faced with new problems.

Additionally, it’s important to understand the underlying logic behind each concept. This will help you not only to solve problems but also to explain your reasoning clearly. Review should involve both practice and comprehension, ensuring that you can both solve problems accurately and understand the processes behind the solutions.