Lesson 5.1 Practice B Geometry Answers

In this section, we explore the essential principles that help in solving complex problems involving shapes, angles, and their relationships. Understanding these foundational ideas is crucial for anyone looking to improve their problem-solving abilities in advanced mathematical topics.

By breaking down each problem into manageable steps, we aim to provide clear solutions that will guide you through the process of identifying patterns and applying formulas. Whether you’re working with basic figures or more intricate scenarios, this guide will help sharpen your skills and boost your confidence.

Strengthening your understanding of these concepts is the key to mastering more challenging exercises. With practice and the right approach, tackling similar problems will become increasingly intuitive. Success in these topics depends on your ability to connect theory with practical application.

Lesson 5.1 Geometry Practice B Overview

This section focuses on tackling fundamental problems related to shapes, angles, and their interrelations. By examining a series of exercises, you’ll strengthen your ability to analyze and solve complex mathematical challenges. The goal is to build a solid foundation in recognizing patterns and applying appropriate formulas to find solutions efficiently.

Throughout this guide, we break down each problem into smaller, manageable parts to make them easier to understand and solve. You’ll find that these problems range from basic concepts to more intricate scenarios, all of which aim to develop your critical thinking and analytical skills.

Topic	Key Focus	Goal
Shapes and Properties	Understanding various geometric figures	Identify key attributes and relationships
Angles	Measuring and calculating angles	Apply angle relationships in problems
Coordinate Systems	Working with grid-based problems	Learn how to plot and interpret coordinates
Proofs	Logical reasoning for geometric theorems	Develop reasoning skills for solving proofs

Understanding Key Concepts in Geometry

Grasping the fundamental principles behind spatial relationships is crucial when solving complex problems. These concepts form the backbone of more advanced topics, allowing individuals to approach various exercises with clarity and precision. Whether dealing with shapes, measurements, or theorems, understanding these core ideas will enable you to navigate through challenges with ease.

By breaking down concepts into their simplest forms, we aim to provide a clear path to mastering the skills needed for problem-solving. Recognizing key relationships between figures and applying the correct methods is essential for reaching the correct conclusions efficiently.

Concept	Definition	Application
Shapes and Properties	Basic characteristics of geometric figures	Understanding their attributes helps in problem-solving
Angle Relationships	How angles interact within figures	Used to solve problems involving intersections and parallel lines
Coordinate Systems	Grid-based plotting of points and shapes	Solving problems that require positioning and measurement
Proofs	Logical reasoning to establish truths	Validates solutions by demonstrating logical connections between facts

Step-by-Step Guide to Practice B

This guide is designed to help you navigate through a series of mathematical challenges, breaking them down into manageable steps. Each problem requires a careful approach, starting with understanding the given information, followed by applying the appropriate methods to find a solution. The process is structured to ensure clarity and improve problem-solving efficiency.

By following this step-by-step method, you will learn how to approach each scenario logically, ensuring that all necessary steps are taken to reach a correct and accurate conclusion. This systematic approach is essential in building confidence and mastering the necessary skills to tackle similar problems in the future.

Step	Description	Goal
Step 1	Read and Understand the Problem	Identify the given information and what needs to be found
Step 2	Draw a Diagram (if applicable)	Visualize the problem to make the relationships clearer
Step 3	Apply Relevant Formulas	Use appropriate formulas to calculate unknown values
Step 4	Perform Calculations	Carry out the necessary operations to find the solution
Step 5	Verify the Solution	Check your results to ensure accuracy and consistency

Common Mistakes to Avoid in Geometry

When working through spatial problems, it’s easy to make errors that can lead to incorrect solutions. These mistakes often arise from misinterpreting given information, applying formulas incorrectly, or overlooking key details in the problem. Being aware of these common pitfalls can help you avoid them and improve your accuracy in solving similar challenges.

One of the most frequent errors is failing to carefully read the problem and missing crucial information. Skipping steps or making assumptions without proper verification can result in mistakes that affect the entire solution process. Additionally, misapplying formulas or neglecting to account for all variables can lead to incorrect calculations and conclusions.

Another common issue is neglecting to double-check the final solution. While it’s easy to assume that the first answer is correct, verifying your work is essential for ensuring accuracy and consistency. Taking the time to review calculations and results will prevent unnecessary mistakes and improve your overall problem-solving skills.

How to Solve Geometry Problems Effectively

Solving spatial problems requires a structured approach to ensure accuracy and efficiency. The key to success is breaking down each task into smaller, manageable steps, allowing you to identify the essential components and relationships within the problem. By following a clear, logical process, you can avoid errors and reach the correct solution more confidently.

Start by carefully analyzing the problem to identify what is given and what needs to be determined. Drawing a diagram can often make the relationships between different elements clearer, especially in complex scenarios. Once you have a clear understanding, apply the appropriate formulas or methods, making sure to account for all relevant factors.

Additionally, it’s important to stay organized and methodical during each step. Rushing through calculations or skipping steps can lead to careless mistakes. Take the time to double-check your work and verify that your solution makes sense in the context of the problem. This approach will help you tackle even the most challenging exercises with greater ease.

Geometry Formulas Used in Lesson 5.1

In this section, several essential mathematical formulas are employed to solve a variety of problems related to shapes, angles, and their properties. These formulas are fundamental tools that help simplify complex problems and enable you to find unknown values with precision. Understanding when and how to apply these formulas is crucial for tackling various exercises effectively.

Each formula serves a specific purpose, whether it’s calculating the area of a polygon, the perimeter of a figure, or the relationship between angles. Some formulas are based on basic principles, while others involve more advanced concepts, such as trigonometry or coordinate systems. Knowing the correct formula to use in each situation is key to solving problems accurately and efficiently.

Here are some commonly used formulas in this context:

Formula	Application
Area = Length × Width	Used to calculate the area of rectangles and squares
Area = 1/2 × Base × Height	Used to find the area of triangles
Perimeter = 2 × (Length + Width)	Used to calculate the perimeter of rectangles
Angle Sum = 180° × (n – 2)	Used to find the sum of interior angles of polygons
Pythagorean Theorem: a² + b² = c²	Used to calculate the sides of right triangles

Tips for Mastering Geometry Practice B

Successfully mastering spatial problems requires both practice and understanding. With the right approach, you can strengthen your problem-solving skills and improve your ability to tackle increasingly complex tasks. The key is to remain focused on the fundamentals and gradually build on your knowledge as you progress.

Start by thoroughly reviewing the concepts related to each problem. Understanding the theory behind the calculations will help you apply the correct methods more effectively. It’s also helpful to break down each question into smaller steps, ensuring that you don’t overlook any critical details. This structured approach not only enhances your accuracy but also boosts your confidence.

Another important tip is to practice regularly. The more you work through similar problems, the more familiar and intuitive the process becomes. Don’t hesitate to revisit problems you’ve already solved to identify areas where you can improve. Finally, don’t forget to double-check your work before finalizing your answers–accuracy is key in these exercises.

Visualizing Geometry Problems for Better Understanding

One of the most effective ways to solve complex spatial problems is by visualizing the situation. Drawing diagrams and creating mental images can help clarify the relationships between various elements, making it easier to understand the problem and apply the correct methods. Visualization not only simplifies abstract concepts but also improves accuracy by making key details more apparent.

When tackling these types of problems, try following these steps to enhance your understanding:

• Sketch the Problem: Always start by drawing a clear diagram based on the information given. This will help you see the problem from a different perspective and highlight relationships between different components.
• Label Key Information: Mark important points, angles, and lengths on your diagram to make it easier to reference as you work through the problem.
• Look for Symmetry: Identifying symmetrical shapes or patterns can often reveal helpful insights that simplify the problem-solving process.
• Break Down the Diagram: If the problem involves multiple steps, divide the diagram into smaller sections, focusing on one part at a time to avoid confusion.

By practicing these visualization techniques, you can build a deeper understanding of spatial relationships, making it easier to approach and solve even the most challenging exercises. The clearer your mental image, the more intuitive the problem-solving process becomes.

Analyzing Angles and Their Relationships

Understanding angles and their interrelationships is crucial when solving spatial problems. Angles play a key role in determining the properties of shapes, and recognizing how they interact can simplify complex calculations. By learning how to classify and analyze different types of angles, you can more easily solve problems that involve lines, intersections, and polygons.

Types of Angles

There are several types of angles that you should be familiar with, each having distinct properties that influence how they interact with one another:

• Acute Angles: Angles that measure less than 90°.
• Right Angles: Angles that measure exactly 90°.
• Obtuse Angles: Angles that measure greater than 90° but less than 180°.
• Reflex Angles: Angles that measure greater than 180° but less than 360°.
• Straight Angles: Angles that measure exactly 180°.

Angle Relationships

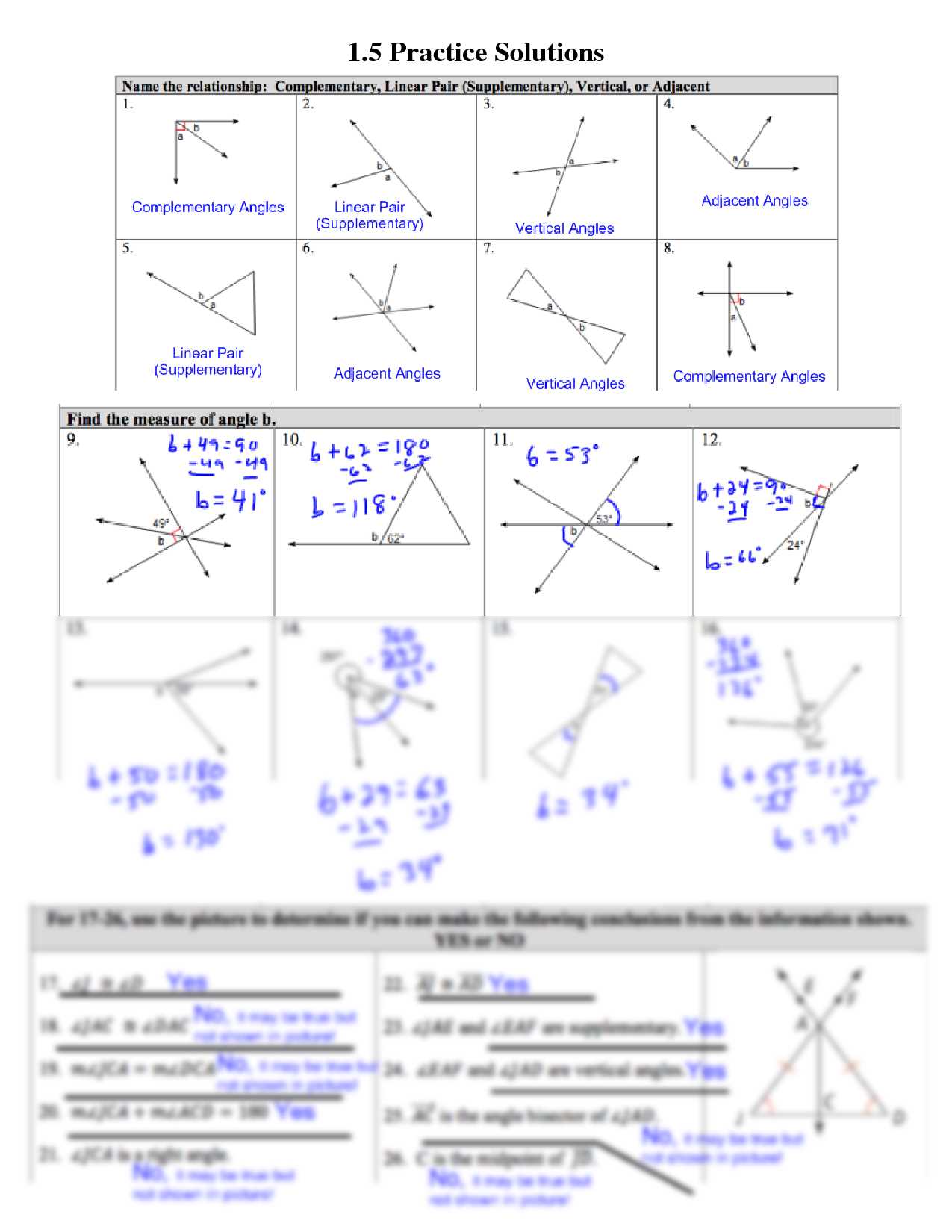
When angles are formed by intersecting lines or polygons, their relationships can be used to solve problems. Here are some common angle relationships to consider:

• Complementary Angles: Two angles that add up to 90°.
• Supplementary Angles: Two angles that add up to 180°.
• Vertical Angles: Angles that are opposite each other when two lines intersect, and they are always equal.
• Adjacent Angles: Angles that share a common vertex and side but do not overlap.

By recognizing these relationships, you can simplify your problem-solving process and apply the correct formulas to find missing values or check for consistency in your results. Analyzing the angles and understanding their properties is a vital skill when working through these types of exercises.

Using Coordinate Geometry in Lesson 5.1

Coordinate-based approaches allow you to solve spatial problems by focusing on points, lines, and shapes within a coordinate plane. This method uses a system where each location is represented by a pair of numerical values, typically referred to as coordinates. Understanding how to manipulate and interpret these coordinates is essential for solving problems that involve distance, slopes, and geometric properties of figures such as triangles and quadrilaterals.

To begin applying coordinate principles, start by plotting points on the Cartesian plane. The x- and y-axes intersect at the origin, giving a reference for all other points. Once points are plotted, you can calculate various properties, such as the distance between two points or the slope of a line connecting them. These calculations can provide valuable insights into the relationships between different geometric elements.

For example, to find the distance between two points (x₁, y₁) and (x₂, y₂), the distance formula is:

Distance Formula:

d = √((x₂ – x₁)² + (y₂ – y₁)²)

This formula helps calculate the straight-line distance between two points on the plane. Similarly, the slope of a line, which indicates its steepness, can be determined using the formula:

Slope Formula:

m = (y₂ – y₁) / (x₂ – x₁)

By mastering these coordinate geometry techniques, you can efficiently solve problems involving various geometric shapes and relationships, from basic lines and angles to more complex figures.

Solving Geometry Proofs in Practice B

Solving geometric proofs requires a logical approach, where you must show that certain properties or relationships hold true based on given information and established theorems. Proofs often involve a series of steps where you deduce one result after another, using both direct and indirect reasoning. These steps are usually supported by definitions, axioms, and previously proven theorems, ensuring that each conclusion follows logically from the preceding ones.

To effectively solve proofs, follow this structured approach:

1. Understand the Given Information: Carefully read the problem to determine what information is provided and what needs to be proven.
2. Identify Known Theorems: Recall relevant theorems and properties that can be used to establish relationships between the geometric figures involved in the problem.
3. Choose a Strategy: Decide on the best approach for the proof, whether it’s using direct reasoning, contradiction, or constructing auxiliary lines or shapes.
4. Write Step-by-Step: Begin with what is given, and logically move from one step to the next, using valid geometric principles to support each statement.
5. Conclude the Proof: Conclude by showing that the desired result has been reached, ensuring that each step is justified and follows from the previous one.

Throughout the process, pay attention to each assumption and step. Geometry proofs require precision and clarity, so ensure that every statement is backed by a valid reason or known property. With practice, this methodical approach will become second nature, allowing you to confidently solve even the most challenging geometric proofs.

Understanding Geometric Shapes and Properties

In the study of spatial relationships, it’s essential to grasp the fundamental properties of various shapes and how they interact within a given space. Geometric figures, ranging from simple triangles to complex polygons, have specific characteristics that define their structure. These properties not only determine how shapes behave but also how they can be measured and analyzed in different contexts, such as area, perimeter, angles, and symmetry.

Each geometric shape has unique features that distinguish it from others. For example, the sum of interior angles in a triangle is always 180°, while the opposite sides of a rectangle are equal in length. Understanding these properties allows for solving more complex problems and proofs that involve these figures. Below, we will explore some common shapes and their defining characteristics:

Triangles

Triangles are one of the most basic shapes in geometry, consisting of three sides and three angles. Their properties include:

• Sum of angles: The interior angles always add up to 180°.
• Types of triangles: Equilateral, isosceles, and scalene, each defined by the number of equal sides or angles.
• Area formula: A = 1/2 * base * height

Quadrilaterals

Quadrilaterals are four-sided polygons, and they include shapes like squares, rectangles, and parallelograms. Key properties include:

• Sum of angles: The sum of interior angles is always 360°.
• Specific properties: A square has four equal sides and four right angles, while a rectangle has opposite sides equal in length.
• Area formula: A = length * width (for rectangles) or A = base * height (for parallelograms).

These are just a few examples, but every geometric shape has a set of unique properties that help in solving mathematical problems and understanding spatial relationships. The key to mastering these concepts is practice, allowing for the development of a deep understanding of how these shapes interact with one another and how their properties can be applied in various scenarios.

Strategies for Tackling Geometry Word Problems

Word problems in the realm of spatial reasoning can often feel overwhelming, especially when they involve complex figures or require multiple steps to solve. However, by following a systematic approach, you can break down even the most challenging problems into manageable parts. The key is to understand the problem, identify the necessary information, and apply the right techniques to reach the solution efficiently.

To approach these problems effectively, consider the following strategies:

1. Read the Problem Carefully

Before diving into calculations or drawing diagrams, take time to read the problem carefully. Identify what is being asked and gather the information provided. Highlight important details like measurements, relationships, and any specific instructions about the figures involved.

2. Draw a Diagram

Whenever possible, visualize the problem by sketching a diagram. This helps in understanding the spatial relationships between different elements and can make it easier to apply relevant formulas or theorems. Label all given values clearly and add any missing information if needed.

3. Identify Key Relationships

Look for known relationships between elements within the problem. These may include:

• Angle properties: Such as complementary or supplementary angles.
• Symmetry: If the figure exhibits symmetry, it can simplify the problem.
• Perimeter and area formulas: Knowing how to calculate the perimeter or area of a shape can help in solving for unknown values.

4. Break the Problem into Smaller Steps

Rather than trying to solve the entire problem at once, break it down into smaller, more manageable steps. Solve for one unknown at a time and use each step to build upon the previous one. This method allows you to focus on one aspect of the problem at a time, reducing errors and confusion.

5. Check Your Work

After reaching a solution, take the time to check your work. Revisit each step and confirm that all calculations and logical reasoning are correct. If possible, substitute your solution back into the original problem to see if it makes sense in the context of the question.

By following these strategies, you can approach word problems with greater confidence and precision. Practice is key, and over time, these steps will become second nature, allowing you to solve even the most complex problems with ease.

Improving Your Geometry Problem-Solving Skills

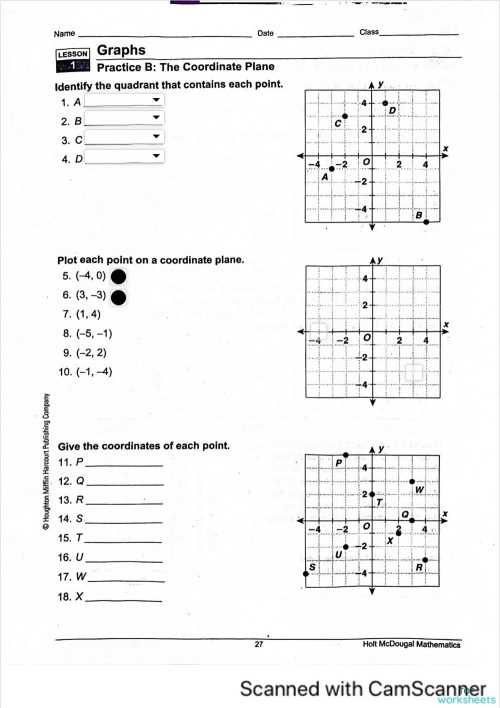
Enhancing your ability to solve spatial reasoning challenges requires both practice and a strategic approach. Understanding key concepts, developing strong visualization skills, and mastering problem-solving techniques are essential to becoming proficient in this area. Over time, with dedication and focus, you can improve your ability to analyze and solve complex problems with greater ease and accuracy.

To enhance your problem-solving skills, consider the following methods:

1. Practice Regularly

Consistent practice is crucial to building a strong foundation. The more problems you work through, the more familiar you will become with different techniques and strategies. Try solving problems of varying difficulty to challenge yourself and refine your skills.

2. Understand the Fundamentals

Before tackling more complex problems, ensure you have a solid understanding of basic concepts. This includes grasping key formulas, theorems, and properties of shapes. Building this foundation will allow you to apply these principles effectively in various scenarios.

3. Work on Visualizing Problems

Many spatial challenges can be solved by creating mental or physical representations of the problem. Drawing diagrams or sketches can help clarify relationships between elements and highlight key features of the problem. This technique is particularly helpful when dealing with complex shapes or angles.

4. Break Down Problems into Smaller Parts

Instead of attempting to solve a problem all at once, break it down into smaller, more manageable steps. Focus on solving for one unknown at a time and use intermediate solutions to guide you toward the final answer. This approach minimizes errors and increases your chances of success.

5. Learn from Mistakes

When you make an error, take the time to analyze where you went wrong. Understanding why a particular approach didn’t work will help you refine your technique and avoid similar mistakes in the future. Learning from your mistakes is an essential part of improving your problem-solving abilities.

6. Seek Additional Resources

If you’re struggling with certain topics or types of problems, don’t hesitate to seek additional resources. This could include textbooks, online tutorials, or discussion forums where you can ask questions and get advice from others. Accessing a variety of materials will deepen your understanding and provide new perspectives.

7. Stay Organized

Keeping your work organized is crucial, especially when dealing with multiple steps. Use clear, logical notation and label your diagrams accurately. This will help you stay on track and ensure that all necessary information is accounted for.

Strategy	Description
Practice Regularly	Consistently work through problems of varying difficulty to build familiarity and confidence.
Understand Fundamentals	Master basic formulas, theorems, and properties before moving on to more complex challenges.
Visualize Problems	Create diagrams or mental images to better understand spatial relationships and solve problems.
Break Problems Down	Divide complex problems into smaller steps for easier problem-solving.
Learn from Mistakes	Analyze errors to understand your missteps and refine your technique.

By implementing these strategies and consistently practicing, you will see marked improvement in your problem-solving abilities. As you gain more experience and insight, you’ll develop the confidence to tackle even the most difficult challenges with ease.

How to Check Your Geometry Answers

Reviewing your solutions is an essential step in the problem-solving process. Ensuring that your calculations, reasoning, and conclusions are accurate not only boosts confidence but also helps to identify and correct potential mistakes. There are several methods you can use to check the validity of your solutions and confirm that everything is correct.

1. Verify the Calculation Steps

Start by double-checking all of your mathematical operations. Revisit each step of the problem and ensure that all formulas, values, and operations have been applied correctly. Small arithmetic errors can often be the cause of an incorrect result.

• Recalculate each operation to verify the result.
• Check if the numbers you used make sense in the context of the problem.
• Ensure that you have applied the correct formulas for each step.

2. Review the Diagram

If the problem involves visual elements, reviewing the diagram is crucial. Ensure that you have accurately represented all the components and relationships between them. Check if your diagram matches the problem’s description and that the labels are clear.

• Confirm that all angles, lines, and shapes are correctly drawn.
• Ensure that all dimensions and labels are accurately placed.
• Check if you have included all the necessary information from the problem statement in the diagram.

3. Cross-Check with Known Theorems

It is helpful to cross-check your results with established geometric theorems or principles. If your solution is consistent with known rules, this serves as an additional confirmation of its correctness.

• Ensure that your solution aligns with the appropriate theorems or postulates.
• Use geometric properties, such as congruence or similarity, to validate your results.

4. Test with a Different Approach

If you’re unsure about your answer, try solving the problem using a different method. A different approach may provide clarity and further confirm your solution’s accuracy.

• Look for alternative strategies that could lead to the same result.
• Check if the solution remains consistent across different methods of solving.

5. Ask for a Second Opinion

If you’re still uncertain, ask a peer, teacher, or tutor to review your work. A fresh set of eyes may spot errors that you might have missed.

• Present your reasoning and steps to someone else for their feedback.
• Explain your solution to ensure you understand it thoroughly.

By following these strategies, you can significantly reduce the chance of making errors and ensure that your solutions are correct. Thoroughly checking your work not only helps you avoid mistakes but also deepens your understanding of the concepts and strengthens your problem-solving skills.

Geometry Practice B Review and Summary

This section provides a comprehensive review of the key concepts and problem-solving techniques encountered in the exercises. By summarizing the methods and strategies used to approach the tasks, learners can solidify their understanding and build confidence in their skills. Reviewing the process not only helps in identifying common pitfalls but also reinforces the application of mathematical principles to solve complex problems.

Key Concepts Covered

Throughout the exercises, several core concepts were addressed. These include working with various shapes, understanding spatial relationships, and applying formulas to calculate properties such as area, perimeter, and angles. Each problem was designed to emphasize logical reasoning and the step-by-step process of deriving correct results.

• Recognizing the properties of different polygons and shapes.
• Using algebraic expressions to solve for unknown values in geometric problems.
• Understanding angle relationships and their significance in solving for other unknowns.

Problem-Solving Strategies

Effective problem-solving in this section relied on breaking down complex problems into manageable steps. By drawing clear diagrams, identifying known and unknown quantities, and applying the right formulas, learners were able to approach each problem systematically. Testing multiple methods of solving helped ensure the accuracy of the solutions.

• Start with understanding the problem and identifying key information.
• Use diagrams to visually represent the problem and relationships between elements.
• Choose the correct mathematical tools (formulas, theorems) and apply them carefully.

In summary, this section reinforced the importance of attention to detail and systematic thinking in solving geometric problems. By reviewing the approach to each exercise and understanding the logic behind each solution, learners can improve their problem-solving abilities and tackle more advanced topics with greater ease.