Princeton Review AP Calculus AB Practice Test 1 Answers

Preparing for a challenging mathematics exam requires more than just understanding theoretical concepts. It involves honing problem-solving skills, practicing time management, and reviewing detailed explanations to reinforce your knowledge. This guide aims to provide you with the necessary tools to navigate through complex questions and evaluate your understanding effectively.

Familiarizing yourself with different problem types is essential to achieving success. By working through practice materials and analyzing their solutions, you can identify common pitfalls and learn how to approach each question methodically. This process will help you develop a deeper understanding and increase your confidence as you prepare for the exam.

In this guide, you will find a comprehensive breakdown of various mathematical challenges, along with insightful strategies for addressing them. By carefully reviewing each solution and learning from your mistakes, you will be well-equipped to excel in the upcoming examination.

Princeton Review AP Calculus AB Practice Test 1 Answers

When preparing for an advanced mathematics exam, it is crucial to engage with a variety of problems and learn the reasoning behind each solution. By reviewing detailed explanations, you can enhance your understanding of key concepts and sharpen your ability to solve similar questions under exam conditions.

How to Approach Problem Solutions

Each problem presents a unique challenge, requiring you to apply different mathematical techniques. To improve your skills, focus on understanding the steps involved in reaching the correct solution. Here’s how you can maximize your study sessions:

• Step-by-step analysis: Break down each question into smaller parts and follow the logical flow from start to finish.
• Identify common patterns: Look for recurring problem types and the methods typically used to solve them.
• Review mistakes: Carefully analyze errors to avoid repeating them in the future and understand the reasoning behind the correct approach.

Key Strategies for Effective Studying

Focusing on improving specific areas can make a significant difference in your performance. Consider the following strategies to boost your preparation:

1. Time management: Set aside dedicated time for each section, ensuring you cover all topics without rushing.
2. Target weak areas: Spend extra time on concepts that you find more challenging to strengthen your overall understanding.
3. Practice regularly: Consistent exposure to similar problems will help reinforce your skills and increase familiarity with the material.

Overview of the Practice Test

This section provides a detailed introduction to a set of sample problems designed to simulate the real exam experience. By engaging with these materials, you can better understand the format and structure of the questions, giving you insight into what to expect on the actual assessment. The problems cover a wide range of topics and allow for comprehensive review.

Types of Questions Included

The set of questions encompasses various mathematical concepts and skills that are essential for success. You will encounter both theoretical and practical problems that test your ability to apply knowledge effectively in different contexts. The goal is to evaluate your understanding and problem-solving capabilities across multiple areas.

Structure and Time Constraints

Each section is designed to replicate the time constraints and pressure of the real exam. By practicing under these conditions, you can develop better time management skills and ensure that you can efficiently tackle each problem within the allotted time.

Key Topics Covered in the Test

The set of problems focuses on essential mathematical concepts that are fundamental to succeeding in the exam. These topics are carefully selected to test a wide range of skills, from basic theory to advanced problem-solving techniques. Understanding these areas will help you build a solid foundation for tackling the full range of challenges you may encounter.

Core Areas of Focus

The following subjects are central to the evaluation, and each section includes a variety of problems designed to assess your grasp of the material:

• Functions and Graphs: Understanding the behavior of different types of functions and their graphical representations.
• Limits and Continuity: Exploring the concept of limits and how they relate to the continuity of functions.
• Derivatives: Applying rules for differentiation and understanding how derivatives describe rates of change.
• Integration: Mastering techniques for finding areas under curves and solving problems involving accumulation.
• Applications of Derivatives and Integrals: Using differentiation and integration to solve real-world problems.

Problem Types and Techniques

In addition to the core topics, you will encounter various problem types that test specific mathematical methods and techniques:

1. Solving equations: Developing strategies for solving both algebraic and differential equations.
2. Optimization problems: Identifying maximum and minimum values of functions in different scenarios.
3. Area and volume calculations: Using integration to find areas between curves and volumes of rotated solids.

How to Use the Answer Key Effectively

Utilizing the solution guide properly is an essential part of your preparation process. It goes beyond simply checking if your answers are correct–it offers an opportunity to gain a deeper understanding of the methods and reasoning behind each solution. By reviewing the steps taken to reach the correct answer, you can identify areas where you may have gone wrong and learn more efficient strategies for solving similar problems.

Start by reviewing your mistakes: Instead of just focusing on correct responses, examine any errors you made. Understanding why your solution didn’t work is just as important as knowing how to arrive at the right one. Ask yourself questions such as, “What concept did I misunderstand?” or “Where did I make an arithmetic mistake?” This helps you pinpoint gaps in your knowledge and avoid repeating the same mistakes.

Analyze the step-by-step solutions: Don’t just accept the final answer; work through each step shown in the solution guide. This will reinforce your understanding of the processes involved. If you don’t understand a particular step, review the underlying concepts and attempt similar problems to solidify your grasp.

Apply the feedback to future questions: Once you’ve understood the solution process, go back to similar questions and apply the insights you’ve gained. This helps you practice more effectively and builds your confidence in solving future problems.

Step-by-Step Solutions for Each Question

Breaking down each problem into manageable steps is crucial for mastering any mathematical concept. By following a structured approach, you can understand not just the correct answer but the reasoning behind it. This section provides detailed explanations of the processes involved in solving each question, allowing you to see how to approach similar problems effectively.

How to Approach Complex Problems

Understanding each phase of the solution is key to grasping the overall concept. Here’s a general approach you can follow for each question:

• Read the problem carefully: Take note of important details, such as given information and what is being asked.
• Identify the correct method: Decide which mathematical concept or technique you need to apply based on the problem type.
• Break it into steps: Solve the problem incrementally, checking each step for accuracy.
• Review the solution: Ensure that your final answer makes sense in the context of the question.

Example Breakdown

Here’s an example of how you can approach a typical problem step-by-step:

Step	Description	Mathematical Operation
1	Read the problem and identify key information.	Extract values and parameters from the question.
2	Choose the appropriate method for solving.	Decide whether to use derivatives, integrals, or other tools.
3	Perform calculations step-by-step.	Follow the correct mathematical procedures.
4	Check your solution and re-evaluate if necessary.	Ensure the answer is logical and consistent with the problem.

Common Mistakes and How to Avoid Them

During any challenging mathematical evaluation, it’s easy to fall into common traps that can lead to incorrect solutions. These errors often arise from misunderstanding key concepts or overlooking important details. Recognizing these mistakes in advance can help you avoid them and improve your performance. This section highlights some frequent pitfalls and provides strategies to prevent them.

Frequent Errors and Their Causes

Below are several common mistakes that students tend to make, along with their underlying causes:

• Misinterpreting the question: Failing to fully understand what is being asked can lead to unnecessary calculations or incorrect methods. Always read the problem carefully and identify the key information.
• Forgetting to apply rules correctly: Many students skip steps in formulas or apply rules incorrectly, such as differentiating or integrating improperly. Be sure to follow each rule step by step to ensure accuracy.
• Skipping units or signs: In many cases, errors occur when students forget to include units or signs in their final answers. Always check that you’ve accounted for these elements throughout your work.
• Rushing through the solution: In an effort to finish quickly, students often make careless mistakes, such as arithmetic errors or sign mistakes. It’s important to pace yourself and double-check your work.

How to Avoid These Mistakes

There are several strategies you can implement to minimize errors during your study sessions:

• Take your time: Don’t rush through problems. Carefully read each question and think through the steps before jumping into calculations.
• Review each step: After completing a problem, revisit each step to ensure it’s correct and follows the logical progression.
• Use process of elimination: If you’re unsure about your answer, check your work and rule out impossible options or approaches.
• Practice regularly: The more you practice, the more familiar you will become with common problem types and how to avoid mistakes.

Understanding the AP Calculus Exam Format

The structure of the exam plays a significant role in how you should approach your preparation. Understanding the organization and timing of each section will help you plan your strategy and ensure that you manage your time efficiently during the actual assessment. This section provides an overview of the format, helping you become familiar with the types of questions and how they are presented.

Two main sections: The exam is typically divided into two primary parts: one that tests your conceptual understanding and another that focuses on application and problem-solving. Each section is designed to evaluate different aspects of your mathematical knowledge and skills.

Multiple-Choice Questions

This section consists of a series of questions where you will be asked to select the correct answer from several options. The multiple-choice format is designed to assess your ability to recognize key concepts and apply techniques quickly.

• Time Management: You’ll need to balance speed and accuracy as you move through these questions.
• Strategy: Focus on eliminating incorrect answers before making your final choice.
• Topics Covered: Expect questions on limits, derivatives, integrals, and their applications.

Free-Response Questions

This section presents a series of open-ended questions that require detailed solutions. You must show all your work and explain your reasoning for arriving at the answer. This is where you can demonstrate a deeper understanding of the material.

• Solution Process: Clearly lay out each step, explaining your approach to the problem.
• Show Work: Make sure to include all necessary calculations and reasoning for partial credit.
• Time Allocation: Allocate time wisely, ensuring you have enough time to complete all parts of the free-response questions.

Time Management Tips for AP Calculus

Efficiently managing your time during the exam is crucial to ensure that you can complete all sections without feeling rushed. Proper time allocation allows you to focus on each question thoroughly, reducing the likelihood of careless mistakes and ensuring that you have ample time to double-check your work. This section provides key strategies for pacing yourself effectively on the day of the assessment.

Before the Exam

Preparation starts long before the exam day. Building strong time management habits during your study sessions will pay off when you’re sitting for the actual exam.

• Simulate Exam Conditions: Regularly practice under timed conditions. Use practice problems or previous assessments to familiarize yourself with the timing constraints.
• Break Down the Material: Allocate specific time slots for each topic, ensuring that you spend enough time on each area without neglecting others.
• Prioritize Weak Areas: Focus more time on challenging topics or areas where you feel less confident, but avoid spending too much time on material you already know well.

During the Exam

On exam day, maintaining control over your time is essential to avoid feeling overwhelmed. Here’s how to allocate your time efficiently during each section:

• Read the Instructions Carefully: Spend the first few minutes reading the instructions and each question carefully. This will prevent misunderstandings that could cost you time later.
• Multiple-Choice Section: Allocate a set amount of time per question (usually around 1 minute). If you’re unsure about an answer, move on and return to it later.
• Free-Response Section: Budget your time so that you can complete each part of the problem. Ensure that you leave a few minutes at the end to review your answers.
• Don’t Overthink: If a problem is taking too long, skip it and return to it after you’ve completed easier questions. Overthinking can waste valuable time.

Time Management Strategies

Effective strategies will help you stay on track and complete the exam without unnecessary stress.

• Time Check: Periodically glance at the clock to make sure you’re on pace. Adjust your speed if necessary.
• Stay Calm: Don’t panic if you hit a tough question. Take a deep breath, move on to another problem, and return to it later.
• Practice Pacing: In your study sessions, practice pacing yourself so you get comfortable with the time constraints.

Improving Problem-Solving Skills for Calculus

Developing strong problem-solving skills is essential for mastering mathematical challenges. The ability to analyze a problem, identify the correct approach, and apply the necessary techniques can make a significant difference in your performance. This section focuses on strategies to enhance your problem-solving abilities, ensuring that you are prepared for even the most complex questions.

One key to success is understanding the fundamental concepts before attempting complex problems. Without a strong grasp of the basics, it’s difficult to apply advanced techniques effectively. Start by revisiting core principles and ensuring that you have a solid foundation in them.

Step-by-Step Approach: Break down each problem into manageable parts. This will help you avoid feeling overwhelmed and allow you to focus on one piece at a time. Here’s how you can approach a problem step by step:

• Identify the Problem: Understand what the question is asking. Look for key terms and information that will guide you.
• Choose the Right Strategy: Decide which mathematical principles or techniques are needed to solve the problem.
• Execute the Solution: Carry out the necessary calculations or steps, ensuring you follow the correct order of operations.
• Double-Check Your Work: After reaching a solution, review your steps and calculations to verify accuracy.

Another useful technique is practicing a variety of problems regularly. By tackling different types of questions, you’ll gain experience with a wider range of techniques, which will help you become more adaptable when facing unfamiliar problems. Don’t be afraid to revisit problems that you found difficult. Each time you attempt a problem, you’ll become better at identifying patterns and solutions more efficiently.

Seek Alternative Solutions: Whenever you solve a problem, try to approach it from different angles. This not only enhances your understanding but also increases your confidence in handling diverse problems.

Detailed Explanations for Complex Problems

Understanding complex mathematical problems requires a clear, step-by-step breakdown of the solution process. The ability to dissect each part of a problem and explain how to approach it systematically is essential for mastering challenging topics. This section provides in-depth explanations for solving difficult problems, focusing on breaking them down into simpler, more manageable components.

Breaking Down the Problem

When faced with a complex question, the first step is to carefully analyze the problem. Start by identifying the key elements and understanding what the question is asking. Focus on isolating the variables and recognizing patterns that can guide you toward the right method.

• Read the Problem Thoroughly: Ensure that you fully understand the question before attempting to solve it. Pay attention to important details and any conditions given in the problem.
• Isolate Key Information: Identify the critical variables or expressions that are crucial for finding the solution.
• Visualize the Problem: If applicable, sketch a diagram or graph to represent the situation. This can often make abstract concepts more concrete.

Step-by-Step Approach to Complex Problems

Once you’ve broken the problem into smaller parts, follow a structured approach to solve it. By taking it one step at a time, you reduce the risk of making mistakes and improve your understanding of the underlying concepts.

• Choose the Right Method: Decide which mathematical method or formula is best suited to solving the problem. This may involve choosing between different techniques such as integration, differentiation, or algebraic manipulation.
• Apply the Method: Carefully apply the selected technique, ensuring that you follow the correct order of operations and that each step is logical.
• Review Your Work: After solving the problem, go over your solution to verify that all steps were carried out correctly and that the final answer makes sense in the context of the problem.

Remember, complex problems often require more than one approach or perspective. If one method doesn’t work or feels cumbersome, it’s helpful to revisit the problem with a different strategy. Regular practice with complex problems will improve your problem-solving abilities and boost your confidence in handling challenging tasks.

How to Review Your Answers Efficiently

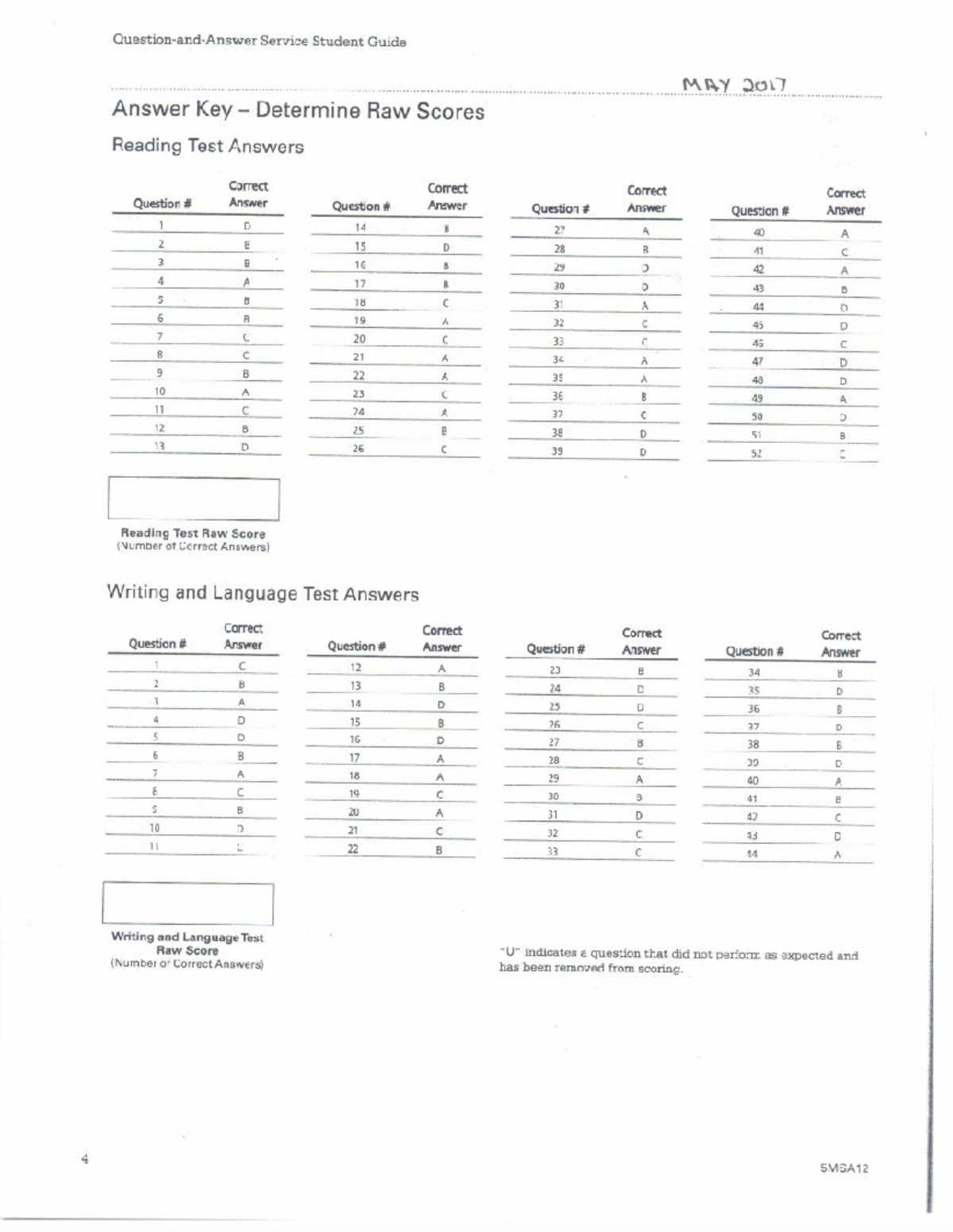
After completing any set of problems, it’s crucial to take the time to carefully assess your work. Reviewing your solutions not only helps identify mistakes but also reinforces your understanding of the material. An efficient review process ensures that you can catch errors early and learn from them, ultimately improving your performance in future challenges.

Organize Your Review Process

To make the most of your review, it’s important to approach it systematically. Rather than rushing through the review, focus on the steps and techniques used to arrive at each solution. Here’s how you can organize your review:

• Check for Accuracy: Start by confirming the calculations in each solution. Make sure that every step follows logically from the previous one, and double-check any arithmetic or algebraic manipulations.
• Identify Common Errors: Look for recurring mistakes, such as misinterpreting the problem, using the wrong method, or making simple arithmetic errors.
• Compare with Solution Key: If available, compare your solutions with a reliable answer guide. This can help identify areas where you may have misunderstood the approach or where more practice is needed.

Focus on Understanding, Not Just Correctness

It’s important to not just check if your answers are right, but to understand why certain methods or approaches work. By reflecting on each solution, you can strengthen your problem-solving skills for the future.

• Revisit Difficult Problems: If you find problems that you struggled with or got wrong, spend extra time understanding the correct approach. Try to identify what went wrong and how to avoid similar mistakes next time.
• Clarify Misunderstandings: For any concepts that were unclear during the process, take the time to revisit the theory behind them. This will ensure that you fully understand the method and can apply it correctly in the future.
• Review Overall Strategy: Take a step back and evaluate your overall strategy. Were there more efficient methods you could have used? Reflecting on your approach can improve your problem-solving skills over time.

By incorporating these review strategies, you not only identify errors but also build a deeper understanding of the material, which will better prepare you for similar challenges in the future.

Practice Test Scoring Guidelines

Understanding how to score your performance on a set of exercises is a crucial step in evaluating your progress and pinpointing areas for improvement. Scoring not only helps gauge how well you have mastered specific concepts but also offers insights into your problem-solving skills and timing under pressure. By following a clear set of scoring rules, you can accurately assess your strengths and weaknesses, and develop a strategy to further enhance your abilities.

How to Score Your Performance

When assessing your results, it’s important to have a clear understanding of the scoring criteria. Each problem should be evaluated based on the steps you took to reach the solution, as well as the final answer itself. Here’s a breakdown of how to score effectively:

• Accuracy of Solutions: Ensure that the final answer matches the expected result. Points are typically awarded for correctly solving the problem, while partial credit may be given for correctly applying a method, even if the final answer is incorrect.
• Method and Approach: The approach you take to solve a problem is just as important as the answer itself. Points are often awarded for using the appropriate methods and showing the necessary steps. This can include setting up equations, applying formulas, and solving them step-by-step.
• Logical Flow: Ensure that the steps follow a logical sequence. Mistakes often happen when the order of operations or reasoning is incorrect, so consistency and clarity are key to scoring well.

How to Calculate Your Total Score

Once you’ve reviewed each problem, it’s time to calculate your total score. Different types of exercises may carry different point values, so make sure to tally up the points according to the provided rubric. Here’s a simple way to track your score:

• Assign Points: Based on the solution’s accuracy, award full points or partial credit where applicable. Some exercises may offer additional points for providing extra explanations or justification for the solution.
• Check the Rubric: Refer to the provided rubric or guidelines to see if any points are awarded for specific steps or methods used in the solution. This will help ensure that you’re scoring each item appropriately.
• Calculate the Total: Add up all points for each problem to get your final score. This will give you an overall view of how well you performed on the exercises.

By following these guidelines, you can efficiently score your work, identify areas that need further attention, and improve your overall performance in future challenges.

Strategies for Mastering AP Calculus AB

Mastering a challenging subject requires more than just understanding the concepts. It involves developing effective study habits, practicing consistently, and learning to approach problems strategically. By applying the right techniques, students can not only improve their knowledge but also their ability to perform under timed conditions. Below are several strategies designed to help you succeed and gain confidence in tackling advanced mathematical problems.

Key Strategies for Success

To truly excel in this subject, focus on the following key strategies:

• Active Learning: Engage with the material through problem-solving rather than passively reading through notes. The more you actively apply the concepts, the more familiar you will become with the techniques and the faster you can recall them during exams.
• Regular Practice: Consistent practice is crucial. Solve problems daily to reinforce your understanding and strengthen your problem-solving skills. Diversify the types of problems you work on to cover all possible topics.
• Conceptual Understanding: Rather than memorizing formulas and procedures, focus on understanding the underlying principles. This deeper comprehension will allow you to approach unfamiliar problems with confidence.
• Time Management: Practice solving problems within a set time limit. This will help you get accustomed to the pace of the exam and ensure you have enough time to complete each section.
• Use of Resources: Leverage available resources such as textbooks, online tutorials, and study groups. Collaborating with peers can provide new insights into complex concepts.

Effective Problem-Solving Techniques

Mastering problem-solving requires strategic approaches that help you tackle various types of questions. The following techniques can guide you through the most complex problems:

Technique	Description
Break Down Complex Problems	Start by dividing the problem into smaller, more manageable steps. This will make it easier to understand the structure and how to approach each part.
Identify Patterns	Recognize recurring patterns or methods in similar problems. Once you identify these, you can apply known strategies to new problems more efficiently.
Work Backwards	In some cases, working backwards from the final goal can help you understand how to reach the correct solution. This is particularly useful for problems involving optimization or rates of change.
Visualize Problems	Draw diagrams or graphs to help conceptualize the problem. A visual representation can make it easier to see relationships between variables and understand the problem’s structure.

By incorporating these strategies and techniques into your study routine, you can strengthen your problem-solving abilities and build the skills needed to succeed on exams. The key to mastery is consistent effort, focused practice, and an understanding of the core principles.

Using Resources for Success

In any subject, access to reliable study materials can significantly enhance your learning experience. When preparing for a challenging exam, it’s essential to use resources that not only cover the core content but also provide strategies for approaching complex questions. The right materials can help you improve both your understanding and performance. Below are some of the key resources that can support your study efforts and contribute to your success.

Essential Resources for Effective Learning

To ensure you are fully prepared, consider incorporating the following resources into your study plan:

• Comprehensive Study Guides: Detailed guides offer in-depth explanations and cover all major topics. These can provide a solid foundation and allow for self-paced learning at your own convenience.
• Practice Questions and Drills: Regularly working through a variety of practice problems helps to solidify your knowledge. This resource is vital for mastering different types of questions and becoming comfortable with the format of the exam.
• Online Video Tutorials: Visual aids and video explanations break down complex topics into manageable steps. These resources are especially helpful for visual learners and can reinforce concepts in ways that text alone cannot.
• Flashcards and Concept Review: Flashcards are excellent for quick revision of key concepts, formulas, and important techniques. Regular review of these can help you retain crucial information for the long term.
• Study Groups and Forums: Collaborating with peers provides opportunities to exchange ideas, clarify doubts, and learn new methods of approaching problems. Online forums and discussion groups can also offer support and motivation.

How to Maximize the Use of These Resources

Simply having access to study materials is not enough. To maximize the effectiveness of your resources, use the following strategies:

Strategy	Description
Set Specific Goals	Before diving into study materials, set clear goals for what you want to accomplish. This helps maintain focus and ensures that each resource is used with a specific purpose in mind.
Mix Different Types of Resources	Variety is key to reinforcing learning. Combine written guides, practice questions, videos, and group discussions to cover topics from multiple angles and prevent boredom.
Track Progress	Regularly evaluate your progress by taking practice exams or quizzes. Tracking your improvement will help you identify areas where further study is needed and boost your confidence.
Use Resources Consistently	Consistency is essential for mastering the material. Make a study schedule and stick to it, ensuring that you use a variety of resources throughout your preparation period.

By utilizing a combination of these resources and following a disciplined study routine, you can increase your chances of success. The key is not only having access to high-quality materials but also using them strategically to maximize their impact.

Preparing for the AP Exam

Proper preparation for an advanced placement exam is essential for achieving the best possible score. Effective study habits, understanding the format, and practicing key concepts are crucial steps in the journey to success. This section will explore the strategies you can use to make your preparation as efficient and productive as possible. Whether you’re starting early or intensifying your review in the final weeks, a structured approach can lead to better results.

Creating a Study Plan

The first step in your preparation is to develop a comprehensive study plan. This plan should prioritize the areas that need the most attention while ensuring that you cover all relevant material. Follow these guidelines to structure your study time:

• Set Clear Goals: Identify what you need to master and break your study sessions into manageable chunks. Focus on understanding key concepts and avoid cramming.
• Allocate Time Wisely: Be realistic about how much time you can devote each day. Allocate more time to challenging topics while maintaining regular review sessions for material you have already mastered.
• Incorporate Different Resources: Use a mix of textbooks, online tutorials, and practice questions to reinforce your learning. Different resources can offer new perspectives and help solidify your understanding.
• Schedule Breaks: Avoid burnout by including breaks in your study plan. These will help you stay refreshed and focused during your study sessions.

Key Topics to Focus On

It’s important to focus on the major topics that are likely to appear on the exam. Prioritize these areas to ensure that you’re fully prepared:

• Limits and Continuity: Understanding the behavior of functions as they approach certain points is a foundational skill that appears in many questions.
• Derivatives: Mastering how to compute derivatives and apply them to real-world problems is essential.
• Integrals: Focus on understanding both the fundamental theorem of calculus and the various techniques for solving integrals.
• Applications of Derivatives and Integrals: Practice using derivatives and integrals to solve optimization problems, area under curves, and other practical scenarios.

Practice and Simulate Exam Conditions

In addition to studying the material, it is essential to practice under exam-like conditions. Time yourself while working through practice problems, and try to simulate the actual testing environment. This will help you become familiar with the pacing of the exam and build confidence in your ability to manage your time effectively.

By creating a structured study plan, focusing on essential topics, and practicing regularly, you will improve your chances of success and be better prepared for the challenges ahead. Consistency and dedication are key to mastering the material and performing well on exam day.

Benefits of Practicing with Test Solutions

Engaging with the solutions to previous questions is one of the most effective ways to deepen your understanding of key concepts and improve problem-solving skills. Reviewing completed questions allows you to identify mistakes, reinforce your knowledge, and better prepare for upcoming challenges. This section will explore the various advantages of working through solutions and how it can contribute to a more successful study experience.

Understanding Mistakes and Correcting Them

When you review a problem after attempting it, you gain valuable insight into areas where you may have misunderstood a concept or made an error. This process of reflection allows you to:

• Identify Common Misunderstandings: Often, errors arise from minor misunderstandings that can be corrected through careful review. Recognizing these patterns helps you avoid repeating the same mistakes.
• Improve Accuracy: Practicing with solutions ensures that you learn the correct methods and techniques, which can help you perform more accurately during the actual exam.
• Strengthen Conceptual Understanding: By seeing the correct steps and explanations, you can solidify your grasp of the underlying principles, enhancing your overall comprehension of the material.

Building Confidence and Speed

Familiarity with question formats and types is crucial for exam success. Working through past problems allows you to:

• Increase Speed: As you practice and review, you’ll become faster at identifying key elements in questions and applying the appropriate solutions. This helps you manage time effectively during the actual exam.
• Boost Confidence: The more problems you solve and understand, the more confident you’ll feel about your ability to tackle new challenges. This sense of preparedness can significantly reduce exam-day anxiety.
• Become Familiar with Question Patterns: Many exams have recurring formats. Reviewing solutions gives you a sense of what to expect and how to approach similar problems in the future.

By consistently practicing with solutions, you build a solid foundation of knowledge, identify potential pitfalls, and improve both your speed and confidence. This method of study is essential for achieving optimal performance in any exam scenario.

Maximizing Your Score with Targeted Practice

Focusing your efforts on specific areas where improvement is needed can significantly enhance your performance on any assessment. By identifying your weaknesses and dedicating time to practicing those areas, you can optimize your study sessions and ensure more effective preparation. This approach allows you to work smarter, not harder, and maximize your results in a shorter period of time.

Identifying Weak Areas

Before diving into focused exercises, it’s essential to assess your current knowledge and pinpoint where you’re struggling. This targeted approach involves:

• Reviewing Incorrect Responses: Analyzing past mistakes gives you a clear understanding of which concepts need more attention.
• Taking Diagnostic Quizzes: These quizzes help you identify patterns in your errors and focus on particular sections that need improvement.
• Seeking Feedback: Ask instructors, peers, or online resources to clarify any doubts or gaps in your understanding.

Effective Focused Practice

Once you’ve identified your weaker areas, it’s time to concentrate on improving those skills. The key to maximizing your performance lies in:

• Targeted Drills: Focus on specific types of problems that challenge you the most. Repeated practice of these problems helps build familiarity and confidence.
• Breaking Down Complex Concepts: Instead of trying to tackle an entire topic at once, break it into smaller, more manageable segments. This allows for deeper understanding and easier retention.
• Utilizing Resources: Make use of available tools, such as instructional videos, textbooks, or online exercises, to reinforce concepts and practice regularly.

Tracking Progress

To ensure you’re getting the most out of your focused practice, it’s crucial to measure your improvement. Tracking your progress helps you stay motivated and adjust your study plan as necessary:

Metric	Method of Measurement	Action
Accuracy	Review scores from drills and quizzes	Track improvement over time and focus on areas with the lowest scores
Speed	Set time limits for practice problems	Increase time limits gradually while maintaining accuracy
Concept Mastery	Self-assessment and feedback	Seek deeper understanding of complex areas, using additional resources

By incorporating targeted practice into your study routine, you can effectively address your weaknesses and refine your strengths. With focused effort and consistent tracking, you will maximize your score and gain confidence in your abilities.