Net Present Value Exam Questions and Answers
In the world of finance, assessing the profitability and feasibility of various investments is crucial. One of the most important methods used for such evaluations involves analyzing future cash flows and understanding how they compare to initial investments. This approach allows decision-makers to determine whether a project or opportunity is worth pursuing based on its potential returns.
To succeed in mastering this evaluation technique, it is essential to have a solid grasp of the core principles that drive these calculations. The process requires not only applying mathematical formulas but also interpreting the outcomes in a way that reflects real-world financial situations. Understanding the key components involved in these calculations can significantly improve your ability to solve related problems effectively and confidently.
Successful preparation for tackling such problems involves familiarizing yourself with the foundational concepts, recognizing potential pitfalls, and practicing different scenarios. By focusing on these aspects, you can enhance your ability to approach complex situations with ease and precision. This guide will walk you through important techniques and provide examples to help reinforce these skills.
Net Present Value Exam Questions and Answers
When preparing for financial assessments, it’s crucial to understand how future cash flows influence decision-making. Mastering the underlying concepts can greatly improve performance in solving related tasks. Focusing on different types of problems involving financial returns will equip you with the necessary skills to analyze complex scenarios effectively. The goal is to identify key factors that impact the overall outcome, leading to more informed investment decisions.
Common Challenges in Financial Calculations
One common challenge in these tasks is properly interpreting the data provided. Understanding how factors such as discount rates and timing affect the final result is essential. Moreover, ensuring accuracy in calculations, particularly when dealing with multiple periods, can be tricky. Recognizing these potential obstacles helps in tackling problems with greater confidence.
Approaching Complex Investment Scenarios
In more complex scenarios, it’s important to break down the task into manageable steps. Start by analyzing the initial investment and comparing it against the future returns, taking into account the time factor. This method ensures a clear path to evaluating whether the financial opportunity is worthwhile. With sufficient practice, tackling a wide range of scenarios becomes more intuitive, leading to improved problem-solving skills.
Understanding Financial Evaluation Concept
At the core of investment decision-making lies the concept of evaluating future returns against initial expenditures. This method allows businesses and individuals to assess whether a project or opportunity will yield sufficient profits to justify the costs. By focusing on the time factor, the approach helps determine the true worth of an investment over time, accounting for the inevitable changes in the financial landscape.
The Role of Time in Financial Assessments
One key aspect to grasp in these calculations is the importance of time. Future returns are not always as valuable as immediate ones due to the concept of opportunity cost. This means that the longer an investment takes to pay off, the less its future returns will be worth. Understanding this principle is crucial in making accurate financial projections and evaluating long-term projects.
Calculating the True Worth of Investments
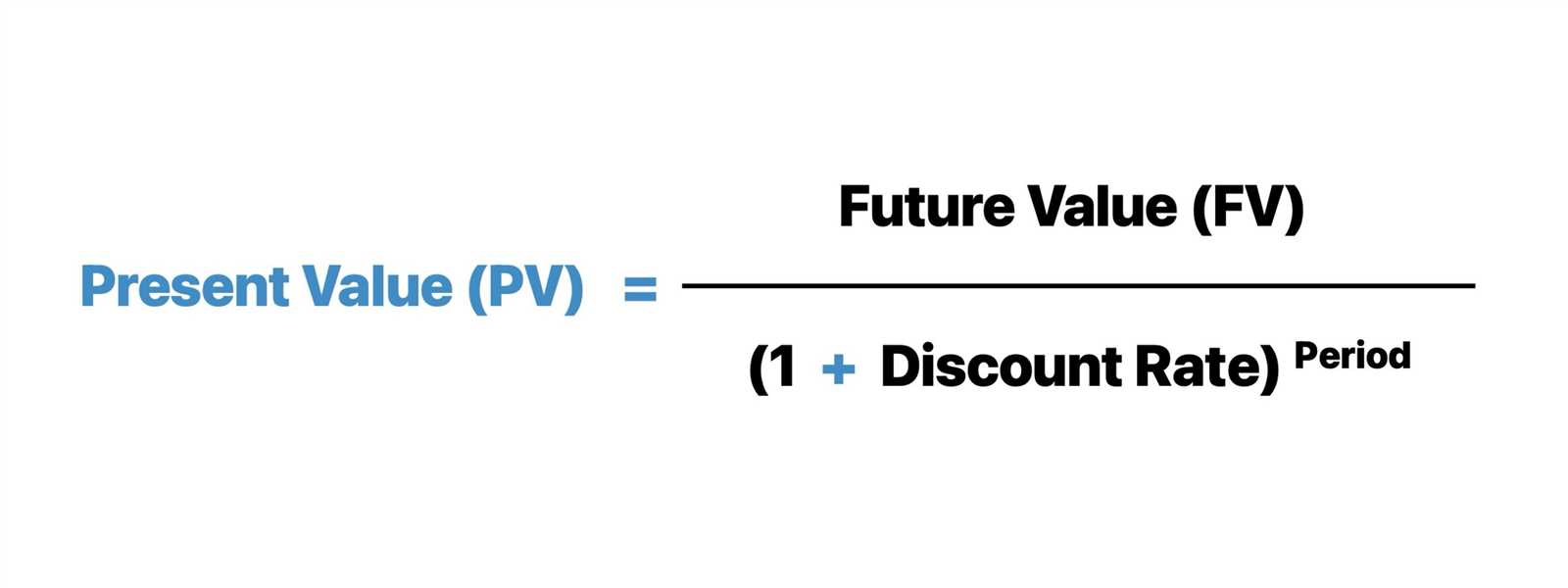
To calculate the true worth of an investment, it’s necessary to consider both expected profits and the timing of those profits. A discount rate is applied to adjust future earnings, ensuring that they are appropriately valued in today’s terms. This adjustment ensures that decision-makers can compare different investment opportunities on an equal footing, enabling better strategic choices.
Importance of NPV in Financial Analysis
In the world of financial evaluation, the ability to measure the profitability of potential investments is essential. The focus is often on determining whether the returns from a project will outweigh the initial costs. A key tool in this process helps to assess how much future earnings are worth in today’s terms, providing a clearer picture of the investment’s feasibility. This method is widely used in both corporate finance and personal investment planning to ensure informed decision-making.
Benefits of Using NPV for Investment Decisions
Using this method allows financial analysts and decision-makers to:
- Compare multiple investment options based on their potential profitability.
- Ensure that the timing of cash flows is accounted for, leading to more accurate assessments.
- Make strategic decisions that align with long-term financial goals.
Impact on Business Strategy
For businesses, understanding how to calculate the profitability of a project or opportunity is crucial for long-term sustainability. By applying this method, companies can prioritize projects that generate the highest returns, leading to:
- Optimized use of capital resources.
- More predictable financial outcomes.
- Reduced risk in investment decisions.
This approach ensures that the business remains on a path that maximizes financial growth while minimizing unnecessary risks.
Key Formulas for NPV Calculation
To accurately assess the profitability of an investment, it’s essential to use the right mathematical models. These formulas allow for the comparison of the initial investment against future cash flows, adjusted for the time factor. Understanding how to apply these formulas will give you the tools necessary for making informed decisions about which opportunities to pursue. Below are the primary calculations you need to know for proper financial assessments.
Basic Formula for Discounting Cash Flows
The primary formula used in this evaluation is the discounting of future cash flows to determine their value in today’s terms. The formula is as follows:
| Formula | Explanation |
|---|---|
| NPV = Σ (Cash Flow / (1 + r)^t) | Where Cash Flow is the money expected in the future, r is the discount rate, and t is the period or time point. |
Understanding the Discount Rate
The discount rate plays a significant role in adjusting future earnings to their present value. A higher rate will reduce the future value of cash flows, while a lower rate will result in a higher value. It’s crucial to choose the appropriate rate based on the project’s risk level and the market conditions. This decision can heavily influence the final result of the evaluation, making it an important factor to consider when using these formulas.
Common Mistakes in NPV Calculations
While performing financial assessments, it’s easy to make errors that can significantly alter the results. These mistakes often arise from misunderstandings of key principles or misapplications of formulas. Being aware of common pitfalls helps ensure that calculations are accurate and that decision-making is based on reliable information. Below are some of the most frequent errors encountered during financial evaluations.
Incorrect Discount Rate Application
One of the most common errors in these calculations involves the misapplication of the discount rate. An incorrect or inconsistent rate can lead to skewed results, making it difficult to accurately assess profitability. Common mistakes include:
- Using a fixed rate for all time periods, even when market conditions change.
- Failing to adjust for inflation or other external factors that affect the rate.
- Using a rate that does not reflect the true risk of the investment.
Ignoring Cash Flow Timing
Another frequent mistake is overlooking the timing of cash flows. The value of money changes over time, so failing to account for when cash flows occur can result in misleading assessments. This includes:
- Disregarding the difference between immediate and future payments.
- Assuming that all cash flows occur at the end of each period rather than spread out throughout the period.
- Misjudging the total amount or frequency of cash flows over time.
By avoiding these errors, you can ensure more accurate calculations and make better-informed investment decisions.
How to Interpret NPV Results

Understanding the outcomes of your financial evaluations is crucial for making informed decisions. The results of such assessments tell you whether an investment is likely to generate positive returns or lead to a loss. By interpreting these results correctly, you can determine whether a project aligns with your financial goals and whether it is worth pursuing. The following table summarizes how to interpret different outcomes of these calculations.
| Result | Interpretation |
|---|---|
| Positive Result | Indicates that the investment is expected to generate more returns than the initial outlay, making it a potentially profitable choice. |
| Negative Result | Suggests that the project is expected to result in a loss, with returns falling short of the original investment, making it an unwise choice. |
| Zero Result | Indicates that the project is expected to break even, where the returns exactly match the initial investment. This suggests neither a gain nor a loss. |
By recognizing these outcomes, you can make more confident decisions about which projects or investments to pursue. A positive result typically means it’s worth moving forward, while a negative result should prompt a reassessment of the investment’s viability. A zero result requires further consideration, such as evaluating alternative opportunities or adjusting assumptions like cash flow or the discount rate.
NPV vs IRR: Key Differences
When evaluating investment opportunities, two of the most commonly used methods are profitability analysis through discounting future cash flows and the rate of return at which an investment breaks even. While both techniques are crucial for assessing financial projects, they differ in approach, interpretation, and the type of decisions they guide. Understanding the distinctions between these two methods helps investors choose the best tool for their specific needs.
Net Cash Flow Evaluation vs Return Rate
One of the primary differences between these two approaches lies in how they measure success. The first method focuses on determining the total monetary value that an investment will generate over time, while the second assesses the rate of return that would make the investment break even. Essentially, the first method gives a concrete amount of profit, while the second gives a percentage rate.
Calculation Methodology
The calculation for the first method involves discounting future earnings to the present to determine the net monetary value of an investment. On the other hand, the second approach calculates the rate at which the present investment must grow to yield zero profits when discounted. Each method requires different formulas, with the first depending on discounting cash flows and the second solving for the discount rate that results in a zero net profit.
Summary of Key Differences:
- NPV measures total value in current terms, while IRR determines the rate of return that makes the project break even.
- NPV can provide a clearer financial value, especially when comparing projects with different scales, while IRR is useful for understanding the efficiency of an investment.
- NPV requires a specified discount rate, while IRR calculates its own internal rate of return based on project cash flows.
Both methods are widely used and important for making informed financial decisions. Understanding their differences ensures that you can choose the most appropriate one for evaluating different types of investments.
Steps to Solve NPV Exam Questions
When tasked with evaluating the profitability of an investment, understanding the systematic process of calculating the financial outcome is essential. The process involves several key steps, from gathering the necessary data to applying the correct formula. Following a structured approach ensures accuracy and efficiency, ultimately helping to make informed decisions based on the results. Below is a step-by-step guide to help solve these types of financial problems.
Step 1: Identify Cash Flows
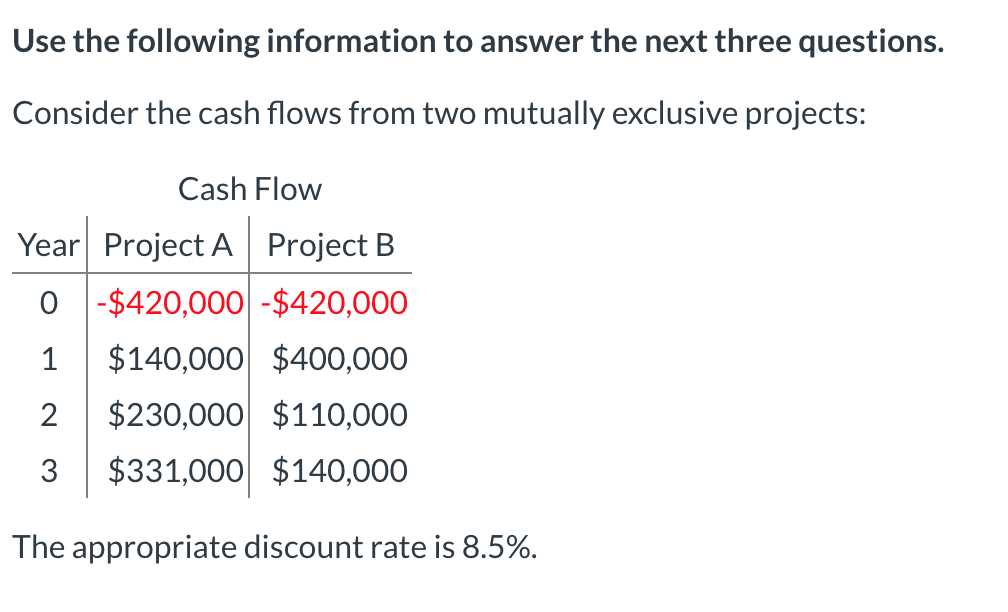
The first step in the calculation process is to gather all the relevant cash flows associated with the investment. These include both the initial outlay (cost) and the expected future returns. Be sure to:
- Account for any initial investment or expenditures.
- List the expected income or savings from the project for each period.
- Ensure that the timing of each cash flow is clear, as this will affect the discounting process.
Step 2: Determine the Discount Rate
The next critical step is determining the appropriate rate at which future cash flows will be discounted to present value. The rate often reflects the cost of capital or the expected rate of return on a similar investment. Consider the following when determining the discount rate:
- The level of risk associated with the investment.
- The time horizon of the project.
- Market interest rates and inflation factors.
Once these elements are established, you can proceed to the next phase of the calculation, applying the rate to discount future cash flows.
Step 3: Apply the Formula and Solve
With all the necessary data in place, the final step is to apply the formula to calculate the overall financial worth of the investment. The general formula is to discount each future cash flow by the determined rate for each time period, then sum them together, subtracting the initial outlay. The result will give you the net profitability of the project.
- If the result is positive, the project is expected to generate more than it costs.
- If the result is negative, the investment is likely unprofitable.
By following these steps, you can methodically evaluate the potential success of any financial opportunity, ensuring that decisions are based on solid, quantitative reasoning.
NPV in Investment Decision Making
When it comes to making financial decisions, evaluating potential returns is critical for determining whether a particular investment is worthwhile. A common approach to this evaluation is assessing the expected future cash flows generated by an investment, which are adjusted for the time value of money. This method provides a clear picture of the project’s long-term profitability and helps decision-makers choose the most beneficial options. Below, we explore how this approach plays a pivotal role in guiding investment choices.
Evaluating Profitability with NPV
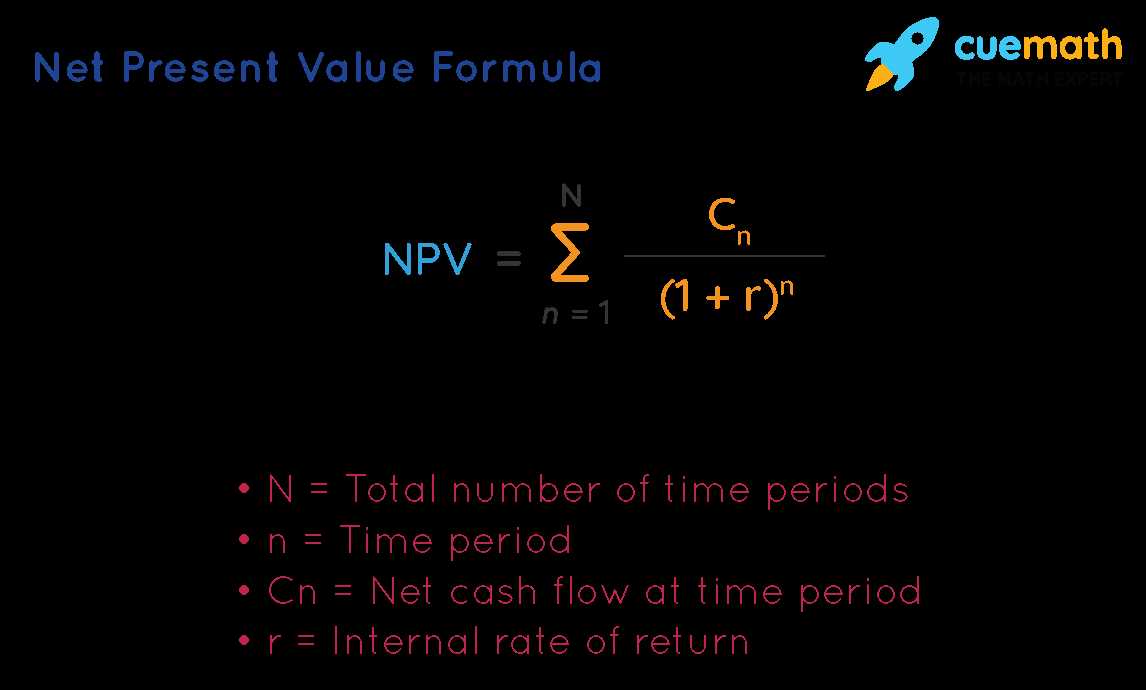
The use of this financial tool allows investors to compare various investment opportunities based on their projected future earnings. By calculating the total monetary benefit in today’s terms, businesses can assess whether the investment will generate enough profit to justify the initial expenditure. A positive result suggests that the project will yield a favorable return, while a negative result indicates that it may lead to a loss. This insight is particularly valuable when comparing investments of different sizes and durations.
Risk and Uncertainty in Decision Making
One of the strengths of this method is its ability to account for risk and uncertainty by incorporating a discount rate that reflects the project’s risk profile. Investments with higher perceived risks will require a higher rate, which reduces the overall calculated value. By adjusting for risk, this approach helps decision-makers determine which projects align with their risk tolerance and financial objectives. Moreover, it provides a tangible metric for comparing the financial viability of alternative options.
In summary, utilizing this financial analysis method aids businesses in making more informed, objective decisions. It helps investors prioritize projects that promise the highest return on investment while considering the costs, risks, and timeframes involved.
Examples of NPV Calculation Problems
Understanding how to apply the method of assessing the profitability of an investment is crucial for making well-informed financial decisions. By walking through real-life scenarios, we can demonstrate how to calculate and interpret results, providing a deeper understanding of this financial technique. Below are examples that will help you apply these concepts in practice.
Example 1: Simple Investment with Fixed Cash Flows
Consider a project where an initial investment of $10,000 is expected to generate cash flows of $3,000 annually for the next 5 years. If the required rate of return is 8%, the objective is to calculate the overall profitability of the investment by discounting the future cash flows to their present value. The process is as follows:
- Initial Investment: $10,000
- Annual Cash Flow: $3,000 for 5 years
- Discount Rate: 8%
Using the formula, we discount each future cash flow at 8% for the appropriate time period and sum them together. The result will give us the total profitability, allowing us to assess if this investment will be worthwhile.
Example 2: Comparing Two Investment Options
Now, let’s compare two investment opportunities. Investment A requires an initial outlay of $20,000, while Investment B requires $25,000. Both projects will generate the same annual cash flow of $6,000 for 6 years. However, Investment A has a required rate of return of 7%, while Investment B has a rate of 9%. The goal is to determine which investment offers the best return by calculating the financial worth of each investment.
- Investment A: Initial Outlay = $20,000, Cash Flow = $6,000, Rate = 7%
- Investment B: Initial Outlay = $25,000, Cash Flow = $6,000, Rate = 9%
By applying the respective rates to each investment’s cash flows, you can compare the resulting profitability and choose the better option. This demonstrates how understanding the discounting process helps in decision-making, especially when comparing different opportunities.
Analyzing Cash Flow Patterns for NPV

In financial analysis, understanding the pattern of cash inflows and outflows is essential to accurately assess the profitability of an investment. The timing, magnitude, and consistency of these flows directly influence the final result of the financial assessment. By analyzing these cash flow patterns, we can gain insights into how an investment behaves over time and determine whether it will generate a sufficient return. This section will delve into the different types of cash flow patterns and their implications for financial decision-making.
Consistent Cash Flow Patterns
In many cases, investments generate regular, predictable cash flows over a specific period. For instance, a project may provide the same amount of cash inflows each year, allowing analysts to use this predictability to assess long-term viability. Consistent patterns simplify the calculation process and provide clearer insights into whether the investment is worth pursuing, as the future cash flows can be easily discounted to present terms using a fixed discount rate.
Irregular Cash Flow Patterns

On the other hand, some investments may exhibit irregular cash flows, with varying amounts or timings. For example, a project may generate high returns in some years but low or negative returns in others. This type of cash flow pattern requires a more nuanced approach, as the irregularity must be factored into the analysis to accurately reflect the investment’s risk and potential reward. When assessing irregular cash flows, it’s important to use flexible models that can accommodate the fluctuations, allowing for a comprehensive evaluation of the overall investment potential.
By carefully analyzing these patterns, investors can make better-informed decisions and optimize their financial strategies. Understanding how different cash flow scenarios affect profitability is a key component of sound investment planning.
Impact of Discount Rate on NPV
The discount rate plays a crucial role in determining the financial worth of an investment, as it reflects the time value of money. It represents the opportunity cost of capital and the risk associated with an investment. A higher discount rate indicates a higher level of risk and a greater opportunity cost, which leads to lower discounted future cash flows. Conversely, a lower discount rate suggests less risk and a lower opportunity cost, resulting in higher future cash flows. Understanding the impact of different discount rates is essential for making sound financial decisions.
Effects of a Higher Discount Rate
A higher discount rate typically reduces the profitability of an investment, as future cash flows are less valuable when discounted at a higher rate. This is because the future returns are being compared to the current investment, which could potentially generate more immediate or safer returns. In scenarios with high perceived risk or uncertainty, investors tend to use a higher discount rate to account for this risk, which leads to:
- Lower present value of future returns
- Increased difficulty in justifying the investment
- Potentially negative NPV, indicating the project may not be profitable
Effects of a Lower Discount Rate

On the other hand, a lower discount rate increases the financial attractiveness of an investment, as future cash flows are worth more in today’s terms. This is especially true in low-risk, stable investments. A lower rate may be used in environments with lower inflation or lower perceived risks, which results in:
- Higher present value of future returns
- Greater likelihood of a positive NPV, suggesting profitability
- Increased investor confidence in the project’s potential
Ultimately, the choice of discount rate is influenced by factors such as the perceived risk of the investment, the investor’s required rate of return, and the broader economic environment. Adjusting the discount rate provides investors with a tool to account for these variables and make informed investment decisions.
Understanding the Time Value of Money
The concept of the time value of money is fundamental to financial decision-making. It reflects the idea that a sum of money today is worth more than the same amount in the future due to its potential to earn returns over time. This principle is based on the understanding that money has the ability to generate interest or investment returns, meaning that its value changes as time passes. Recognizing this concept is crucial for evaluating investments, assessing project profitability, and making informed financial decisions.
The Core Principle
At the heart of this concept is the opportunity to earn a return on money invested today. The longer you wait to receive a payment or return, the less valuable it becomes because of the missed opportunity to earn interest or investment gains. For example, if you receive $1,000 today, you can invest it and earn interest, whereas receiving $1,000 in a year means missing out on that growth potential.
- Money today can be invested to generate returns
- Future amounts are worth less due to inflation and opportunity cost
- The longer you wait, the lower the value of future sums
Key Factors Affecting the Time Value
Several factors influence how the time value of money is calculated and understood:
- Interest Rates: The rate at which money can grow over time. Higher interest rates increase the potential for returns and therefore the value of money today.
- Inflation: The rate at which the purchasing power of money decreases. Higher inflation reduces the future value of money.
- Risk: The uncertainty of receiving future payments or returns. The higher the risk, the less valuable future money is.
These factors all combine to shape how financial analysts determine the worth of future cash flows, investments, and projects. A strong understanding of the time value of money helps investors and decision-makers accurately assess the potential of their financial ventures.
Real-Life Applications of NPV
The concept of evaluating future financial flows plays a critical role in various sectors, guiding decisions that shape the economic landscape. By assessing the profitability of projects and investments, it allows businesses and investors to determine the feasibility of long-term commitments. Understanding this approach is key to making informed choices in a wide range of fields, from corporate finance to public sector projects.
One of the primary applications is in investment decision-making, where companies use this method to decide whether to proceed with capital-intensive projects. Whether it’s launching a new product line, expanding into a new market, or building a new facility, organizations must weigh the projected costs against the expected returns over time.
Additionally, this concept is crucial in project financing, where it helps to determine whether a proposed investment will generate enough returns to justify the initial expenditure. Real estate development, infrastructure projects, and mergers and acquisitions all rely on such evaluations to minimize risk and maximize return on investment.
Financial analysts also use this method to assess the viability of different investment opportunities, comparing options with varying timeframes and risk levels. It enables them to provide strategic advice on which investments are likely to bring the best returns over time, based on a thorough analysis of future cash flows and discount rates.
Challenges in NPV Calculations
While calculating the financial worth of future cash flows is a powerful tool for decision-making, there are several challenges that can complicate its accuracy and application. These obstacles often arise from uncertainties in forecasting, assumptions about future performance, and the complex nature of financial modeling. Addressing these issues requires careful planning, sound judgment, and a clear understanding of the underlying assumptions.
Estimating Future Cash Flows
One of the most significant challenges lies in accurately predicting future revenues and expenses. Cash flows are inherently uncertain, and even minor errors in estimation can drastically affect the results. Market conditions, economic fluctuations, and changing consumer behavior can all lead to unexpected variations, making forecasts difficult to achieve with precision. As such, relying on overly optimistic or pessimistic assumptions can distort the financial analysis.
Choosing the Appropriate Discount Rate
Another major hurdle is selecting the correct discount rate. The rate must reflect the cost of capital, the risk associated with the investment, and the time value of money. However, determining an appropriate rate can be subjective and depends on various factors, such as industry standards, investor expectations, and macroeconomic conditions. A small change in the discount rate can lead to substantial differences in the final result, making this step crucial for accurate calculations.
Additionally, using a uniform discount rate for projects with varying risk profiles or time horizons can lead to misleading outcomes. Different projects may require different rates based on their specific risk characteristics, requiring analysts to carefully tailor their assumptions to each situation.
NPV for Mutually Exclusive Projects

When comparing projects that cannot be undertaken simultaneously, the financial evaluation process becomes more critical. These types of investments, known as mutually exclusive projects, require careful analysis to determine which option will deliver the highest return. The ability to assess the financial feasibility of each alternative plays a vital role in making the optimal choice. In such cases, the decision-makers rely on discounted cash flow methods to evaluate which project provides the best financial advantage over time.
Choosing the Right Project
In scenarios involving mutually exclusive projects, selecting the best investment option becomes a matter of choosing the one that provides the highest financial return. This is achieved by calculating the projected inflows and outflows of each project and discounting them to their present value. Once all future cash flows have been accounted for, the project with the highest value should be selected, as it is expected to bring the most benefit relative to its cost.
Table of Project Comparison
| Project | Initial Investment | Annual Cash Flows | Discount Rate | NPV |
|---|---|---|---|---|
| Project A | $500,000 | $150,000 for 5 years | 10% | $120,500 |
| Project B | $500,000 | $170,000 for 5 years | 10% | $130,500 |
| Project C | $500,000 | $200,000 for 5 years | 10% | $150,000 |
As shown in the table above, after evaluating the different projects, the one with the highest calculated value should be chosen. In this example, Project C would be the preferred choice due to its highest NPV. This approach ensures that the resources are allocated to the most profitable venture, maximizing the potential return on investment.
Practical Tips for NPV Exam Success
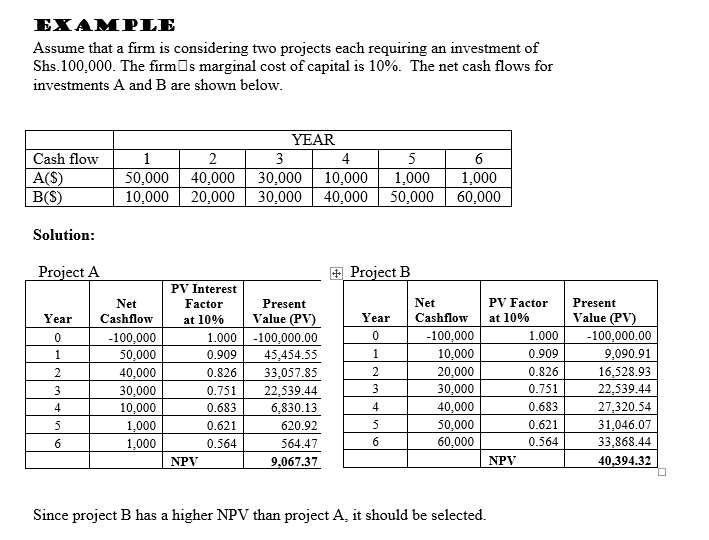
Mastering financial assessments involves understanding key concepts and being able to apply them efficiently under timed conditions. When preparing for tests involving investment evaluations, it’s crucial to develop a solid approach to solving problems accurately and quickly. A strategic mindset can make all the difference in achieving success. Below are several tips to help you approach these tasks with confidence and precision.
Preparation Strategies
- Understand the Key Formulas: Make sure you are familiar with the calculation formulas used in evaluations. Memorizing the basic formula for discounting cash flows and understanding how to manipulate it will help you save time during the test.
- Practice with Sample Problems: Solve a wide variety of practice problems to familiarize yourself with different scenarios. The more examples you work through, the more confident you’ll feel when it’s time to take the test.
- Focus on Cash Flow Analysis: Pay close attention to the cash flow structure in each question. Recognizing the pattern of inflows and outflows will help you make the correct calculations quickly.
- Use a Calculator Efficiently: Familiarize yourself with the financial functions of your calculator to streamline your calculations. This will prevent errors and save time during the actual test.
Time Management Tips
- Prioritize Simple Questions: Start with the problems you find easiest. This ensures you accumulate points early and builds confidence.
- Break Down Complex Problems: For tougher questions, break them down into smaller, manageable steps. This helps prevent confusion and ensures accuracy.
- Keep an Eye on the Clock: Allocate a set amount of time per question. Don’t spend too long on any single problem. If you’re stuck, move on and come back to it later.
By focusing on these strategies, you’ll be well-prepared to tackle any financial evaluation task with speed and accuracy. Developing a clear understanding of the fundamentals and practicing regularly will go a long way in boosting your performance on test day.