Regents Exam Questions G.SRT.B.5 Similarity 1 Answers

Geometry problems often require students to apply a variety of mathematical principles to understand the relationships between shapes, especially when they involve proportions and scaling. One of the key skills in this area is identifying and solving for unknowns in triangles with similar properties. These problems test your ability to recognize when figures share the same angles and how to use their proportional sides to find missing measurements.

In this section, we will explore essential techniques for tackling geometric problems that involve proportional reasoning and triangle relationships. With a focus on clear, step-by-step approaches, we aim to guide you through the process of determining missing values based on known properties. Through practice and understanding of core concepts, you’ll gain the confidence to solve complex problems efficiently and accurately.

Understanding the core principles behind these mathematical relationships is crucial for solving challenging problems. By honing your skills in recognizing proportionality and applying the right methods, you can approach these types of challenges with ease and precision. Let’s dive into the techniques that will help you excel in these tasks.

Regents Exam Questions G.SRT.B.5 Similarity 1 Answers

Understanding geometric relationships is essential when solving problems involving proportionality. In these tasks, you are often required to identify how figures relate to each other in terms of size and shape. The ability to recognize and apply these relationships allows you to find missing information based on known values. Mastery of these concepts is key to progressing through related problems with confidence and accuracy.

When dealing with these types of problems, it’s important to focus on the properties of figures and the ways in which their sides and angles correspond. A well-established approach involves using proportionality rules to set up equations that will lead to the correct solution. This method ensures that you can handle a variety of problems efficiently and consistently.

Proportionality plays a central role in solving these tasks. By recognizing that two figures share the same shape but differ in size, you can apply known ratios to determine unknown dimensions. Careful attention to detail and clear understanding of geometric principles will guide you through the process and help you achieve accurate results every time.

Understanding G.SRT.B.5 Similarity Concept

In geometry, the relationship between two shapes can often be defined by their proportionality. When two figures are similar, they share the same shape, but their sizes may differ. Recognizing this relationship allows you to solve for unknown dimensions based on known values. The key to mastering such problems lies in understanding how the sides of similar shapes relate to each other through ratios.

Key Principles of Proportionality

The core idea behind these types of problems is proportional reasoning. When dealing with figures that are similar, the corresponding sides are proportional. This means that the ratio of one side of a figure to the corresponding side of another figure remains constant. This concept helps in setting up equations to find missing measurements when certain values are already known.

Applying Proportions to Solve Problems

To apply the concept of proportionality, one must recognize corresponding parts of the figures and set up a proportion. This proportion will allow for solving for unknown sides or angles by equating the ratios of corresponding sides. The following table outlines a basic example of how proportions are used in similar triangles.

Triangle 1	Triangle 2	Proportionality Equation
Side 1 = 5	Side 1 = 10	5/10 = x/y
Side 2 = 8	Side 2 = y	8/y = 5/10
Side 3 = 12	Side 3 = x	x/12 = 5/10

By solving the proportion equations, one can find the missing values for the sides of the second triangle, illustrating how proportionality leads to the solution. This method can be applied to a variety of geometric problems involving similar figures.

Key Strategies for Solving Regents Problems

When approaching geometry problems, especially those that involve relationships between shapes and proportionality, a structured strategy can make all the difference. The ability to break down a problem into manageable steps, apply relevant mathematical principles, and check the work is crucial for success. Developing a methodical approach helps you stay organized and focused, allowing you to solve problems more effectively.

Step-by-Step Approach

One of the most effective strategies is to tackle each problem step by step. Start by carefully reading the problem to identify known and unknown values. Next, determine which mathematical principles apply–whether it’s proportionality, angle relationships, or geometric formulas. Then, set up the equations that will lead to the solution. By following these steps in order, you ensure a thorough and accurate solution process.

Using Proportions and Ratios

In many geometric problems, the use of proportions is essential. When two figures are related by proportionality, you can set up ratios to find unknown sides or angles. Ensuring that the corresponding parts of the figures are correctly matched is critical in these types of problems. The table below demonstrates how proportions are applied in a sample problem.

Figure 1	Figure 2	Proportion
Side A = 6	Side A = 12	6/12 = x/16
Side B = 9	Side B = y	9/y = 6/12
Side C = 18	Side C = z	z/18 = 6/12

By solving these proportions, you can find the missing values for the second figure, demonstrating the effectiveness of using ratios in geometric problems.

How to Approach Similarity in Geometry

When solving geometric problems that involve proportional relationships, it’s essential to focus on understanding how shapes relate to each other. Figures that share the same shape but differ in size can be solved using specific principles. The key to handling these problems is recognizing corresponding parts of the shapes and applying proportionality rules to determine unknown values. By breaking down the problem and focusing on these relationships, you can solve complex geometric challenges with confidence.

The first step in approaching these types of problems is to identify the corresponding sides and angles between the shapes. Once you have mapped out these relationships, you can set up equations using ratios that reflect the proportionality of the figures. This method ensures that each calculation is based on accurate geometric principles, leading to correct and reliable results.

After setting up your ratios, the next step is solving the equations. This often involves cross-multiplying and simplifying, followed by calculating the missing side lengths or angle measures. By consistently applying these strategies, you can approach any geometric problem that involves proportionality with a clear and logical process.

Identifying Similar Triangles in Geometry

In geometric problems involving triangles, it is important to recognize when two triangles share the same shape, even if their sizes differ. Identifying these relationships is the first step in solving problems that involve proportionality. The key is to check for corresponding angles that are equal and sides that are proportional. By identifying these patterns, you can establish that two triangles are related and apply the necessary mathematical principles to solve for unknowns.

One of the most reliable ways to determine if two triangles are similar is by using the Angle-Angle (AA) Criterion. This criterion states that if two triangles have two corresponding angles that are equal, the triangles are similar. In addition to the AA Criterion, the Side-Angle-Side (SAS) and Side-Side-Side (SSS) criteria can also be used to confirm similarity, as long as the corresponding sides of the triangles are proportional.

Once you have identified the similar triangles, you can then use the proportionality of their sides to set up equations that will allow you to find missing side lengths or other measurements. Understanding how to match corresponding parts and applying these criteria effectively will make it easier to solve problems involving similar triangles.

Step-by-Step Guide to Solving Geometry Problems

When faced with geometric problems that involve proportional relationships between shapes, it’s crucial to approach them in a structured and logical manner. Breaking down the problem into smaller, manageable steps helps ensure that all necessary calculations are made correctly and that the relationships between the figures are fully understood. This guide will walk you through a systematic approach to solve these types of problems with confidence.

The first step is to carefully analyze the given information. Identify the known and unknown values and determine the type of relationship between the shapes involved. Often, this will involve recognizing which sides and angles are proportional. Once you’ve established the relationship, you can proceed with setting up the necessary equations based on the proportionality rules.

Next, solve for the unknowns by applying basic algebraic techniques. This may involve simplifying ratios, cross-multiplying, or using the properties of proportional triangles. Once you have your equation set up, perform the necessary operations to isolate the unknown value. Finally, double-check your calculations to ensure that the results are consistent with the proportional relationships you identified earlier.

Common Mistakes in Geometry Problems

In geometric problems that involve proportionality and relationships between shapes, there are several common mistakes that students often make. These errors can stem from misidentifying corresponding parts, incorrectly setting up proportions, or overlooking key geometric principles. Recognizing and avoiding these mistakes is crucial to achieving the correct solution and developing a deeper understanding of the concepts involved.

One of the most frequent errors is mixing up corresponding sides or angles when dealing with similar shapes. It’s essential to carefully match corresponding parts, as using incorrect sides or angles will lead to incorrect ratios and, ultimately, wrong answers. Another common mistake is failing to simplify the ratios properly, which can result in unnecessary complexity and confusion.

The table below outlines some common mistakes and how to avoid them:

Mistake	Explanation	How to Avoid
Mixing up corresponding sides	Using the wrong sides in the proportion can lead to incorrect ratios.	Carefully label and match corresponding sides and angles before setting up ratios.
Overlooking angle congruence	For two triangles to be proportional, corresponding angles must be equal.	Always check that the angles are congruent before proceeding with the problem.
Not simplifying ratios	Failing to simplify ratios can lead to unnecessarily complicated calculations.	Always simplify fractions to their lowest terms before solving.
Incorrectly applying proportionality	Not all shapes or figures are proportional, and applying proportionality to the wrong figures can lead to errors.	Confirm that the figures are indeed proportional before setting up your equations.

By being aware of these common mistakes and following the correct steps, you can improve your accuracy and confidence in solving geometric problems involving proportionality.

Tips for Efficiently Solving Geometry Problems

Solving geometric problems that involve proportionality can be time-consuming and complex if not approached systematically. To solve these problems efficiently, it’s important to focus on key strategies that streamline the process. By following some simple but effective tips, you can improve both your speed and accuracy when working through problems involving proportional relationships between shapes.

1. Identify Corresponding Parts Early

One of the first steps in solving these problems is identifying the corresponding sides and angles of the figures. This will help you set up the necessary ratios and ensure that you are comparing the correct parts. Quickly identifying corresponding parts will save you time and prevent errors.

• Label all sides and angles before starting calculations.
• Mark corresponding parts clearly to avoid confusion during calculations.
• Check if angles are congruent before proceeding with proportionality tests.

2. Simplify Ratios and Fractions

Many students get bogged down by complex ratios or fractions. Simplifying them early on can save a lot of time and prevent errors in the later stages of problem-solving. Always simplify your ratios to their lowest terms as soon as possible.

• Divide both the numerator and denominator by their greatest common divisor (GCD).
• Always simplify fractions when setting up equations to avoid unnecessary complexity.
• Use decimal equivalents for easier comparison when possible, but only if needed for the final result.

3. Organize Your Work

Keeping your work neat and well-organized allows you to track your steps and avoid mistakes. Write out every step clearly, especially when setting up equations, so you can follow your reasoning and check for errors later.

• Write down all given information at the start.
• Break problems into smaller, manageable steps.
• Use tables or diagrams to visualize relationships between figures and their parts.

By following these strategies, you’ll be able to solve geometric problems involving proportionality more efficiently and with greater accuracy, leading to better results and a stronger understanding of the concepts involved.

Real-World Applications of Similar Triangles

The concept of proportional relationships between shapes, particularly triangles, has many practical applications in the real world. Understanding how to recognize and apply these relationships can help solve everyday problems in fields such as engineering, architecture, navigation, and even art. By using the principles of proportionality and geometric similarity, professionals can make precise calculations and design efficient solutions to complex challenges.

One of the most common uses of similar triangles is in construction and architecture. By understanding the relationship between different parts of a structure, architects can create accurate blueprints and scale models, ensuring that buildings and other structures are designed correctly and safely. Similarly, similar triangles are used in navigation to calculate distances that are otherwise difficult to measure directly.

• Surveying and Mapping: Geographers and surveyors use similar triangles to measure distances across large areas, such as rivers or mountains, where direct measurement would be impractical.
• Shadow and Height Calculations: Similar triangles help in determining the height of an object when the distance from the object and the angle of elevation or depression are known. This principle is often applied in determining the height of tall buildings or trees.
• Scaling Models: Architects and engineers use the properties of similar triangles to scale down large models while maintaining proportionality for accurate representations of real-world objects.

Understanding these applications not only enhances the practical utility of geometric concepts but also illustrates the importance of mathematical principles in solving real-life problems. By mastering these ideas, one can easily apply them in various fields where precision and accuracy are crucial.

How to Use Proportions in Geometry

Proportions play a crucial role in geometry, particularly when working with figures that share certain similarities or when comparing different parts of a shape. They help establish relationships between corresponding parts, allowing us to solve for unknown values or to compare different geometric figures accurately. By understanding how to set up and solve proportions, you can tackle a wide range of geometric problems with confidence and precision.

To use proportions effectively in geometry, the first step is recognizing when two ratios are equal. This can occur when two shapes are similar, meaning their corresponding angles are congruent, and their sides are proportional. For example, when working with triangles, you can set up a proportion to find missing side lengths, given the corresponding side lengths of a similar triangle.

• Setting Up a Proportion: Identify two ratios that involve corresponding sides or parts of the figures. For example, in two similar triangles, the ratio of one triangle’s side to the corresponding side of the other triangle is constant.
• Cross-Multiplying: Once the proportion is set up, use cross-multiplication to solve for the unknown value. This involves multiplying the numerator of one ratio by the denominator of the other ratio and setting the two products equal to each other.
• Checking Proportions: Always check if the ratios are set up correctly and if the final solution makes sense in the context of the problem. This ensures that the proportion you have used is valid and the result is accurate.

Using proportions is an essential skill in geometry that allows you to solve for unknowns, prove relationships between shapes, and understand how different parts of a figure are related to one another. By mastering proportions, you will gain a powerful tool for analyzing and solving a wide variety of geometric problems.

Common Assessment Question Formats

In many standardized assessments, certain question formats are commonly used to test a variety of mathematical concepts, including geometry and proportionality. These formats are designed to assess not only your knowledge of geometric principles but also your ability to apply these concepts to solve problems efficiently. By understanding these formats, students can better prepare for the types of problems they may encounter.

Multiple-Choice Questions

One of the most frequent formats is the multiple-choice question, where students are presented with a problem and given several possible answers to choose from. These questions often test your understanding of basic concepts, such as ratios, angles, and properties of shapes. To excel in these questions, it is essential to be familiar with the formulas and techniques commonly used to solve geometry problems.

Show Your Work Questions

Another common format involves problems where students are required to show their work in order to earn full credit. These questions typically involve multiple steps, where you need to apply mathematical principles, such as setting up proportions, solving for unknowns, and demonstrating the reasoning behind your solution. Practice is key to succeeding with this format, as it allows you to organize your thoughts and provide a clear, logical solution to the problem at hand.

Understanding these common formats and practicing with them will not only help you become familiar with the structure of the questions but also improve your problem-solving skills. By approaching each question with a clear strategy, you’ll be better equipped to tackle the challenges posed in any assessment.

Understanding Ratios in Similar Triangles

When studying triangles with proportional sides, one of the most important concepts to grasp is the ratio between corresponding sides. In many geometric problems, triangles are said to be similar, meaning their corresponding angles are equal and their sides are proportional. By understanding how to work with these ratios, you can solve various problems that involve similar triangles with ease.

Ratios in similar triangles are used to compare the lengths of corresponding sides. The fundamental principle is that the ratio of one pair of corresponding sides is equal to the ratio of the other pair of corresponding sides. This relationship allows for the solving of missing side lengths and proves the similarity between triangles.

• Proportionality of Sides: In similar triangles, the ratio of the lengths of corresponding sides is constant. For example, if triangle ABC is similar to triangle DEF, then the ratio of AB to DE will be equal to the ratio of BC to EF, and the ratio of AC to DF.
• Finding Unknown Lengths: By setting up a proportion, you can solve for unknown side lengths. For example, if you know the lengths of two sides in one triangle and the corresponding sides in the other triangle, you can use the ratio to find the missing side.
• Using the Scale Factor: The constant ratio between corresponding sides is called the scale factor. This factor helps in scaling one triangle to another and is used in many practical applications like map reading, architectural design, and model making.

Understanding how ratios work in similar triangles is essential for solving many geometric problems. By practicing these concepts and applying the correct ratios, you can accurately determine missing values and demonstrate the similarity between triangles in various contexts.

How to Find Missing Sides in Triangles

When solving geometric problems involving triangles, one common task is finding the length of a missing side. In many cases, the triangles will either be right-angled or similar, which provides specific relationships between the sides that can be used to determine unknown values. Understanding these relationships and applying the right methods is essential for solving such problems efficiently.

Using the Pythagorean Theorem

For right triangles, the Pythagorean theorem is a fundamental tool. This theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. The formula is:

a² + b² = c², where c is the hypotenuse, and a and b are the legs of the triangle.

If two sides are known, you can use this formula to solve for the missing side. For example, if you know the lengths of the legs and need to find the hypotenuse, you would rearrange the formula to:

c = √(a² + b²).

Using Proportions for Similar Triangles

In similar triangles, the ratios of corresponding sides are equal. If two triangles are similar, the lengths of their corresponding sides are proportional. This means you can set up a proportion to find the length of a missing side.

For example, if triangle ABC is similar to triangle DEF, and you know the lengths of two sides in both triangles, you can set up the proportion:

AB/DE = BC/EF = AC/DF

By solving this proportion, you can find the missing side of either triangle. This method is particularly useful when dealing with scaled or proportional figures in geometry.

By using these methods–whether applying the Pythagorean theorem in right triangles or using proportions in similar triangles–you can easily find missing side lengths and solve geometric problems effectively.

Visualizing Similar Triangles with Diagrams

Understanding the relationship between two triangles becomes much clearer when visualized with diagrams. Diagrams not only help identify corresponding sides and angles but also provide a concrete way to see how two triangles relate to each other geometrically. By drawing the triangles and labeling their elements, it becomes easier to apply principles like proportionality and angle congruence.

Steps for Creating Diagrams

When working with similar triangles, creating accurate diagrams is crucial. Here are the steps you can follow to visualize the triangles effectively:

• Draw the Triangles: Start by sketching two triangles that you suspect are similar. Make sure to maintain their proportional relationships, even if they are not drawn to scale.
• Label the Angles: Clearly label each angle in the diagram. For similar triangles, corresponding angles will be congruent, so it’s important to mark them appropriately.
• Mark Corresponding Sides: Identify and mark the corresponding sides of the two triangles. These sides will have proportional lengths in similar triangles.
• Use Color or Shading: To make the relationships between corresponding sides and angles more apparent, use color or shading to highlight the matching parts of the triangles.

Why Diagrams Help

Creating diagrams offers several advantages when working with similar triangles:

• Clarifies Relationships: Diagrams make it easier to understand the proportionality between corresponding sides, helping to solve problems faster.
• Enhances Problem Solving: A well-labeled diagram serves as a visual aid, reducing errors and aiding in calculations when working through problems.
• Improves Geometric Understanding: Visualizing triangles in this way enhances your ability to understand geometric concepts, such as similarity, scale factors, and proportionality.

By following these steps and creating detailed diagrams, you can better visualize the geometric relationships between triangles and simplify the problem-solving process.

Reviewing Previous Exam Questions

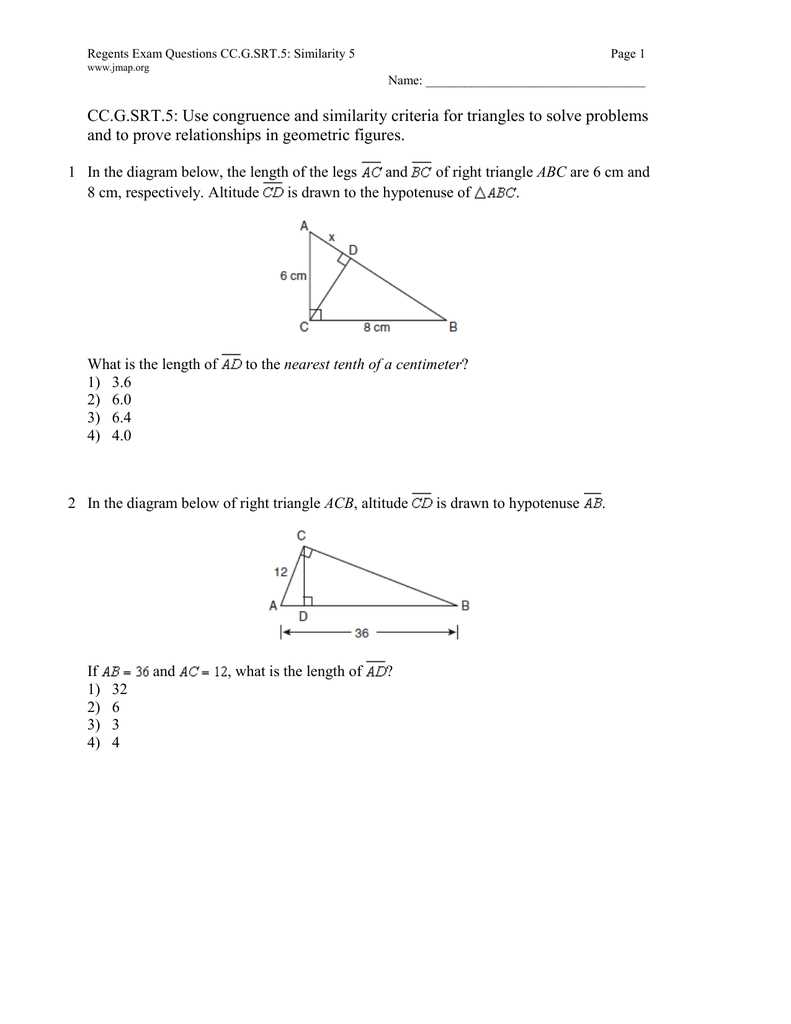
One of the most effective ways to prepare for any academic assessment is to review past problems. By looking at questions from previous tests, you can identify recurring patterns, understand the types of challenges that are commonly presented, and become familiar with the format. This review process helps to build confidence and sharpen problem-solving skills, allowing you to approach similar tasks more efficiently in the future.

Benefits of Revisiting Past Problems

Reviewing previous tests offers numerous advantages, particularly when it comes to mastering complex concepts:

• Identifying Common Themes: Certain concepts tend to appear repeatedly in assessments. By practicing these problems, you can better anticipate what might come up in future tests.
• Recognizing Question Patterns: Understanding the format and structure of typical problems allows you to approach them more strategically and manage your time more effectively.
• Building Confidence: Successfully solving past problems gives you a sense of accomplishment and helps reduce test anxiety.
• Clarifying Doubts: Reviewing questions you found challenging the first time gives you the opportunity to revisit tricky concepts and clarify any misunderstandings.

How to Review Effectively

Simply going over past questions isn’t enough; it’s important to review them strategically. Here are some tips for an effective review:

• Analyze Mistakes: Carefully examine any incorrect answers. Understanding why you made a mistake is key to improving.
• Practice Under Test Conditions: Time yourself while working through past questions to simulate the pressure of a real assessment.
• Use Solutions to Learn: Don’t just look at the answers; understand the step-by-step reasoning behind the correct solutions.
• Seek Help for Unclear Areas: If a concept still isn’t clear, seek assistance from a teacher, tutor, or study group.

By regularly reviewing past questions, you strengthen your problem-solving abilities and gain the insight needed to perform at your best in future assessments.

How to Prepare for Similarity Questions

When tackling geometric problems involving proportional relationships between shapes, it is crucial to understand the underlying principles and strategies that can make these problems more manageable. The key to solving these challenges lies in recognizing patterns, applying proportional reasoning, and mastering the relationships between corresponding parts of figures. With consistent practice and a clear approach, you can develop the skills needed to solve these problems efficiently.

Understanding Proportions and Ratios

The foundation of most problems in this category is the concept of ratios and proportions. These mathematical tools help relate the dimensions of similar figures. To prepare effectively, focus on:

• Identifying Corresponding Sides: In similar figures, the corresponding sides are proportional. Understanding how to match up these sides will simplify calculations.
• Mastering Proportionality: Being comfortable with setting up and solving proportion equations is essential for finding missing lengths or areas in geometric problems.
• Working with Scale Factors: Recognizing and applying scale factors is another key aspect when comparing similar shapes. This involves understanding how enlargement or reduction of shapes affects their dimensions.

Effective Practice Techniques

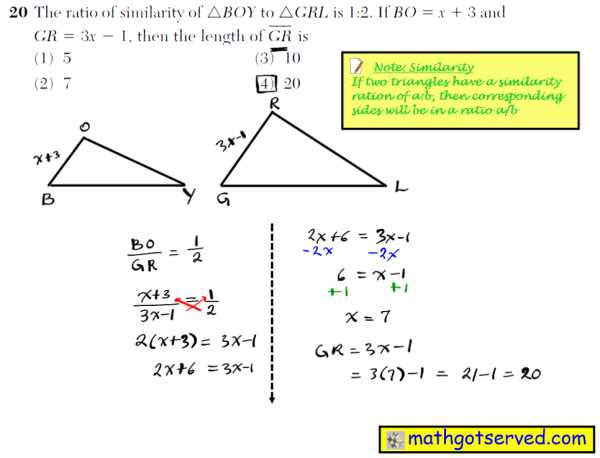
Practicing with a variety of problems will help you build confidence. Here are some methods to make your preparation more effective:

• Solve a Range of Problems: Work on both straightforward problems and more complex ones that require multiple steps to solve. This will help you become comfortable with different scenarios.
• Use Diagrams and Visual Aids: Drawing or visualizing shapes can clarify relationships between figures and make it easier to see how proportionality works.
• Review Mistakes: After solving practice problems, review any mistakes carefully. Understanding where and why you went wrong is crucial for improvement.
• Time Yourself: Practicing under timed conditions helps simulate real test situations, allowing you to work more efficiently under pressure.

With focused practice, a deep understanding of proportions and relationships, and strategic problem-solving techniques, you’ll be well-prepared to tackle any related challenges with confidence.