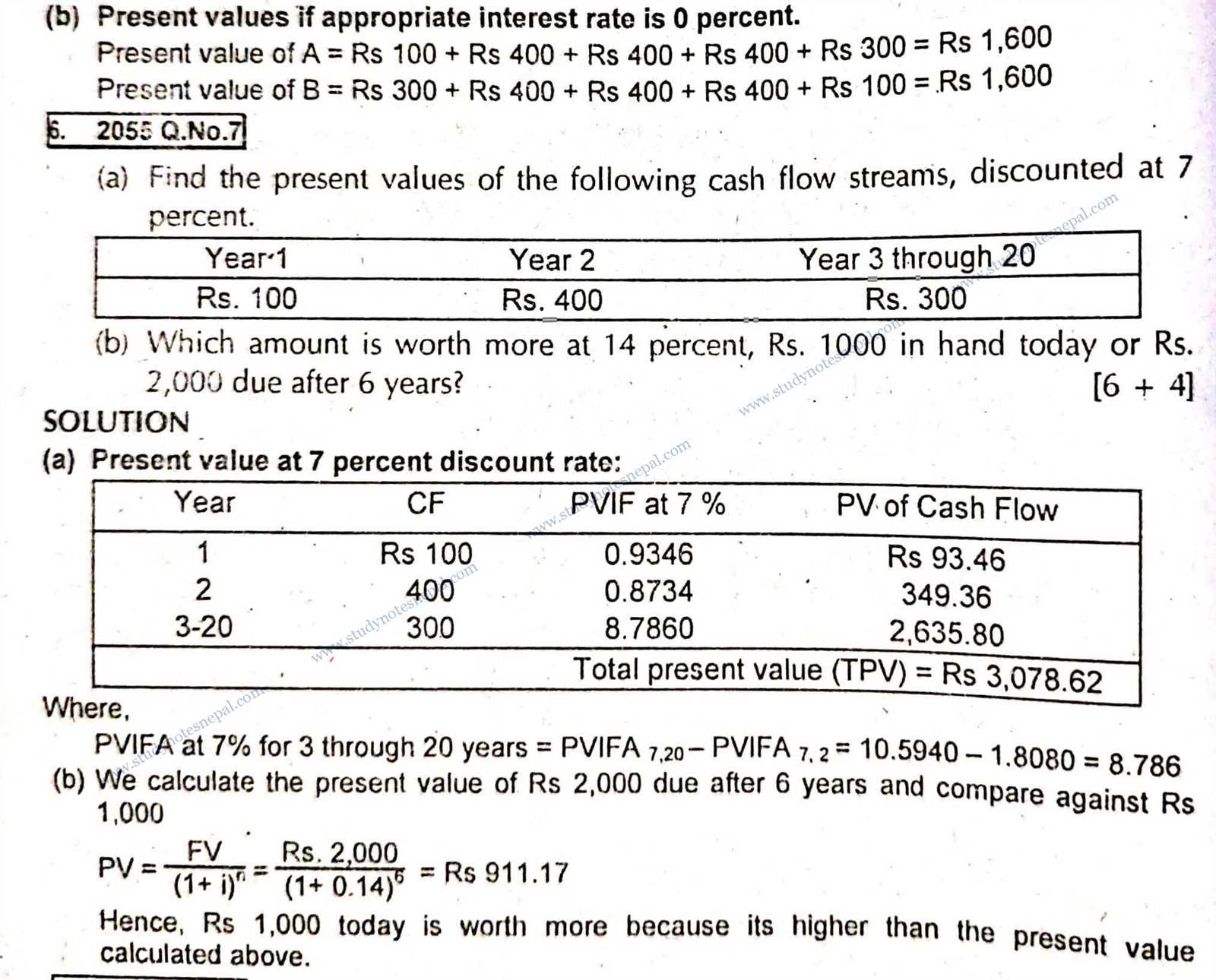
Time Value of Money Exam Questions and Answers

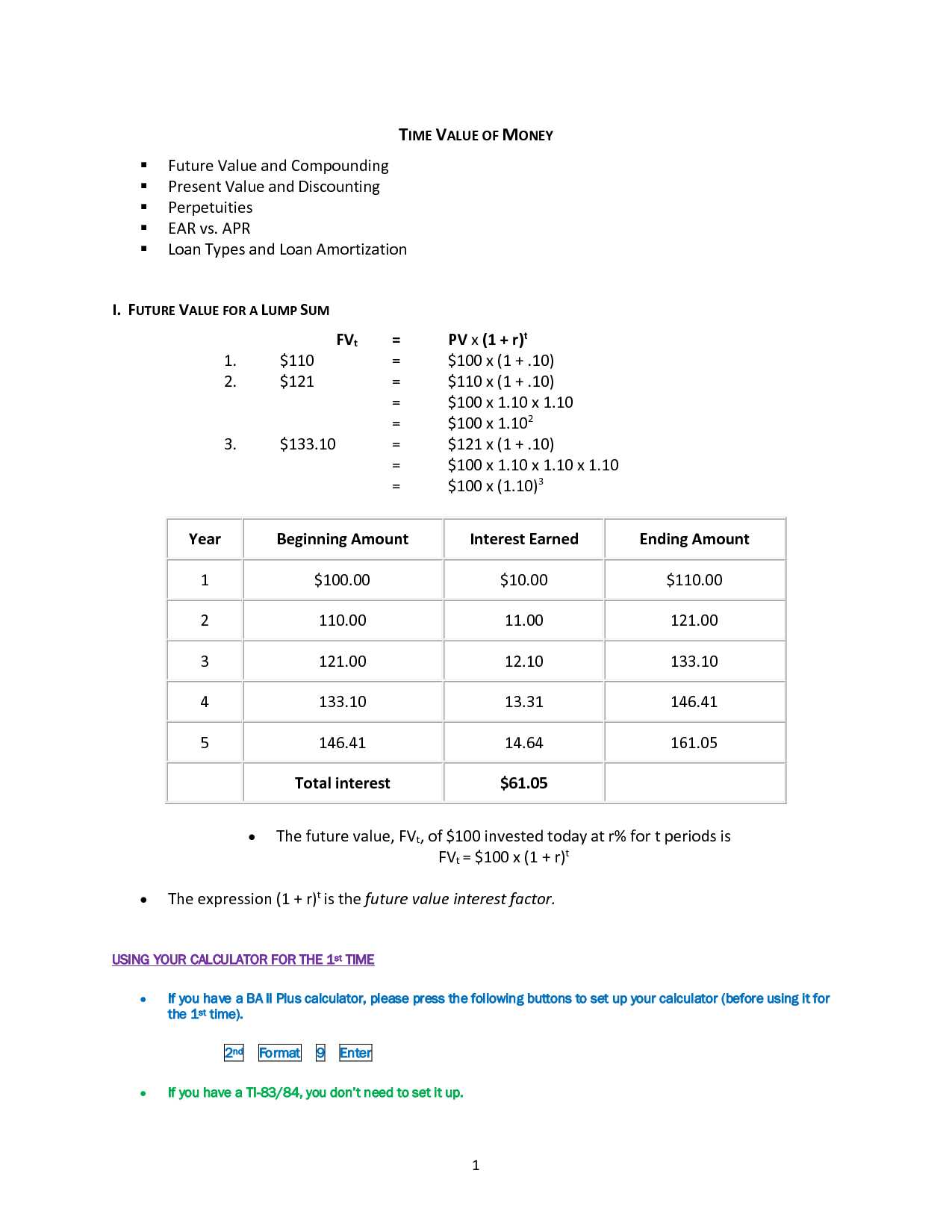
In any field of finance, understanding how to evaluate the worth of investments over time is crucial. These concepts help assess the potential returns and risks of financial decisions, allowing for better planning and forecasting. The ability to apply these principles effectively is vital for making informed choices in both personal and professional finance.

Many students face challenges when solving related problems, as they involve intricate calculations and the application of key formulas. Mastering these techniques is essential for success in any financial evaluation, whether it’s for academic purposes or practical investment scenarios. This section aims to break down complex topics into understandable segments, focusing on the most important methods and approaches.

By familiarizing yourself with the core principles, you will be better prepared to tackle various scenarios. Whether you are solving for future amounts, discounting cash flows, or calculating present worth, this knowledge provides a foundation for making strategic financial decisions.

With practice and understanding, the challenging calculations will become manageable, helping you build the necessary skills for mastering any related assessments.

Time Value of Money Exam Questions and Answers

Understanding how to evaluate financial amounts at different points in time is essential for assessing the profitability of investments or loans. The ability to apply formulas and techniques to determine future worth or present cost allows individuals to make informed decisions regarding financial transactions. This section aims to provide clarity on these fundamental principles by offering practical examples and solutions to common problems.

When solving related problems, it is important to grasp the key methods involved, such as compounding, discounting, and calculating interest rates. By mastering these techniques, you will be equipped to approach complex scenarios with confidence, whether in an academic setting or in real-world financial analysis. The following examples demonstrate various types of calculations you may encounter and how to approach them effectively.

Practice with diverse problems is crucial for building a strong foundation in these concepts. The more you work with different formulas and solve for unknowns, the easier it becomes to apply these principles to various financial contexts. With focused effort, you will be able to handle challenges related to investments, loans, and other financial evaluations smoothly.

Key Concepts in Time Value of Money

To understand financial assessments, it is crucial to grasp the essential principles behind how amounts change over time. These concepts form the foundation for evaluating the cost or return of investments and understanding financial growth or loss. The ability to calculate the worth of a sum at different points allows for better decision-making in both personal finances and business strategies.

Present and Future Calculations

At the core of these principles are two key calculations: determining the present value (how much a future sum is worth today) and the future value (how much a present sum will be worth at a later time). Both calculations are influenced by factors like interest rates and time periods, making them critical for accurate financial forecasting.

Interest Rates and Compounding

Interest rates play a significant role in how amounts grow or shrink over time. Compounding allows interest to be added to the principal sum at regular intervals, making future amounts higher than the initial investment. The more frequent the compounding periods, the greater the final value will be.

Concept	Description
Present Value	The current worth of a future sum, discounted at a given rate.
Future Value	The amount a current sum will grow to in the future, considering interest.
Interest Rate	The percentage that determines how much a sum grows over time.
Compounding	The process of adding interest to the principal and earned interest periodically.

Understanding Present and Future Value

In financial analysis, it’s crucial to understand how the worth of amounts changes over different periods. Calculating the current worth of future sums or predicting how today’s investments will grow is essential for informed decision-making. These calculations allow for a deeper understanding of the financial impact of different decisions, whether they involve investments, loans, or savings plans.

Present Worth of Future Cash Flows

The present amount of future inflows is determined by discounting those future amounts using a specific interest rate. This helps assess how much a future sum is truly worth today, considering factors like the time frame and interest rate. The formula for present worth involves reducing the future sum to reflect the impact of time and the rate of return.

Future Worth of Current Investments

On the other hand, determining how much a current investment will grow to in the future requires calculating its future worth. This involves applying an interest rate over a specific period to estimate how an initial sum will appreciate over time. The more frequent the compounding intervals, the greater the growth of the original amount.

Time Value of Money Formula Explained

At the heart of financial calculations is a formula that helps determine how much a given amount will be worth in the future or how much it is worth today. This formula takes into account factors such as interest rates, time periods, and the frequency of compounding, which are essential for understanding the growth or discounting of sums. By using the formula, you can accurately assess the impact of these factors on financial decisions.

The General Formula

The general formula used for calculating either the future or present amount is:

Future Value (FV) = PV * (1 + r)^n

Where:

• FV is the future worth of the sum.
• PV is the present amount or initial investment.
• r is the interest rate per period.
• n is the number of periods or time intervals.

Discounting Formula

To calculate the present value from a future amount, you use a similar formula, but with a discount factor:

Present Value (PV) = FV / (1 + r)^n

This formula allows you to determine how much a future sum is worth today, given a particular interest rate and time frame.

How Interest Rates Affect Calculations

Interest rates are a key factor in determining how amounts grow or decrease over time. They directly influence the calculation of both future sums and present values. Whether you’re assessing the return on an investment or the cost of a loan, the rate at which interest is applied can significantly alter the results. Understanding the impact of interest rates is essential for making accurate financial forecasts and decisions.

As interest rates increase, the future worth of a sum also increases, as the growth of the investment accelerates. Conversely, when the rate decreases, the potential growth slows down. This is true whether you’re compounding interest on savings or discounting future amounts to the present. The effect of interest rates is compounded over time, making them even more influential as the number of periods increases.

Interest Rate	Impact on Future Worth	Impact on Present Worth
High Rate	Increases future amount significantly	Reduces present value, making future payments seem less valuable
Low Rate	Moderate or low increase in future sum	Increases present value, making future payments seem more valuable
No Rate	No change to future sum	No discounting to the present, values remain the same

Compounding and Discounting Explained

Compounding and discounting are two fundamental concepts that help evaluate how amounts change over different periods. Compounding refers to the process of growing a sum by adding interest or returns to the principal, which then earns additional interest itself. On the other hand, discounting involves calculating the current worth of a future sum, reflecting the impact of time and interest rates. Both methods are critical in understanding how financial decisions are affected by time and rates.

Compounding: Growing Investment Over Time

When an amount is compounded, it earns interest on both the original principal and the accumulated interest. The more frequent the compounding intervals, the greater the final value of the investment will be. The following factors influence compounding:

• Interest Rate: Higher rates result in faster growth.
• Compounding Frequency: More frequent compounding leads to greater accumulation of interest.
• Time Period: The longer the time period, the greater the impact of compounding.

Discounting: Reducing Future Amounts to Present Worth

Discounting is the reverse process of compounding. It involves adjusting a future amount to its current worth by applying a discount factor, which is based on the interest rate and the time period. The higher the interest rate or the longer the period, the lower the present value of a future sum. The following factors influence discounting:

• Interest Rate: Higher rates reduce the present worth of future sums.
• Time Period: The longer the wait, the more the future value is reduced.

Types of Interest and Their Impact

The way interest is applied to financial sums plays a significant role in determining how amounts grow or are repaid over time. There are various types of interest, each affecting calculations differently. Understanding the differences between simple interest, compound interest, and other forms can help in making better financial decisions, whether you’re dealing with loans, investments, or savings plans.

Each type of interest has its own method of calculation, and the impact on the final result can vary greatly depending on factors like the rate, the frequency of application, and the duration involved. It’s important to know how each type works to accurately assess the financial implications of borrowing or investing under different conditions.

The primary types of interest are:

• Simple Interest: Calculated only on the principal amount, resulting in a linear growth over time.
• Compound Interest: Interest is calculated on both the principal and accumulated interest, leading to exponential growth.
• Continuous Interest: An advanced form where interest is compounded constantly over time, resulting in the highest possible growth.

Each of these types has a different effect on the growth of an investment or the cost of a loan. While simple interest is easier to calculate, compound interest results in greater accumulation, especially over longer periods. Understanding when to use each type can help you optimize your financial strategy, whether you’re saving or borrowing.

Simple vs Compound Interest Comparison

When comparing different interest structures, understanding the distinction between simple and compound interest is essential for making informed financial decisions. Both types determine how an initial amount grows or accrues over time, but they differ in how interest is applied and accumulated. This comparison helps to evaluate which option is more advantageous for a specific financial situation, whether you’re saving, borrowing, or investing.

Simple Interest Overview

Simple interest is straightforward. It is calculated solely on the original principal, meaning that interest is added to the principal at a fixed rate over each period. This type of interest results in a constant growth rate, as the interest does not compound or accumulate on the interest earned. It is often used in short-term loans or investments where the growth is intended to remain linear.

Key Characteristics:

• Fixed interest rate applied to the original amount.
• Linear growth without reinvestment of interest.
• Typically used for short-term financial agreements.

Compound Interest Overview

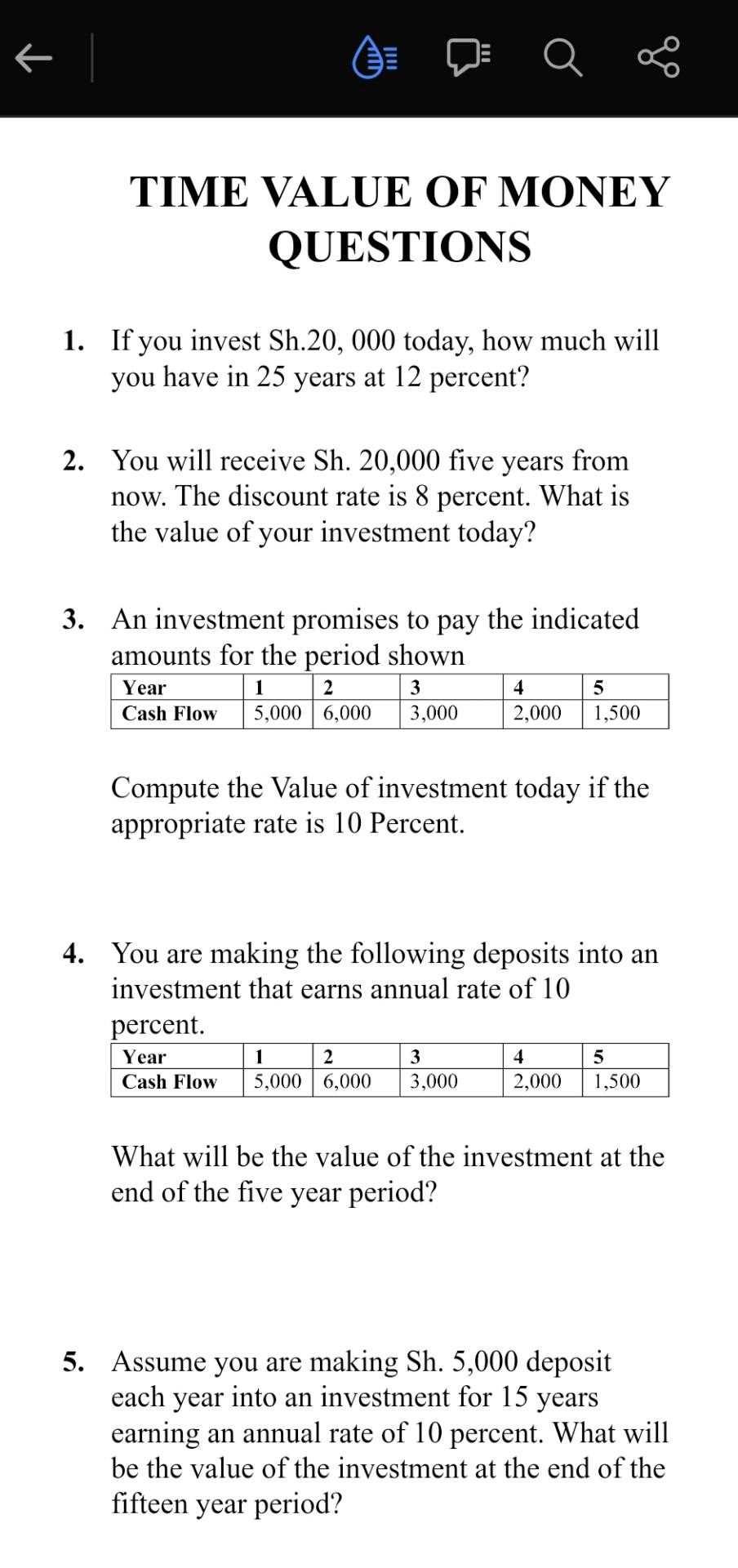
Compound interest, on the other hand, involves interest being calculated on both the initial amount and any accumulated interest. This leads to exponential growth, as the interest itself starts earning more interest. The more frequently interest is compounded, the greater the final value of the investment or loan. Compound interest is commonly used for long-term investments, savings, or loans where growth is desired over time.

Key Characteristics:

• Interest is applied to both the principal and accumulated interest.
• Exponential growth resulting from interest compounding over time.
• Preferred for long-term financial commitments.

In summary, simple interest offers predictable, linear growth, while compound interest can result in much higher returns or costs, especially over longer periods. The choice between the two depends on your financial goals, the length of time involved, and how the interest is structured in your particular agreement.

Solving for Unknown Variables in Problems

When working through financial problems, there are often unknown values that need to be determined in order to complete the calculation. These variables could represent the interest rate, the duration, the initial amount, or the future value, depending on the problem. Solving for these unknowns requires understanding the relationship between the known values and applying the correct formulas to isolate and solve for the missing variable.

The key to solving these types of problems is to rearrange the formula appropriately, ensuring that the unknown is on one side of the equation and all other terms are on the other. Once this is done, it’s a matter of performing the necessary arithmetic steps to arrive at the correct answer. Each variable plays a specific role in the overall calculation, so understanding how they interact is critical to accurately solving the problem.

To illustrate the process, let’s consider a common approach to solving for unknowns:

• Step 1: Identify the known and unknown values in the problem.
• Step 2: Select the appropriate formula based on the type of problem.
• Step 3: Rearrange the formula to isolate the unknown variable.
• Step 4: Perform the necessary calculations to find the unknown.

By following this systematic approach, you can confidently solve for the unknown values in various types of financial problems, whether it’s determining the future worth, present worth, or the interest rate involved in a transaction.

Practical Examples for Exam Preparation

To effectively prepare for any test involving financial concepts, working through practical examples is essential. Real-world scenarios allow you to apply theoretical knowledge to practical situations, helping to solidify your understanding of key principles. By solving a variety of problems, you can become more confident in your ability to tackle different types of calculations and scenarios.

Example 1: Calculating Future Value

Consider an individual who invests $1,000 at an annual interest rate of 5% for 5 years. Using the formula for compound interest, you can determine the future value of this investment. Let’s break down the steps:

• Initial investment: $1,000
• Interest rate: 5%
• Time period: 5 years
• Formula: Future Value = Principal × (1 + Rate)^Time
• Calculation: Future Value = 1,000 × (1 + 0.05)^5 = $1,276.28

This simple example helps reinforce how interest accumulates over time and how to apply the formula to calculate the final value.

Example 2: Finding the Present Value of a Loan

Suppose you need to calculate the present value of a $2,000 loan that will be repaid in 3 years with an annual interest rate of 6%. This example requires applying the formula for discounting future amounts back to the present value:

• Future payment: $2,000
• Interest rate: 6%
• Time period: 3 years
• Formula: Present Value = Future Value / (1 + Rate)^Time
• Calculation: Present Value = 2,000 / (1 + 0.06)^3 = $1,681.42

This example demonstrates how to adjust future sums to reflect their present worth, which is crucial for evaluating loans, investments, or other financial decisions.

Working through such examples will not only improve your problem-solving skills but also enhance your ability to understand and manipulate key financial concepts in real-world contexts.

Common Mistakes in Time Value Problems

While solving financial problems, there are several errors that can occur, especially when applying formulas and concepts incorrectly. These mistakes often arise from misunderstandings of key principles or simple miscalculations, and they can significantly affect the final result. Recognizing these common pitfalls can help you avoid them and improve your accuracy in solving such problems.

1. Misunderstanding the Interest Rate Period

One of the most frequent mistakes is using the incorrect interest rate for the period of time involved. It’s important to always match the rate to the correct time frame (annual, monthly, etc.). Failing to do so can result in inaccurate calculations. For example:

• Incorrect: Using an annual rate of 6% for a monthly calculation.
• Correct: Converting the annual rate to a monthly rate by dividing by 12.

2. Ignoring Compounding Frequency

Another common error occurs when the compounding frequency is ignored or misunderstood. When interest compounds more frequently than annually, the formula must account for this by adjusting both the rate and the number of periods. Forgetting to adjust for this can lead to substantial errors in your final result.

• Incorrect: Using the annual interest rate without considering compounding frequency.
• Correct: Adjust the rate and number of periods based on how often compounding occurs (e.g., monthly, quarterly).

3. Confusing Present and Future Value

Confusing the direction of the calculation (i.e., whether you are solving for present or future value) is another common mistake. This typically happens when interpreting the terms in the formula. Be careful to identify which amount is being calculated – whether it’s the current value of a future amount or the future value of a present amount.

• Incorrect: Applying the wrong formula based on the scenario.
• Correct: Ensure the correct formula is applied depending on whether you’re calculating the value now or in the future.

4. Overlooking the Time Factor

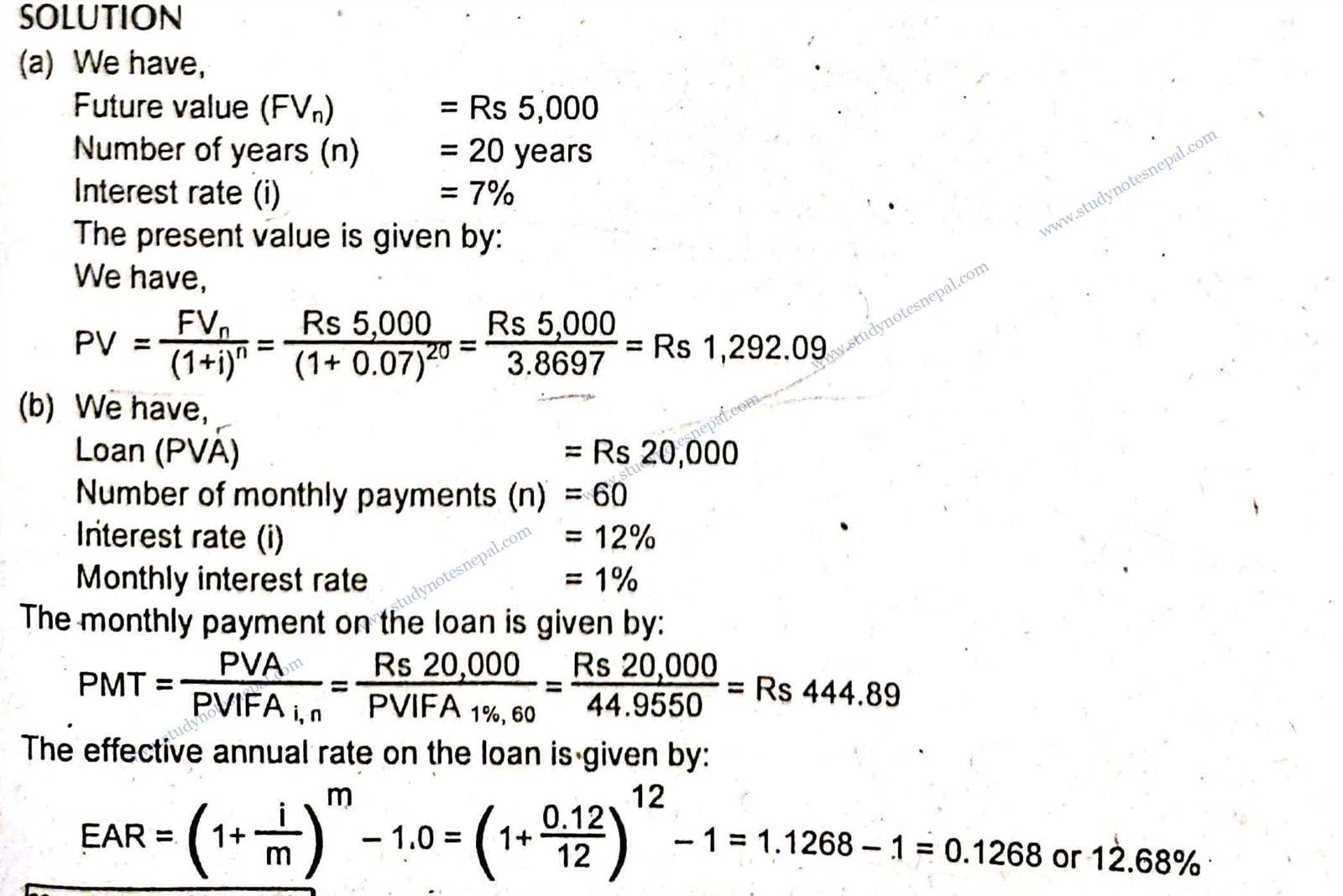
Some learners make the mistake of underestimating the impact of time on financial calculations. Time is a crucial factor that influences interest accumulation or discounting, and it should always be carefully considered when performing calculations.

• Incorrect: Using the wrong number of periods or misinterpreting the time frame.
• Correct: Always check the time period and adjust the calculation accordingly.

5. Rounding Too Early

Rounding intermediate values too early in the calculation can lead to inaccurate results. It’s better to carry out the full calculation first and only round the final answer to the desired precision.

• Incorrect: Rounding during intermediate steps.
• Correct: Round only the final result.

Avoiding these common errors can significantly improve your ability to solve financial problems accurately. By understanding the underlying concepts and double-checking your work, you can ensure that your calculations reflect the true values and lead to correct results.

Understanding Annuities and Perpetuities

In financial theory, both annuities and perpetuities represent structured series of payments made over time. While they share similarities, they differ in the duration of these payments and their calculations. Understanding the distinction between these two concepts is crucial for correctly applying them in various financial models and scenarios.

Annuities

An annuity is a series of payments made at regular intervals for a set period. These payments can either be made at the beginning or the end of each period, influencing the calculation of present and future amounts. Annuities are commonly used in retirement planning and loans.

• Fixed Payment: Payments are consistent throughout the duration of the term.
• Finite Duration: Annuities have a set end date after which payments cease.
• Types of Annuities: There are various types such as ordinary annuities, annuities due, and variable annuities.

Perpetuities

Perpetuities, on the other hand, represent a never-ending series of payments that continue indefinitely. These are often used in cases like government bonds or certain types of endowments. The key characteristic of a perpetuity is that the payments continue forever, making it unique compared to other financial instruments.

• Infinite Duration: Perpetuities do not have a set end date and continue indefinitely.
• Constant Payments: Payments remain the same over time and are not subject to change.
• Common Uses: Perpetuities are often seen in charitable endowments or some bond instruments.

Differences Between Annuities and Perpetuities

The primary difference between an annuity and a perpetuity lies in their duration. While annuities have a defined period for payments, perpetuities continue indefinitely. This distinction has a significant impact on their present and future value calculations. Annuities require calculations based on the number of periods, while perpetuities assume an infinite number of periods.

• Duration: Annuities have a finite period, while perpetuities continue forever.
• Calculation: Annuities use more complex formulas involving time, while perpetuities typically use simpler present value formulas.

Grasping the differences and the calculations behind these financial instruments is essential for making informed investment and financial decisions. Both annuities and perpetuities play critical roles in various financial strategies, and understanding how they work can provide greater insight into future projections and valuations.

Real-Life Applications of TVM Concepts

The concepts of valuing cash flows over time have widespread real-world applications in numerous industries. From personal financial planning to large-scale corporate decision-making, understanding how to assess the worth of future cash flows compared to their present equivalent is essential. These concepts are used to make informed choices about investments, loans, savings, and other financial strategies.

Investment Decisions

When individuals or companies evaluate investment opportunities, they often use time-based calculations to determine the profitability of an investment. Understanding the future benefits of an investment and comparing them to the initial cost is crucial in making smart decisions. For instance, when choosing between two projects, one might use present value analysis to determine which investment will yield better returns in the long run.

• Capital Budgeting: Companies assess large investments through discounted cash flow models to decide which projects to pursue.
• Stock Valuation: Investors use future cash flow projections to assess whether a stock is undervalued or overvalued.

Loans and Mortgages

When taking out a loan or mortgage, understanding how interest affects future payments is vital. Financial institutions often use time-based formulas to determine the amount to be repaid over the life of a loan. Knowing how to calculate the true cost of borrowing allows individuals to compare loan offers and select the best option based on their financial situation.

• Loan Amortization: Borrowers pay both principal and interest over time, and understanding this structure helps them plan repayments.
• Interest Rate Comparisons: Comparing different loan offers requires calculating the total cost of borrowing based on the interest rate and the repayment period.

Retirement Planning

For those planning for retirement, understanding how to grow savings over time is key to ensuring financial security. Retirement accounts often depend on compound growth, where interest on the initial investment accumulates over time. Being able to calculate how much needs to be saved today in order to meet future goals is an essential part of any retirement strategy.

• Retirement Fund Growth: Using compound interest to forecast how savings will accumulate over time.
• Withdrawal Planning: Calculating how much can be safely withdrawn from retirement accounts based on expected future returns.

By applying the concepts of future value, present value, and interest rate calculations, individuals and organizations can make more informed financial decisions that align with their goals. Whether for investing, borrowing, or saving, understanding these principles is critical in managing financial outcomes effectively.

Discounted Cash Flow Method in Practice

The discounted cash flow (DCF) method is widely used in finance to evaluate the attractiveness of an investment or project. This approach helps assess the present worth of expected future cash flows, considering the time-based factors that influence their value. By applying a discount rate, the future benefits of a project or investment are adjusted to reflect their present-day equivalent, enabling better decision-making in terms of profitability and risk management.

In practice, the DCF method is used across various fields, from evaluating business ventures to assessing the viability of individual projects. This technique enables companies and investors to understand whether the returns from a potential investment will justify the initial costs or the risks involved.

Steps in Applying the DCF Method

To use the DCF method effectively, several key steps are involved:

• Forecast Future Cash Flows: The first step involves estimating the expected cash flows for each period of the investment’s life cycle. These projections should account for factors like revenue, costs, taxes, and depreciation.
• Select a Discount Rate: The discount rate is typically chosen based on the risk level of the investment and the required rate of return. Commonly, this rate is based on the company’s cost of capital or the investor’s desired return.
• Calculate Present Value: The future cash flows are then discounted using the chosen rate, which converts them into their present value. This step is critical for comparing the value of money now with the value it would have in the future.
• Sum the Present Values: After discounting all future cash flows, the total present value of the investment is calculated by summing the individual present values.

Practical Example

Imagine a company considering a new project with expected cash flows of $50,000 annually for the next five years. If the discount rate is 8%, the DCF method would be used to determine the present value of those future cash flows. By applying the discount rate, the company can assess whether the future cash flows, adjusted for time, justify the initial investment required to undertake the project.

Using this method, investors and managers can compare different investment opportunities, accounting for varying levels of risk and return over time. The discounted cash flow method provides a clear and structured way to assess investments, making it an invaluable tool in both corporate finance and personal investment decisions.

Using Financial Calculators for Time Value

Financial calculators are powerful tools that simplify the complex calculations involved in evaluating the future worth of investments or determining the appropriate discount rate. These calculators help streamline the process of assessing how amounts change over different periods, making them indispensable for both professionals and students working with financial concepts. By inputting key variables such as cash flows, rates, and time periods, one can quickly obtain the necessary results without the need for extensive manual computation.

Using these tools, individuals can calculate various financial metrics such as the present value, future value, internal rate of return, and annuities. The calculators use preset formulas and time-based adjustments, ensuring accurate and quick results that would otherwise require much more time if done manually.

Key Features of Financial Calculators

Financial calculators come with several built-in functions that cater to the most common financial problems, including:

• Cash Flow Analysis: Allows users to input varying amounts over different periods to calculate present or future worth.
• Rate Calculation: Provides the option to find the rate of return or discount rate that equates cash flows over time.
• Amortization Schedules: Breaks down loans or investments into periodic payments, showing the principal and interest components.
• NPV and IRR Functions: Calculates the Net Present Value (NPV) and Internal Rate of Return (IRR), key indicators of investment viability.

Practical Example

Consider a scenario where an investor is looking to evaluate an investment project that offers $20,000 per year for the next five years. Using a financial calculator, the user can input these cash flows, the expected rate of return, and the time frame to calculate the present value of these future amounts. The calculator will instantly provide the result, saving the user time and effort.

Furthermore, financial calculators allow users to adjust for different variables like fluctuating rates or changing cash flow patterns, which are common in real-world scenarios. This flexibility ensures that financial decisions are based on accurate, real-time data, making these calculators invaluable tools in any financial analysis process.

Time Value of Money in Investment Decisions

In making investment decisions, understanding how future returns compare to current investments is crucial. The principle behind this concept helps investors assess the worth of an asset based on the potential cash flows it generates over time. This approach allows individuals and companies to determine whether a particular investment is worth pursuing by factoring in the impact of time on the value of returns.

By considering how money changes over periods, decision-makers can determine whether the return from a potential investment justifies its initial cost. This helps in comparing different opportunities and assessing their relative profitability, enabling investors to select the most promising options. Financial metrics such as the present value, future value, and internal rate of return are typically used in this analysis, guiding investors toward more informed choices.

Investment Evaluation Methods

There are several key methods used to evaluate investments, all of which rely on understanding how the value of returns evolves over time:

• Net Present Value (NPV): This method calculates the difference between the present value of cash inflows and outflows, helping investors determine if an investment is worthwhile. A positive NPV indicates a profitable investment.
• Internal Rate of Return (IRR): The IRR measures the rate at which an investment’s cash flows break even, allowing investors to compare different investment opportunities.
• Payback Period: This method evaluates how long it takes for an investment to recover its initial cost. Shorter payback periods are typically preferred in decision-making.

Real-World Application

Consider a real estate project that promises annual returns of $100,000 over the next ten years. By using financial tools that incorporate this principle, investors can calculate how much these returns are worth in today’s terms, accounting for factors such as inflation and opportunity cost. This calculation helps determine whether the project is a good choice when compared to alternative investment options.

Ultimately, this concept plays a pivotal role in evaluating the potential of investments, helping decision-makers prioritize opportunities that offer the highest return relative to their cost and associated risks. By accounting for the changing nature of value over time, investors can make better, more informed decisions about where to allocate resources.