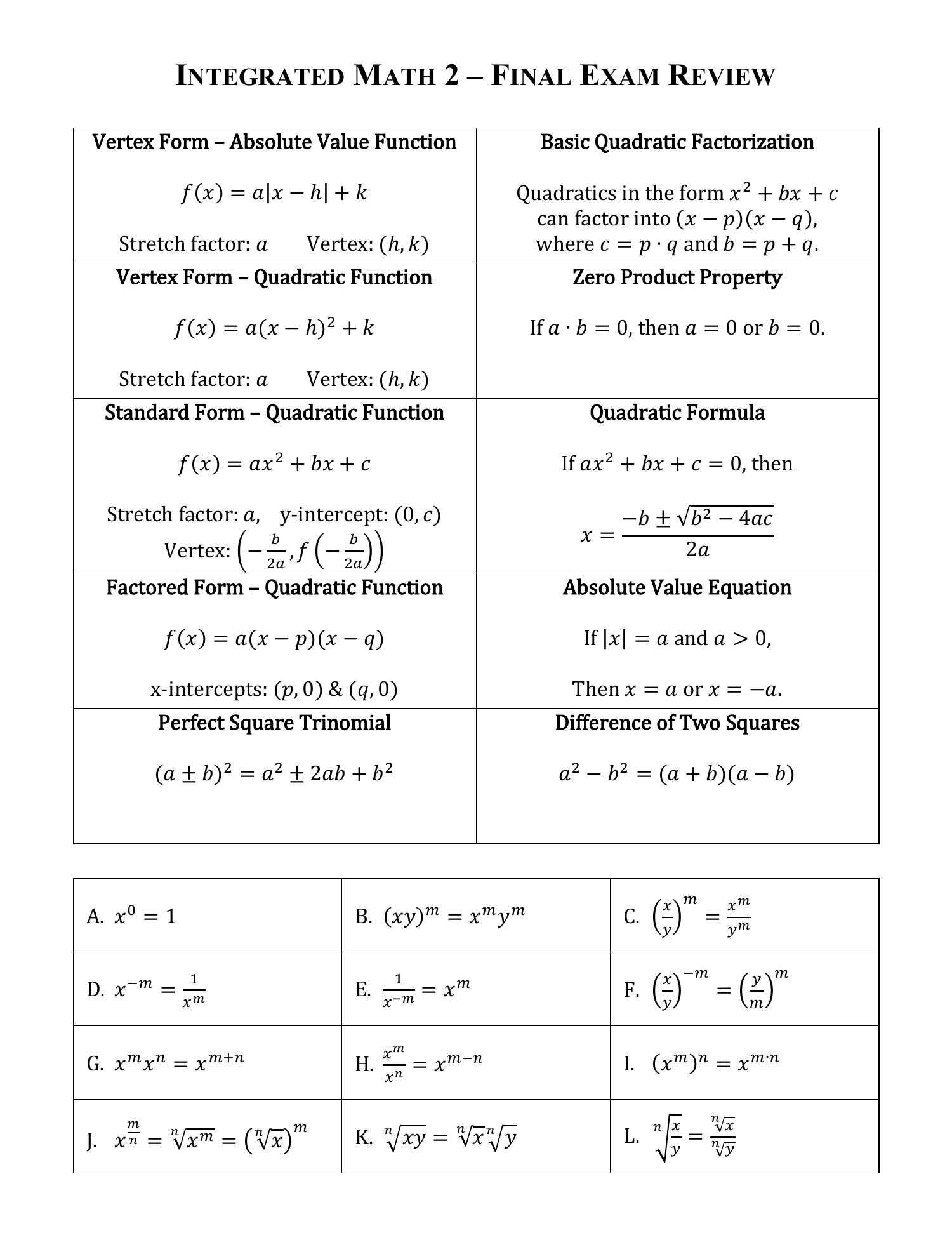
Algebra 2 Final Exam Review Semester 2

As the school year draws to a close, it’s time to consolidate your knowledge and ensure a strong understanding of the critical topics covered. The second half of the course brings together various mathematical principles, and being prepared is essential to achieving your best performance. Whether you’re reviewing specific techniques or reinforcing overall comprehension, this guide will help you strengthen your skills and boost your confidence.

Mastering key concepts requires more than just memorizing formulas; it involves a deep understanding of how different areas of mathematics connect and how to apply them to solve problems effectively. From solving equations to graphing complex functions, each topic builds on the previous, making a solid foundation essential for success.

With focused practice and strategic review, you can tackle the challenges ahead with ease. This article will provide you with essential tools to approach your upcoming assessments, offering detailed insights into each concept and practical tips to help you navigate any problem you might face.

Algebra 2 Key Concepts for Exam

To succeed in the upcoming assessment, it’s crucial to have a strong grasp of the core mathematical concepts that are essential for problem-solving. Each topic covered during the course is interconnected, requiring a comprehensive understanding of both fundamental and advanced techniques. The ability to apply these concepts to different types of questions will be key to achieving a high score.

Focus on mastering equation solving, graphing functions, and understanding the behavior of complex expressions. Recognize the importance of polynomial factoring and rational expressions, as they are commonly tested. Additionally, proficiency in manipulating exponents and logarithms is vital for tackling more advanced problems.

As you prepare, aim to reinforce your skills in systems of equations, and ensure you can comfortably work with both real and complex numbers. Understanding how to apply these concepts to word problems and theoretical scenarios will enhance your ability to solve problems effectively under time constraints.

Solving Quadratic Equations Efficiently

Quadratic equations are a fundamental part of mathematical problem solving, and learning how to solve them efficiently can save both time and effort during assessments. These equations typically involve variables raised to the power of two, and solving them requires an understanding of various methods to find their solutions. Mastering the most efficient techniques is essential for tackling these problems quickly and accurately.

Common Methods for Solving Quadratics

There are several methods for solving quadratic equations, each useful in different scenarios. Understanding when to apply each method is crucial for efficiency. The most common techniques include:

• Factoring: This method works best when the equation is easily factorable. It involves finding two binomials whose product equals the quadratic expression.
• Quadratic Formula: This universal method can be applied to any quadratic equation and provides a reliable way to find the roots of the equation.
• Completing the Square: Useful when the quadratic is not easily factored, this method transforms the equation into a perfect square trinomial, making it easier to solve.

Tips for Efficiently Solving Quadratics

To maximize efficiency and accuracy, keep these tips in mind when solving quadratic equations:

1. Always check if the equation can be factored first, as this is the quickest method.
2. If factoring is not possible, apply the quadratic formula to ensure you don’t miss any solutions.
3. When using the quadratic formula, be mindful of signs and carefully simplify the equation to avoid mistakes.
4. For equations that require completing the square, ensure you move the constant term to the other side of the equation before proceeding.

By practicing these methods and knowing when to apply each one, you’ll be able to solve quadratic equations efficiently and confidently.

Understanding Polynomial Functions

Polynomial functions play a central role in mathematics, offering a wide range of applications and solutions to real-world problems. These functions involve expressions with multiple terms, where each term is a product of a constant and a variable raised to a power. Understanding how these functions behave and how to manipulate them is crucial for tackling more complex mathematical challenges.

The degree of a polynomial and the coefficients of its terms significantly impact its graph and properties. A polynomial function can have one or more terms, and depending on its degree, it may exhibit a variety of behaviors, such as multiple turning points or specific end behavior. Recognizing these characteristics allows you to predict the graph’s shape and understand how the function will respond to different inputs.

Key to working with polynomial functions is knowing how to perform operations such as addition, subtraction, multiplication, and division. Additionally, factoring and solving polynomial equations are essential skills. These techniques can help you break down complex expressions and find the solutions or zeros of the function.

Factoring Techniques for Algebra 2

Factoring is an essential skill in solving polynomial equations and simplifying expressions. It involves rewriting an expression as the product of its factors, making it easier to find solutions and analyze the equation’s properties. Mastering different factoring methods is crucial, as some techniques work better with certain types of expressions than others.

Common Factoring Methods

There are several approaches to factoring polynomials, each suited to different situations:

• Factoring by Grouping: This method is useful when a polynomial has four terms. It involves grouping terms in pairs and factoring out the greatest common factor from each pair before factoring further.
• Difference of Squares: When a polynomial is the difference between two perfect squares, it can be factored as the product of two binomials. This method is quick and effective when applicable.
• Trinomial Factoring: Often used for quadratics, this method involves finding two numbers that multiply to the constant term and add to the middle coefficient, allowing you to factor the expression into two binomials.
• Sum or Difference of Cubes: Special formulas exist for factoring cubic expressions. These formulas can help break down cubic polynomials into simpler factors.

Tips for Efficient Factoring

To factor effectively, always begin by looking for the greatest common factor (GCF) in the polynomial. Once the GCF is factored out, the remaining terms are usually easier to work with. Additionally, be familiar with common patterns, such as the difference of squares and perfect square trinomials, which can help speed up the process.

Consistent practice with these techniques will help you factor complex expressions quickly and accurately, improving your problem-solving skills in future assessments.

Working with Rational Expressions

Rational expressions are fractions that involve polynomials in both the numerator and the denominator. These expressions are a crucial part of solving more advanced mathematical problems. Understanding how to manipulate and simplify rational expressions allows you to solve equations, evaluate functions, and perform algebraic operations with greater ease.

The process of working with rational expressions involves several key steps, including simplifying, adding, subtracting, multiplying, and dividing. Simplification typically requires factoring the numerator and denominator and canceling out common factors. When performing addition or subtraction, finding a common denominator is essential, just like with numerical fractions.

Multiplying and dividing rational expressions require a solid understanding of the rules of fractions. In multiplication, you multiply the numerators and denominators directly, while division involves multiplying by the reciprocal of the divisor. Ensuring that you correctly factor and simplify expressions before performing operations is key to avoiding mistakes and making calculations more manageable.

Exploring Exponential and Logarithmic Functions

Exponential and logarithmic functions are powerful tools in mathematics, often used to model real-world situations involving growth and decay. While these functions may seem complex at first, understanding their behavior and how to manipulate them is key to solving a variety of problems. Exponential functions involve repeated multiplication, while logarithmic functions are the inverse of exponentiation, providing a way to solve for exponents in equations.

When working with these functions, it is important to understand their key properties and the relationships between them:

• Exponential Growth and Decay: Exponential functions describe processes that increase or decrease rapidly, such as population growth or radioactive decay. The base of the exponential function determines the rate of change.
• Logarithmic Functions: These are the inverse of exponential functions, helping you solve equations where the unknown appears as an exponent. Logarithms simplify the process of dealing with large numbers and are often used in fields like science and engineering.
• Base of the Function: The base of an exponential or logarithmic function significantly affects its graph. Common bases include 2, 10, and the natural base e. Understanding how the base affects the graph’s shape and behavior is crucial.

Mastering these functions involves knowing how to graph them, solve equations involving them, and apply logarithmic properties to simplify expressions. Both functions have practical applications in fields ranging from finance to physics, making them essential for advanced problem-solving.

Mastering Systems of Equations

Solving systems of equations involves finding the values of multiple variables that satisfy more than one equation at the same time. These problems can be approached in several ways, depending on the complexity of the system and the type of equations involved. Mastering these methods is crucial for solving real-world problems where multiple conditions need to be satisfied simultaneously.

The most common approaches to solving systems of equations include:

• Substitution Method: This technique involves solving one equation for a single variable and substituting this expression into the other equation. It’s most useful when one of the equations is easily solvable for one variable.
• Elimination Method: This method involves adding or subtracting equations to eliminate one variable, making it easier to solve for the remaining unknowns. It’s particularly effective when the coefficients of one variable are the same or can be made the same.
• Graphing Method: This method involves graphing both equations on the same coordinate plane and identifying the point where the graphs intersect. While this method is intuitive, it can be less precise, especially when dealing with non-integer solutions.

Additionally, it’s important to recognize the different types of solutions a system can have. Some systems may have a unique solution, others may have infinitely many solutions, and some may have no solution at all. Understanding the nature of the system based on its equations can save time and guide your approach to solving it.

Analyzing Sequences and Series

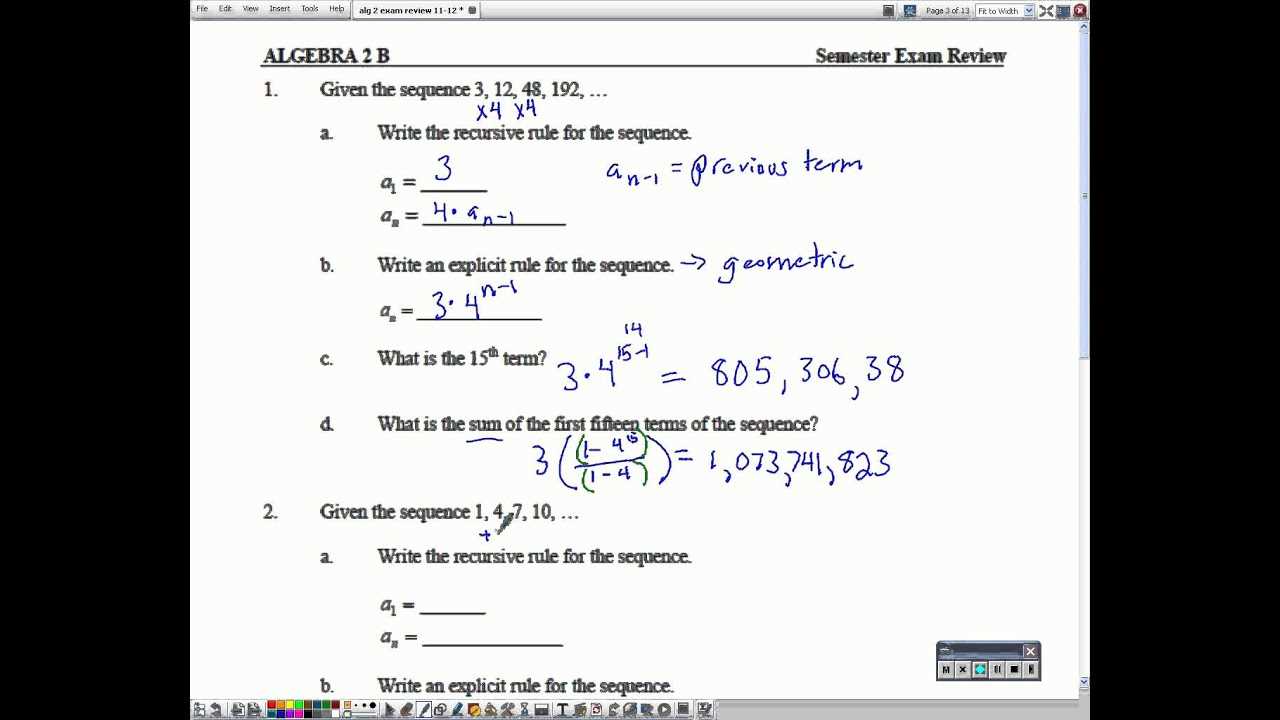
Understanding sequences and series is a key part of mathematical analysis. Sequences represent ordered lists of numbers that follow a specific pattern, while series are the sums of the terms of a sequence. These concepts are essential for solving problems related to patterns, growth models, and many real-world applications in areas like finance, physics, and computer science. Analyzing the behavior of sequences and summing the terms of series can provide valuable insights into the structure and limits of these mathematical constructs.

Types of Sequences

There are several types of sequences, each with distinct properties. The two most commonly studied types are:

• Arithmetic Sequences: In these sequences, the difference between consecutive terms is constant. For example, the sequence 2, 5, 8, 11, … is arithmetic because each term increases by 3.
• Geometric Sequences: These sequences have a constant ratio between consecutive terms. For example, 3, 6, 12, 24, … is a geometric sequence where each term is multiplied by 2.

Summing Series

Once a sequence is identified, the next step is often to find the sum of its terms, known as a series. There are specific formulas for summing both arithmetic and geometric series:

Series Type	Formula
Arithmetic Series	Sn = (n/2) * (2a + (n-1)d)
Geometric Series	Sn = a * (1 – rn) / (1 – r), r ≠ 1

These formulas allow you to quickly calculate the sum of the first n terms of a sequence, helping you to analyze patterns and solve problems more efficiently. Understanding how to use these formulas is essential when dealing with complex problems involving sequences and series.

Graphing Parabolas and Circles

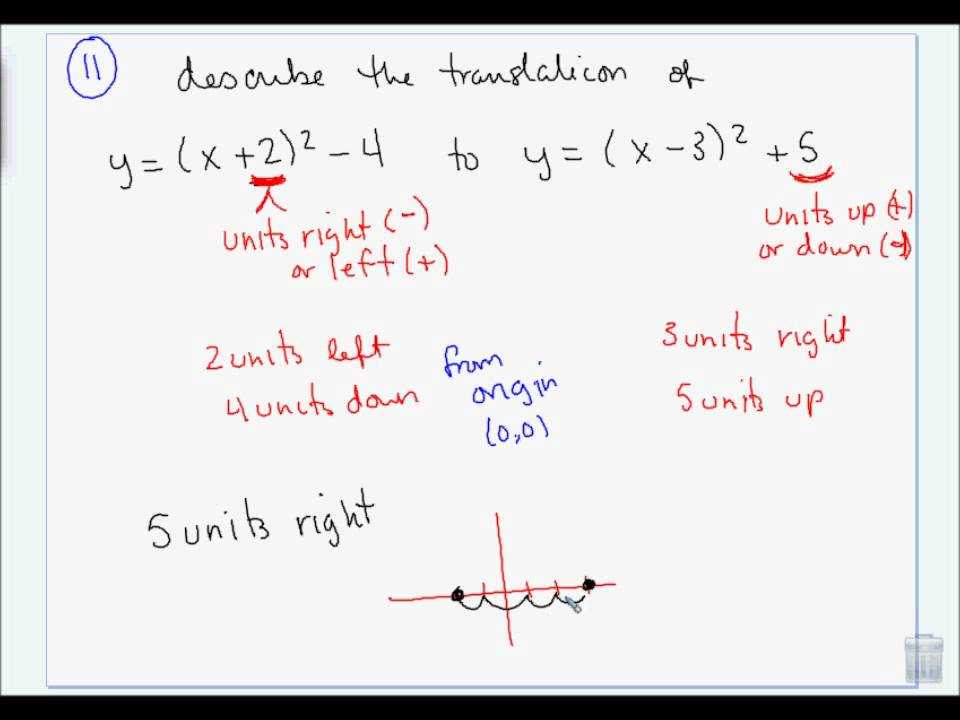
Graphing conic sections, such as parabolas and circles, is a fundamental skill in understanding geometric shapes and their properties. These curves have specific characteristics that can be easily identified and plotted on a coordinate plane. By recognizing the general forms of their equations, you can determine key features such as vertex, focus, radius, and center, which are crucial for accurate graphing and solving related problems.

Graphing Parabolas

A parabola is a U-shaped curve that can open upward, downward, left, or right, depending on the direction of its axis of symmetry. The general equation for a parabola is:

y = ax² + bx + c (for vertical parabolas) or x = ay² + by + c (for horizontal parabolas).

To graph a parabola, identify the following key features:

• Vertex: The highest or lowest point of the parabola, depending on whether it opens upward or downward.
• Axis of Symmetry: A vertical or horizontal line that divides the parabola into two mirror-image halves.
• Focus and Directrix: These determine the shape and direction of the parabola. The focus is a fixed point inside the curve, and the directrix is a line outside the curve.

Graphing Circles

A circle is a set of all points in a plane that are equidistant from a fixed point called the center. The general equation of a circle is:

(x – h)² + (y – k)² = r², where (h, k) represents the center and r is the radius.

To graph a circle, follow these steps:

• Find the center: This is given by the values (h, k) in the equation.
• Determine the radius: The radius is the square root of r².
• Plot the center and use the radius: From the center, move outward in all directions by the radius to draw the circle.

Understanding how to graph parabolas and circles helps in visualizing their behavior, solving related equations, and applying these concepts to real-world problems in physics, engineering, and other fields.

Complex Numbers and Their Operations

In mathematics, numbers are not limited to the familiar real numbers. The introduction of an imaginary component allows for the creation of a new number system that expands the range of solvable equations. These numbers are composed of a real part and an imaginary part, making it possible to solve problems that otherwise have no solution within the real number system.

Key Operations with Complex Numbers

When working with complex numbers, there are several essential operations that must be mastered. These include addition, subtraction, multiplication, and division. Each of these operations follows a set of rules that involve handling both the real and imaginary components separately.

• Addition: To add two complex numbers, simply add the corresponding real and imaginary parts. For example, (3 + 4i) + (2 + 5i) = 5 + 9i.
• Subtraction: Subtraction is performed similarly to addition. Subtract the real parts and imaginary parts separately. For example, (7 + 3i) – (4 + 2i) = 3 + i.
• Multiplication: Multiplying complex numbers involves distributing each part and using the rule that i² = -1. Example: (1 + 2i)(3 + 4i) = 3 + 4i + 6i + 8i² = 3 + 10i – 8 = -5 + 10i.
• Division: To divide complex numbers, multiply both the numerator and the denominator by the conjugate of the denominator. This eliminates the imaginary part from the denominator, simplifying the result.

Conjugates and Moduli

The conjugate of a complex number a + bi is a – bi. The conjugate is useful in simplifying complex expressions, particularly in division. Additionally, the modulus of a complex number represents its distance from the origin in the complex plane and is calculated using the formula:

modulus = √(a² + b²)

By mastering these operations and properties, you can confidently work with complex numbers and apply them in various areas such as engineering, physics, and advanced mathematics.

Applications of the Binomial Theorem

The Binomial Theorem is a powerful tool in mathematics, providing a way to expand expressions that involve powers of binomials. This theorem not only simplifies computations but also has wide-reaching applications in various fields, including probability, combinatorics, and calculus. By understanding how to apply it, you can solve complex problems that would otherwise be difficult or time-consuming.

The expansion of a binomial expression, such as (a + b)^n, involves finding terms that help express the polynomial in a more manageable form. The Binomial Theorem gives a systematic method for generating these terms, making it particularly useful when dealing with large exponents.

Expanding Powers of Binomials

One of the most direct applications of the Binomial Theorem is in expanding binomial expressions. For instance, the expansion of (x + y)^3 using the theorem results in:

(x + y)^3 = x³ + 3x²y + 3xy² + y³

This allows for quick, efficient calculation without the need for repeated multiplication, particularly when the exponent is large. The coefficients in the expansion correspond to the entries in Pascal’s Triangle, a pattern that makes the process even more accessible.

Applications in Probability

Another significant application of the Binomial Theorem is in probability theory, particularly when calculating the outcomes of binomial experiments. For example, in a series of trials where each trial has two possible outcomes (success or failure), the Binomial Theorem can be used to calculate the probability of getting a certain number of successes.

For instance, if the probability of success in a single trial is p and the number of trials is n, the probability of exactly k successes is given by:

P(k successes) = C(n, k) * p^k * (1 – p)^(n – k)

Where C(n, k) is the binomial coefficient, which can be computed using the Binomial Theorem.

In conclusion, the Binomial Theorem is not just a theoretical concept, but a practical tool that simplifies complex calculations and is essential in solving real-world problems, particularly in fields involving probabilities and statistical analysis.

Tips for Solving Inequalities

Solving inequalities is an essential skill for mastering various mathematical concepts. These problems often involve finding the range of values that satisfy a given condition rather than determining a specific solution. While solving inequalities may seem challenging at first, there are several strategies that can simplify the process and ensure accuracy.

One key to success is understanding how to manipulate inequalities while maintaining their integrity. This involves the same techniques used in solving equations, but with special attention to the direction of the inequality sign. Here are some effective tips to help you navigate these problems:

Key Strategies for Solving Inequalities

Tip	Explanation
Flip the Inequality Sign	When multiplying or dividing by a negative number, remember to reverse the direction of the inequality.
Isolate the Variable	Just like with equations, isolate the variable on one side of the inequality to simplify the problem.
Check for Extraneous Solutions	Always verify that your solution makes sense within the context of the inequality, especially when dealing with rational expressions.
Graph the Solution	For inequalities with one variable, graphing can help visualize the solution set and make it easier to interpret.
Use Test Points	When solving compound inequalities, use test points in different regions to determine which parts of the solution satisfy the inequality.

Example of Solving an Inequality

Consider the inequality: 2x + 3 > 7

To solve, follow these steps:

1. First, subtract 3 from both sides: 2x > 4
2. Then, divide both sides by 2: x > 2
3. The solution is all values greater than 2, represented as (2, ∞).

By applying these tips and strategies, solving inequalities becomes a more systematic process. With practice, you will become more efficient and accurate in finding the solution sets to a variety of inequality problems.

Understanding Conic Sections in Detail

Conic sections are a class of curves that are formed by the intersection of a plane and a double-napped cone. These curves are not only foundational in geometry but also have various applications in science, engineering, and physics. Understanding the different types of conic sections, their properties, and how to work with them is essential for mastering many advanced mathematical concepts.

There are four primary types of conic sections: circles, ellipses, parabolas, and hyperbolas. Each of these shapes has unique characteristics and equations that define them. The key to mastering conic sections lies in recognizing how their general forms relate to the geometric figure they represent. Let’s explore each of these shapes in more detail.

Circle

A circle is a set of all points that are equidistant from a central point. The equation of a circle can be written as:

x² + y² = r²

Where r represents the radius, and the center of the circle is at the origin (0, 0) in the simplest form. Circles have applications in areas ranging from navigation to architecture.

Ellipse

An ellipse looks like a stretched circle and is defined as the set of all points where the sum of the distances from two fixed points, called foci, is constant. The general equation for an ellipse is:

(x - h)² / a² + (y - k)² / b² = 1

Where a and b are the lengths of the major and minor axes, and (h, k) represents the center. Ellipses appear in planetary orbits and in the design of various optical systems.

Parabola

A parabola is a curve that results from the intersection of a plane with a cone at a specific angle. The general form of the equation for a parabola is:

y = ax² + bx + c

Where a, b, and c are constants. Parabolas are common in projectile motion and satellite dishes, as their shape helps focus light or signals on a single point.

Hyperbola

A hyperbola consists of two separate curves that open away from each other. It is the result of a plane intersecting both nappes of a cone. The equation of a hyperbola is:

(x - h)² / a² - (y - k)² / b² = 1

Where a and b determine the distance between the vertices and foci. Hyperbolas are used in navigation and in the analysis of certain types of waveforms.

Understanding the characteristics and equations of conic sections allows for better manipulation and solving of problems in geometry and algebra. These curves have deep connections to real-world applications and are essential in a variety of scientific and technological fields.

Behavior of Rational Functions

Rational functions are mathematical expressions formed by dividing two polynomials. The behavior of these functions can vary greatly depending on the structure of the numerator and denominator. Understanding how these functions behave is essential for analyzing their graphs and solving related problems effectively.

The key to understanding rational functions lies in identifying important features such as vertical and horizontal asymptotes, intercepts, and domain restrictions. These characteristics provide insight into the overall behavior of the function as the input values approach certain critical points or infinity.

Asymptotes and Discontinuities

Asymptotes represent lines that the graph of a rational function approaches but never crosses. There are two types of asymptotes to consider:

• Vertical Asymptotes: These occur where the denominator of the rational function equals zero but the numerator does not. This results in the function approaching infinity as it gets closer to the vertical line.
• Horizontal Asymptotes: These describe the behavior of the function as the input value approaches infinity. A rational function may approach a constant value as x increases or decreases.

Intercepts and Domain

Intercepts are points where the graph of the function intersects the axes:

• x-intercept: The point where the function equals zero, which occurs when the numerator is zero (provided the denominator is not zero).
• y-intercept: This is the value of the function when x = 0. To find the y-intercept, substitute x = 0 into the function.

The domain of a rational function is the set of all possible input values for which the function is defined. This is restricted by any values of x that cause the denominator to become zero, leading to vertical asymptotes or discontinuities.

By understanding these key aspects of rational functions, you can better predict their behavior and effectively solve problems involving them. Whether dealing with graphing, solving equations, or analyzing real-world scenarios, a solid grasp of rational function behavior is essential for success.

Functions and Their Inverses

Understanding how functions and their inverses interact is crucial for solving many types of mathematical problems. A function takes an input, processes it through a rule, and produces an output. The inverse of a function essentially reverses this process, mapping the output back to the original input. Recognizing when a function has an inverse and how to compute it is vital for both graphing and algebraic manipulations.

What is a Function Inverse?

A function inverse undoes the work of the original function. If a function f maps input x to output y, the inverse function f⁻¹ maps y back to x. Mathematically, this means that applying the original function followed by its inverse brings you back to the original value. This relationship is expressed as:

• f(f⁻¹(y)) = y and f⁻¹(f(x)) = x

Conditions for Inverses to Exist

Not all functions have inverses. For a function to have an inverse, it must be one-to-one, meaning that each output corresponds to only one input. This ensures that the function does not “repeat” any values. To determine if a function is one-to-one, you can use the horizontal line test. If any horizontal line intersects the graph of the function at more than one point, the function does not have an inverse.

Once you’ve determined that a function has an inverse, there are various methods for finding it. For simple functions, you can solve the equation y = f(x) for x and then swap the roles of x and y to get the inverse function. In more complex cases, graphical and numerical methods may be needed.

Knowing the relationship between a function and its inverse not only helps in solving equations but also aids in understanding how certain mathematical operations, such as transformations and symmetries, behave. Whether you’re working with linear, quadratic, or more advanced functions, this concept is a foundation for many areas of mathematics.

Probability and Statistics in Algebra 2

Understanding probability and statistics is essential for making sense of data and making informed predictions. These concepts explore how likely certain outcomes are and how data can be analyzed to find patterns and make decisions. By combining mathematical reasoning with real-world applications, probability and statistics offer powerful tools for analyzing uncertainty and variability.

Key Concepts in Probability

Probability measures the likelihood of an event occurring. It is expressed as a number between 0 and 1, where 0 indicates an impossible event, and 1 indicates a certain event. Some key principles to understand in probability include:

• Experiment: A process that leads to a set of outcomes, like flipping a coin or rolling a die.
• Event: A specific outcome or a set of outcomes from an experiment.
• Sample Space: The set of all possible outcomes in an experiment.
• Probability of an Event: The ratio of the number of favorable outcomes to the total number of possible outcomes.

To calculate the probability of simple events, use the formula:

• P(Event) = Number of favorable outcomes / Total number of outcomes

Understanding Statistics

Statistics involves collecting, analyzing, interpreting, and presenting data. Key concepts include measures of central tendency and spread:

• Mean: The average of a data set, found by summing all values and dividing by the number of values.
• Median: The middle value of a data set when the values are arranged in order.
• Mode: The value that appears most frequently in a data set.
• Range: The difference between the highest and lowest values in a data set.

In addition, understanding probability distributions, such as normal distributions, helps in analyzing data and making predictions about future events.

Probability and statistics are not only essential for solving theoretical problems, but also for interpreting data in fields ranging from business and economics to science and medicine. By mastering these concepts, you can better assess risks, predict trends, and make well-informed decisions based on data.

Trigonometric Identities Review

Trigonometric identities are fundamental tools used to simplify expressions and solve problems involving angles and their relationships. By understanding and applying these identities, one can manipulate trigonometric functions to find unknown values, solve equations, and make complex calculations more manageable. These identities serve as shortcuts for transforming one trigonometric expression into another, making them essential for solving a wide range of problems in mathematics.

Basic Trigonometric Identities

The foundation of trigonometry lies in several core identities that relate the basic trigonometric functions to one another. The most essential are:

• Reciprocal Identities: These express each trigonometric function as the reciprocal of another:
  • sin(θ) = 1/csc(θ)
  • cos(θ) = 1/sec(θ)
  • tan(θ) = 1/cot(θ)
• Pythagorean Identities: These are based on the Pythagorean theorem and describe the relationships between sine, cosine, and tangent functions:
  • sin²(θ) + cos²(θ) = 1
  • 1 + tan²(θ) = sec²(θ)
  • 1 + cot²(θ) = csc²(θ)

Sum and Difference Identities

Sum and difference identities allow you to express trigonometric functions of sums or differences of angles as products or simpler functions. These are especially useful when simplifying expressions or solving equations involving multiple angles. The key identities are:

• Sum of Sines: sin(A + B) = sin(A)cos(B) + cos(A)sin(B)
• Difference of Sines: sin(A – B) = sin(A)cos(B) – cos(A)sin(B)
• Sum of Cosines: cos(A + B) = cos(A)cos(B) – sin(A)sin(B)
• Difference of Cosines: cos(A – B) = cos(A)cos(B) + sin(A)sin(B)

By mastering these identities, one can significantly simplify complex trigonometric expressions and solve problems more efficiently. Whether dealing with angles in right triangles, unit circles, or wave functions, these identities provide a systematic way to handle and manipulate trigonometric functions in a variety of contexts.

Preparing for Word Problems on the Exam

Word problems often challenge students to apply their mathematical understanding to real-world situations. The key to solving these problems is to carefully analyze the information provided, identify the relationships between different quantities, and then choose the appropriate mathematical techniques to find a solution. By practicing strategies for breaking down complex scenarios into manageable parts, you can improve your ability to approach these questions with confidence and accuracy.

To successfully solve word problems, it’s important to follow a structured approach:

• Read Carefully: Take the time to understand the problem. Look for important details such as units of measurement, relationships between quantities, and any conditions or restrictions that may apply.
• Identify What is Being Asked: Focus on the question being asked. What are you trying to find? This will guide your solution process.
• Define Variables: Assign variables to the unknowns. This step allows you to translate the problem into a mathematical expression or equation.
• Set Up the Equation: Based on the relationships described in the problem, write the equation(s) that will help you solve for the unknowns. Ensure the equation accurately represents the situation described.
• Solve and Check: Solve the equation, then check your work by verifying that your solution makes sense within the context of the problem.

Practicing with a variety of word problems will help you become more comfortable with different types of questions. The more you practice, the better you will become at identifying patterns and applying the right techniques to solve them efficiently.