Algebra 2 Semester 1 Final Exam Answers

Mathematics can often seem like a challenging subject, especially when it involves complex formulas, equations, and problem-solving techniques. As students approach the end of their course, it’s essential to review the core concepts that will appear on the assessment. This section will help guide you through the critical topics you need to focus on, ensuring that you’re well-prepared for the upcoming challenges.

The main goal is to build a strong understanding of the material, so that you can approach each question with confidence and clarity. By breaking down difficult topics and identifying patterns, you’ll improve your ability to solve problems efficiently and accurately. Let’s explore the key areas that often require extra attention and how mastering these can make all the difference.

Algebra 2 Semester 1 Final Exam Answers

Preparing for the end-of-course assessment requires a deep understanding of the topics covered throughout the term. This section highlights the essential concepts and techniques that are crucial for achieving success when it comes time to tackle the test. By reviewing the material thoroughly, students can ensure they’re fully equipped to approach the challenges with confidence.

The key to mastering the subject lies in practicing problem-solving strategies and becoming familiar with the types of questions that are likely to appear. By honing skills in topics such as solving equations, graphing functions, and understanding relationships between variables, students can perform effectively under test conditions. This guide provides insight into the most frequently tested areas, giving you the tools to navigate each problem with precision.

Overview of Algebra 2 Exam Structure

The structure of this important assessment is designed to evaluate a student’s comprehension and application of the key concepts covered throughout the course. It typically consists of a variety of question types that assess both conceptual understanding and problem-solving abilities. The format is structured to test knowledge in multiple areas, ensuring that students can demonstrate their ability to apply learned skills in different contexts.

In general, the assessment includes a mix of multiple-choice questions, short-answer problems, and sometimes longer, more complex questions that require detailed solutions. Each section is focused on specific topics, such as solving equations, graphing functions, and interpreting mathematical relationships. The goal is to ensure that students are not only able to recall information but also use it effectively to solve real-world problems.

Key Topics Covered in Algebra 2

This section highlights the fundamental areas of study that form the backbone of the course. Each topic is crucial for developing a solid understanding of mathematical principles, and mastering these concepts will significantly impact your success on the assessment. These topics range from basic equations to more complex functions and transformations that require a deeper level of problem-solving.

Core Mathematical Concepts

Throughout the course, several key areas are explored in depth. These include working with functions, graphing, solving systems of equations, and understanding various types of expressions. A strong grasp of these subjects is necessary for tackling more advanced material as well as real-life applications.

Topic	Key Skills
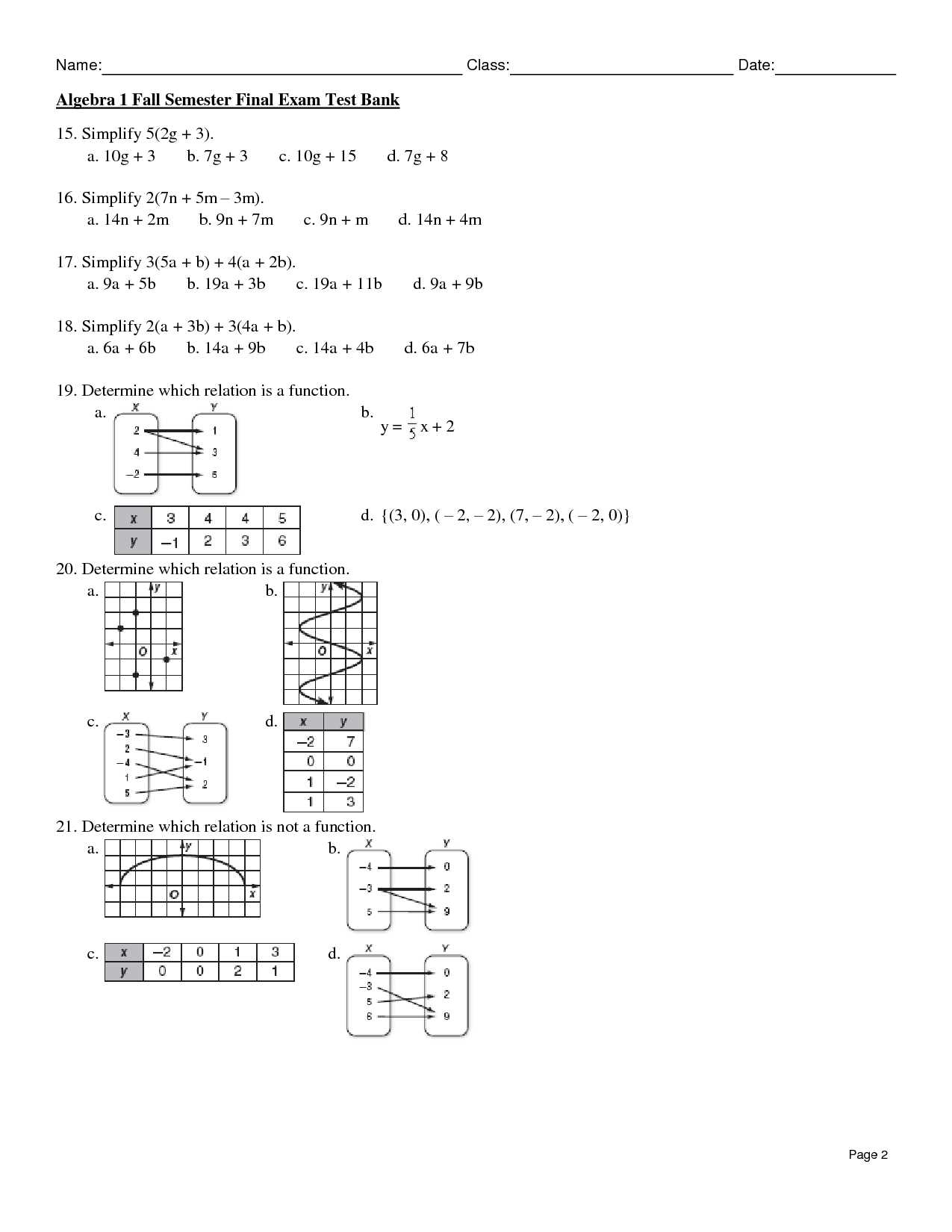
Linear Equations	Solve, graph, and interpret solutions
Quadratic Functions	Factor, solve, and analyze graphs
Rational Expressions	Simplify, solve, and apply
Exponential Functions	Evaluate, graph, and solve equations
Systems of Equations	Solve using substitution and elimination

Advanced Topics and Applications

As students progress, the course delves into more intricate areas such as logarithmic functions, conic sections, and complex numbers. These topics not only build upon foundational knowledge but also introduce methods that have broader uses in higher mathematics and practical scenarios.

Understanding Quadratic Equations

Quadratic equations are among the most important concepts in mathematics, often serving as a foundation for more advanced topics. These equations take the form of a polynomial of degree two and involve variables raised to the second power. Understanding how to solve and analyze these equations is essential for tackling more complex problems and applications.

To solve a quadratic equation, there are several methods that can be used, each suited to different types of problems. Below are the most common approaches:

• Factoring: Breaking down the equation into simpler binomials, making it easy to find the solutions.
• Quadratic Formula: A universal formula that can be used to solve any quadratic equation, even when factoring is not possible.
• Completing the Square: A method where you manipulate the equation to form a perfect square trinomial, simplifying the solving process.

In addition to solving quadratic equations, it’s crucial to understand their graphical representation. Quadratic functions form a parabola when graphed, and the solutions to the equation correspond to the points where the graph intersects the x-axis. These points are called the roots of the equation. The shape of the parabola is determined by the leading coefficient of the quadratic term.

1. Direction of the Parabola: If the coefficient of the squared term is positive, the parabola opens upwards; if negative, it opens downwards.
2. Vertex: The vertex represents the highest or lowest point of the parabola, depending on its direction.
3. Discriminant: The value under the square root in the quadratic formula, which indicates the nature of the roots (real, repeated, or imaginary).

By mastering the different methods and understanding the properties of quadratic equations, students can solve a wide range of problems efficiently and with confidence.

Systems of Linear Equations Explained

Solving systems of linear equations is an essential skill in mathematics that involves finding the values of variables that satisfy multiple equations simultaneously. These systems can represent real-world problems, such as determining the point where two lines intersect or solving for quantities in different scenarios. Understanding how to solve these systems is crucial for tackling a wide range of mathematical challenges.

There are several methods used to solve these systems, each suited to different types of problems:

• Substitution Method: Solve one equation for one variable, then substitute that expression into the second equation to find the other variable.
• Elimination Method: Add or subtract the equations to eliminate one of the variables, making it easier to solve for the remaining variable.
• Graphing Method: Graph both equations on the same coordinate plane and find the point where the lines intersect, which represents the solution.

Each method has its advantages and is useful in different situations. For example, the substitution method is often most effective when one equation is easy to manipulate, while the elimination method is quicker when the coefficients of one variable are already aligned. The graphing method provides a visual representation, which can be helpful for understanding the relationship between the equations.

Once the system is solved, the solution can be interpreted as the values of the variables that satisfy all the equations. In some cases, a system may have no solution (if the lines are parallel and never intersect) or an infinite number of solutions (if the equations represent the same line).

Exponential Functions and Their Use

Exponential functions are an important class of mathematical expressions that model growth or decay processes. These functions are commonly used to represent situations where quantities increase or decrease at a constant rate over time, such as population growth, radioactive decay, or financial interest. Understanding how to work with these functions is key to solving problems involving exponential changes.

Key Properties of Exponential Functions

Exponential functions have distinct characteristics that set them apart from other types of functions. The general form of an exponential function is y = a * b^x, where a is a constant, b is the base of the exponential (usually a positive real number), and x is the exponent. The behavior of the graph depends on the value of the base b: if b is greater than 1, the function represents growth, and if b is between 0 and 1, it represents decay.

Applications of Exponential Functions

Exponential functions are widely applied in various fields. For example:

• Population Growth: Exponential models can predict how a population grows over time under ideal conditions.
• Compound Interest: In finance, exponential functions are used to calculate compound interest, where the investment grows at a rate proportional to its current value.
• Radioactive Decay: The amount of a radioactive substance decreases exponentially over time.

By understanding the properties and applications of exponential functions, students can better analyze and model real-world situations that involve rapid change. Mastering these functions will help in solving a wide range of mathematical and practical problems.

Polynomials and Their Properties

Polynomials are a fundamental concept in mathematics, commonly used to express relationships between variables and constants in a variety of contexts. These expressions consist of terms that are combined using addition, subtraction, and multiplication, where each term includes a variable raised to a non-negative integer power. Understanding the properties of polynomials is crucial for solving equations, analyzing graphs, and modeling real-world phenomena.

Key Characteristics of Polynomials

Polynomials are defined by several important features, including the degree, leading coefficient, and the number of terms. The degree of a polynomial is determined by the highest power of the variable present in the expression. The leading coefficient is the coefficient of the term with the highest degree, which greatly influences the shape and behavior of the graph. The number of terms in a polynomial can vary, leading to different types of polynomials:

• Monomial: A polynomial with only one term.
• Binomial: A polynomial with two terms.
• Trinomial: A polynomial with three terms.

Graphing and Solving Polynomial Equations

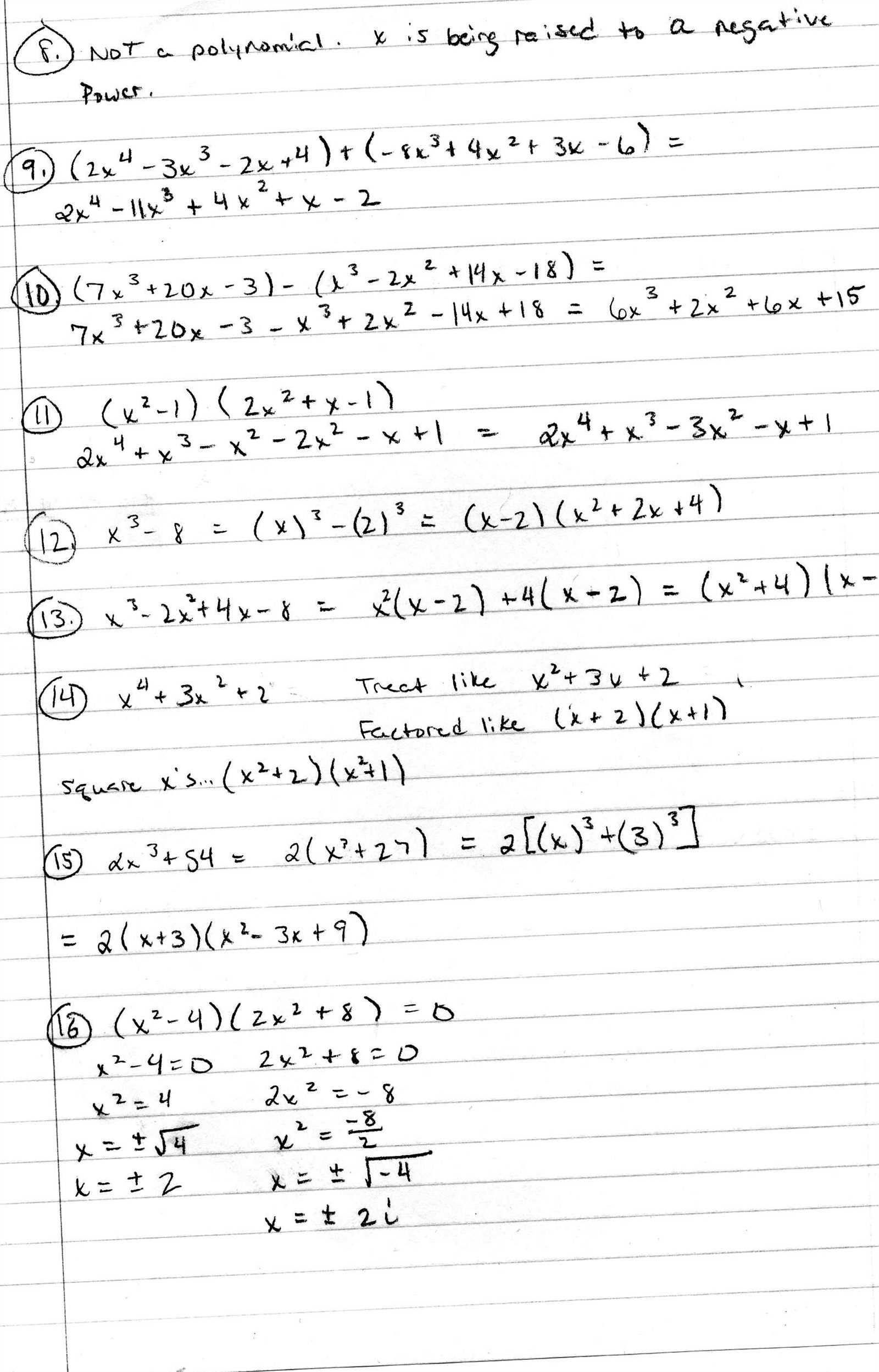
When graphed, polynomials exhibit specific behaviors based on their degree and leading coefficient. For instance, polynomials of even degree have graphs that either open upwards or downwards, while those of odd degree have graphs that extend in opposite directions. The solutions to polynomial equations, or roots, are the values where the graph intersects the x-axis. These roots can often be found through factoring or using the quadratic formula in the case of quadratics.

By analyzing the properties of polynomials, such as their degree and the signs of their coefficients, students can gain a deeper understanding of how these functions behave, allowing for more effective problem solving and graphical analysis.

Factoring Techniques for Algebra 2

Factoring is a key skill that simplifies the process of solving polynomial equations. By expressing a polynomial as a product of its factors, you can find solutions more easily and recognize patterns in complex expressions. Mastering factoring techniques is crucial for solving higher-degree equations and simplifying expressions in a variety of mathematical contexts.

Common Factoring Methods

There are several methods used to factor polynomials, each applicable depending on the structure of the expression. Below are some of the most commonly used techniques:

• Greatest Common Factor (GCF): Identify the largest factor that divides all terms in the polynomial and factor it out.
• Difference of Squares: Factor expressions in the form a² – b² into (a + b)(a – b).
• Trinomial Factoring: Factor quadratics in the form ax² + bx + c by finding two numbers that multiply to ac and add to b.
• Factoring by Grouping: Group terms in pairs, factor out the GCF from each pair, and then factor out the common binomial.

Example Table: Factoring Techniques

Polynomial	Method	Factored Form
x² – 9	Difference of Squares	(x + 3)(x – 3)
2x² + 5x + 3	Trinomial Factoring	(2x + 3)(x + 1)
x² + 5x + 6	Trinomial Factoring	(x + 2)(x + 3)
x² + 6x + 8	Factoring by Grouping	(x + 2)(x + 4)

Each of these techniques is suited to different types of polynomials. By practicing these methods, you’ll gain confidence in simplifying complex expressions and solving equations more efficiently.

Working with Rational Expressions

Rational expressions are fractions where both the numerator and denominator are polynomials. These expressions are fundamental in mathematics and are used to represent relationships that involve division between algebraic terms. Understanding how to manipulate rational expressions is essential for simplifying complex problems, solving equations, and analyzing mathematical relationships more effectively.

Working with rational expressions often involves simplifying, adding, subtracting, multiplying, and dividing them. The process typically starts with factoring both the numerator and denominator, then reducing the expression to its simplest form by canceling out common factors. Additionally, special attention must be given to the restrictions placed on the variable to avoid division by zero.

Simplifying Rational Expressions

To simplify a rational expression, follow these steps:

1. Factor both the numerator and denominator completely.
2. Cancel out any common factors between the numerator and denominator.
3. Write the simplified expression.

For example, the expression (x² – 9) / (x² – 6x + 9) can be simplified as follows:

• Factor the numerator: (x² – 9) = (x + 3)(x – 3)
• Factor the denominator: (x² – 6x + 9) = (x – 3)(x – 3)
• Cancel the common factor of (x – 3).

This results in the simplified expression: (x + 3) / (x – 3).

Adding and Subtracting Rational Expressions

Adding and subtracting rational expressions require a common denominator. To add or subtract, first find the least common denominator (LCD) and then adjust the numerators accordingly. Once the numerators are combined, simplify the result by factoring and canceling common terms.

For example, to add 1/(x + 2) and 2/(x – 3), the LCD is (x + 2)(x – 3). The numerators are adjusted and then combined to form a single rational expression.

Mastering rational expressions allows you to simplify complex algebraic fractions and solve equations more efficiently, making it an essential skill for higher-level mathematics.

Solving Absolute Value Equations

Absolute value equations involve expressions where the variable is inside absolute value symbols. The absolute value of a number represents its distance from zero on the number line, regardless of direction. When solving such equations, it’s important to account for both the positive and negative solutions, as the absolute value of a number is always non-negative.

To solve absolute value equations, you typically set up two separate cases: one where the expression inside the absolute value equals the positive value, and another where it equals the negative value. Solving each case individually will yield the potential solutions. It is also important to check the solutions against the original equation to verify their validity.

Steps to Solve Absolute Value Equations

Follow these steps to solve an absolute value equation:

1. Isolate the absolute value expression on one side of the equation.
2. Set up two separate equations: one where the expression equals the positive value and one where it equals the negative value.
3. Solve both equations.
4. Check the solutions to ensure they satisfy the original equation.

Example: Solving an Absolute Value Equation

Consider the equation |x – 3| = 5. To solve:

• Set up two equations: x – 3 = 5 and x – 3 = -5.
• Solve the first equation: x = 8.
• Solve the second equation: x = -2.
• The solutions are x = 8 and x = -2.

Both solutions satisfy the original equation, as |8 – 3| = 5 and .

By carefully considering both positive and negative solutions, you can effectively solve equations involving absolute values, ensuring a thorough understanding of their behavior in different contexts.

Mastering Radical Expressions

Radical expressions involve the use of roots, such as square roots, cube roots, and higher-order roots. These expressions are essential for solving various types of problems in mathematics, especially when dealing with polynomial equations, geometric calculations, and real-world applications. Mastering the manipulation and simplification of radical expressions is crucial for advanced problem-solving skills.

Working with radical expressions requires an understanding of the rules for simplifying them, including rationalizing the denominator, combining like terms, and transforming expressions into simpler forms. By following these principles, you can simplify complex radical expressions and make them easier to handle in more advanced mathematical contexts.

Simplifying Radical Expressions

To simplify a radical expression, look for factors inside the radical that are perfect squares (or higher powers, depending on the root). Extract these factors from the radical and simplify the expression accordingly.

• For example, √36 simplifies to 6 because 36 is a perfect square.
• Similarly, √(4x²) simplifies to 2x.
• In more complex expressions, factor out perfect squares from the radicand and simplify each factor separately.

Rationalizing the Denominator

When a radical expression appears in the denominator of a fraction, it is often useful to rationalize the denominator. This involves multiplying both the numerator and denominator by the same radical to eliminate the root from the denominator.

• For instance, to rationalize 1/√2, multiply both the numerator and denominator by √2, resulting in √2/2.
• By rationalizing the denominator, you ensure that the denominator is a rational number, which makes the expression easier to work with in subsequent steps.

By mastering these techniques, you can confidently simplify, manipulate, and combine radical expressions, making them much more manageable and ready for use in further calculations.

Complex Numbers in Algebra 2

Complex numbers extend the concept of numbers beyond the real number system, introducing a new dimension to solving equations that don’t have real solutions. These numbers are made up of two parts: a real component and an imaginary component. The ability to work with complex numbers is essential in solving higher-level equations and in understanding many phenomena in engineering, physics, and other fields.

The imaginary unit, denoted as i, represents the square root of -1, a concept that allows us to define and manipulate complex numbers. By combining real numbers and imaginary numbers, we can solve equations that previously had no solution within the real number system.

Structure of Complex Numbers

A complex number is expressed in the form a + bi, where:

• a is the real part of the number.
• b is the coefficient of the imaginary part.
• i represents the imaginary unit, where i² = -1.

For example, 3 + 4i is a complex number where 3 is the real part and 4 is the imaginary part. The operations involving complex numbers, such as addition, subtraction, multiplication, and division, are based on combining like terms and applying the properties of i.

Operations with Complex Numbers

When performing arithmetic with complex numbers, you treat the real and imaginary parts separately:

• For addition and subtraction, combine the real parts and the imaginary parts independently.
• For multiplication, apply the distributive property and use the fact that i² = -1.
• For division, multiply both the numerator and denominator by the conjugate of the denominator to eliminate the imaginary part from the denominator.

For instance, to multiply (2 + 3i)(4 – i), use the distributive property:

• 2(4) + 2(-i) + 3i(4) + 3i(-i) = 8 – 2i + 12i – 3
• Simplifying: 5 + 10i

Complex numbers provide a powerful way to solve equations that involve non-real solutions, and their manipulation opens the door to more advanced concepts in mathematics and other scientific fields.

Graphing Functions and Transformations

Graphing functions is a fundamental skill in mathematics, helping to visualize relationships between variables and understand the behavior of different mathematical expressions. By plotting a function on a coordinate plane, we can observe patterns, analyze trends, and make predictions. However, many functions require specific adjustments to their basic form, known as transformations, to represent more complex behaviors.

Transformations modify the graph of a function in various ways, such as shifting, stretching, reflecting, or compressing it. These changes allow for a more versatile understanding of how a function behaves under different conditions and can be applied to a wide range of mathematical contexts, from simple linear functions to more advanced expressions like quadratics and exponentials.

Types of Transformations

There are several key types of transformations that affect the graph of a function:

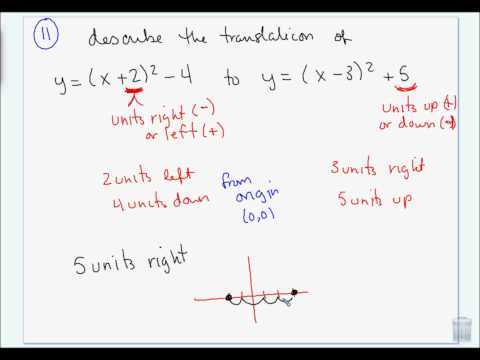
• Translation: This involves shifting the graph horizontally or vertically without altering its shape. A positive or negative value added to the function’s variable results in horizontal translation, while modifying the constant term shifts it vertically.
• Reflection: Reflecting the graph flips it across a specific axis. A reflection over the x-axis changes the sign of the output, while a reflection over the y-axis changes the sign of the input.
• Stretching and Shrinking: These transformations change the size of the graph. Stretching pulls the graph away from the axis, while shrinking compresses it towards the axis. This can be achieved by multiplying the function by a constant.

Example of a Transformation

For instance, consider the function f(x) = x², which is a simple parabola. By applying different transformations:

• Translation: The function f(x) = (x – 2)² shifts the graph 2 units to the right.
• Reflection: The function f(x) = -x² reflects the graph over the x-axis.
• Stretching: The function f(x) = 2x² stretches the graph vertically by a factor of 2.

Understanding these transformations is key to mastering how functions behave in various contexts. By combining different transformations, one can manipulate graphs to model complex real-world scenarios and further study mathematical phenomena.

Conic Sections Overview

Conic sections are curves that arise from the intersection of a plane and a cone. These geometric shapes are fundamental in mathematics and physics, as they model various natural phenomena and mathematical structures. Depending on the angle at which the plane intersects the cone, different conic sections are formed. Each type of conic section has distinct properties and can be used to represent a wide range of real-world situations, such as the orbits of planets or the shape of a satellite dish.

The main types of conic sections include circles, ellipses, parabolas, and hyperbolas. Each one is unique in its structure and equation, yet they all share common mathematical principles. Understanding these curves and their equations is essential in solving problems related to geometry, physics, and engineering.

Types of Conic Sections

The following table summarizes the key characteristics of the four primary conic sections:

Conic Section	Equation	Shape	Properties
Circle	x² + y² = r²	Round, all points equidistant from the center	Symmetrical, constant radius
Ellipse	(x² / a²) + (y² / b²) = 1	Oval, with two axes of symmetry	Foci define the shape, sum of distances to foci is constant
Parabola	y = ax² + bx + c	U-shaped, can open upwards or downwards	Symmetrical around a single axis, focus and directrix determine shape
Hyperbola	(x² / a²) – (y² / b²) = 1	Two separate curves, opening away from each other	Asymptotes define the shape, difference of distances to foci is constant

Applications of Conic Sections

Conic sections are not only theoretical objects but also have numerous applications in real-world scenarios. For example:

• Circles are often used in design and architecture for their symmetry and aesthetic properties, such as in the design of wheels or circular structures.
• Ellipses describe the orbits of planets and satellites, following Kepler’s laws of planetary motion.
• Parabolas appear in the design of reflectors and satellite dishes, where they focus incoming signals to a single point.
• Hyperbolas are seen in the paths of objects moving at high speeds, such as certain types of astronomical phenomena or in navigation systems.

By mastering the equations and properties of these curves, students can gain a deeper understanding of both pure and applied mathematics. Whether in science, engineering, or technology, conic sections provide valuable insights into the behavior of various systems and structures.

Probability and Statistics in Algebra

Probability and statistics play a crucial role in understanding and analyzing data. These mathematical concepts help in making predictions and interpreting the likelihood of events occurring. They also provide tools for organizing and summarizing large sets of information, allowing for better decision-making based on data. In various real-world applications, from risk management to scientific research, these topics are indispensable.

When studying data relationships and patterns, two fundamental areas are explored: probability theory and statistical analysis. Probability helps us assess the chances of specific outcomes, while statistics involves collecting, organizing, and interpreting data to make informed conclusions. Both areas are often integrated into mathematical models to provide deeper insights into various phenomena.

Key Concepts in Probability

• Probability of Events – The likelihood that a specific event will occur, expressed as a number between 0 and 1.
• Conditional Probability – The probability of an event occurring given that another event has already occurred.
• Independent and Dependent Events – Events that either do or do not influence each other’s outcomes.
• Permutations and Combinations – Counting methods used to calculate possible outcomes in a given scenario.

Statistical Analysis Tools

• Mean – The average of a set of numbers, calculated by summing all values and dividing by the total number of values.
• Median – The middle value in a set of data when it is ordered from least to greatest.
• Mode – The value that appears most frequently in a data set.
• Standard Deviation – A measure of the spread or variability of a set of values.

These concepts are interconnected and essential for understanding the behavior of random events and data sets. By mastering these principles, students can gain a deeper understanding of statistical analysis and its practical applications in various fields, from economics to healthcare.

Preparing for the Assessment

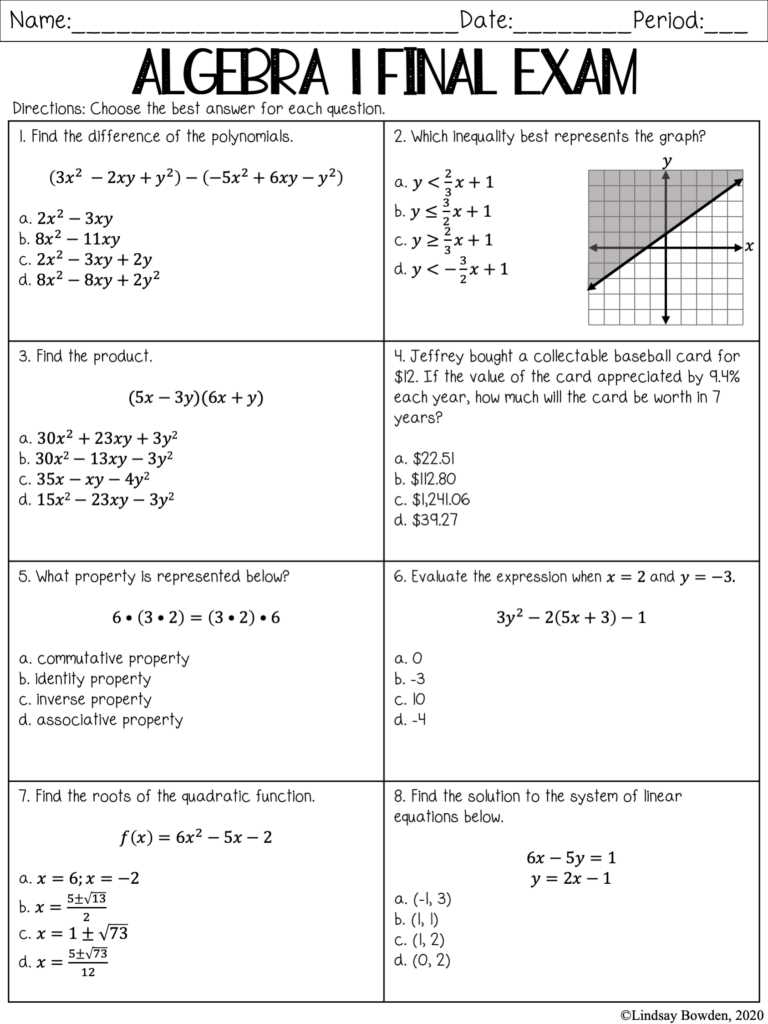
Effective preparation for any significant assessment involves thorough review, practice, and strategy. Understanding the material, practicing problem-solving techniques, and ensuring readiness for a variety of question types are all key steps. It is essential to identify areas of weakness and strengthen those through focused study. Organizing study sessions and following a structured plan will help increase confidence and reduce anxiety as the date approaches.

In order to succeed, students should approach their preparation methodically, breaking down the material into manageable sections. Prioritizing the most important concepts and practicing with sample questions can help build familiarity with the content and the format of the test. Additionally, reviewing past assignments, quizzes, and class notes can provide insight into areas that may require additional attention.

Study Tips for Success

• Review Key Concepts: Focus on the most important topics covered in the course and ensure a clear understanding of them.
• Practice Regularly: Solve sample problems and work through practice tests to become comfortable with the types of questions you might encounter.
• Group Study: Discussing concepts with peers can provide new insights and reinforce your own understanding.
• Identify Weak Areas: Take time to focus on topics that you find challenging or confusing. Seek help if needed.

Test-Taking Strategies

• Manage Your Time: Allocate time to each section based on its difficulty and importance. Make sure to leave time for review at the end.
• Stay Calm: Try to remain calm and focused during the assessment. If you get stuck on a question, move on and come back to it later.
• Answer What You Know First: Start with the questions you are most confident about, which will help build momentum.
• Review Your Work: If time allows, check your answers for mistakes and ensure you haven’t missed any important details.

By following these preparation strategies and practicing regularly, students can maximize their performance and feel more confident going into the test. Consistent effort and careful planning will lead to better results and greater success in mastering the material.

Common Mistakes to Avoid

When preparing for a significant assessment, it’s crucial to be aware of common errors that can hinder performance. Mistakes often occur due to misunderstandings of key concepts, rushed problem-solving, or neglecting to carefully follow instructions. By recognizing these pitfalls early and addressing them, students can improve their accuracy and increase their chances of success. Below are some of the most frequent missteps to watch out for during preparation and the actual assessment process.

Frequent Pitfalls to Avoid

Common Mistake	How to Avoid It
Misinterpreting the Problem	Always read each question carefully and make sure you understand what is being asked before attempting to solve it.
Skipping Steps	Show all your work and avoid skipping intermediate steps. This helps ensure accuracy and makes it easier to catch errors.
Overlooking Negative Signs	Be especially cautious with negative signs, particularly when multiplying or dividing. Double-check each calculation.
Not Reviewing Answers	If time permits, go back and review your answers to catch simple mistakes and confirm your solutions.
Failing to Manage Time	Allocate time for each section and ensure you have a few minutes at the end for a final review.

Tips for Avoiding Common Mistakes

• Read Carefully: Take time to understand each question before starting, ensuring you’re solving the right problem.
• Practice Methodically: The more you practice solving problems step by step, the less likely you are to overlook important details.
• Stay Organized: Keep your work neat and well-organized. It’s easier to spot errors if everything is clearly laid out.
• Check Your Work: If possible, go over your solutions to ensure you haven’t made any mistakes. Verify the logic and calculations used.

Avoiding these common mistakes will help streamline your approach to problem-solving and improve your overall performance. Careful attention to detail, thorough practice, and time management are key to succeeding in the assessment.

Helpful Resources for Algebra 2

When tackling complex mathematical topics, having access to the right tools and resources can make a significant difference in understanding and mastery. Various materials are available to help reinforce key concepts, practice problem-solving techniques, and clarify difficult topics. These resources are designed to complement your study and provide valuable support as you work through challenging problems.

Online Platforms and Websites

Numerous websites offer interactive lessons, practice exercises, and video tutorials to help deepen your understanding of advanced mathematical concepts. These platforms provide a flexible and convenient way to learn at your own pace.

• Khan Academy: A free platform offering in-depth lessons and practice problems, perfect for reinforcing foundational concepts.
• Desmos: An online graphing calculator that allows you to visualize functions and equations, aiding in understanding their behavior.
• Wolfram Alpha: A computational tool that solves problems and explains step-by-step solutions, useful for both practice and clarification.
• PatrickJMT: A YouTube channel featuring short and clear tutorials on various mathematical topics, from basics to more advanced techniques.

Books and Study Guides

Books and study guides provide structured lessons, practice questions, and solutions to guide you through complex concepts. These resources are ideal for focused study sessions and often include tips and strategies for effective problem-solving.

• The Complete Idiot’s Guide to Mathematics: A beginner-friendly guide that explains concepts in simple terms with plenty of examples.
• Scholastic Study Guides: A well-organized resource offering practice problems and review sections to reinforce learning.
• Practice Problem Books: Books dedicated to providing a wide variety of practice problems with step-by-step solutions for deeper understanding.

By using these resources, you can gain a stronger grasp of difficult material, enhance your problem-solving skills, and be better prepared to apply your knowledge effectively.