Integrated Math 1 Semester 1 Final Exam Review Answers

As you approach the conclusion of this academic period, it’s crucial to consolidate your understanding of key concepts. This section is designed to help you focus on the most important topics you’ll encounter in the upcoming assessment. Whether you’re aiming to reinforce your knowledge or fill in any gaps, a strategic approach will ensure you’re well-prepared.

Mastering fundamental principles is the foundation of success. It’s important to revisit the core ideas that form the basis of more complex problems. Building confidence in these areas will allow you to tackle challenges with greater ease and efficiency.

Throughout this guide, we will explore effective techniques for solving various types of questions. From understanding equations to interpreting data, each section offers targeted advice to sharpen your skills. By the end, you’ll have a clearer picture of how to approach different types of tasks and maximize your performance.

Preparation for the Upcoming Assessment

This section focuses on providing guidance and practical solutions for the topics covered in the first part of your course. The aim is to help you consolidate your knowledge and refine your problem-solving techniques. By reviewing essential concepts and practicing key types of questions, you can approach the test with confidence and clarity.

For success, understanding the core principles behind various problems is crucial. Working through examples will give you a clear idea of how to apply these principles in different contexts. Focus on the processes, not just the results, and aim to master the steps needed to reach the correct solutions efficiently.

As you progress, take the time to revisit any challenging areas and seek out additional practice where needed. This approach will allow you to strengthen your foundation, making it easier to tackle more complex scenarios with a systematic approach.

Key Concepts to Focus On

In order to perform well, it is essential to concentrate on the foundational ideas that form the basis of more complex problems. Recognizing these core concepts will allow you to approach questions systematically and with confidence. By understanding the underlying principles, you will be better equipped to apply your knowledge effectively during the test.

Algebraic operations such as solving equations and simplifying expressions are central to many tasks. These skills will serve as the building blocks for more advanced topics, so ensure you’re comfortable manipulating variables and constants.

Another critical area is functions and their properties, including interpreting graphs and understanding how different functions behave. Mastering these concepts will help you navigate problems involving transformations, rate of change, and real-world applications.

Lastly, don’t overlook geometric principles, which are often tested in various contexts. Understanding shapes, angles, and measurements will give you the tools to solve problems efficiently and accurately.

Understanding Algebraic Expressions

Algebraic expressions are a fundamental aspect of problem-solving, as they represent relationships between quantities using variables, constants, and operators. Mastering these expressions allows you to translate real-world situations into mathematical language and solve them efficiently. A strong grasp of how to work with expressions is crucial for tackling a wide range of questions.

To begin, it’s essential to understand how to simplify expressions by combining like terms and applying the correct operations. This skill helps streamline problems and makes more complex tasks manageable. Recognizing patterns in expressions also aids in determining the appropriate methods for solving.

Another key point is knowing how to distribute and factor expressions. These processes often appear in more advanced topics, such as solving equations or working with polynomials. By practicing these techniques, you will be better equipped to handle a variety of algebraic challenges.

Tips for Solving Linear Equations

Solving linear equations is a key skill that forms the foundation for more complex mathematical tasks. The process involves finding the value of the unknown variable that satisfies the equation. With practice, you can develop a systematic approach to solving these types of problems efficiently.

Step-by-Step Approach

Begin by simplifying the equation. Combine like terms on both sides and eliminate any parentheses. Next, isolate the variable on one side of the equation by performing inverse operations. These steps will help you break down the problem and find the solution more easily.

Check Your Work

After finding the solution, always substitute the value back into the original equation to verify your answer. This step ensures accuracy and helps you avoid small mistakes that can lead to incorrect results.

Equation	Steps	Solution
2x + 4 = 10	Simplify: 2x = 6, then divide by 2	x = 3
3x – 5 = 16	Simplify: 3x = 21, then divide by 3	x = 7

Mastering Quadratic Equations

Quadratic equations represent a class of problems that often appear in various mathematical contexts. These equations involve terms where the variable is squared, and solving them requires a strategic approach. Understanding how to manipulate and solve these types of equations is essential for progressing to more advanced topics.

Methods for Solving Quadratics

There are several methods to solve quadratic equations, such as factoring, completing the square, and using the quadratic formula. Each approach has its advantages, depending on the form of the equation. Familiarity with all these techniques allows you to choose the most efficient method for any given problem.

Using the Quadratic Formula

The quadratic formula is one of the most reliable tools for solving equations in the form ax² + bx + c = 0. The formula is:

x = (-b ± √(b² – 4ac)) / 2a

By substituting the values of a, b, and c from the equation, you can easily find the solutions. This method is particularly useful when factoring is difficult or impossible.

How to Solve Word Problems

Word problems require you to translate real-world situations into mathematical expressions. These problems can seem challenging at first, but with a clear approach, they become manageable. The key is identifying the relevant information, understanding the relationships between different elements, and then applying the appropriate mathematical methods to solve them.

Identify the problem by reading the scenario carefully and pinpointing what is being asked. Look for key numbers, relationships, and any operations that may be involved, such as addition, subtraction, multiplication, or division. Once you’ve understood the situation, decide how to represent it mathematically.

Set up an equation based on the information provided. For example, if the problem involves a rate or a total amount, express it in terms of a variable. Then, solve the equation using the relevant techniques, whether it’s simple arithmetic or a more complex method, like solving a system of equations.

Functions and Their Graphs

Understanding functions and how they are represented visually is crucial for solving many types of problems. A function describes a relationship between two quantities, where each input corresponds to exactly one output. The graph of a function provides a visual representation of this relationship, helping to identify patterns and solve problems more efficiently.

Types of Functions

There are various types of functions, including linear, quadratic, and exponential. Each function has a unique graph, and understanding the shape and properties of these graphs allows you to interpret and solve problems more effectively. For example, linear functions create straight lines, while quadratic functions produce parabolas.

Interpreting Graphs

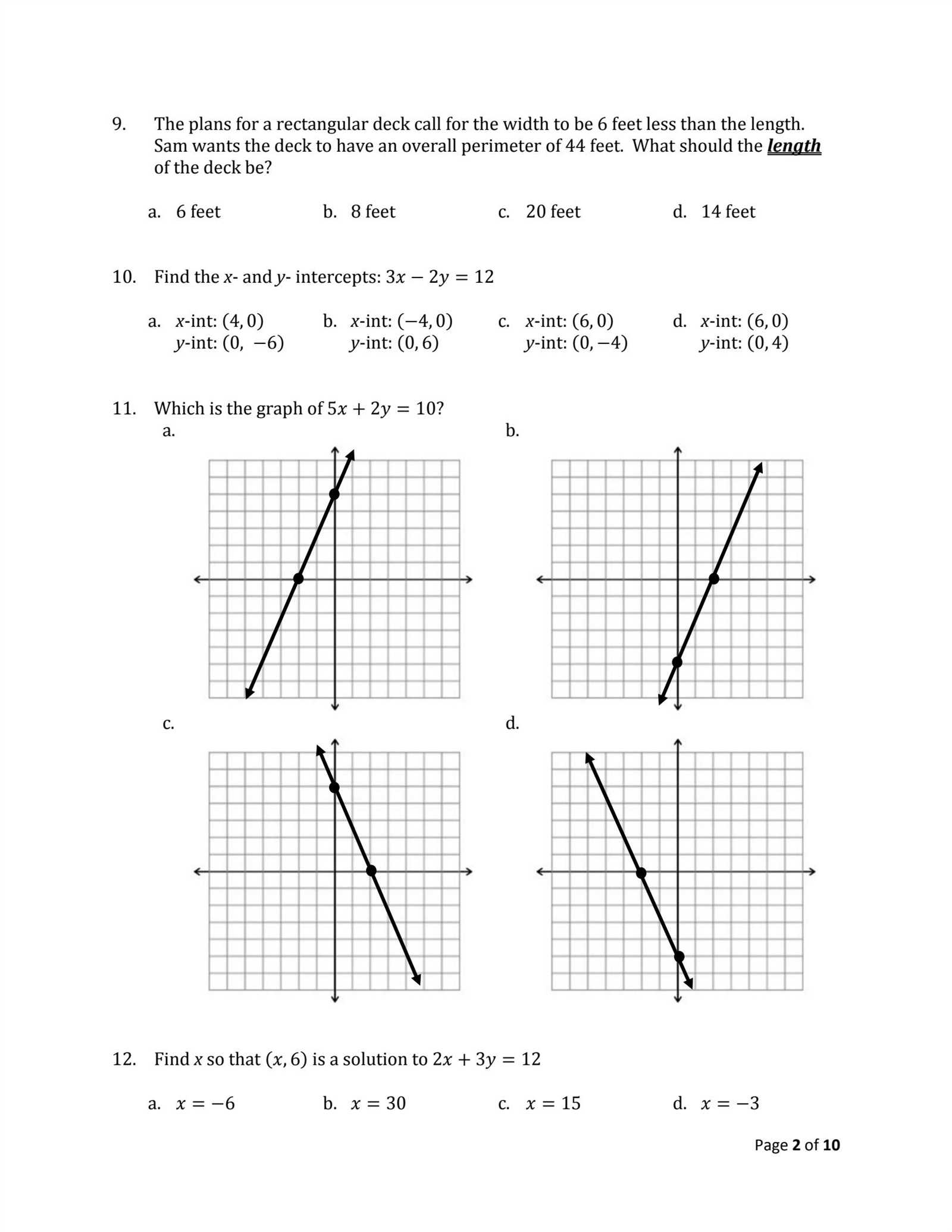
When working with graphs, focus on identifying key features such as intercepts, slopes, and asymptotes. These elements provide valuable information about the behavior of the function. For instance, the x-intercept represents the point where the function crosses the horizontal axis, and the slope shows how steep the line is in linear functions.

Working with Inequalities

In solving inequalities, the goal is to find the range of values that satisfy a given condition. Unlike equations that provide a single solution, inequalities represent a set of possible solutions. Understanding how to manipulate and interpret these conditions is crucial for solving many types of problems, especially when dealing with constraints or limits.

There are several important steps to follow when solving inequalities:

• Simplify both sides of the inequality by combining like terms and applying the correct operations.
• Isolate the variable on one side by using inverse operations. Be mindful of the inequality sign, especially when multiplying or dividing by negative numbers.
• Graph the solution to visually represent the set of solutions. For example, a solution may be represented as a range on a number line.

Special Considerations

• Reversing the inequality sign: When multiplying or dividing both sides by a negative number, always reverse the inequality symbol.
• Compound inequalities: When dealing with compound inequalities, break the problem into two separate parts and solve each one individually before combining the solutions.

By practicing these techniques, you can become more comfortable working with inequalities and applying them in a variety of problem-solving situations.

Strategies for Simplifying Polynomials

Simplifying polynomials is an essential skill that helps in solving algebraic problems efficiently. The process involves combining like terms, applying distributive properties, and sometimes factoring expressions to make them more manageable. With a systematic approach, you can break down complex polynomials into simpler forms that are easier to work with.

Combining Like Terms

The first step in simplifying a polynomial is to combine like terms. Like terms are terms that have the same variable raised to the same power. By adding or subtracting these terms, you reduce the polynomial to its simplest form. This process eliminates unnecessary complexity and makes further operations easier to handle.

Using the Distributive Property

Another important strategy is applying the distributive property, especially when dealing with expressions that involve parentheses. This property allows you to multiply each term inside the parentheses by the factor outside, simplifying the expression significantly.

Expression	Simplified Form
3x + 5x	8x
4(x + 2)	4x + 8
2x² + 3x – x² + 4x	x² + 7x

By mastering these techniques, you can simplify complex polynomials and prepare them for further operations like factoring or solving equations.

Understanding Systems of Equations

Systems of equations involve finding the values of variables that satisfy multiple conditions simultaneously. These systems often arise when dealing with real-world problems that have more than one relationship or constraint. Solving such systems means determining the values that make all equations true at the same time, which can be done using various methods.

There are several ways to approach solving a system of equations, such as substitution, elimination, and graphing. Each method provides a unique advantage depending on the form of the equations and the complexity of the problem. Understanding how and when to use each method can make solving these systems more efficient and straightforward.

Key Geometry Principles for the Exam

Geometry involves understanding shapes, their properties, and the relationships between their various elements. Key principles in this area provide the foundation for solving problems related to angles, lengths, areas, and volumes. Mastering these concepts is essential for tackling a wide range of questions that may appear on the test.

Some of the most important principles to focus on include the properties of triangles, circles, and polygons, as well as the Pythagorean theorem, which connects the sides of right-angled triangles. Understanding the relationships between different geometric figures will help you solve for missing dimensions, compute areas, and apply relevant formulas efficiently.

Trigonometry Basics for Beginners

Trigonometry is the branch of mathematics that deals with the relationships between the sides and angles of triangles. It is especially useful when working with right-angled triangles, allowing you to determine unknown sides or angles based on known values. Understanding the fundamental concepts of trigonometry can open up a wide range of problem-solving techniques, making it a valuable tool in many areas of study.

The three primary trigonometric ratios–sine, cosine, and tangent–are based on the relationships between the angles and sides of a right triangle. These ratios form the foundation for solving various types of problems involving angles and distances, both in theoretical and real-world applications. Mastering these basic principles can help you tackle more complex trigonometric problems with ease.

Using the Pythagorean Theorem Effectively

The Pythagorean theorem is a fundamental principle in geometry that helps determine the length of a side in a right-angled triangle when the other two sides are known. It is an essential tool for solving problems related to distance, height, and space, providing a direct relationship between the sides of a right triangle. Mastering its use allows you to efficiently solve many geometric and real-world problems.

Key Steps for Applying the Theorem

To use the theorem effectively, follow these basic steps:

1. Identify the right triangle and label the sides: the two legs and the hypotenuse (the side opposite the right angle).
2. Apply the Pythagorean formula: a² + b² = c², where a and b are the legs, and c is the hypotenuse.
3. Substitute the known values into the formula to solve for the unknown side.
4. Ensure the units of measurement are consistent across the problem.

Common Applications

The Pythagorean theorem can be used in a variety of situations, including:

• Calculating the distance between two points on a coordinate plane.
• Finding the height of an object when the base and slant height are known.
• Determining the length of a diagonal in rectangular spaces such as rooms or buildings.

By practicing and applying this theorem regularly, you will improve your ability to solve problems quickly and accurately.

Practice with Rational Numbers

Rational numbers are numbers that can be expressed as the ratio of two integers, where the denominator is not zero. Understanding how to work with these numbers is crucial, as they appear frequently in various mathematical problems. Being able to perform operations such as addition, subtraction, multiplication, and division with rational numbers is essential for solving more complex problems in algebra and beyond.

Operations with Rational Numbers

Here are the basic steps for performing operations with rational numbers:

• Addition and Subtraction: When adding or subtracting fractions, make sure the denominators are the same. If they are different, find a common denominator first.
• Multiplication: Multiply the numerators and denominators directly. Simplify the result if necessary.
• Division: To divide by a fraction, multiply by its reciprocal (flip the fraction and multiply).

Common Challenges and Tips

Working with rational numbers can sometimes be tricky, especially when dealing with negative values or complex fractions. To improve accuracy and speed, practice simplifying fractions as much as possible before performing operations. Also, be mindful of signs when adding or subtracting negative numbers.

By consistently practicing operations with rational numbers, you’ll develop a solid understanding of how to handle them in more advanced mathematical contexts.

Interpreting Data and Graphs

Understanding how to interpret data and graphs is a vital skill that allows you to analyze information visually. Graphs are used to represent numbers and patterns in a concise way, making complex data easier to understand. By learning how to read and analyze various types of graphs, you can draw conclusions, make predictions, and solve problems more effectively. This section focuses on the key elements of interpreting different types of data visualizations.

Key Types of Graphs

Here are the common types of graphs you may encounter:

• Bar Graphs: Used to compare quantities across different categories. Pay attention to the scale and the height of the bars to understand the data being represented.
• Line Graphs: Ideal for showing trends over time. Focus on the slope and direction of the line to understand how the data is changing.
• Pie Charts: Used to represent parts of a whole. Each slice represents a percentage, so check for the proportions of each section to understand the relationship between parts.
• Histograms: Similar to bar graphs, but used to represent frequency distributions. Look for the distribution of data across different intervals to identify patterns.

Analyzing Data Trends

When interpreting data, it’s important to observe trends and patterns. Ask questions like:

• Is there a steady increase or decrease in the data over time?
• Are there any spikes or drops that stand out?
• What do the extremes in the data represent?
• Is the data consistent or are there noticeable fluctuations?

By understanding these trends, you can make informed decisions based on the information presented in the graph. Practicing the interpretation of different data sets will help you become more proficient in recognizing important patterns and making accurate conclusions.

Test-Taking Strategies for Success

Effective preparation and a calm, strategic approach during a test can significantly improve your performance. Having the right mindset and tools at your disposal ensures that you not only understand the material but also approach each question with confidence. This section will cover practical strategies to help you succeed during the test-taking process.

Preparation is Key

Before the test, it’s important to organize your study sessions. Focus on:

• Understanding Key Concepts: Review the most important topics that are likely to appear. Clarify any areas of confusion before the test date.
• Practice Problems: Solve practice questions to familiarize yourself with the test format and question styles.
• Time Management: Allocate specific time blocks for each subject or topic to ensure a balanced review.

During the Test

Once you are in the test environment, apply the following strategies:

• Read Instructions Carefully: Ensure you understand what is being asked before you start answering.
• Answer Easy Questions First: Begin with the questions you find easiest. This boosts your confidence and ensures you don’t miss points on familiar topics.
• Time Management: Keep track of time to avoid spending too much on any one question. If you get stuck, move on and return later if time allows.
• Double-Check Your Work: If you finish early, use the remaining time to review your answers for mistakes.

By using these strategies, you can maximize your efficiency and reduce anxiety during the test. The key to success lies in preparation, a clear mindset, and smart execution on test day.

Common Mistakes to Avoid During the Exam

During any assessment, it’s easy to make simple mistakes that can cost you valuable points. Recognizing common pitfalls and avoiding them can help you stay focused and perform at your best. This section highlights typical errors students make during tests and offers tips on how to prevent them.

Rushing Through the Questions

One of the most frequent mistakes is rushing through the questions without fully considering each one. While time is a factor, it’s essential to take your time and approach each problem thoughtfully. Avoid:

• Skipping important details: Always read the problem carefully and pay attention to specific instructions, especially if they affect how you solve the problem.
• Incorrect calculations: Rushed calculations can lead to simple arithmetic errors. Double-check your work whenever possible.

Neglecting to Show Your Work

Even if you’re confident in your solution, leaving out steps can hurt you, especially on complex problems. Remember to:

• Write down intermediate steps: This not only helps you stay organized but also earns partial credit if your final answer is incorrect.
• Check for common errors: For example, sign mistakes or misplacing decimals can happen if you skip over your work too quickly.

Misinterpreting the Question

Sometimes the most straightforward question can trip you up if it’s misunderstood. To prevent this, make sure to:

• Underline key terms: Focus on the crucial aspects of the question, such as “find the solution,” “simplify,” or “evaluate.”
• Clarify complex wording: If a question is unclear, take a moment to rephrase it in your own words before proceeding.

Leaving Questions Blank

Another common error is not attempting a question at all, either out of uncertainty or because you ran out of time. Even if you don’t know the full solution, try to:

• Make an educated guess: Eliminate obviously incorrect choices or work through a problem logically, even if you’re unsure of the final answer.
• Answer all questions: It’s always better to attempt a solution than to leave a blank, especially if there’s no penalty for wrong answers.

By avoiding these common mistakes, you can improve your accuracy, confidence, and overall performance. Be mindful, take your time, and ensure each response is your best effort.