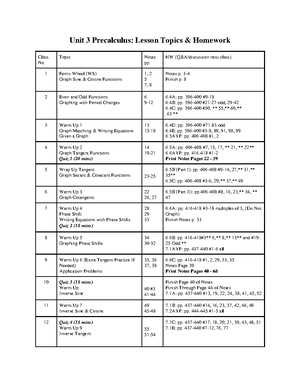
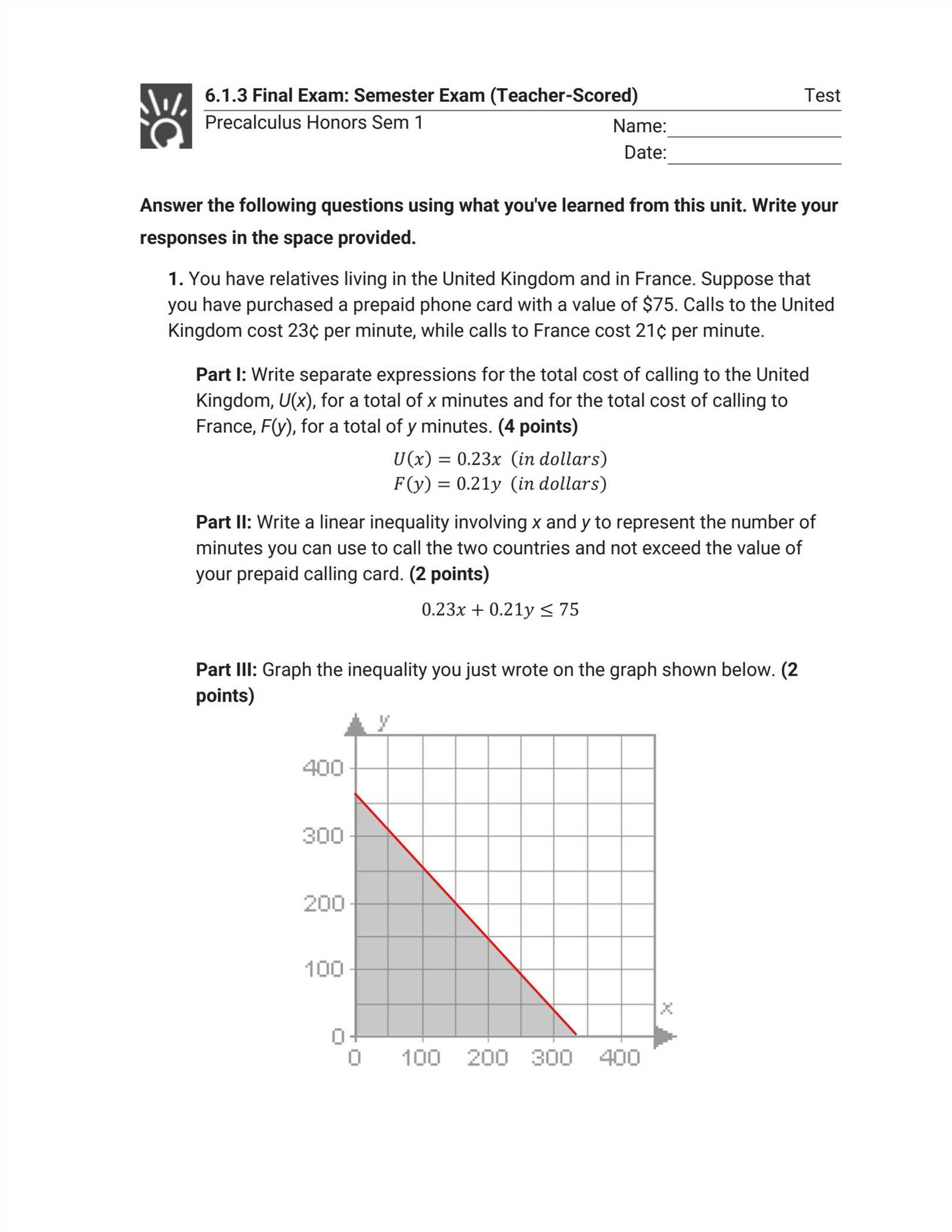
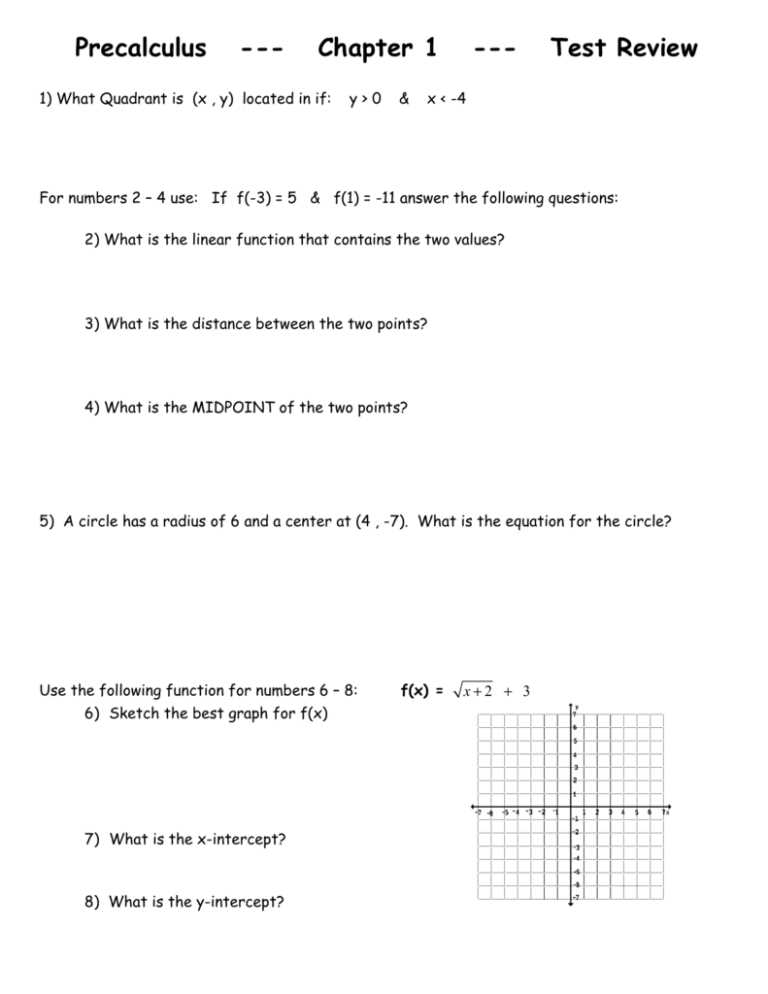
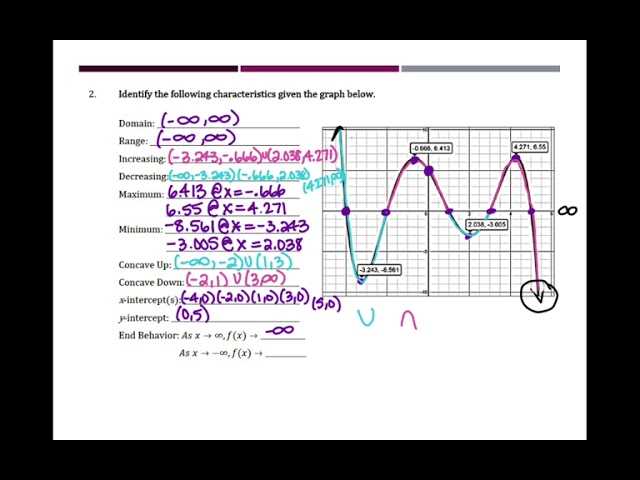
Precalculus Semester 1 Final Exam Review Answers

Success in any mathematical course requires a strong understanding of core principles and the ability to apply them to various problems. As you prepare for the upcoming assessment, it is crucial to focus on the most important topics that will be covered. The right strategies can significantly improve your performance, allowing you to tackle even the most challenging questions with confidence.

Mastering essential formulas and methods is critical for achieving accuracy in problem-solving. By thoroughly reviewing these concepts, you will be well-equipped to handle a wide range of questions. This guide highlights the major areas that demand your attention and offers practical advice for efficient study and problem-solving techniques.

Whether it’s working with functions, understanding the relationships between different mathematical elements, or solving complex equations, the following sections will provide the tools and insights necessary for thorough preparation. Focus on building your understanding step by step, and the results will speak for themselves.

Comprehensive Preparation Guide

To excel in the upcoming assessment, it is crucial to understand the fundamental concepts and techniques that will be tested. Mastering these key areas will help you approach problems confidently and efficiently. Focusing on essential topics, formulas, and problem-solving methods ensures you are well-prepared to tackle any challenge that comes your way.

Start by reinforcing your understanding of core mathematical principles. This will allow you to approach questions logically and methodically, using the right strategies for each problem type. The following areas should be prioritized:

• Equations and Inequalities: Be familiar with different types, including linear, quadratic, and rational equations, and learn how to solve them accurately.
• Functions and Graphs: Understanding the behavior of various functions and how to graph them is essential for many problem types.
• Trigonometry: Master key identities, angle measurements, and solving trigonometric equations.
• Polynomials and Rational Functions: Know how to perform operations on polynomials and work with rational functions, including simplification and solving.
• Systems of Equations: Be prepared to solve systems using methods like substitution, elimination, and graphical solutions.

In addition to theoretical knowledge, practice is vital. Work through various practice problems to improve your speed and accuracy. This will help you recognize patterns and solutions more quickly during the actual assessment.

Lastly, don’t forget to manage your time effectively. Prioritize questions based on difficulty and ensure you leave time to review your work. The more you practice and familiarize yourself with these core topics, the more confident and prepared you will be when it’s time to tackle the challenge.

Key Topics to Focus On

When preparing for an upcoming assessment, it’s essential to concentrate on the most important concepts that are likely to appear. A solid understanding of these topics will provide a strong foundation for solving a variety of problems with ease. Focus on mastering both theoretical knowledge and practical application to ensure you can confidently tackle any challenge.

Core Mathematical Functions

One of the most critical areas to focus on is understanding various mathematical functions. These include linear, quadratic, and exponential functions, as well as how to manipulate and graph them. Be sure to review their properties, such as domain, range, and key characteristics. Additionally, practice solving equations involving these functions, as this is a common area of testing.

Trigonometric Concepts

Trigonometry plays a significant role in many problems. It’s essential to be familiar with trigonometric identities, angle measurements, and how to apply them to solve problems. Focus on mastering key formulas, such as the Pythagorean identity, sum and difference identities, and solving for unknown angles or sides in right triangles. These concepts are fundamental to solving a wide range of questions efficiently.

Strengthening your grasp of these key topics will improve your problem-solving abilities and help you achieve greater success. As you review, make sure to practice various problem types to build your confidence and speed.

Essential Formulas for the Assessment

To succeed in any mathematical challenge, it is crucial to be familiar with the key formulas that will be needed for solving problems. These formulas act as the foundation for a wide range of questions and provide a framework for efficient problem-solving. A solid grasp of these essential equations will help you quickly recognize the most appropriate method for any given problem.

Focus on mastering formulas related to algebra, geometry, and trigonometry, as they are frequently tested and often require quick recall during problem-solving. Understanding how to apply these formulas effectively is just as important as memorizing them. Practice using them in various contexts to ensure you’re fully prepared.

Examples of essential formulas to focus on include:

• Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a for solving quadratic equations.
• Pythagorean Theorem: a² + b² = c² for calculating the sides of a right triangle.
• Trigonometric Identities: sin²θ + cos²θ = 1 for working with angles and ratios.
• Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) for determining the slope between two points on a line.
• Logarithmic Properties: logₐ(xy) = logₐ(x) + logₐ(y) for solving logarithmic equations.

By reviewing and practicing these formulas, you will improve both your speed and accuracy, ensuring you’re ready to approach each question with confidence.

Common Mistakes to Avoid

During any challenging mathematical assessment, it’s easy to make simple yet costly mistakes that can undermine your performance. Recognizing and addressing these errors beforehand will help ensure that you approach problems methodically and confidently. Avoiding these common pitfalls is key to achieving accuracy and maximizing your score.

Misunderstanding Key Concepts

A frequent mistake is misinterpreting the problem or overlooking crucial details in the question. Often, students rush into solving the problem without fully understanding the concept behind it, leading to incorrect solutions. To prevent this, take time to read each problem carefully and ensure you understand what is being asked before proceeding.

• Always highlight key information and write down any formulas or steps you plan to use.
• Don’t assume that the problem is simple just because it appears familiar–verify each step before moving on.
• Look for keywords like “solve for x,” “simplify,” or “find the value of” to ensure you are addressing the right question.

Calculation Errors

Another common issue is making mistakes during calculations, such as incorrect multiplication, division, or signs. These errors can easily slip through if you’re working too quickly or not double-checking your work. A small calculation mistake can lead to a major discrepancy in your final answer.

• Always check your work at the end of each problem to catch any errors.
• Pay close attention to signs, especially when dealing with negative numbers or exponents.
• Use a systematic approach to solve equations step by step to reduce the chance of overlooking simple arithmetic mistakes.

By staying mindful of these common mistakes, you’ll improve both your speed and accuracy, giving you a better chance at success.

Solving Equations Efficiently

One of the most important skills in mathematics is the ability to solve equations quickly and accurately. Whether you’re dealing with linear, quadratic, or more complex expressions, knowing the most effective methods can save valuable time and prevent mistakes. Efficiency doesn’t just mean speed; it also involves using the right approach to simplify and solve problems systematically.

Start by identifying the type of equation you’re working with, as different forms require different strategies. For example, linear equations are often solved by isolating the variable, while quadratic equations may need factoring or the quadratic formula. Understanding the structure of the equation is key to choosing the most efficient method.

Here are a few tips for solving equations more efficiently:

• Isolate the variable early: In linear equations, try to move terms to one side to isolate the variable as soon as possible.
• Factor when possible: For quadratic equations, factoring can often provide the quickest solution without resorting to the quadratic formula.
• Check for common factors: In more complex equations, look for common factors that can simplify the expression before solving.
• Use substitution or elimination: For systems of equations, substitution or elimination methods can lead to faster solutions compared to graphing.

By practicing these strategies and applying them to a variety of problems, you’ll become more adept at solving equations efficiently, improving both your speed and accuracy in tackling mathematical challenges.

Graphing and Analyzing Functions

Understanding how to graph and analyze functions is a fundamental skill in mathematics, essential for solving a wide range of problems. The ability to interpret the behavior of different types of functions through graphs not only helps visualize their characteristics but also aids in solving equations more effectively. Knowing how to plot points, identify key features, and analyze trends will significantly improve problem-solving accuracy.

Start by becoming familiar with the basic shapes of functions such as linear, quadratic, and exponential. Each function type has distinct features that influence its graph, including slope, intercepts, and curvature. By mastering these key properties, you’ll be able to recognize and sketch their graphs quickly.

Here are a few strategies for graphing and analyzing functions efficiently:

• Identify key features: Always start by finding the function’s domain, range, intercepts, and symmetry. These characteristics provide a clear starting point for sketching the graph.
• Plot points carefully: For more complex functions, select a range of values for the independent variable to find corresponding points. The more points you plot, the more accurate your graph will be.
• Understand transformations: Be able to identify how shifts, stretches, or reflections affect the graph’s position and shape. For example, adding or subtracting a constant can move the graph up or down.
• Analyze behavior: Look for asymptotes, intervals of increase or decrease, and local maxima or minima. These can provide valuable information about the function’s overall behavior.

By practicing these techniques, you’ll gain a deeper understanding of how functions behave and develop the ability to graph them accurately with minimal effort.

Understanding Polynomial Operations

Polynomial operations are essential tools for solving various algebraic problems. Mastering the fundamental operations–addition, subtraction, multiplication, and division–allows for efficient manipulation of polynomial expressions. These skills are foundational for tackling more complex problems and for simplifying expressions that arise in mathematical analysis.

Addition and Subtraction of Polynomials

When adding or subtracting polynomials, the key step is to combine like terms. Like terms are terms that have the same variable raised to the same power. Ensure that you align similar terms and perform the operation (addition or subtraction) only on the coefficients of those terms.

• For example, (3x² + 5x + 7) + (2x² – 4x + 3) becomes 5x² + x + 10 after combining like terms.
• When subtracting, remember to distribute the negative sign to all terms in the second polynomial.

Multiplying Polynomials

Multiplying polynomials involves applying the distributive property (also known as the FOIL method for binomials). Each term in one polynomial must be multiplied by each term in the other polynomial. After multiplying, combine any like terms to simplify the expression.

• For example, (x + 3)(x – 2) becomes x² + x – 6 after applying the distributive property.
• In cases with more complex polynomials, break the multiplication into smaller steps to avoid mistakes.

By mastering these operations, you’ll be better equipped to handle more advanced topics that involve polynomials and algebraic expressions.

Trigonometric Identities You Must Know

Trigonometric identities are fundamental equations that establish relationships between different trigonometric functions. Mastering these identities allows you to simplify expressions, solve equations, and evaluate trigonometric functions more efficiently. These identities are essential tools for anyone tackling trigonometric problems and help to break down complex expressions into manageable parts.

Basic Trigonometric Identities

The most essential identities to know are the fundamental ones involving sine, cosine, and tangent. These serve as building blocks for more complex identities and are used frequently in problem-solving.

• Reciprocal Identities:
  • sin(θ) = 1/csc(θ)
  • cos(θ) = 1/sec(θ)
  • tan(θ) = 1/cot(θ)
• Ratio Identities:
  • tan(θ) = sin(θ)/cos(θ)
  • cot(θ) = cos(θ)/sin(θ)

Pythagorean Identities

The Pythagorean identities are key tools for simplifying trigonometric expressions and solving equations. They derive from the fundamental Pythagorean theorem and relate the squares of the sine, cosine, and tangent functions.

• sin²(θ) + cos²(θ) = 1
• 1 + tan²(θ) = sec²(θ)
• 1 + cot²(θ) = csc²(θ)

By mastering these basic identities and understanding how they interact with one another, you’ll be well-equipped to handle more advanced trigonometric problems with ease.

Tips for Word Problems in Mathematics

Word problems can often be challenging because they require translating real-world scenarios into mathematical expressions. To solve these problems efficiently, it’s important to understand how to extract key information, identify relationships between variables, and choose the right mathematical approach. By following a systematic method, you can break down complex problems and find solutions step by step.

Here are a few strategies to tackle word problems with confidence:

• Read carefully: Take the time to read the problem thoroughly and highlight important details such as numbers, units, and relationships between variables.
• Define variables: Assign variables to the unknowns in the problem. Clearly label what each variable represents to avoid confusion as you work through the solution.
• Set up an equation: Translate the problem into a mathematical expression or equation. Identify the operations and relationships between the variables based on the context of the problem.
• Work step by step: Break the problem down into smaller steps. Solve one part of the problem at a time and check your work as you go.
• Check units: Always ensure that the units in your calculations are consistent. Converting units if necessary can help avoid errors in your final answer.

By practicing these techniques and maintaining a methodical approach, you can improve your ability to solve word problems and build a strong foundation for more advanced mathematical concepts.

How to Approach Limits and Continuity

Understanding the behavior of functions as they approach specific points is a crucial aspect of calculus. Limits help determine how a function behaves near a particular value, while continuity ensures that a function’s graph is unbroken at that point. Mastering these concepts provides the foundation for more advanced mathematical topics, including derivatives and integrals.

When tackling problems related to limits and continuity, follow these strategies to simplify the process:

Approaching Limits

• Substitute the value: Start by directly substituting the given value into the function. If the result is a defined number, the limit is that value.
• Factor when necessary: If direct substitution results in an indeterminate form like 0/0, try factoring the expression to cancel out common terms.
• Use rationalization: If the expression involves square roots, rationalize the numerator or denominator to simplify the limit calculation.
• Check one-sided limits: If the function behaves differently when approaching from the left or right, evaluate the left-hand and right-hand limits separately.

Understanding Continuity

• Check for undefined points: A function is continuous at a point if it is defined at that point, the limit exists, and the function value equals the limit.
• Examine the behavior around the point: Determine if there are any jumps, holes, or vertical asymptotes in the graph. These discontinuities indicate where the function is not continuous.
• Piecewise functions: When dealing with piecewise functions, ensure that the function’s value from one piece matches the value from the next at the point where the pieces connect.

By following these steps and practicing regularly, you can develop a strong understanding of how limits and continuity interact, making it easier to solve related problems efficiently.

Working with Exponents and Logarithms

Exponents and logarithms are fundamental concepts that provide a powerful way to express and manipulate numbers, especially when dealing with large values or complex equations. Understanding how to simplify expressions with powers and their inverses allows for efficient problem-solving in a variety of mathematical contexts. These tools are essential for solving exponential equations, modeling growth, and understanding the relationships between different variables.

When working with exponents and logarithms, it is important to master the following techniques:

Exponent Rules

• Product Rule: When multiplying terms with the same base, add the exponents: a^m * a^n = a^(m+n).
• Quotient Rule: When dividing terms with the same base, subtract the exponents: a^m / a^n = a^(m-n).
• Power Rule: When raising a power to another power, multiply the exponents: (a^m)^n = a^(m*n).
• Negative Exponent Rule: A negative exponent indicates a reciprocal: a^(-n) = 1/a^n.

Logarithm Rules

• Product Rule: The logarithm of a product is the sum of the logarithms: log_b(xy) = log_b(x) + log_b(y).
• Quotient Rule: The logarithm of a quotient is the difference of the logarithms: log_b(x/y) = log_b(x) – log_b(y).
• Power Rule: The logarithm of a power is the exponent times the logarithm: log_b(x^n) = n * log_b(x).
• Change of Base Formula: Allows you to calculate logarithms with different bases: log_b(x) = log_c(x) / log_c(b), where c is any base.

By mastering these rules, you can simplify complex expressions, solve equations more easily, and gain a deeper understanding of exponential growth and decay, making them indispensable tools for higher-level mathematics.

Key Properties of Rational Functions

Rational functions are mathematical expressions formed by dividing two polynomials. Understanding their properties is crucial for analyzing their behavior, identifying key features such as asymptotes, and solving related equations. These functions are essential in various real-world applications, including modeling rates of change and physical phenomena.

Here are the primary characteristics to focus on when studying rational functions:

Domain and Range

• Domain: The domain of a rational function consists of all real numbers except where the denominator equals zero. To find these values, solve for the points where the denominator is zero.
• Range: The range is determined by the overall behavior of the function, including the limitations imposed by the asymptotes and any holes in the graph.

Asymptotes

• Vertical Asymptotes: These occur where the denominator is zero and the numerator is non-zero. To find vertical asymptotes, solve for values of x that make the denominator equal to zero.
• Horizontal Asymptotes: Horizontal asymptotes describe the behavior of the function as x approaches infinity. If the degree of the numerator is less than the denominator, the horizontal asymptote is y = 0. If the degrees are equal, the asymptote is y = ratio of the leading coefficients.
• Oblique Asymptotes: When the degree of the numerator is exactly one more than the degree of the denominator, the function has an oblique asymptote, which can be found using polynomial long division.

Holes in the Graph

• Factorization: If both the numerator and denominator have a common factor, there may be a hole in the graph at the point where the factor cancels. To find holes, factor the expression and simplify it, identifying the values of x where the cancellation occurs.

By understanding these essential properties, you can graph rational functions more accurately, determine their behavior at extreme values, and identify key points that affect their overall shape and behavior.

Sequences and Series Review

Understanding sequences and series is fundamental in various mathematical concepts, particularly when dealing with patterns, sums, and progressions. A sequence is a list of numbers that follow a specific order or rule, while a series represents the sum of terms in a sequence. These concepts are essential in fields such as calculus, algebra, and even financial mathematics.

In this section, we’ll cover the key principles of sequences and series, including arithmetic and geometric sequences, and how to find the sums of these series. It’s important to grasp the formulas and techniques for evaluating both infinite and finite sums to efficiently solve problems involving these mathematical structures.

Arithmetic Sequences

An arithmetic sequence is a sequence in which each term after the first is obtained by adding a constant difference to the previous term. The general formula for the nth term of an arithmetic sequence is:

a_n = a₁ + (n-1) * d,

where a_n is the nth term, a₁ is the first term, d is the common difference, and n is the term number.

Geometric Sequences

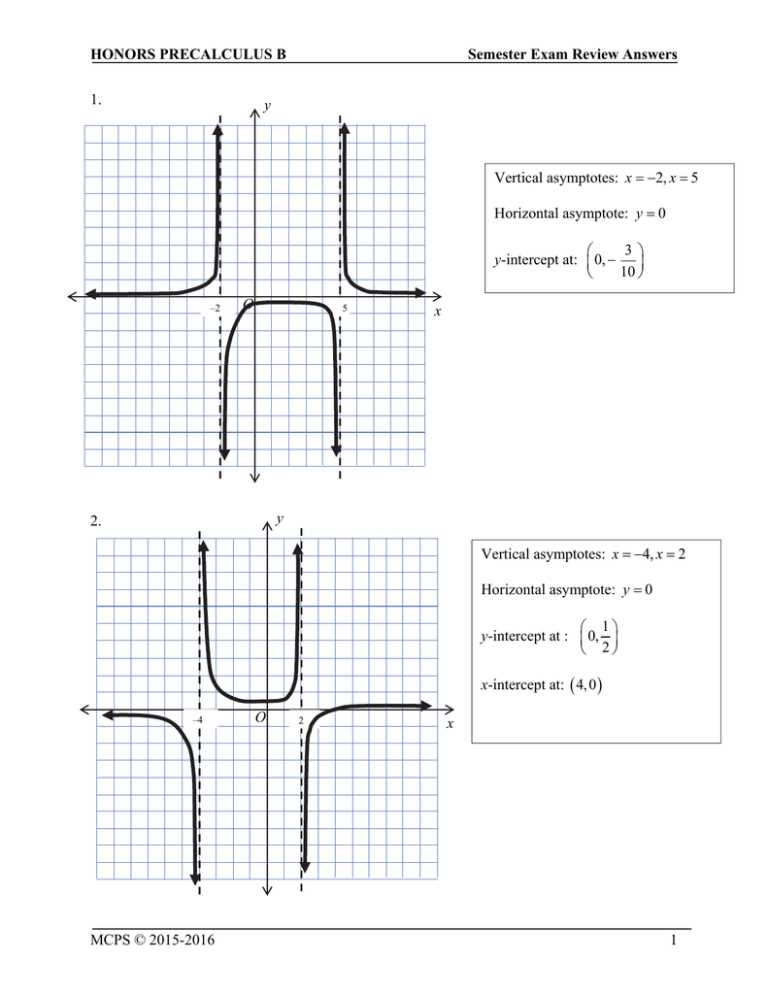
A geometric sequence is one where each term after the first is found by multiplying the previous term by a constant ratio. The formula for the nth term of a geometric sequence is:

a_n = a₁ * r^n-1,

where a₁ is the first term, r is the common ratio, and n is the term number.

Sum of Sequences

The sum of terms in a sequence can be found using specific formulas for both arithmetic and geometric series. Below is a table summarizing the sum formulas for both types of series:

Type of Sequence	Sum Formula
Arithmetic Series	Sn = n/2 * (a1 + an), where n is the number of terms, a1 is the first term, and an is the nth term.
Geometric Series	Sn = a1 * (1 – rn) / (1 – r), where a1 is the first term and r is the common ratio.

Both arithmetic and geometric series can be extended to infinite sums. For an infinite geometric series, the sum converges only if the absolute value of the common ratio is less than one. The formula for the sum of an infinite geometric series is:

S = a₁ / (1 – r), where r is the common ratio and |r| .

By mastering these concepts and formulas, you can efficiently tackle problems involving sequences and series in various mathematical contexts.

Systems of Equations Explained

In mathematics, a system of equations involves multiple equations that are solved simultaneously. These equations often have common variables, and the goal is to find the values for those variables that satisfy all the given equations at the same time. Solving such systems requires understanding how the equations relate to each other and how to manipulate them to find the solutions.

There are several methods for solving systems of equations, including substitution, elimination, and graphical methods. Each method offers a different approach, depending on the complexity of the system and the available tools. A system can have one solution, no solution, or infinitely many solutions, depending on the relationships between the equations.

Substitution Method

The substitution method involves solving one equation for one variable and then substituting that expression into the other equation(s). This method is particularly useful when one of the equations is easily solvable for a single variable. Here’s a step-by-step process:

• Choose one of the equations and solve for one variable.
• Substitute that expression into the other equation(s).
• Solve the resulting equation for the second variable.
• Substitute the value of the second variable back into the first equation to find the first variable.

Elimination Method

The elimination method involves adding or subtracting the equations to eliminate one variable, making it easier to solve for the other. The goal is to manipulate the equations so that the coefficients of one variable are opposites, allowing them to cancel out. Here’s how it works:

• Multiply one or both of the equations to align the coefficients of one variable.
• Add or subtract the equations to eliminate that variable.
• Solve the resulting equation for the remaining variable.
• Substitute this value into one of the original equations to find the other variable.

Both methods are effective, and the choice of which to use depends on the structure of the system and personal preference. By practicing these techniques, you can gain confidence in solving systems of equations and tackle more complex mathematical problems efficiently.

Reviewing Conic Sections

Conic sections are curves that result from the intersection of a plane and a cone. These curves have unique properties and equations, and understanding them is crucial for solving various mathematical and real-world problems. The four main types of conic sections are circles, ellipses, parabolas, and hyperbolas. Each type of conic section has distinct characteristics, equations, and applications, making them fundamental in fields such as physics, engineering, and astronomy.

Types of Conic Sections

The different conic sections arise depending on the angle at which the plane intersects the cone. Below is a brief overview of each type:

Conic Type	Equation	General Shape
Circle	(x – h)2 + (y – k)2 = r2	Round, all points equidistant from the center
Ellipse	(x – h)2 / a2 + (y – k)2 / b2 = 1	Oval shape, two foci
Parabola	y = a(x – h)2 + k	U-shaped, one focus
Hyperbola	(x – h)2 / a2 – (y – k)2 / b2 = 1	Two opposite, asymptotic curves

Key Features and Applications

Each type of conic section has specific characteristics and uses in different fields:

• Circles: Defined by a fixed radius, circles are often used in navigation, design, and physics to model rotational symmetry and uniform motion.
• Ellipses: With two foci, ellipses are found in planetary orbits and in the design of optical devices such as telescopes.
• Parabolas: Parabolic shapes are essential in the study of projectile motion and are frequently used in satellite dish design and reflector shapes.
• Hyperbolas: Hyperbolas describe the path of objects under certain conditions, such as in the study of radioactive decay or the reflection of sound waves.

Mastering conic sections is essential for solving various geometry and algebra problems, as well as understanding their real-world applications. Practicing the different forms of their equations and recognizing their unique properties will help build a solid foundation for advanced studies in mathematics and science.

Preparing for the Final Exam Strategy

Successfully approaching any major assessment requires strategic planning and a focused mindset. The key to doing well is not only reviewing the material but also implementing an effective study routine that reinforces your understanding and builds confidence. Breaking down the content into manageable sections, practicing with examples, and mastering problem-solving techniques will significantly enhance your preparation. This section covers important steps and strategies to help you succeed in your preparation process.

Effective Study Techniques

To prepare efficiently, consider using a combination of different study methods. Here are some techniques that will help reinforce your learning:

• Active Recall: Focus on recalling information from memory instead of just rereading notes. Try to explain concepts in your own words.
• Practice Problems: Solve as many practice problems as possible, especially those that are similar to what you expect to see. This will help you become familiar with question formats and types.
• Spaced Repetition: Review material regularly over time to improve long-term retention and avoid cramming.
• Group Study: Sometimes working with others can help clarify concepts and give you a broader understanding of the material.

Key Areas to Focus On

Make sure to prioritize your time based on the topics that are most challenging or have the greatest weight in the assessment. Here’s how to prioritize your study:

1. Understand the Fundamentals: Focus on mastering the foundational concepts, as these will help you solve more complex problems.
2. Identify Weak Spots: Pay special attention to areas where you struggle. Break down difficult problems step by step and make sure to fully understand each part.
3. Work on Time Management: During your preparation, simulate exam conditions by timing yourself as you solve practice questions to improve your speed and accuracy.
4. Focus on Formulae: Make sure you are familiar with essential formulas and equations, as these will help you quickly solve problems without wasting time looking up information.

By incorporating these strategies into your preparation, you’ll be able to approach your study sessions with confidence and a clear plan, leading to a more successful outcome. Remember that consistency and practice are key when aiming to master the material and perform at your best.

Time Management During the Exam

Effective time management is essential when it comes to tackling any assessment efficiently. The ability to allocate the right amount of time to each question or section can greatly impact your performance. By approaching the task strategically, you can ensure that you have enough time to answer all the questions while also reviewing your work. This section will explore key strategies for managing time effectively during an assessment, ensuring that you maximize your chances for success.

Prioritize Questions Based on Difficulty

Start by quickly scanning through the entire assessment before diving into any individual question. Identify the questions that seem the easiest or most familiar, and tackle them first. These should be questions you can solve confidently and quickly, helping you build momentum. For the more challenging questions, allocate more time but don’t get stuck on them for too long. If you’re unsure, move on and come back to them later.

Set a Time Limit for Each Section

Assign a specific amount of time to each section or question. For example, if the test has multiple sections, try to divide the total time by the number of sections and set time limits for each. This strategy prevents you from spending too much time on any one area, ensuring you can complete everything. Use a watch or timer to keep track of the time without distractions.

Keep an Eye on the Clock

Regularly check the time to make sure you’re staying on track. It’s easy to lose track of time when you get focused on a difficult question, so it’s important to have periodic reminders to assess whether you’re spending the appropriate amount of time on each task. If you’re falling behind, adjust by working more quickly or skipping particularly tricky questions.

Leave Time for Review

At the end of the test, always leave some time to go over your answers. A final review allows you to catch any mistakes or double-check your work. If time is running short, focus on reviewing the questions that carry the most points. This can help you ensure that you haven’t overlooked any critical errors and boost your confidence in your work.

By managing your time effectively, you can stay calm, organized, and confident throughout the assessment, ultimately improving your performance. The key is preparation and practice, so incorporate time-management strategies in your study sessions to help make them second nature during the actual assessment.