Dividing Rational Expressions Worksheet Answers

Working with algebraic fractions involves various skills that are essential for simplifying and solving complex mathematical problems. Understanding how to manipulate these fractions correctly is crucial for progressing in algebra and beyond. The process requires knowledge of multiplication, division, and factoring techniques to simplify the terms involved.
In this guide, we will walk through essential strategies for handling these types of problems, offering a step-by-step approach that will make tackling more challenging equations easier. With practice, you’ll be able to break down complicated expressions and solve them accurately and efficiently.
Mastering these techniques will not only improve your algebraic abilities but also provide a deeper understanding of mathematical principles that apply in real-world situations. By reviewing key concepts and practicing problems, you’ll be prepared for any algebraic challenge that comes your way.
Understanding the Basics of Fractional Algebraic Equations
To tackle complex mathematical problems involving fractions, it’s essential to grasp the underlying principles that govern their manipulation. Mastering these core concepts will help simplify calculations and ensure accuracy when solving equations. The key lies in understanding how to simplify, factor, and perform operations on algebraic fractions correctly.
Key Concepts to Know
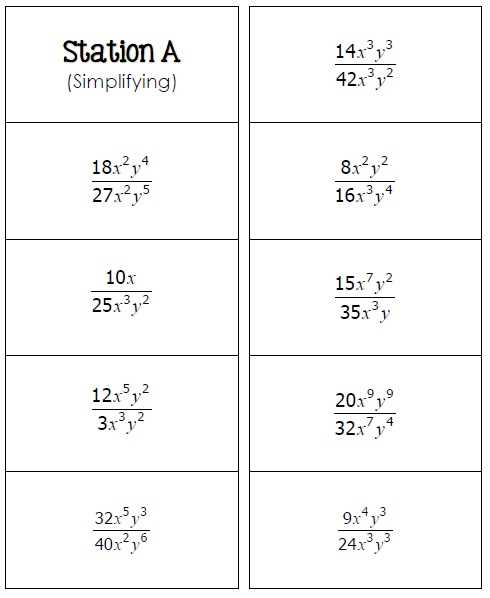
The main idea is to treat algebraic fractions similarly to numerical fractions, with additional steps for factoring and simplifying terms. This involves identifying factors that can be canceled out, recognizing common denominators, and applying rules for multiplication or addition where needed. These foundational techniques set the stage for solving more advanced problems in algebra.
Importance of Simplification
Simplifying the terms before performing any operations reduces the complexity of the problem. By factoring both the numerator and denominator, you can cancel out common factors, making the equation easier to solve. Mastering these basic skills is the first step toward confidently working with more challenging algebraic problems.
Key Steps in Handling Fractional Algebraic Equations
When working with fractions that involve algebraic terms, it’s important to follow a systematic approach to ensure accurate results. The process involves a few clear steps that make it easier to manipulate these fractions and reach the correct solution. By following these steps, you can simplify the problem and reduce the risk of errors.
The first step is always to factor the numerators and denominators as much as possible. This allows you to identify common factors that can be canceled out, streamlining the equation. Once the terms are simplified, perform the necessary operations, such as multiplying or simplifying terms, while maintaining careful attention to each factor.
Another crucial aspect is checking for any terms that could cause division by zero, which is undefined. Always ensure that no variable leads to an invalid operation when simplifying the equation. By being thorough in each of these steps, you can confidently handle complex algebraic fractions.
Common Mistakes When Working with Algebraic Fractions
When solving problems that involve algebraic fractions, several common errors can occur that lead to incorrect results. Being aware of these mistakes is key to improving your skills and avoiding pitfalls. These missteps are often related to simplifying terms, handling factors, and performing operations correctly.
Top Errors to Avoid
- Ignoring Common Factors: Failing to cancel out common factors between the numerator and denominator can lead to unnecessary complexity and errors in the solution.
- Incorrectly Handling Negative Signs: Misplacing or misinterpreting negative signs in the fractions can significantly affect the final result.
- Forgetting to Factor: Not factoring the terms before performing any operations leads to more complicated calculations and increases the likelihood of mistakes.
Other Potential Pitfalls
- Not Checking for Division by Zero: It’s crucial to check that no variable will lead to division by zero, as this results in an undefined expression.
- Overlooking Simplification: Sometimes, students skip the simplification step, which can make the equation more challenging to solve and result in a higher chance of mistakes.
How to Simplify Algebraic Fractions Before Solving
Before performing any operations on algebraic fractions, it’s essential to simplify them as much as possible. This step not only makes the equation easier to work with but also reduces the risk of making errors during the process. By simplifying the terms first, you can ensure a smoother and more accurate calculation.
Key Techniques for Simplification
The primary approach to simplification is factoring both the numerator and the denominator. By factoring out common terms, you can identify factors that will cancel out, thus reducing the complexity of the problem. This is especially useful when dealing with polynomials in the numerator and denominator.
Check for Common Factors
Once the terms are factored, look for common factors between the numerator and denominator. These factors can be canceled out, making the fraction simpler. Always double-check that no common terms are overlooked before proceeding with further calculations.
Examples of Working with Algebraic Fractions
To better understand how to manipulate algebraic fractions, it is helpful to go through several examples. These examples will demonstrate the process step by step, showing how to simplify the terms and apply the necessary operations. By working through such problems, you will gain a clearer understanding of the techniques involved.
Example 1: Simple Fractional Equation
Consider the following equation:
(2x² + 6x) / (4x) ÷ (x + 3) / 2
First, simplify each part. Factor the numerator and denominator of the first fraction:
2x(x + 3) / 4x
Now, cancel out the common factor of x:
2(x + 3) / 4
Next, invert the second fraction and multiply:
2(x + 3) / 4 × 2 / (x + 3)
Cancel out (x + 3), and simplify:
2 / 4 × 2 = 1
Example 2: More Complex Fractional Equation
Now consider a slightly more complicated problem:
(x² – 9) / (x + 3) ÷ (x + 2) / (x – 3)
Start by factoring both the numerator and the denominator of the first fraction:
(x – 3)(x + 3) / (x + 3)
Cancel out the common factor of (x + 3), then invert the second fraction and multiply:
(x – 3) / 1 × (x – 3) / (x + 2)
Finally, simplify:
(x – 3)² / (x + 2)
Practice Problems for Working with Algebraic Fractions
To strengthen your understanding and skills, it’s important to practice solving problems that involve manipulating algebraic fractions. These problems will help you apply the steps you’ve learned, such as simplifying terms, factoring, and performing operations. Regular practice is essential to mastering the techniques needed for accurate and efficient problem-solving.
Problem 1
Simplify the following equation:
(x² – 4) / (x + 2) ÷ (x – 2) / (x + 2)
Solution: Begin by factoring the first fraction:
(x – 2)(x + 2) / (x + 2)
Cancel out the common factor of (x + 2):
(x – 2) ÷ (x – 2)
After canceling, the result is 1.
Problem 2
Simplify the following equation:
(3x² – 12) / (x + 4) ÷ (x² + 8x + 16) / (x + 4)
Solution: Start by factoring the first fraction:
3(x – 2)(x + 2) / (x + 4)
Factor the second fraction:
(x + 4)(x + 4) / (x + 4)
Cancel out the common (x + 4) term from both fractions:
3(x – 2)(x + 2) / (x + 4)
This leaves you with the simplified fraction 3(x – 2)(x + 2) / (x + 4).
Real-World Applications of Algebraic Fractions
Algebraic fractions are not only important for solving mathematical problems in the classroom, but they also have a wide range of practical applications in everyday life and various professional fields. Understanding how to work with these fractions allows individuals to solve real-world issues, from budgeting and construction to engineering and science. By applying the principles of simplifying and manipulating these fractions, we can model and solve complex situations efficiently.
Applications in Engineering
In engineering, algebraic fractions are often used to calculate load-bearing capacities, material strengths, and other factors that involve ratios. Engineers may work with fractions when designing systems where one element’s behavior is related to another, such as the ratio of force to surface area. Understanding how to manipulate these fractions is essential to ensuring safety and precision in engineering projects.
Applications in Economics
Algebraic fractions also appear in economics, where they are used to calculate profit margins, cost ratios, and return on investment. By working with these equations, economists can model scenarios where different variables influence outcomes in a proportional relationship. This helps in forecasting trends and making data-driven decisions.
| Application | Example | How Algebraic Fractions Help |
|---|---|---|
| Construction | Mixing cement with water in specific ratios | Understanding the ratio of materials ensures the correct consistency and strength of concrete |
| Medicine | Dosage calculations for medications | Accurate dosages are determined using ratios based on weight or body surface area |
| Physics | Calculating velocity, acceleration, and distance | By using algebraic fractions, physicists can relate multiple variables and predict motion |
Factoring Techniques for Simplifying Expressions

Factoring is a key skill for simplifying algebraic equations, particularly when dealing with fractions or more complex expressions. By factoring terms, we can break down an equation into smaller, manageable parts that are easier to work with. This process can help identify common factors, cancel out terms, and ultimately simplify the equation to its most efficient form.
Factoring by Grouping
One common technique for factoring is called “factoring by grouping.” This method works well when you have four terms in a polynomial. The idea is to group terms in pairs, factor out the greatest common factor (GCF) from each pair, and then factor the resulting binomial. This can often simplify the equation significantly.
For example, consider the following expression:
x² + 5x + 2x + 10
Group the terms:
(x² + 5x) + (2x + 10)
Factor out the GCF from each group:
x(x + 5) + 2(x + 5)
Now factor out the common binomial:
(x + 5)(x + 2)
Factoring Quadratic Expressions
Another essential technique is factoring quadratics, which are expressions of the form ax² + bx + c. To factor these, we need to find two numbers that multiply to ac and add to b. Once these numbers are found, we can rewrite the middle term as a sum or difference and factor by grouping.
For instance, consider:
x² + 7x + 10
We need two numbers that multiply to 10 (the constant term) and add up to 7 (the coefficient of x). These numbers are 2 and 5. Rewrite the expression as:
x² + 2x + 5x + 10
Now, group and factor:
x(x + 2) + 5(x + 2)
Factor out the common binomial:
(x + 2)(x + 5)
What Happens If You Divide by Zero
In mathematics, dividing by zero is undefined and leads to problems that cannot be resolved within the standard rules of arithmetic. When we attempt to divide any number by zero, it causes contradictions and creates expressions that lack meaning. This issue arises because division is essentially the inverse of multiplication, and there is no number that you can multiply by zero to get a non-zero result.
Let’s explore why this is the case. Consider the equation:
a / 0 = x
If this were possible, then multiplying both sides of the equation by zero would give:
a = x * 0
However, no matter what value x is, the result of x * 0 will always be zero, making it impossible for a to equal anything other than zero. Thus, the operation is undefined, and no meaningful solution exists.
Real-World Implications
In real-world situations, attempting to divide by zero can lead to errors in calculations, whether in computer programs, engineering models, or financial forecasting. Many systems are designed to detect and prevent division by zero to avoid incorrect results. For example, in computing, division by zero typically generates an error message, signaling that the operation cannot be completed.
Exceptions and Limits

Although division by zero is undefined, there are exceptions in certain mathematical contexts, such as limits in calculus. In these cases, we may approach division by zero as a limit, but the result still depends on how the expression behaves as it gets closer to zero, rather than actually dividing by zero itself.
How to Handle Complex Rational Expressions
When dealing with complicated algebraic fractions, it’s essential to simplify and manage them step by step. Complex fractions, which involve fractions within fractions, can appear intimidating at first, but by breaking them down methodically, they become easier to work with. The key is to first simplify the numerator and denominator separately before combining them into one expression.
Step 1: Simplify the Numerator and Denominator
The first thing to do when approaching a complex fraction is to simplify both the numerator and the denominator individually. If either part contains fractions, we must perform the appropriate operations to eliminate them. This often involves multiplying both the numerator and denominator by a common factor to clear out any nested fractions.
For example, consider the following expression:
(1 / x + 2) / (3 / y – 1)
First, simplify each part:
- For the numerator 1 / x + 2, combine into a single fraction if needed.
- For the denominator 3 / y – 1, convert into a single fraction as well.
Step 2: Combine the Fractions
After simplifying the numerator and denominator, the next step is to combine them into a single fraction. This often involves finding a common denominator for both parts, and then performing the necessary operations (addition, subtraction, multiplication, etc.) to reduce the overall complexity.
For example, if the simplified numerator becomes (1 + 2x) / x and the denominator simplifies to (3y – 1) / y, the complex fraction can now be rewritten as:
((1 + 2x) / x) / ((3y – 1) / y)
At this point, the two fractions can be multiplied by flipping the denominator and multiplying across, resulting in:
((1 + 2x) * y) / (x * (3y – 1))
By simplifying the terms further, you will arrive at the final form of the complex fraction, now simplified and easier to work with.
Step-by-Step Guide to Solving Problems
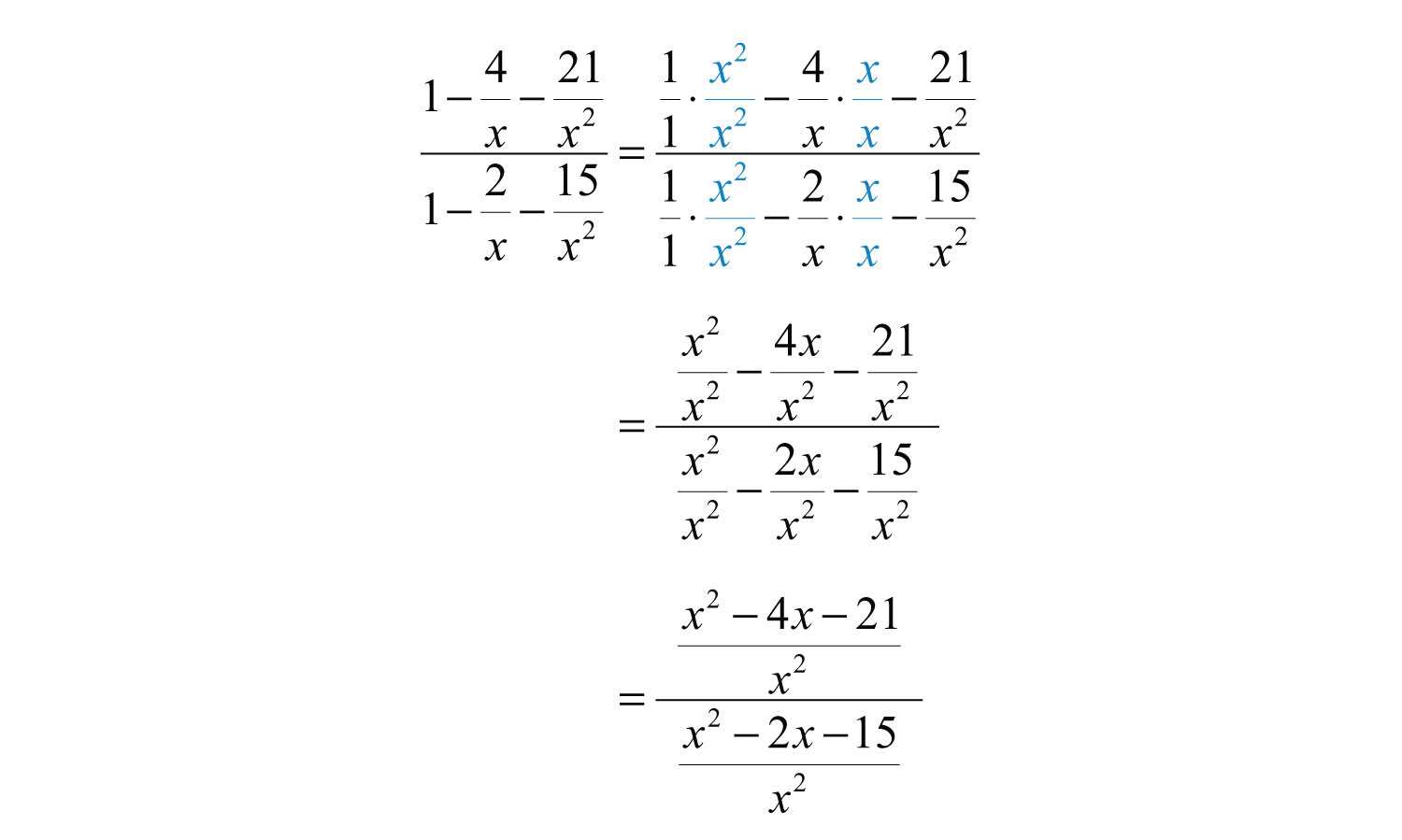
Solving algebraic problems can often seem challenging, but breaking them down into manageable steps makes the process much more straightforward. The key to success lies in approaching each problem methodically, simplifying expressions where necessary, and performing operations step by step. This guide will walk you through the process, ensuring that you stay organized and accurate throughout each stage.
Step 1: Analyze the Problem
The first step is always to carefully read the problem and identify what is being asked. Look for key variables, constants, and the operations involved. Understanding the structure of the problem helps you decide the most efficient method for solving it.
Step 2: Simplify and Rearrange
Before jumping into calculations, it’s important to simplify any complex parts of the problem. This might involve factoring expressions, removing unnecessary terms, or reordering components so that they align with the operations you need to perform. A well-organized problem is much easier to solve.
For instance, if you are working with an algebraic fraction, consider simplifying both the numerator and the denominator separately. This can prevent errors down the road and lead to more accurate results.
Step 3: Apply Operations
Once the problem is simplified, proceed by applying the required operations–whether it’s addition, subtraction, multiplication, or more complex operations like factoring or combining terms. Keep in mind that each step should be logical and sequential to avoid confusion.
Here’s an example breakdown of an algebraic problem:
| Step | Action | Result |
|---|---|---|
| 1 | Identify key variables and constants | Variables: x, y; Constants: 3, 2 |
| 2 | Simplify fractions or expressions if necessary | (2x + 3) / y becomes (x + 3) / y |
| 3 | Perform the required operation (e.g., multiplying both parts) | Multiply the simplified terms |
| 4 | Combine results and simplify further | Final result: (x + 3) / (y + 1) |
By following this method, you’ll ensure that your work is both accurate and efficient, minimizing errors and leading to clearer solutions.
Using Worksheets to Master Algebraic Concepts
Practicing algebraic techniques through targeted exercises is an effective way to build proficiency and confidence. By regularly working through problems, students can reinforce their understanding, recognize patterns, and become more adept at handling complex situations. Worksheets designed with focused tasks are a great resource for mastering mathematical concepts, offering the structure needed for continued learning.
Benefits of Regular Practice
Working through exercises consistently helps you identify areas that need improvement and solidify your knowledge. Some of the key advantages of using practice sheets include:
- Reinforcement: Repeating similar problems enhances memory retention and understanding.
- Problem-solving skills: Tackling a variety of problems improves your ability to approach different types of questions with confidence.
- Time management: Regular practice hones your ability to solve problems more efficiently within a set time frame.
- Self-assessment: Worksheets allow you to test your understanding and track your progress over time.
How to Maximize the Benefits
To get the most out of your practice sessions, consider the following strategies:
- Start with easier problems: Begin with simpler exercises to build your confidence before progressing to more challenging ones.
- Break down complex problems: If a problem feels overwhelming, break it into smaller, more manageable parts. This will help you focus on one step at a time.
- Review mistakes: Take the time to understand errors. This process deepens your understanding and prevents repeating the same mistakes.
- Work on a variety of problems: Seek out worksheets with a range of problems to ensure that you’re exposed to different scenarios and types of questions.
Incorporating worksheets into your study routine can transform the learning process, making it more structured and effective. By practicing regularly and following these strategies, you’ll improve both your problem-solving abilities and your overall understanding of the subject.
Exploring Different Methods of Simplification
Simplifying mathematical expressions is a crucial skill that helps make complex problems more manageable. Whether working with algebraic fractions, polynomial terms, or other forms of expressions, using different strategies can lead to more efficient solutions. Understanding and applying various techniques ensures a deeper grasp of mathematical principles and streamlines the problem-solving process.
Key Simplification Techniques
There are several methods to simplify expressions, each applicable in different situations. Here are some of the most commonly used techniques:
- Factorization: Breaking down expressions into factors allows for the cancellation of common terms, simplifying the overall expression.
- Combining like terms: Grouping similar terms together makes the expression easier to handle by reducing redundancy.
- Reducing common factors: Identifying and eliminating common factors between the numerator and denominator is a quick way to simplify complex expressions.
- Using identities: Applying mathematical identities, such as the difference of squares or the distributive property, can help simplify expressions more efficiently.
Choosing the Right Method
While these techniques are effective, selecting the right one for a given problem is key. Here’s how to determine the best approach:
- Look for common factors: Always start by checking for any common factors that can be canceled out.
- Check for like terms: If the expression contains terms that can be grouped, this can simplify the process significantly.
- Examine the structure: Depending on whether the expression is a fraction, polynomial, or a combination, some methods might be more appropriate than others.
- Practice with different problems: The more you practice, the better you’ll become at identifying the most efficient method for each type of problem.
By mastering these simplification techniques, you can approach problems with confidence and precision, streamlining your calculations and ensuring accurate results.
Why Understanding Dividing Rational Expressions Matters
Mastering the process of simplifying complex algebraic fractions is essential for solving advanced mathematical problems efficiently. It helps build a solid foundation for understanding higher-level concepts, such as calculus, linear algebra, and engineering applications. Without a clear grasp of how to manipulate and simplify these types of problems, tackling more intricate equations can become overwhelming and prone to errors.
Real-World Applications
In many fields, such as science, economics, and technology, the ability to work with algebraic fractions is not just an academic exercise–it is a vital skill. For example, engineers may encounter formulas requiring the manipulation of variables, and medical professionals might use statistical models that rely on simplified equations. By understanding the principles behind simplifying these fractions, professionals can interpret and apply mathematical models with greater precision.
Enhancing Problem-Solving Skills
When you know how to approach complex algebraic problems, you can break them down into manageable steps, which leads to quicker and more accurate solutions. This skill also improves logical thinking, as it encourages you to recognize patterns, spot relationships between variables, and apply appropriate strategies. Moreover, practicing the manipulation of these expressions sharpens your ability to solve a wide range of mathematical challenges, making you more proficient in the subject.
Overall, grasping how to simplify and manipulate algebraic fractions not only makes solving equations easier but also builds a stronger mathematical mindset that can be applied across various disciplines and real-life situations.
Tips for Practicing Dividing Rational Expressions
To become proficient at simplifying and solving algebraic fractions, consistent practice is key. As with any mathematical skill, repetition helps reinforce concepts and improves problem-solving speed. By regularly working through problems and understanding the steps involved, you’ll develop the confidence to tackle increasingly complex challenges. Below are a few tips to enhance your practice sessions.
Break Down Complex Problems
When faced with complicated problems, it’s easy to feel overwhelmed. The best way to approach such problems is by breaking them into smaller, manageable parts. Start by factoring each term, simplifying the numerators and denominators, and then proceed with the operations. This approach makes the problem less intimidating and allows you to focus on one step at a time.
Practice with Different Problem Types
It’s essential to practice a variety of problems to gain a comprehensive understanding of the subject. Work through different levels of difficulty, from simple problems to more challenging ones that involve multiple steps. By doing so, you’ll become familiar with various scenarios and learn to adapt your strategies accordingly. The more diverse your practice problems, the better prepared you’ll be for any situation.
Additionally, check your work after each step to ensure accuracy. Mistakes are a natural part of the learning process, but identifying them early can help you avoid repeating them in the future. With consistent effort and focus, you’ll gain mastery in simplifying algebraic fractions and be ready to apply your skills in more advanced areas of mathematics.