Chapter 12 Circles Test Answers and Solutions

In this section, we explore the key concepts behind geometric figures with rounded shapes, focusing on their properties, relationships, and various calculations. Understanding the fundamentals of these figures is crucial for solving a wide range of mathematical problems, from basic measurements to complex geometric proofs.

By examining specific challenges related to these shapes, you can enhance your problem-solving skills and improve your ability to apply various formulas. This guide will help you gain a deeper understanding of how to approach problems involving radii, diameters, and angles formed by curved lines.

With clear explanations and detailed solutions, this resource will equip you with the tools to confidently tackle a variety of questions related to these geometric figures. Whether you’re preparing for exams or simply looking to sharpen your math skills, this guide offers valuable insights to support your learning journey.

Chapter 12 Circles Test Overview

This section focuses on evaluating the essential concepts related to rounded geometric shapes. It covers the fundamental principles that form the basis for a wide range of problems, exploring the relationships between different components such as diameters, radii, and angles. Understanding these relationships is key to solving complex challenges in this area of mathematics.

The problems featured here require applying formulas for areas, perimeters, and angles formed by arcs and lines. You’ll encounter a variety of question types, from straightforward calculations to more intricate logical reasoning tasks. This overview provides insight into how each problem is structured, offering a step-by-step approach to reach the correct solutions.

By mastering the core ideas of this section, you’ll be equipped to tackle more advanced problems in the future. Whether working through exercises or preparing for assessments, this overview aims to strengthen your grasp of crucial geometric concepts and their practical applications.

Key Concepts in Circle Geometry

Understanding the key principles of geometry involving round shapes is essential for solving related problems. These concepts encompass the relationships between different elements, such as distances from the center, various angles, and the properties of lines that intersect the shape. Mastering these concepts allows for accurate calculations and deeper insights into the structure of these geometric figures.

Properties of a Geometric Figure with a Rounded Shape

Several core attributes define these figures. The most fundamental include the radius, diameter, and circumference, all of which are interrelated through specific formulas. Additionally, angles formed by intersections of lines or tangents offer essential clues for solving complex questions.

Key Formulas to Remember

To solve problems effectively, it’s important to be familiar with key formulas that govern the relationships within these figures. These formulas allow for the calculation of the perimeter, area, and various angles, all of which are necessary for understanding the geometry of round shapes.

Formula	Application
Radius = Diameter ÷ 2	Used to find the radius when the diameter is known.
Area = π × r²	Calculates the area of a rounded shape.
Circumference = 2 × π × r	Used to determine the perimeter of the shape.
Angle of Arc = (Arc Length ÷ Circumference) × 360°	Helps in calculating the angle formed by an arc.

By learning these fundamental principles and applying the relevant formulas, you’ll be well-prepared to tackle a variety of geometry problems involving round shapes. A solid grasp of these concepts will provide a strong foundation for further study and practical applications in mathematics.

Understanding Circle Theorems and Formulas

Mastering the fundamental theorems and formulas related to geometric shapes with a rounded structure is crucial for solving related problems. These principles provide the foundation for calculating important values such as area, perimeter, and angles, allowing for a more comprehensive understanding of the figure’s properties. Theorems often serve as the stepping stones to deeper insights, guiding the process of deriving unknown values.

One key aspect of working with these geometric shapes is the application of established formulas that relate the different components, such as the radius, diameter, and circumference. In addition to basic formulas, there are several theorems that describe how these components interact, especially when lines, tangents, and arcs are involved.

To solve problems efficiently, it’s essential to understand both the theoretical concepts and the practical application of formulas. By learning these, you will be able to navigate through a wide range of problems, from simple calculations to more complex geometric proofs.

Step-by-Step Solutions for Circles Test

Breaking down complex problems involving round geometric shapes into smaller, manageable steps is crucial for achieving accurate results. This approach ensures that every aspect of the problem is carefully considered and properly solved. In this section, we will guide you through detailed solutions, making it easier to understand the process from start to finish.

The key to solving these types of problems is following a systematic procedure. By understanding the relationships between various elements like radius, diameter, and angles, you can apply the right formulas and methods to arrive at the correct solution. Below are the general steps for solving most questions related to these shapes:

1. Identify the known values (radius, diameter, angle, etc.) from the problem.
2. Determine which formula or theorem applies to the situation.
3. Substitute the known values into the appropriate formula.
4. Perform the necessary calculations carefully, following the correct order of operations.
5. Double-check your results for accuracy.

For more complex problems, it may be necessary to break down the calculations into smaller parts. For example, solving for an angle might require first calculating the length of an arc or the measure of a segment. Here are some helpful tips:

• Draw a diagram to visualize the problem and the relationships between elements.
• Label all known and unknown values clearly.
• Use consistent units throughout the calculations.
• When working with angles, remember the key properties of tangents, secants, and chords.

By following these steps and using the right approach, you can effectively solve any problem involving geometric shapes with curved boundaries. With practice, these steps will become second nature, allowing you to confidently tackle more challenging problems.

Common Mistakes in Circle Problems

When solving problems related to geometric shapes with curved boundaries, it’s easy to overlook crucial details or misapply formulas. These mistakes can lead to incorrect results, especially when dealing with complex relationships between components like radii, tangents, and arcs. Understanding common errors can help you avoid them and improve your problem-solving accuracy.

Incorrect Formula Application

One of the most frequent mistakes is using the wrong formula for a given situation. This often happens when the problem involves multiple relationships, and it’s easy to confuse the formulas for area, perimeter, or angle calculations. It’s important to clearly identify the values you have and choose the appropriate equation for each specific case.

Misunderstanding Angle Relationships

Another common mistake occurs when working with angles, particularly when tangents, secants, or chords are involved. The relationships between these elements can be tricky, and overlooking key rules can lead to errors in angle calculation. Remember to account for all relevant theorems and properties when solving for angles in a shape with curved edges.

Common Mistake	Why It Happens	How to Avoid It
Confusing the radius and diameter	Mixing up the two can result in incorrect calculations for area or circumference.	Always remember that the radius is half the diameter.
Using the wrong angle formula	Forgetting which formula applies to the specific angle (e.g., central angle vs. inscribed angle).	Review the properties of angles and identify their type before applying a formula.
Forgetting to square the radius	This can happen when calculating the area of a rounded figure.	Always double-check your formulas to ensure correct use of powers, such as in the area formula (πr²).

By paying close attention to these potential mistakes and double-checking each step, you can ensure greater accuracy when solving problems involving round geometric shapes. With practice and awareness, you’ll be able to avoid these common pitfalls and achieve more reliable results.

How to Approach Circle Test Questions

When faced with problems involving round geometric figures, it’s important to have a structured approach. These types of questions often require applying several related concepts, including distances, angles, and relationships between different elements. A clear, methodical approach helps you break down each problem and ensure you apply the correct principles at every step.

Step-by-Step Approach to Solving Problems

The key to effectively solving these problems is following a consistent process. Below are the general steps you should take when approaching questions in this area:

1. Read the problem carefully: Ensure you understand all the given information and identify what is being asked.
2. Draw a diagram: Visualize the problem by sketching the figure, labeling known values such as radii, angles, and line segments.
3. Identify the key relationships: Determine which formula or theorem applies to the problem, whether you’re calculating area, perimeter, or angles.
4. Set up the appropriate equation: Substitute known values into the formula, ensuring all units are consistent.
5. Perform the calculations: Follow the correct order of operations and solve for the unknown values.
6. Check your work: Review each step for accuracy and ensure your final answer makes sense in the context of the problem.

Helpful Tips for Efficient Problem Solving

• Always double-check your diagram to ensure all given information is correctly represented.
• Remember to use the correct units, especially when dealing with measurements like angles or lengths.
• In complex problems, break them into smaller steps to simplify the process.
• If you’re stuck, try looking for patterns in similar problems you’ve solved before.
• Don’t rush through the calculations–precision is key to obtaining the correct result.

By adopting a systematic approach and following these strategies, you can confidently tackle any problem involving round geometric figures. Practice and patience will further help refine your problem-solving skills, making each new challenge more manageable.

Important Properties of Circles Explained

Understanding the fundamental characteristics of round geometric shapes is crucial for solving problems involving these figures. These properties define how different parts of the shape–such as the boundary, center, and lines that interact with it–relate to each other. By mastering these essential concepts, you can approach problems more confidently and solve them more efficiently.

One of the most important properties is the relationship between the radius and diameter. The radius is the distance from the center to any point on the boundary, while the diameter spans the entire width of the shape, passing through the center. The diameter is always twice the length of the radius.

Another key concept is the circumference, which is the total length around the boundary of the shape. This can be calculated using the formula C = 2πr, where r represents the radius. The area inside the shape is given by the formula A = πr², which allows you to calculate the total space enclosed by the figure.

Additionally, understanding the angles formed by intersecting lines or tangents is essential for solving more complex problems. The angle between a tangent and a radius at the point of contact is always 90°, and this property is fundamental when working with problems that involve these two elements.

These key properties not only simplify calculations but also form the basis for solving more advanced problems related to round geometric shapes. Grasping them will enhance your problem-solving skills and prepare you for more challenging questions involving these figures.

Solving Angle Problems in Circles

Angle-related problems in geometric shapes with a curved boundary can be challenging, but understanding the key principles makes them much easier to tackle. These problems often involve relationships between angles formed by lines, tangents, or arcs, and they require applying theorems that govern how these angles behave. By mastering these concepts, you’ll be able to approach these problems with confidence and accuracy.

There are several types of angles commonly encountered in problems involving circular shapes. These include central angles, inscribed angles, and angles formed by tangents. Understanding the specific properties of each of these angles is essential for solving related problems correctly.

Types of Angles and Their Properties

• Central Angles: These are angles whose vertex is at the center of the shape. The measure of a central angle is equal to the measure of the arc it intercepts.
• Inscribed Angles: Angles formed by two chords that meet at a point on the boundary. The measure of an inscribed angle is half the measure of the arc it intercepts.
• Angles Between a Tangent and a Chord: The angle formed between a tangent line and a chord that passes through the point of tangency is equal to half the measure of the intercepted arc.
• Angles Formed by Secants: When two secant lines intersect outside the shape, the angle between them is half the difference between the measures of the intercepted arcs.

Step-by-Step Method for Solving Angle Problems

1. Read the Problem: Carefully note all the given information, including any angles, arcs, and line segments provided.
2. Draw a Diagram: Sketch the shape and label all known elements, such as points, lines, and angles.
3. Identify the Angle Type: Determine whether the problem involves a central angle, inscribed angle, or one of the other types of angles.
4. Apply the Correct Formula: Use the appropriate theorems or properties based on the angle type to calculate the unknown values.
5. Check for Additional Relationships: Look for any other angle relationships or congruencies that may help simplify the problem.
6. Verify Your Answer: Double-check your calculations to ensure accuracy, especially when working with angles and arcs.

By following these steps and applying the appropriate formulas, you can confidently solve angle-related problems in geometric shapes. With practice, these concepts will become second nature, and you’ll be well-prepared to handle even the most complex angle problems.

Using Radius and Diameter in Calculations

In problems involving round geometric figures, the radius and diameter are two of the most important elements to consider. These measurements help in determining key properties such as area, circumference, and even angles in certain cases. Understanding how to use the radius and diameter correctly is essential for solving these types of problems effectively.

The radius is the distance from the center to any point on the boundary, while the diameter stretches across the figure, passing through the center and touching the boundary on both sides. The diameter is always twice the length of the radius. These two measurements are closely related and serve as the foundation for various calculations.

To calculate the circumference of a round figure, you use the formula C = 2πr, where r is the radius. Alternatively, you can use the diameter with the formula C = πd, where d represents the diameter. Both formulas essentially give the same result but use different parameters for convenience, depending on the information provided.

For the area, the formula is A = πr², where r is the radius. This formula shows that the area is directly proportional to the square of the radius, meaning that even small changes in the radius can significantly affect the total area. If the diameter is given instead, you can find the area by first dividing the diameter by 2 to obtain the radius, and then applying the same formula.

Using these two measurements allows for quick and efficient calculations in problems involving various properties of round shapes. Whether determining the space inside the figure or the distance around it, the radius and diameter are fundamental to the process and must be handled with care in any calculation.

Application of Circle Area Formula

The formula for calculating the area of a round shape is one of the most useful and frequently applied concepts in geometry. By using the relationship between the radius and the enclosed space, we can easily determine how much area a circular figure occupies. This formula is essential in various practical applications, from real-world problems involving land measurement to scientific calculations in physics and engineering.

To find the area, the basic formula is A = πr², where r is the radius of the figure. This simple yet powerful equation helps calculate the total space within the boundary of a circle, which is vital for numerous calculations in fields like architecture, astronomy, and environmental science.

Practical Uses of the Area Formula

One common use of the area formula is in determining the amount of space a circular object, such as a garden or a tank, occupies. For example, if you are designing a round garden, you can easily calculate the area to understand how much soil is needed for planting. Similarly, in engineering, knowing the area of circular sections in pipes or machines is essential for determining flow rates and material requirements.

Using the Formula for Irregular Shapes

The circle area formula can also be extended to situations where the circular section is part of a larger, irregular shape. For instance, when calculating the area of a circular section within a square or a circular sector, you can still apply the formula by considering the relevant radius. In some cases, the circle area formula helps simplify complex calculations, breaking down larger figures into manageable sections.

In conclusion, the circle area formula is not just a theoretical concept but a practical tool used in a wide range of disciplines. Whether you’re measuring the space inside a round object or calculating the material required for a project, this formula plays an important role in both everyday applications and specialized fields of study.

Perimeter of Circles and Related Problems

When dealing with round shapes, calculating the perimeter, often referred to as the circumference, is essential for understanding the boundary length. This measurement plays a significant role in a variety of practical situations, from determining the amount of material needed to surround a circular area to calculating the distance around an object. The perimeter is directly related to the radius or diameter, which simplifies the process of determining this important value.

The general formula for calculating the perimeter (circumference) of a circular shape is C = 2πr, where r represents the radius. If the diameter is known instead of the radius, the formula can be written as C = πd, where d is the diameter. Both formulas essentially give the same result but use different parameters depending on the given information.

Solving Problems Involving Perimeter

One common problem involving perimeter is finding the distance around circular tracks or fields. For example, if you need to know how much fencing is required to surround a circular garden, knowing the radius allows you to easily calculate the total perimeter using the appropriate formula. Similarly, when working with circular shapes in manufacturing or construction, perimeter calculations are crucial for determining the amount of material to use for borders or edges.

Application in Real-World Scenarios

In real-world scenarios, the perimeter of round objects is also essential for calculating distances in transportation and navigation. For instance, the circumference of a round object like a wheel determines the distance traveled in one full rotation. Whether calculating the material needed for a circular border or determining the distance around an object for navigation, understanding how to calculate and apply the perimeter is vital for solving these types of problems.

Understanding Tangents and Secants

In geometry, understanding the relationship between lines and round shapes is fundamental to solving many problems. Two types of lines that interact with circular objects are tangents and secants. These lines have distinct properties, yet both play crucial roles in geometric calculations and proofs.

A tangent is a line that touches a circular figure at exactly one point. Unlike other lines, it does not pass through the shape but rather “grazes” it at a single location. This point where the tangent touches the boundary is called the point of tangency. A key property of tangents is that they are always perpendicular to the radius drawn to the point of tangency.

On the other hand, a secant is a line that intersects a circular object at two distinct points. Unlike the tangent, the secant passes through the circle, dividing it into two segments. The interaction between the secant and the figure is useful in various geometric theorems and problems, particularly in finding lengths and areas related to the circular shape.

Properties of Tangents

Some important properties of tangents include:

• The distance from the center of the circle to the point of tangency is always equal to the radius.
• Tangents from a common external point to a circle are always equal in length.
• The angle formed between the tangent and the radius at the point of tangency is always 90 degrees.

Applications of Secants

Secants are often used to solve problems that involve intersecting lines and circles. They are especially useful when working with chord lengths, sectors, or angles between lines. A common application of secants is in determining the lengths of segments created by the intersection of a line with a circular figure. The secant-tangent theorem, for example, relates the lengths of secant segments and tangent lines, allowing for deeper insights into geometric relationships.

Circles in Coordinate Geometry

In coordinate geometry, the study of round shapes within a defined plane is essential for solving various problems related to distances, angles, and positions. Understanding the equation of a round figure in a two-dimensional plane allows for precise analysis of geometric relationships and is fundamental in both theoretical and applied mathematics.

The general equation for a round shape in a coordinate plane is given by (x – h)² + (y – k)² = r², where h and k represent the coordinates of the center, and r is the radius. This formula allows one to determine the set of all points that are equidistant from a fixed center point. By manipulating this equation, one can derive various properties, including distances, tangents, and other geometric figures that interact with the shape.

Equation of a Round Shape

The equation (x – h)² + (y – k)² = r² describes a circle where:

• h is the x-coordinate of the center.
• k is the y-coordinate of the center.
• r is the radius, which determines the size of the figure.

Applications in Coordinate Geometry

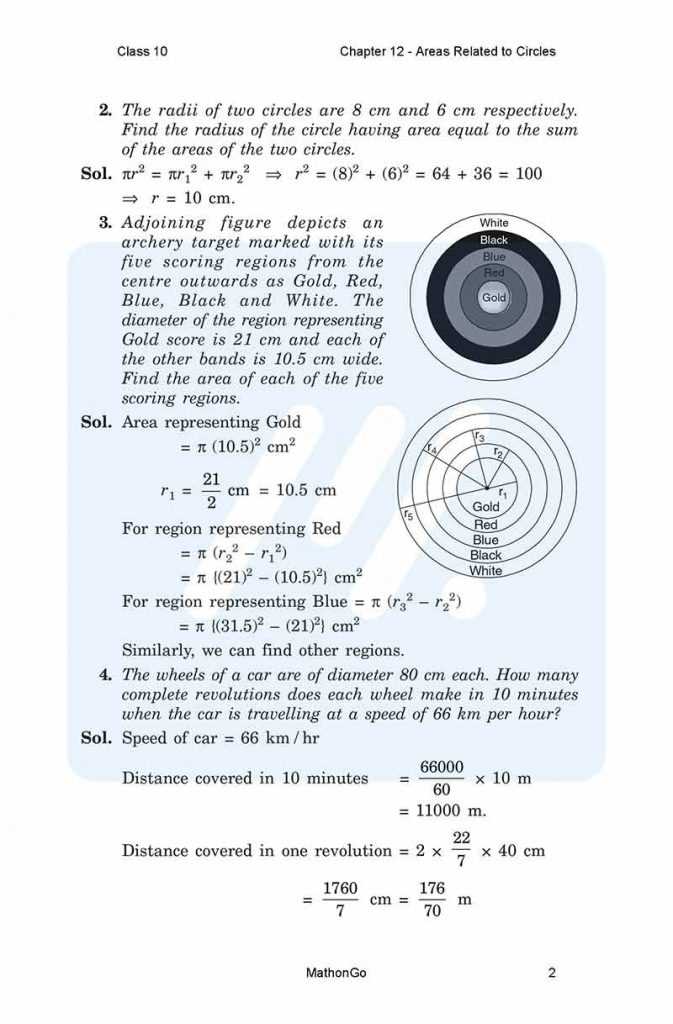
In coordinate geometry, this equation is crucial for solving problems such as finding the intersection points of lines and round shapes, determining the distance between two points, and identifying tangents. It is also used to calculate the center and radius when only certain points or distances are known, enabling one to reconstruct the geometric properties of the figure.

• Finding the center and radius: Given a circle’s equation, the center is at (h, k) and the radius is r.
• Distance from a point to the circle: By using the equation, one can calculate the shortest distance from any external point to the nearest edge of the circle.
• Intersection with a line: Solving the system of equations involving the equation of a line and the equation of a circle can give the points of intersection, if they exist.

Exploring Chords and Arcs in Circles

In the study of round shapes, two essential elements are the straight line segments that connect two points on the boundary and the sections of the boundary itself. These elements play a key role in understanding the structure and properties of these geometric forms. By examining the relationships between these segments and portions of the shape, one can uncover various fundamental properties that are crucial for both theoretical exploration and practical applications.

A chord is a straight line connecting two distinct points on the circumference of the shape, while an arc is the curved segment of the boundary between these two points. Both elements are interrelated, and their lengths or measurements often provide useful information about the figure, such as the central angle, the area enclosed, and other geometric properties.

Understanding Chords and Their Properties

A chord always divides the shape into two segments, and depending on the position of the chord, these segments may have different properties. For instance, a chord passing through the center of the shape is known as a diameter, and it has unique characteristics, such as being the longest possible chord in the shape.

• Length of a Chord: The length of a chord can be determined using the distance formula, or through trigonometric methods, depending on the known information.
• Perpendicular from the Center: A perpendicular dropped from the center of the figure to a chord bisects the chord, dividing it into two equal parts.

Exploring Arcs and Their Significance

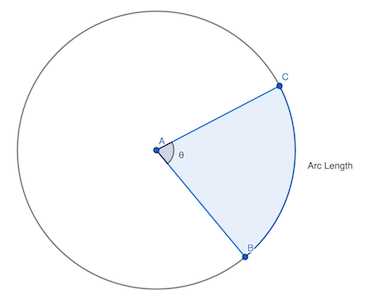
Arcs represent the curved portions of the boundary between two points, and their lengths are proportional to the angle at the center subtended by the arc. The measurement of arcs is crucial in calculating the area of sectors or determining the length of the boundary over a given interval.

• Major and Minor Arcs: The arc with the smaller central angle is called the minor arc, while the larger one is the major arc.
• Arc Length Formula: The length of an arc can be calculated using the formula: Arc length = (θ/360) × 2πr, where θ is the central angle in degrees and r is the radius of the shape.

Using Geometry Software for Circle Tests

Modern technology provides valuable tools that can assist in solving complex geometric problems. Geometry software allows for interactive visualization and accurate calculation, making it easier to understand and analyze various shapes, their properties, and relationships. These tools can enhance learning and improve the efficiency of solving problems related to round shapes, angles, and other geometric elements.

By using specialized programs, students and professionals alike can experiment with different parameters and instantly observe the effects of changes on the figure. This dynamic approach allows for better insight into geometric principles and the relationships between components like radii, tangents, and chords.

Benefits of Geometry Software

One of the key advantages of geometry software is its ability to visually represent complex problems. This visual representation helps users see how changes in one aspect of a shape affect other properties. For example, modifying the radius can show its impact on circumference or area, providing immediate feedback on the mathematical relationships involved.

• Interactive Learning: Allows users to experiment with various figures and manipulate parameters in real-time to gain a deeper understanding of geometric principles.
• Accuracy: Provides precise calculations and measurements, eliminating human error and improving the reliability of results.
• Time Efficiency: Speeds up the problem-solving process by automating repetitive calculations and providing quick visual feedback.

Common Geometry Software Tools

Several software options are available for those looking to improve their geometric problem-solving skills. These programs range from simple tools to advanced platforms, each with unique features tailored to different levels of users.

• GeoGebra: A popular free tool that offers interactive features for algebra, geometry, and calculus. It is widely used by students and educators for exploring geometric shapes and their properties.
• Desmos: Primarily known for its graphing calculator, Desmos also provides powerful tools for visualizing geometric figures and performing calculations related to them.
• Cabri Geometry: A dynamic geometry software that allows users to construct and manipulate geometric figures in a visually interactive environment.

Advanced Circle Problems and Solutions

Solving complex geometric problems often requires a deeper understanding of the relationships between different elements of a figure. Advanced problems may involve intricate calculations, multiple theorems, and the application of various principles such as angles, tangents, and arcs. These problems typically require not only strong mathematical skills but also strategic thinking and careful consideration of how different properties interact with each other.

In this section, we explore more challenging problems that push the limits of geometric understanding, focusing on situations that involve advanced formulas, reasoning, and visualizing multiple aspects of a shape simultaneously. The key to solving these problems is often breaking down complex scenarios into smaller, more manageable steps and applying fundamental principles in creative ways.

Example 1: Finding the Length of a Chord

Consider a problem where you are given a circle with a known radius and the distance from the center to a point on the circle. The goal is to find the length of a chord that connects two points on the circumference.

Given Information	Formula	Solution
Radius: 10 units, Distance from center to chord: 6 units	Chord Length = 2 * √(r² – d²)	Chord Length = 2 * √(10² – 6²) = 2 * √(100 – 36) = 2 * √64 = 2 * 8 = 16 units

In this example, we used the Pythagorean theorem to calculate the length of the chord. By identifying the relationship between the radius, the distance from the center, and the chord, we were able to solve the problem systematically.

Example 2: Tangent and Secant Problem

In another advanced scenario, suppose you are given a circle with a known radius and a tangent drawn from a point outside the circle. The problem asks you to calculate the length of the secant drawn from the same point that intersects the circle at two points.

Given Information	Formula	Solution
Radius: 5 units, Distance from external point to center: 12 units, Tangent length: 9 units	Tangent² = Secant × (Secant – Tangent)	9² = Secant × (Secant – 9) → 81 = Secant² – 9×Secant → Secant² – 9×Secant – 81 = 0

This problem is a classic example of using the tangent-secant theorem to solve for the length of the secant. By setting up the quadratic equation, we can solve for the secant length and ensure that we apply the right geometric concepts to find the solution.

Tips for Scoring High on Geometry Assessments

Achieving a high score on geometry-related assessments requires both understanding the underlying concepts and practicing effective problem-solving strategies. Success depends not only on grasping key geometric principles but also on applying them efficiently under time constraints. Focusing on the most important skills and strategies can make a significant difference when tackling challenging questions. Below are some tips to help you maximize your performance and tackle geometry problems with confidence.

1. Master the Fundamental Concepts

Before attempting complex problems, ensure that you have a solid understanding of basic geometric principles. This includes familiarizing yourself with essential formulas, relationships, and theorems. Understanding the fundamentals will give you a strong foundation, allowing you to quickly identify which concepts to apply in various scenarios. Key areas to focus on include:

• Area and perimeter formulas
• Properties of tangents, chords, and secants
• Angle relationships within geometric figures
• Understanding symmetry and congruence in shapes

2. Practice Regularly with Sample Problems

Repetition is crucial to success in any subject, especially in geometry. Regular practice will not only reinforce your knowledge but also help you develop the speed and accuracy needed to solve problems under timed conditions. Start with simpler problems to build confidence, and gradually move on to more complex scenarios. By practicing a variety of problem types, you’ll become familiar with different methods of solution and learn to identify patterns quickly.

Additionally, time yourself while solving problems to get used to working within a set time frame. This will help you manage your time more efficiently during the actual assessment, ensuring that you can complete all questions while maintaining accuracy.