Mastering the Algebra 2 Regents Exam
Preparing for standardized assessments in mathematics can be a challenging yet rewarding experience. These tests are designed to evaluate a student’s grasp of critical concepts, problem-solving abilities, and analytical skills. Success in such assessments not only reflects your knowledge but also shapes future academic and career opportunities.
In this guide, we will focus on the essential components of the test, outlining key strategies to maximize your performance. From understanding the format to mastering complex topics, this article will provide the tools needed to confidently tackle each section. Whether you’re reviewing foundational principles or refining advanced techniques, the right approach is crucial to achieving the best possible outcome.
Algebra 2 Regents Exam Overview
The purpose of this assessment is to measure a student’s understanding of mathematical concepts and their ability to apply them in various contexts. It covers a broad range of topics that students have encountered throughout their coursework. A strong performance indicates proficiency in the critical skills required for further study in mathematics and related fields.
This particular test is structured to challenge students on both basic and advanced principles. The format typically includes a combination of multiple-choice questions and open-ended problems, each designed to assess different aspects of mathematical knowledge. Successful preparation involves mastering both fundamental techniques and more complex problem-solving strategies.
| Section | Content | Weight |
|---|---|---|
| Multiple Choice | General concepts, calculations, and theory | 50% |
| Open-Ended Problems | Complex problem-solving, reasoning, and application | 50% |
Understanding the test structure and content distribution is essential for effective preparation. Each section demands a specific approach, with multiple-choice questions often testing quick recall of formulas and concepts, while open-ended problems require deeper analysis and logical reasoning.
What to Expect on Exam Day
On the day of the assessment, it is important to be well-prepared both mentally and physically. The test environment will be structured to allow you to demonstrate your knowledge and problem-solving abilities. You will encounter a mix of question types, each designed to assess your understanding of various mathematical concepts.
Before the test begins, you will be given instructions on how to complete each section. Multiple-choice questions will challenge your ability to quickly recall formulas and perform calculations, while open-ended problems will require deeper analysis and clear written explanations of your reasoning process. Be prepared to use mathematical reasoning and critical thinking throughout the entire test.
It is essential to manage your time effectively. The entire assessment will be timed, and each section will have a set amount of time to complete. Stay calm, pace yourself, and ensure that you allocate enough time to answer every question.
Key Topics Covered in Algebra 2
The assessment focuses on a variety of mathematical concepts that students are expected to master throughout their coursework. These topics are carefully selected to evaluate your understanding of both foundational and advanced principles. A strong grasp of these areas is essential for success, as they build the critical skills necessary for more complex subjects in mathematics and related fields.
Among the key areas covered are functions, polynomials, and rational expressions, which form the backbone of the test. You will also encounter topics related to quadratic equations, exponential and logarithmic functions, and systems of equations. Mastery of these concepts will allow you to confidently approach a wide range of problems, demonstrating both your knowledge and problem-solving abilities.
In addition to these topics, you will need to apply your understanding to real-world problems, interpreting data and using mathematical models to make predictions. Being able to translate abstract concepts into practical applications is a crucial skill that will be tested throughout the assessment.
Understanding the Exam Format
The structure of the test is designed to assess a wide range of mathematical skills, from basic knowledge to advanced problem-solving. It consists of different types of questions, each testing specific abilities. The format is meant to provide a comprehensive evaluation of how well you have mastered key concepts and how effectively you can apply them in various situations.
The test is typically divided into two main sections: multiple-choice questions and open-ended problems. The first section includes a series of questions with predefined answer choices, aimed at testing your understanding of fundamental concepts and your ability to quickly apply formulas. The second section consists of problems that require more detailed solutions, where you will need to show your work and explain your reasoning clearly.
Each section is timed separately, and careful time management is crucial to ensure you can complete all questions. While the multiple-choice part is designed for quicker responses, the open-ended section allows more time for thoughtful analysis and step-by-step problem solving.
How to Create a Study Plan
Creating an effective study plan is a critical step in preparing for any assessment. A well-structured schedule helps you manage your time efficiently, focus on key topics, and ensure that you are fully prepared for each section of the test. The goal is to break down the material into manageable chunks, allowing you to review thoroughly without feeling overwhelmed.
Start by identifying the areas where you need the most improvement. Review past lessons and tests to pinpoint which topics require more attention. Once you have a clear understanding of the material, allocate time to each topic based on its difficulty and your level of familiarity with it. Be sure to include regular review sessions to reinforce what you’ve learned.
Incorporate a mix of study techniques, such as practice problems, flashcards, and group study sessions, to keep your sessions varied and engaging. Additionally, make sure to schedule breaks to avoid burnout and stay focused. With a solid plan in place, you can approach your studies with confidence and increase your chances of success.Error in message stream
Effective Study Resources for Success
To achieve success in any academic assessment, using the right study tools is essential. A variety of resources are available to help reinforce concepts, improve problem-solving skills, and build confidence. The key is selecting the most effective materials tailored to your learning style and focusing on the areas that require the most attention. Whether through interactive platforms, practice problems, or detailed guides, the right tools can make all the difference in mastering the material.
One of the most efficient ways to prepare is by utilizing practice problems. These not only simulate the type of questions you will encounter but also help you understand how to approach various types of challenges. Additionally, comprehensive study guides break down complex topics into manageable sections, making it easier to focus on one area at a time.
| Resource Type | Description | Example Platforms |
|---|---|---|
| Practice Problems | Numerous problems covering a range of topics help reinforce learned concepts and improve accuracy. | Khan Academy, Mathway, Wolfram Alpha |
| Interactive Quizzes | Timed quizzes provide an opportunity to practice under exam-like conditions. | |
| Study Guides | In-depth guides focus on breaking down topics and key strategies for problem-solving. | McGraw-Hill, Pearson |
| Video Tutorials | Visual learners can benefit from step-by-step video explanations of difficult concepts. | YouTube, Coursera, Udemy |
Combining these resources with consistent practice and time management will significantly improve your preparation. Creating a study schedule and sticking to it ensures that each topic is given the attention it needs. Staying organized and setting clear goals will allow you to make steady progress and increase your chances of success.
Top Strategies for Time Management
Effective time management is crucial when preparing for any academic challenge. It helps prioritize tasks, reduces stress, and ensures that each topic receives the necessary focus. Developing a structured approach to studying not only maximizes productivity but also ensures sufficient time for review and rest. Here are some strategies to manage your time more effectively:
- Create a Study Schedule: Organize your time by breaking down study sessions into manageable chunks. Allocate specific hours for each topic and stick to the plan to avoid procrastination.
- Prioritize Tasks: Focus on areas where you feel less confident or those that are more complex. Tackling difficult concepts early ensures you don’t run out of time when preparing.
- Use Timed Sessions: Set a timer for each study block to simulate real-time conditions. This practice helps you stay focused and teaches you how to pace yourself under pressure.
- Take Regular Breaks: Avoid burnout by incorporating short breaks into your schedule. A 5-10 minute break every 30-45 minutes of study keeps your mind fresh and improves retention.
- Review Progress: At the end of each week, assess what you’ve covered and adjust your schedule accordingly. This will help you stay on track and make any necessary adjustments.
By following these strategies, you can enhance your ability to manage time efficiently and prepare thoroughly for any assessment. Staying organized and focused will boost your chances of success and minimize last-minute stress.
Common Mistakes to Avoid
When preparing for an academic assessment, it’s easy to fall into certain pitfalls that can hinder progress. Recognizing and avoiding these common mistakes can significantly improve your chances of success. Many learners make these errors due to lack of focus, poor planning, or rushing through tasks. By being mindful of these common missteps, you can better streamline your preparation and enhance your performance.
Poor Time Management
- Procrastination: Delaying study sessions until the last minute increases stress and reduces the quality of preparation.
- Overloading Sessions: Trying to cover too many topics in a single study block can lead to burnout and lower retention.
- Ignoring Breaks: Skipping breaks can cause mental fatigue, resulting in decreased focus and productivity.
Neglecting to Review Mistakes
- Not Analyzing Errors: Failing to review mistakes in practice problems means you may repeat them in future exercises.
- Relying on Guesswork: Guessing answers without understanding the underlying concepts can lead to misunderstandings and hinder long-term learning.
- Skipping Practice: Avoiding practice questions and focusing solely on theory can leave you unprepared for applying concepts under time constraints.
Avoiding these common mistakes will help you stay on track and make the most of your study time. Stay organized, review regularly, and prioritize your learning to ensure better outcomes.
How to Tackle Multiple Choice Questions
Multiple choice questions often appear in various academic assessments and can be tricky if approached without a strategy. With careful attention and a systematic approach, you can effectively navigate through these types of questions and improve your chances of selecting the correct answer. The key is to read carefully, eliminate incorrect options, and use logical reasoning to make the best choice.
Step-by-Step Approach
- Read the Question Carefully: Before looking at the answer choices, fully understand what the question is asking. Pay attention to key details and any qualifiers like “always,” “never,” or “most likely.”
- Eliminate Obvious Wrong Answers: Start by crossing out the options that are clearly incorrect. This increases the probability of selecting the correct answer from the remaining choices.
- Look for Clues in the Question: Sometimes the question or other options can provide hints to the correct answer. Look for subtle clues that may guide your decision.
- Use Logic and Process of Elimination: If unsure, use logical reasoning to narrow down the options. Even if you can’t pinpoint the exact answer, narrowing the choices can improve your odds of guessing correctly.
Handling Difficult Questions
- Don’t Rush: Take your time with each question. If you’re stuck, move on to the next one and return later with a fresh perspective.
- Trust Your First Instinct: Research shows that the first answer you choose is often the most accurate. If you change your answer, make sure you have a clear reason for doing so.
- Review Before Submitting: If time permits, double-check your answers. Sometimes you may spot a mistake or remember an important detail that you missed the first time.
With these strategies, you can approach multiple choice questions with confidence and make more informed decisions, even when the material feels challenging.
Tips for Solving Open-Ended Problems
Open-ended questions require a deeper understanding of the material, as they often do not have a single correct answer but demand a process or reasoning to arrive at a solution. These types of problems test your ability to apply concepts creatively and think critically. To successfully solve them, it’s important to approach the problem methodically and to demonstrate your reasoning clearly.
Step-by-Step Approach
- Understand the Problem: Carefully read the question to identify what is being asked. Pay attention to any conditions or restrictions provided in the problem.
- Break It Down: Divide the problem into smaller, manageable parts. This makes it easier to approach and ensures that you don’t miss any important details.
- Use Diagrams or Charts: Visual aids can help clarify complex concepts and provide a clearer path to solving the problem.
- Show Your Work: Clearly write out each step you take. Not only does this help ensure you don’t make mistakes, but it also allows you to review your reasoning later.
Managing Time and Stress
- Stay Organized: Keep track of your time and avoid spending too long on any one problem. If you get stuck, move on and return to it later.
- Focus on Key Concepts: Stick to the core principles that are relevant to the problem. Avoid overcomplicating things or adding unnecessary steps.
- Double-Check Your Solution: Once you have a solution, review it to ensure it makes sense in the context of the problem and meets the requirements.
By following these strategies, you can approach open-ended problems with confidence and demonstrate clear, logical thinking in your solutions.
Using Practice Exams to Prepare
One of the most effective ways to prepare for any academic assessment is by practicing with mock tests. These simulated exercises allow you to familiarize yourself with the format of the questions, identify areas of weakness, and fine-tune your time management skills. Practicing under real-time conditions helps reduce anxiety and boosts confidence, ensuring that you are well-prepared when the actual test day arrives.
Why Practice Tests Are Important
- Familiarity with Question Formats: Practice exams expose you to the types of questions you will encounter, allowing you to become comfortable with the format.
- Identifying Weak Areas: Through practice, you can pinpoint the topics where you need more focus and dedicate extra time to reviewing those concepts.
- Time Management: Simulating the time constraints of the actual assessment helps improve your ability to allocate time appropriately and avoid rushing during the test.
- Improving Confidence: Regular practice allows you to track your progress, which helps to reduce test-related stress and increases your overall confidence.
How to Make the Most of Practice Tests
- Simulate Real Conditions: Take practice tests under timed conditions to replicate the pressure of the actual assessment.
- Review Mistakes: After completing each practice test, thoroughly review your incorrect answers to understand why they were wrong and learn from them.
- Take Multiple Practice Tests: The more practice tests you take, the more comfortable and familiar you will become with the content and format.
- Track Your Progress: Keep track of your scores and note any improvements or areas that still need work. This will help you stay motivated and focused on your preparation.
| Resource Type | Purpose | Examples |
|---|---|---|
| Official Practice Tests | Provide a close approximation to the real assessment. | Official test prep books, school-provided tests |
| Online Practice Platforms | Offer interactive questions and immediate feedback on performance. | Khan Academy, Quizlet, TestPrepOnline |
| Printable Worksheets | Allow for offline practice and can be used for targeted review. | PDF worksheets, printable practice sets |
By incorporating practice exams into your study routine, you can significantly improve your performance and arrive on test day feeling confident and well-prepared.
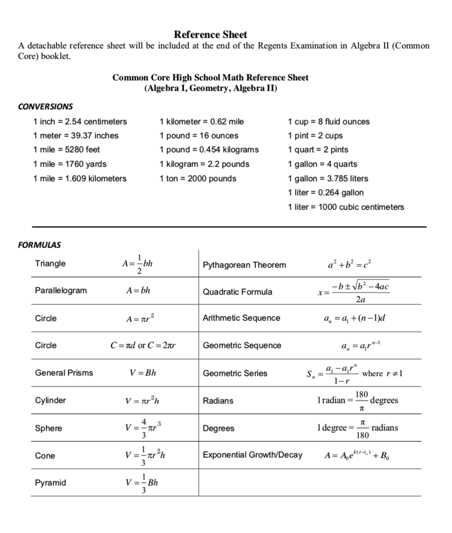
Important Formulas to Know
Having a solid grasp of key mathematical formulas is essential for solving a wide range of problems. These formulas serve as tools that simplify complex concepts and provide a structured approach to finding solutions. Memorizing and understanding these critical formulas can greatly improve problem-solving efficiency and accuracy, especially when dealing with various types of problems in an assessment setting.
Basic Mathematical Formulas
- Quadratic Formula: Used to solve quadratic equations.
x = (-b ± √(b² - 4ac)) / 2ad = √((x₂ - x₁)² + (y₂ - y₁)²)a² + b² = c²m = (y₂ - y₁) / (x₂ - x₁)Advanced Formulae
- Binomial Theorem: Expands binomials raised to any power.
(a + b)ⁿ = Σ (nCk * aⁿ⁻ᵏ * bᵏ)y = y₀ * e^(kt)logₐ(x) = y ↔ aᵧ = xMastering these formulas is not only essential for exams but also for applying mathematical concepts in real-world problems. By practicing and memorizing them, you can approach even the most challenging questions with confidence and clarity.
How to Stay Calm During the Test
Staying calm during an assessment is crucial for performing at your best. Test anxiety can impair your ability to think clearly and make decisions under pressure. By implementing certain techniques and strategies, you can manage stress and remain composed throughout the entire process. The key lies in maintaining focus, staying organized, and trusting in your preparation.
Pre-Test Preparation
- Practice Deep Breathing: Deep breathing exercises can help reduce stress and clear your mind. Taking slow, deep breaths before and during the test can help maintain focus.
- Get Plenty of Rest: Ensure you have a good night’s sleep before the test. Fatigue can increase anxiety and affect concentration.
- Eat a Balanced Meal: Eating a healthy, nutritious meal before the test can stabilize energy levels and prevent distractions caused by hunger.
- Arrive Early: Arriving early allows you to settle in, reduce anxiety, and avoid the stress of rushing.
In-Test Strategies
- Start with Easy Questions: Begin with the questions you are most confident about. This builds momentum and can help reduce feelings of being overwhelmed.
- Take Breaks if Needed: If allowed, take brief pauses to stretch and refocus. A few seconds of rest can help reset your mind.
- Use Positive Self-Talk: Replace negative thoughts with affirmations like “I am prepared” or “I can do this.” A positive mindset helps reduce anxiety.
- Keep Track of Time: Stay aware of the time, but don’t obsess over the clock. If you get stuck, move on and come back later.
By following these strategies, you can maintain a calm and clear mindset, giving yourself the best chance to succeed in the assessment.
Understanding Grading and Scoring
Grading and scoring systems are designed to assess a student’s understanding and application of the material. Knowing how these systems work helps you set realistic expectations and focus your efforts on areas that carry more weight. These systems often involve a combination of points for correct answers, partial credit for showing correct processes, and penalties for incorrect responses. Understanding the breakdown of how each section is scored can guide your study approach and help you perform more effectively.
Types of Grading Criteria
- Multiple Choice Questions: Typically, these are scored based on the number of correct answers. Each correct answer earns a set number of points, while incorrect answers may not affect your score.
- Open-Ended Questions: These are scored based on both the accuracy of your solution and the clarity of your reasoning. Showing the process can often earn partial credit, even if the final answer is incorrect.
- Bonus Points: Some tests offer extra points for completing additional questions or tasks beyond the required ones. Taking advantage of these opportunities can boost your overall score.
Scoring Breakdown
- Point System: Most assessments assign specific point values to each question or section. The total score is the sum of the points earned across all sections.
- Weight of Sections: Some sections, such as complex problem-solving tasks, may be weighted more heavily than others. Understanding this can help you prioritize your efforts during preparation.
- Partial Credit: In many cases, even if you do not reach the correct final answer, demonstrating the correct approach can earn partial credit. Be sure to show your work clearly and logically.
By familiarizing yourself with how grading and scoring systems are structured, you can approach your study sessions more strategically, ensuring that you maximize your potential for success.
Improving Your Problem-Solving Skills
Problem-solving is a critical skill that requires a combination of logic, creativity, and persistence. Enhancing your ability to tackle various types of questions involves developing a structured approach to breaking down complex issues, identifying key information, and applying appropriate strategies. By practicing consistently and refining your techniques, you can become more confident and efficient in addressing even the most challenging problems.
Steps to Enhance Problem-Solving Techniques
- Understand the Problem: Carefully read the question to ensure you understand what is being asked. Identify key information and determine what is given and what needs to be found.
- Break the Problem into Smaller Parts: If a problem seems overwhelming, divide it into smaller, manageable steps. Solving one part at a time makes the process less daunting.
- Choose the Right Strategy: Depending on the problem type, choose an appropriate approach, such as using formulas, drawing diagrams, or applying logical reasoning. Familiarize yourself with various methods and adapt accordingly.
- Check Your Work: After solving the problem, review your steps to ensure there are no errors. Revisiting your calculations can often reveal mistakes you might have missed initially.
Practicing Problem-Solving Techniques
- Work on a Variety of Problems: The more problems you solve, the more experience you gain. Practice with different types of questions to strengthen your adaptability and familiarity with various concepts.
- Learn from Mistakes: If you make an error, analyze where you went wrong and understand why the correct method works. Learning from mistakes is an essential part of the problem-solving process.
- Seek Alternative Methods: Sometimes, there is more than one way to approach a problem. Experiment with different strategies and find the methods that work best for you.
By continuously improving your problem-solving abilities, you will not only perform better in assessments but also develop critical thinking skills that will benefit you in everyday life.
The Role of Graphing Calculators
Graphing calculators are powerful tools that help students visualize mathematical concepts and solve complex problems with greater ease. These calculators provide a way to quickly graph equations, analyze data, and explore functions interactively. They are particularly useful for tasks involving large sets of data, complicated formulas, or advanced graphing techniques, making them an invaluable resource for improving understanding and efficiency in mathematical studies.
Benefits of Using Graphing Calculators
- Visualization: Graphing calculators allow students to see the graphical representation of equations, helping them understand the relationship between variables and the behavior of functions.
- Efficient Problem Solving: With built-in functions for solving equations, graphing calculators can quickly compute values, such as roots or intersections, saving time during problem-solving.
- Exploration: These devices enable students to experiment with different values and parameters, offering an interactive approach to learning that reinforces mathematical concepts.
- Data Analysis: Graphing calculators are excellent for performing regression analysis, plotting statistical data, and exploring trends, which is especially beneficial in higher-level math and applied sciences.
Tips for Effective Use of Graphing Calculators
- Learn the Functions: Familiarize yourself with the calculator’s capabilities, such as graphing, solving equations, and using statistical features. Understanding how to access and use these tools will enhance your problem-solving ability.
- Double-Check Results: While graphing calculators are accurate, it’s still important to verify results by using other methods when possible. Rely on the calculator as a tool, but not as the sole solution.
- Practice with Settings: Experiment with different settings, such as the viewing window for graphs, to improve how you interpret and analyze graphical outputs. Adjusting settings can reveal more information and help clarify complex functions.
By incorporating graphing calculators into your study routine, you can gain deeper insights into mathematical concepts and improve both your understanding and performance in solving complex problems.
Post-Exam: What Comes Next?
After completing an assessment, it’s important to reflect on the experience and understand the next steps. The period following the test is an opportunity for both evaluation and preparation for future challenges. This time can be used to review performance, identify areas for improvement, and set new goals for further academic or personal growth. Understanding what comes after the test can help reduce any anxiety and put your efforts into perspective as you continue to progress.
Once the results are available, take the time to carefully analyze the feedback. If there are areas where you struggled, use this as a learning opportunity. Review the concepts that were most challenging, and consider seeking additional resources or assistance to strengthen your understanding. Additionally, celebrate the areas where you performed well, as this will help build confidence for upcoming tasks.
It’s also helpful to stay organized and keep track of any upcoming projects or assessments. Being proactive about future deadlines and maintaining good study habits will ensure continued success. Lastly, if you’re planning for further academic pursuits or career goals, now is a good time to reflect on your path and consider the next steps in your educational journey.
How to Review Your Results
Reviewing your results after completing an assessment is a crucial step in improving your performance and understanding your strengths and weaknesses. This process allows you to identify areas where you excelled and where you may need additional practice or focus. By approaching the review strategically, you can make the most out of the feedback and prepare more effectively for future challenges.
Start by carefully going through each question or task you answered. For the ones you got wrong, try to understand why. Did you misinterpret the question, make a simple calculation error, or fail to apply the correct method? Take note of any recurring mistakes so that you can work on those specific areas. If you don’t understand why you made an error, consider discussing it with a teacher or a peer to gain clarity.
Next, assess the questions you answered correctly. While it’s important to focus on mistakes, reviewing correct answers helps reinforce good habits and solidify your knowledge. Pay attention to the strategies you used and consider if there are ways to apply those strategies more efficiently in the future.
Lastly, use the review process as a learning opportunity. If the results show that certain topics or types of problems are consistently challenging, dedicate time to reviewing those concepts in depth. Practice more problems and explore different resources to strengthen your understanding.