​

Preparing for a major assessment in mathematics requires a strong grasp of essential principles and problem-solving techniques. This section will guide you through the critical topics that are commonly tested and provide insights into how to approach each type of question effectively. Whether you’re tackling equations, graphing, or working with functions, understanding the core ideas is crucial for achieving success.

As you approach your review, focus on building confidence in key areas like solving equations, understanding relationships between variables, and applying formulas to solve real-world problems. These skills will not only help you in the test but also lay a solid foundation for future mathematical challenges.

Strategic preparation involves more than just memorizing formulas; it’s about knowing how to apply them in various contexts. By practicing different problem types, you will be able to recognize patterns and solve complex problems more efficiently. This approach will give you the tools to tackle any question with ease.

Preparing for a major assessment in mathematics requires a strong grasp of essential principles and problem-solving techniques. This section will guide you through the critical topics that are commonly tested and provide insights into how to approach each type of question effectively. Whether you’re tackling equations, graphing, or working with functions, understanding the core ideas is crucial for achieving success.

As you approach your review, focus on building confidence in key areas like solving equations, understanding relationships between variables, and applying formulas to solve real-world problems. These skills will not only help you in the test but also lay a solid foundation for future mathematical challenges.

Strategic preparation involves more than just memorizing formulas; it’s about knowing how to apply them in various contexts. By practicing different problem types, you will be able to recognize patterns and solve complex problems more efficiently. This approach will give you the tools to tackle any question with ease.

Understanding Key Mathematical Concepts

Mastering fundamental mathematical ideas is essential for solving problems efficiently. These core principles serve as the building blocks for more advanced topics and ensure that you can approach any question with a clear strategy. A solid understanding of the key concepts will allow you to break down complex problems into manageable steps.

Topics such as working with variables, solving equations, and recognizing relationships between different quantities are at the heart of many problems. By focusing on how these elements interact, you can develop a deeper understanding of mathematical structures and improve your problem-solving abilities.

Once you familiarize yourself with the main concepts, you’ll find it easier to apply them in various scenarios. Whether it’s simplifying expressions, finding solutions to equations, or interpreting graphs, having a strong grasp of the basics will boost your confidence and increase your accuracy in solving challenges.

How to Solve Linear Equations

Solving linear equations is a crucial skill in mathematics that allows you to find the value of unknown variables. These types of problems typically involve an equation where the variable is raised to the first power, and the goal is to isolate the variable on one side of the equation. With the right approach, solving such problems becomes straightforward and systematic.

To solve linear equations efficiently, follow these basic steps:

1. Simplify both sides of the equation if necessary. Combine like terms and remove any parentheses.
2. Isolate the variable by moving all terms containing the variable to one side of the equation and constant terms to the other side. Use addition or subtraction to achieve this.
3. Eliminate coefficients that are multiplying the variable by using division or multiplication.
4. Check your solution by substituting the value of the variable back into the original equation to verify its correctness.

By practicing these steps, you can become proficient at solving linear equations quickly and accurately, preparing you for more complex challenges ahead.

Exploring Systems of Equations

Systems of equations involve multiple equations that share common variables. The goal is to find values for these variables that satisfy all equations simultaneously. These problems often appear more complex than single equations, but with the right approach, they can be solved efficiently using various methods.

Methods of Solving Systems

There are several techniques for solving systems, each with its advantages depending on the problem. The most common methods include:

• Substitution Method: Solve one equation for one variable and substitute that expression into the other equation to find the remaining variable.
• Elimination Method: Add or subtract equations to eliminate one of the variables, simplifying the system into a single equation.
• Graphing: Plot both equations on a graph and identify the point of intersection, which represents the solution.

Interpreting Solutions

The solution to a system of equations can be unique, infinite, or non-existent. A unique solution occurs when the lines or curves representing the equations intersect at a single point. No solution exists if the lines are parallel, and infinitely many solutions arise when the equations represent the same line or curve.

Mastering Quadratic Functions

Quadratic functions play a significant role in mathematics, as they model many real-world scenarios. These functions involve variables raised to the second power and can be represented in a variety of forms. Mastering quadratic functions involves understanding their key characteristics and learning how to solve related problems efficiently.

The standard form of a quadratic function is usually written as ax² + bx + c = 0, where “a,” “b,” and “c” are constants, and “x” is the variable. By analyzing the function’s graph, you can gain valuable insights into its properties, such as the vertex, axis of symmetry, and the direction of the parabola’s opening.

Once you become familiar with the basic structure, solving quadratic equations becomes more intuitive. Methods like factoring, completing the square, and using the quadratic formula can help find the solutions to these equations, depending on the given context and the nature of the equation.

Key Properties of Exponents

Exponents are a fundamental concept in mathematics that help simplify the expression of repeated multiplication. Understanding the properties of exponents allows you to manipulate and simplify complex expressions efficiently. These rules provide a structured way to handle powers and are essential for solving a wide range of problems.

One of the most important properties is the product rule, which states that when multiplying two numbers with the same base, you can add their exponents. Another key rule is the power of a power, which involves multiplying exponents when raising an exponent to another power. Additionally, the quotient rule helps simplify division of terms with the same base by subtracting the exponents.

These basic properties can be combined and applied to solve more complicated expressions. Whether simplifying expressions or solving equations, knowing how to apply exponent rules will make the process faster and more efficient.

Factoring Techniques for Polynomials

Factoring is a crucial technique for simplifying expressions and solving equations that involve polynomials. By breaking down complex polynomial expressions into simpler factors, you can make calculations easier and uncover the underlying solutions to problems. Mastering these methods allows you to approach a variety of problems with confidence and efficiency.

Common Factoring Methods

There are several techniques to factor polynomials, each suited to different types of expressions. Some of the most frequently used methods include:

• Greatest Common Factor (GCF): Identify the largest factor shared by all terms in the polynomial, and factor it out.
• Factoring by Grouping: Group terms in pairs or sets, factor out common factors from each group, and then factor further if possible.
• Difference of Squares: When a polynomial is the difference between two perfect squares, it can be factored into two binomials.
• Trinomial Factoring: Factor a trinomial expression into two binomials by finding two numbers that multiply to the constant term and add to the middle coefficient.

Steps for Factoring Trinomials

Factoring trinomials typically follows these steps:

1. Identify the first and last terms in the trinomial.
2. Find two numbers that multiply to give the product of the first and last terms, while also adding to the middle coefficient.
3. Rewrite the middle term using the two numbers found, and then factor by grouping.

By practicing these methods regularly, you can become proficient at factoring polynomials quickly and accurately, making it easier to solve more advanced problems in mathematics.

Analyzing Rational Expressions

Rational expressions are fractions where both the numerator and the denominator are polynomials. Understanding how to analyze and simplify these expressions is a key skill in solving many types of mathematical problems. By recognizing the structure of rational expressions, you can simplify them, identify restrictions, and perform operations such as addition, subtraction, multiplication, and division.

Simplifying Rational Expressions

To simplify a rational expression, follow these steps:

• Factor both the numerator and denominator: Look for common factors and factor them out.
• Cancel out common factors: If the same factor appears in both the numerator and the denominator, cancel it out to simplify the expression.
• Check for restrictions: Identify any values that would make the denominator equal to zero, as these values are not allowed.

Operations with Rational Expressions

Once simplified, rational expressions can be manipulated just like numerical fractions. When performing operations on them, remember:

• Addition/Subtraction: Find a common denominator before adding or subtracting the numerators.
• Multiplication: Multiply the numerators and denominators directly, then simplify the result.
• Division: Multiply the first expression by the reciprocal of the second expression and simplify.

By mastering these techniques, you can solve problems involving rational expressions efficiently and accurately.

Solving Inequalities in Mathematics

Inequalities are mathematical expressions that show the relationship between two values when they are not necessarily equal. Solving inequalities involves finding a range of values that satisfy the given condition, rather than a single solution. These problems require similar techniques to solving equations, but with additional attention to the direction of the inequality sign.

Basic Steps for Solving Inequalities

When solving inequalities, the general process involves:

• Isolating the variable: Just like with equations, start by moving all terms involving the variable to one side of the inequality and constants to the other side.
• Performing operations: Apply addition, subtraction, multiplication, or division to both sides of the inequality to simplify it.
• Reversing the inequality sign: If you multiply or divide by a negative number, remember to flip the inequality sign to maintain the correct relationship.

Interpreting Solutions

Once you’ve isolated the variable, the solution is usually expressed as a range of values, often written in interval notation. If the inequality involves “greater than” or “less than,” the solution represents a set of values that fall within a specified range. If the inequality includes “greater than or equal to” or “less than or equal to,” the boundary points are included in the solution set.

Solving inequalities requires careful attention to detail, but with practice, you’ll gain the ability to quickly and accurately interpret the solutions.

Understanding Radical Expressions

Radical expressions involve the use of roots, such as square roots or cube roots, and are a fundamental part of working with more complex mathematical concepts. These expressions allow us to represent numbers that cannot be simplified to whole numbers, offering a way to handle irrational numbers and solve various types of problems. Understanding how to simplify and manipulate these expressions is essential for tackling more advanced topics in mathematics.

Key Components of Radical Expressions

A radical expression typically consists of a radical symbol, which represents the root, and a radicand, the number or expression under the root. The most common roots are the square root and cube root, but higher roots can also be used. Key components include:

• Radicand: The number or expression inside the radical symbol.
• Index: The type of root being taken (e.g., square root, cube root). If no index is shown, it is understood to be a square root.
• Radical Symbol: The symbol used to denote the root (√ for square root, ∛ for cube root, etc.).

Simplifying Radical Expressions

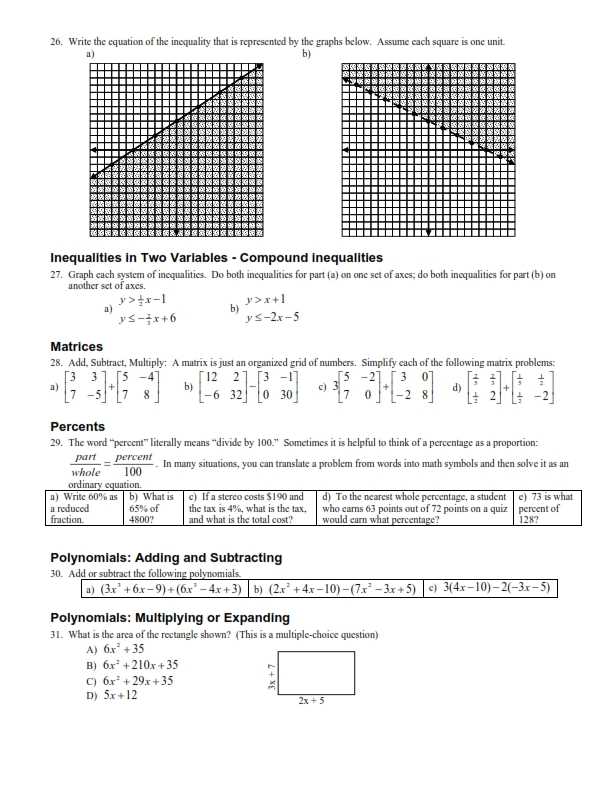
Simplifying radical expressions involves reducing them to their simplest form. This can be done by:

• Factoring the radicand: Break down the number or expression under the root into its prime factors.
• Pairing factors: For square roots, look for pairs of identical factors that can be taken outside the radical.
• Combining like terms: If multiple radical expressions have the same index and radicand, you can combine them by adding or subtracting the coefficients.

By understanding these fundamental rules, you can simplify radical expressions, making it easier to solve problems involving them.

Working with Absolute Value Equations

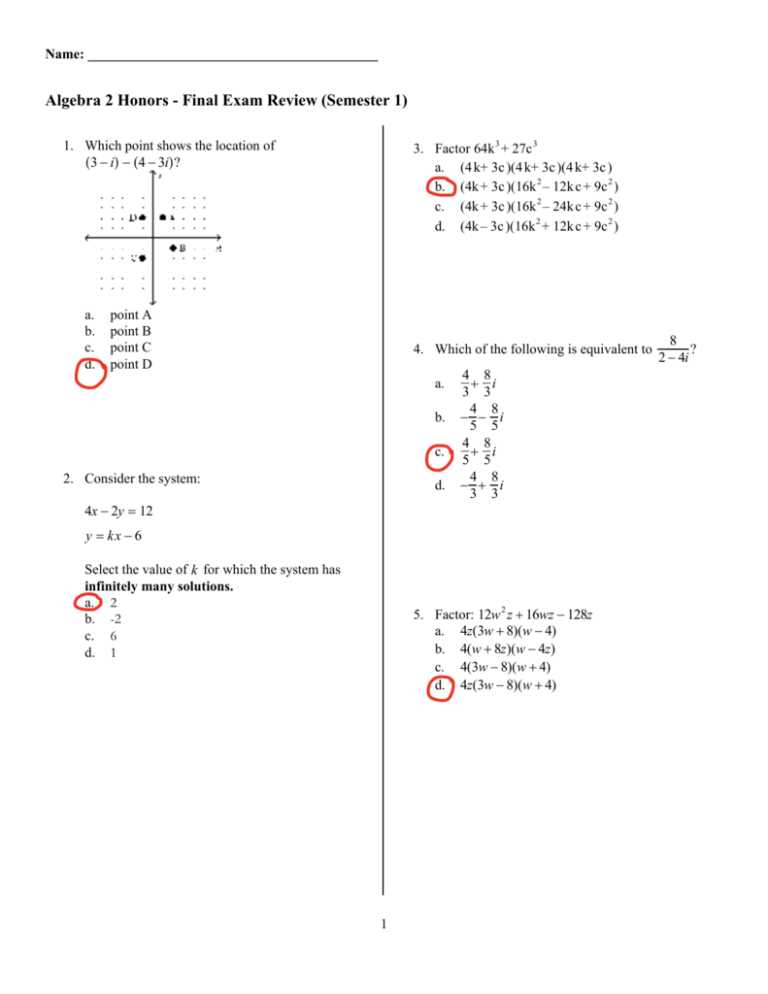
Absolute value equations involve expressions where the variable is inside absolute value symbols. These equations represent the distance of a number from zero on the number line, regardless of direction. Solving these types of equations requires understanding that the absolute value of a number can yield two possible solutions: one positive and one negative. This dual solution approach is key to finding all possible answers to these equations.

To solve absolute value equations, follow these general steps:

• Isolate the absolute value expression: If the absolute value expression is part of a larger equation, first isolate it on one side of the equation.
• Create two separate equations: Set the expression inside the absolute value equal to both the positive and negative value of the other side of the equation.
• Solve each equation: Solve for the variable in both cases.
• Check for extraneous solutions: After finding the solutions, substitute them back into the original equation to verify they are valid.

By carefully following these steps, you can handle any absolute value equation, ensuring that all possible solutions are found and validated.

Graphing Linear and Quadratic Equations

Graphing equations provides a visual way to understand the relationships between variables. Linear equations represent straight lines, while quadratic equations describe curves known as parabolas. By plotting points and understanding the characteristics of these equations, you can create accurate graphs that showcase the behavior of the equations across different values.

To graph linear equations:

• Identify the slope and y-intercept: The slope represents how steep the line is, and the y-intercept is where the line crosses the vertical axis.
• Plot the y-intercept: Start by placing a point at the y-intercept on the graph.
• Use the slope: From the y-intercept, move according to the slope to plot another point. Repeat this process to draw the line.

For quadratic equations, the process is slightly different:

• Identify the vertex: The vertex is the highest or lowest point on the parabola, depending on whether the curve opens upward or downward.
• Plot additional points: Choose values for x, calculate the corresponding y values, and plot these points around the vertex to form the curve.
• Determine the direction of the parabola: If the leading coefficient is positive, the parabola opens upward; if negative, it opens downward.

By following these methods, you can graph both linear and quadratic equations effectively, gaining a deeper understanding of how these functions behave.

Solving Word Problems Effectively

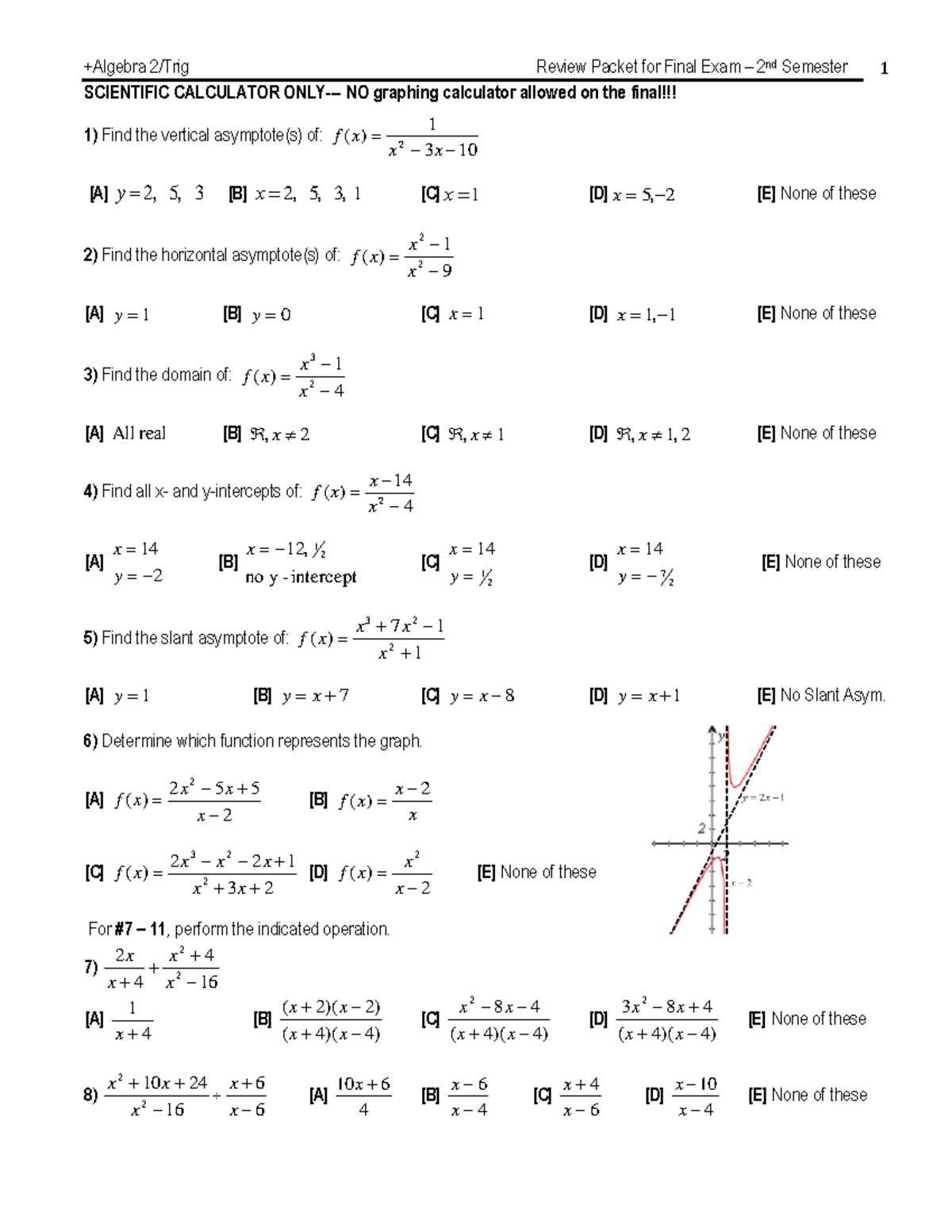
Word problems require translating real-world situations into mathematical expressions. The key to solving these problems lies in understanding the context, identifying the variables, and systematically forming equations based on the information provided. Once the equation is set up, the next step is to solve it and interpret the solution in terms of the original scenario.

Steps to Solve Word Problems

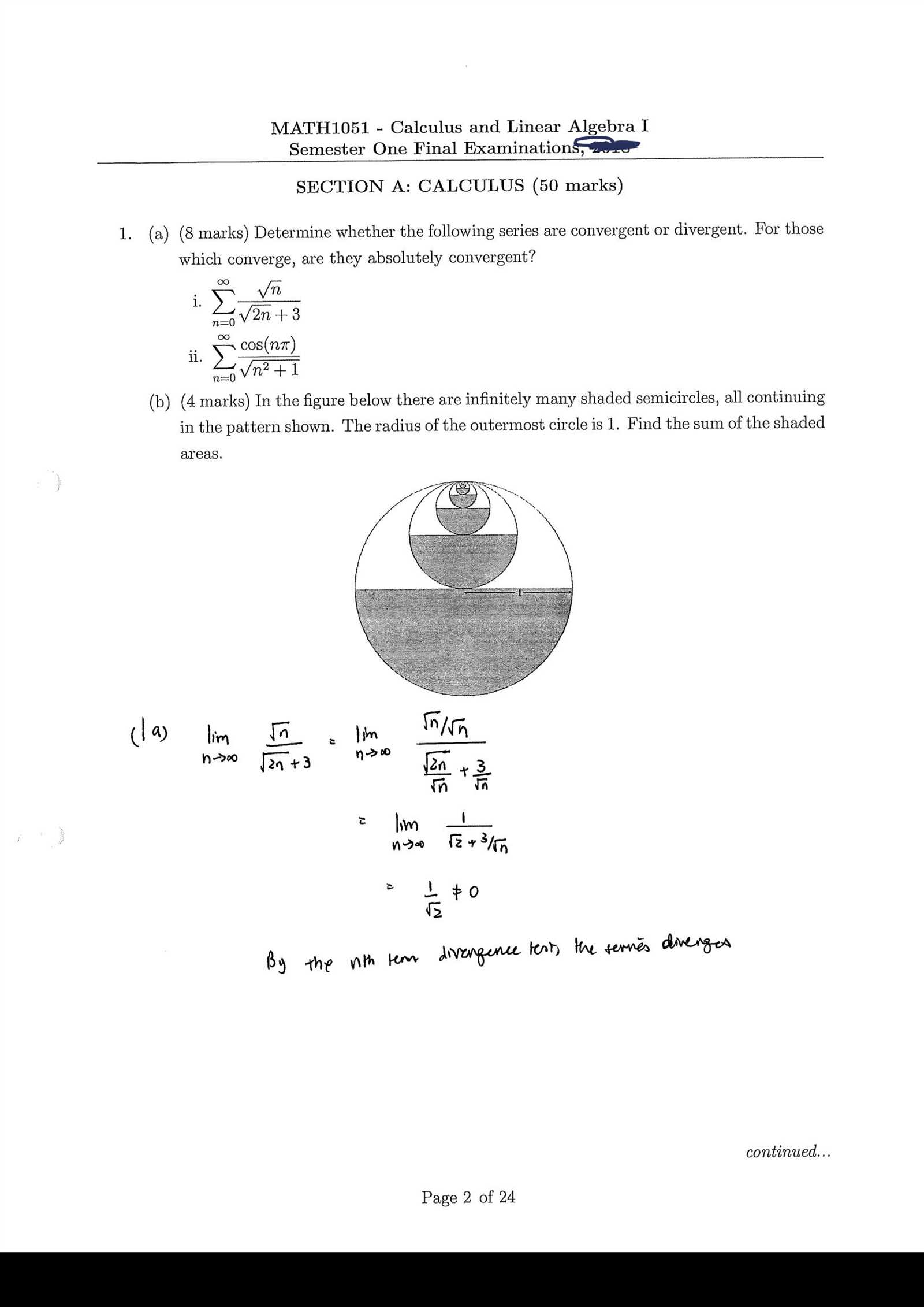
To tackle word problems effectively, follow these steps:

• Read the problem carefully: Pay close attention to the details and identify what is being asked. Highlight key information such as quantities, rates, or relationships between variables.
• Define the variables: Assign a letter or symbol to represent the unknown quantity or quantities. This will make it easier to form an equation.
• Set up an equation: Translate the information into a mathematical equation that models the situation. This could involve basic operations such as addition, subtraction, multiplication, or division.
• Solve the equation: Once the equation is set up, use appropriate techniques to solve for the unknown variable(s).
• Check the solution: After finding the solution, go back to the context of the problem to ensure that it makes sense and answers the original question.

Common Techniques for Word Problems

Some common strategies for solving word problems include:

• Work backward: In some cases, you can solve a problem by starting from the desired outcome and working your way backward to find the unknowns.
• Break the problem into parts: For more complex problems, break the problem into smaller, manageable parts, and solve each part step-by-step.
• Use diagrams or charts: Visual aids can help clarify relationships between quantities and make the problem easier to solve.

By carefully following these strategies, you can approach word problems with confidence and solve them accurately, translating real-world situations into solvable mathematical equations.

Tips for Time Management During Exams

Effective time management during assessments is essential for success. When faced with a time constraint, it’s important to stay organized and focused to ensure you can complete all sections of the test. Planning ahead and pacing yourself will help you avoid unnecessary stress and maximize your performance. By using the right strategies, you can efficiently manage your time and tackle even the most challenging questions with confidence.

Strategies for Managing Your Time

To optimize your time during an assessment, consider these helpful techniques:

• Prioritize Easy Questions: Start by answering the questions you feel most confident about. This will help you gain momentum and build confidence for the more challenging problems.
• Set Time Limits: Allocate a specific amount of time to each section or question. Keep track of time to avoid spending too long on any single part of the test.
• Leave No Question Blank: If you’re unsure about an answer, try to make an educated guess. Skipping questions means missing out on potential points.
• Review Your Work: If time permits, review your answers at the end. This can help you catch mistakes or refine your answers before submitting.

Time Management Tips at a Glance

Tip	Explanation
Start with simple questions	Answer the questions you know first to secure easy points.
Set time limits	Give yourself a specific time frame to work on each section to stay on track.
Use a process of elimination	For multiple-choice questions, eliminate clearly wrong answers to improve your odds.
Stay calm and focused	Don’t let stress slow you down. Stay calm and move steadily through the questions.

By following these strategies and maintaining good time management, you can complete the assessment effectively, reduce stress, and increase your chances of achieving a great result.

Reviewing Important Formulae and Theorems

Having a strong grasp of key formulae and theorems is crucial for success in any mathematical assessment. These foundational principles provide the tools necessary to solve complex problems efficiently. Whether it’s for calculating areas, solving equations, or proving relationships, understanding and memorizing these essential concepts can greatly improve your performance. Reviewing and practicing these formulas will help ensure you’re prepared for any question that comes your way.

Key Formulae and Theorems

Below are some of the most important formulae and theorems that are commonly used in mathematical assessments:

Formula/Theorem	Application
Quadratic Formula	Used to solve quadratic equations in the form ax² + bx + c = 0.
Pythagorean Theorem	Relates the sides of a right triangle: a² + b² = c².
Area of a Circle	Calculates the area using the radius: A = πr².
Slope Formula	Determines the slope of a line between two points: m = (y₂ – y₁) / (x₂ – x₁).
Distance Formula	Finds the distance between two points: d = √((x₂ – x₁)² + (y₂ – y₁)²).

By reviewing and applying these formulas and theorems, you can quickly tackle a wide variety of mathematical problems. Remember to not only memorize them but also practice using them in different contexts to reinforce your understanding and problem-solving skills.

Common Mistakes to Avoid on the Test

During an assessment, it’s easy to overlook small details or make simple errors that can lead to incorrect results. Being aware of common pitfalls can help you avoid costly mistakes and improve your overall performance. Whether it’s misinterpreting a question or making calculation errors, understanding where students often struggle allows you to take proactive steps in preventing these issues.

Key Mistakes to Watch Out For

• Misunderstanding the Question: Often, students rush through the problems without fully reading the instructions or details, leading to misinterpretations. Take your time to understand what is being asked before attempting a solution.
• Skipping Steps in Calculations: Skipping intermediate steps or performing calculations too quickly can result in simple arithmetic mistakes. Show all your work and double-check your calculations to ensure accuracy.
• Incorrectly Applying Formulas: Using the wrong formula or misapplying the correct one can lead to incorrect answers. Familiarize yourself with the key formulas and understand when and how to use them.
• Sign Mistakes: One of the most common mistakes is overlooking positive and negative signs during operations, especially when adding or subtracting numbers. Always check your signs before finalizing your answer.
• Rushing Through Word Problems: Word problems require careful analysis and planning. Rushing to solve them without breaking down the information can lead to overlooking important details. Take the time to outline the problem and organize your solution.

Strategies to Avoid Mistakes

• Review Your Work: If time permits, always review your answers at the end of the test to catch any errors you might have missed.
• Practice with Mock Tests: Familiarize yourself with the types of questions and problem formats you may encounter by practicing with sample problems or past assessments.
• Stay Calm and Focused: Anxiety can lead to careless errors. Maintain a steady pace and stay focused throughout the test.

By staying mindful of these common mistakes and implementing strategies to avoid them, you can significantly improve your accuracy and confidence during the assessment.