August 2025 Algebra Regents Answers and Solutions

When approaching a major mathematics examination, it’s crucial to focus on key concepts, strategies, and practice techniques. Familiarity with the types of problems and common question formats can significantly improve performance. This guide aims to assist students in mastering essential mathematical principles and performing confidently during their test.
Mastering problem-solving skills is central to success. Breaking down complex equations, understanding step-by-step solutions, and applying effective techniques are crucial for achieving high marks. Through practice, students can improve both speed and accuracy, ensuring they tackle even the most challenging questions with ease.
In addition to practicing sample questions, reviewing past tests offers valuable insight. By analyzing previous exam solutions, students can identify recurring themes and problem-solving patterns. This approach will provide a clearer understanding of what to expect and help prioritize areas for focused study.
Exam Solutions and Key Insights

Understanding the solution process for a mathematics exam is essential for anyone preparing for a standardized test. The ability to break down problems into manageable parts and apply logical steps leads to a deeper comprehension of mathematical concepts. This section provides a detailed overview of how to approach complex questions and check your work efficiently.
Problem Breakdown and Effective Approaches
Each question presents unique challenges, requiring different strategies to solve. The key to success lies in recognizing patterns within the problem and selecting the most effective method. Whether working through equations, graphing functions, or applying formulas, taking a systematic approach ensures accuracy and clarity in your solutions.
Where to Find Comprehensive Solutions
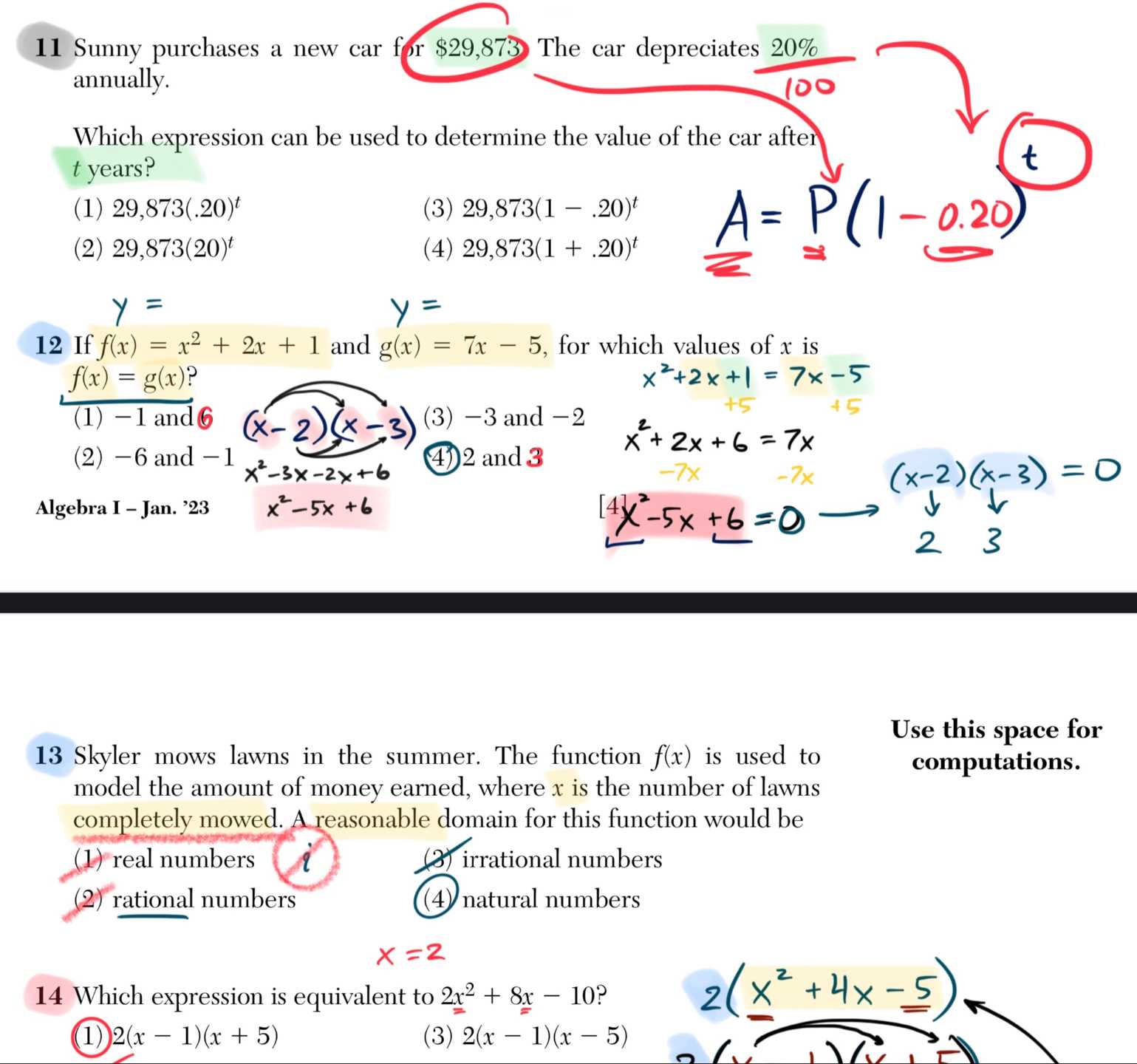
To further enhance your preparation, it’s important to explore resources that provide full solutions and explanations. By studying how each step leads to the final result, you can gain a deeper understanding of the underlying principles. These resources often include detailed walkthroughs, which help reinforce key strategies and mathematical reasoning.
Overview of August 2025 Exam
This section provides a comprehensive look at the upcoming mathematical exam, detailing what students can expect and how to best prepare. The test is designed to assess a broad range of skills, from fundamental problem-solving techniques to advanced concepts. A strong understanding of the material and effective test-taking strategies are key to achieving a high score.
The exam will feature a variety of question types, each targeting different areas of mathematical understanding. Students should focus on mastering core concepts and practicing problem-solving methods. Below is an outline of the key areas covered:
- Equations and Inequalities: Solving linear, quadratic, and other types of equations.
- Functions: Understanding and applying different types of functions, including linear, quadratic, and exponential.
- Statistics: Interpreting data, calculating probabilities, and analyzing trends.
- Geometry: Understanding key geometric principles, including transformations and area calculations.
- Data Analysis: Analyzing graphs and tables, drawing conclusions from given data.
Effective preparation involves not only reviewing these areas but also practicing past exams and sample problems. Below are some strategies for maximizing your performance:
- Focus on areas of weakness by reviewing practice problems and seeking additional help if necessary.
- Time yourself when practicing to simulate exam conditions and improve time management.
- Familiarize yourself with the format and structure of the test to reduce anxiety on exam day.
- Review detailed solutions to practice problems to understand the thought process behind each step.
By covering all these topics and strategies, students will be well-prepared to approach the test with confidence and efficiency.
Key Topics Covered in the Mathematics Exam

The exam assesses a wide range of mathematical skills, from foundational concepts to more advanced techniques. A solid grasp of the following topics is essential for success. Understanding each area not only helps in answering questions accurately but also builds the critical thinking necessary for solving complex problems.
Core Mathematical Concepts
Equations and Systems: Solving linear equations, systems of equations, and inequalities is crucial. Expect questions that require solving for unknowns in different types of problems. Mastery of these concepts forms the backbone of many other topics covered in the exam.
Functions and Graphing: A deep understanding of various types of functions, such as linear, quadratic, and exponential, is essential. The ability to graph these functions and interpret their meaning is frequently tested. Focus on recognizing key features like slope, intercepts, and vertex points.
Advanced Problem-Solving Skills

Polynomials and Factoring: Working with polynomials, including factoring expressions and solving polynomial equations, is a core skill. Students should be familiar with different factoring techniques, such as difference of squares and factoring trinomials.
Statistics and Data Analysis: Analyzing data sets, understanding measures of central tendency (mean, median, mode), and interpreting statistical graphs are vital components. Make sure to practice interpreting word problems that involve data analysis and probability.
Mastering these key topics ensures a well-rounded understanding of the material and provides the tools necessary to tackle any problem presented on the test.
Understanding the Format of the Mathematics Exam
Knowing the structure and organization of the exam is crucial for effective preparation. The test is designed to assess a wide variety of skills, with different question types aimed at evaluating both fundamental concepts and problem-solving abilities. Being familiar with the exam format can reduce anxiety and improve time management on test day.
Types of Questions
The exam includes multiple question formats, each targeting different areas of mathematical knowledge. You will encounter:
- Multiple Choice Questions: These questions test your ability to identify the correct answer from a list of options. It is important to read each choice carefully before selecting your answer.
- Short-Answer Questions: These questions require you to show your work and provide a written response. Clarity and accuracy are key when presenting solutions.
- Extended Response Questions: These problems ask for detailed solutions, including multiple steps. Demonstrating your understanding of the entire process is critical for scoring well on these questions.
Time Management and Strategies

Efficient time management is essential during the exam. Allocate time to each section based on the number of questions and their complexity. It is advisable to start with easier questions to build confidence and leave the more challenging problems for later. Remember, reviewing your answers can make a significant difference in your final score, so try to leave time at the end for a quick check.
Understanding the exam structure will help you approach each section strategically, ensuring that you perform to the best of your abilities.
How to Prepare for the Mathematics Exam
Effective preparation is key to performing well in any examination. With the right approach, you can master the required skills, build confidence, and reduce test anxiety. The goal is to develop a solid understanding of the concepts, practice problem-solving techniques, and familiarize yourself with the exam format.
Study the Core Concepts

Start by reviewing the key topics that will appear on the exam. Understanding fundamental concepts such as solving equations, graphing functions, and analyzing data is essential. Break down each topic into manageable sections and ensure you grasp both the theory and practical applications. Focus on areas where you feel less confident, as these are often the most challenging during the test.
Practice with Sample Questions
Once you’ve reviewed the material, practicing sample questions is crucial. Working through practice tests simulates the actual exam environment and helps you become familiar with the types of questions you will face. Time yourself when practicing to improve your ability to manage time during the exam. Additionally, after each practice session, review your answers to understand any mistakes and correct them.
Consistent preparation, including reviewing material, practicing problems, and timing yourself, will help you feel ready for the exam and increase your chances of success.
Common Mistakes in the Mathematics Exam
Even the most prepared students can make mistakes during an exam, often due to misinterpretation of questions or simple calculation errors. Recognizing the most common pitfalls can help you avoid them and improve your performance. Being aware of these mistakes allows you to approach the exam more confidently and with greater precision.
Misreading Questions
One of the most frequent mistakes is misreading the questions, leading to incorrect answers. It is crucial to carefully read each problem and ensure that you understand what is being asked. For example, some questions may require you to find the solution to an equation, while others may ask for the equation itself. Always highlight key information and pay attention to keywords like “solve,” “simplify,” or “explain.”
Calculation Errors and Careless Mistakes
Mathematical exams often involve multiple steps, and it’s easy to make small calculation errors. Whether it’s adding, subtracting, or multiplying, even minor mistakes can lead to incorrect results. Double-check your work and make sure you haven’t missed a step. Verifying calculations, especially when working with fractions, decimals, or complex equations, is a simple but effective strategy to minimize these errors.
Avoiding these common mistakes requires practice and careful attention during both preparation and the actual exam. By learning to recognize these pitfalls, you can greatly enhance your performance and avoid costly errors.
Step-by-Step Guide to Answering the Mathematics Exam
Approaching an exam with a clear, methodical strategy can make a significant difference in your performance. Breaking down each question into manageable steps allows you to stay focused, minimize mistakes, and ensure that you are providing complete and correct solutions. This guide outlines a step-by-step approach to tackling each problem efficiently and accurately.
Step 1: Read the Question Carefully
The first step in answering any question is to understand exactly what is being asked. Read through the problem at least twice to make sure you grasp all the details. Pay attention to important keywords and phrases like “find,” “simplify,” or “graph.” Understanding the requirements will help you determine the right approach.
Step 2: Plan Your Approach
Once you’ve fully understood the problem, plan your solution. Break it down into logical steps and decide on the best method to use. For example, if the question involves solving an equation, determine if you need to isolate the variable or if you should use substitution or elimination. If graphing is involved, identify the coordinates, slopes, and other relevant details.
| Action | Details |
|---|---|
| Step 1 | Read and understand the question. |
| Step 2 | Plan your approach based on the problem type. |
| Step 3 | Execute the solution, showing each step. |
| Step 4 | Review your answer to ensure accuracy. |
Step 3: Show Your Work
It’s crucial to document each step of your process. Even if the answer is simple, showing your work demonstrates that you understand how to reach the solution. This will also help you identify any errors if your final answer doesn’t match expectations. Write down intermediate steps, calculations, or logical reasoning as you go.
Step 4: Review and Double-Check
After completing the problem, take a few moments to review your work. Check your calculations, re-read the question to ensure you haven’t missed anything, and verify that your answer makes sense. Even small errors can lead to incorrect answers, so thorough checking is essential.
By following this methodical approach, you’ll improve your chances of answering questions correctly and efficiently, making your exam experience more manageable and less stressful.
Tips for Improving Mathematical Skills
Improving your mathematical abilities takes time, practice, and a strategic approach. Whether you are struggling with basic concepts or advanced problem-solving, consistent effort can lead to significant progress. The following tips will help you sharpen your skills and build confidence in your mathematical abilities.
Focus on Core Concepts
Mastering the foundational principles is crucial for success in mathematics. A strong understanding of basic operations, equations, and functions is necessary for solving more complex problems. Here are some strategies to strengthen your core knowledge:
- Review Fundamental Rules: Refresh your memory on key formulas, properties, and theorems.
- Understand Rather Than Memorize: Focus on understanding why certain rules work instead of just memorizing them.
- Practice Regularly: Consistent practice helps reinforce what you’ve learned and improves retention.
Enhance Problem-Solving Techniques
Developing effective problem-solving strategies is essential to tackling more challenging problems. Here are some methods to help you become a more efficient problem solver:
- Break Down Complex Problems: Divide larger problems into smaller, manageable parts to simplify your approach.
- Work Backwards: If you’re stuck, try working backwards from the solution to understand the steps needed.
- Analyze Mistakes: When you make an error, take time to understand what went wrong and how to avoid it in the future.
By implementing these strategies and staying consistent with your practice, you’ll gradually improve your mathematical skills and feel more confident in your ability to solve problems effectively.
What to Expect on the Upcoming Exam
The upcoming exam is designed to test your understanding of various mathematical concepts and your ability to apply them to real-world problems. The test will challenge your problem-solving skills and your grasp of essential theories and principles. Preparing effectively involves knowing what to expect so that you can approach each section with confidence.
Key Areas of Focus
The exam will cover a broad range of topics, and it’s important to be prepared for each area. Here are some of the main subjects that you should expect to encounter:
- Equations and Inequalities: Be prepared to solve both linear and quadratic equations, as well as work with inequalities.
- Functions and Graphs: Expect questions on graphing and interpreting different types of functions, including linear and exponential functions.
- Data Analysis: There may be questions that involve interpreting graphs, calculating measures of central tendency, and working with probability.
- Polynomials and Factoring: You’ll likely encounter problems related to factoring polynomials and simplifying expressions.
Test Format
The exam will typically consist of multiple-choice questions, short-answer questions, and extended response items. Each section is designed to test different skills and levels of understanding. Here’s an overview of the test format:
- Multiple-Choice Questions: These questions assess your ability to recognize correct answers and eliminate incorrect options.
- Short-Answer Questions: You’ll be asked to solve problems and show your work for partial credit.
- Extended Response Questions: These will require a more detailed explanation and step-by-step solutions.
Understanding the structure and content of the exam will help you focus your preparation efforts and manage your time effectively during the test.
How to Check Your Math Exam Responses

After completing a math exam, reviewing your responses carefully is crucial to ensure accuracy and identify any mistakes. A systematic approach can help you catch errors you may have missed initially and improve your final score. Below are several strategies for checking your work effectively before submitting your test.
Step 1: Review Your Work for Common Mistakes
The first step is to look over your calculations for any simple mistakes, such as arithmetic errors or incorrect sign handling. Check that you’ve followed each step of the problem-solving process correctly. Some common mistakes to look for include:
- Forgotten negative signs when working with subtraction or negative numbers.
- Misplaced decimal points in calculations.
- Incorrect application of mathematical rules or formulas.
Step 2: Recheck Key Steps and Final Results
Once you’ve scanned for obvious errors, go back through the key steps to ensure you didn’t overlook any important details. If your answer seems unusual, consider whether your approach to the problem was correct. Here are some techniques to verify your work:
- Plug in the Solution: If possible, plug your solution back into the original equation to check if it holds true.
- Reverse the Process: For word problems or calculations involving multiple steps, reverse the operations to see if you reach the same result.
- Estimate Before Finalizing: Before finalizing your answers, quickly estimate the result to check if the magnitude of your solution makes sense.
By carefully checking your responses using these methods, you can increase the chances of submitting a flawless exam and maximize your score.
Breaking Down Complex Math Problems
Complex problems can often feel overwhelming, but breaking them down into smaller, more manageable steps can make them easier to solve. By identifying key components, recognizing patterns, and applying systematic approaches, you can simplify even the most challenging questions. The following methods will help you tackle difficult problems with greater confidence and efficiency.
Step-by-Step Approach
The first step in solving a complex problem is to decompose it into smaller, simpler parts. Focus on one section at a time to ensure that no details are overlooked. Here are some tips to help guide your problem-solving process:
- Identify the Goal: Determine what the question is asking and what kind of solution is needed (e.g., a specific value, a function, etc.).
- Break Down the Equation: Simplify the equation or expression step by step. Start with operations inside parentheses or simplifying fractions.
- Apply Relevant Formulas: Identify and apply any formulas or rules relevant to the problem.
- Check for Simplifications: Look for opportunities to simplify the equation further as you go along.
Using a Table for Organization
For more complex questions involving multiple variables or operations, organizing your work in a table format can help keep everything clear. This approach allows you to track each step in a logical manner and minimizes the chance of errors.
| Step | Action | Result |
|---|---|---|
| 1 | Simplify the equation | Reduced equation |
| 2 | Apply formulas | Intermediate value |
| 3 | Check for common factors | Simplified result |
| 4 | Final calculation | Final solution |
By following these steps and using organizational tools like tables, you can break down even the most challenging problems into manageable sections and ensure that each part is handled accurately. With practice, this approach will help you approach complex problems with greater confidence.
Resources for Math Exam Practice
Preparation is key to mastering any exam, and having access to the right resources can make all the difference. A variety of study tools and materials can help reinforce your understanding of mathematical concepts, improve problem-solving skills, and provide practice under timed conditions. Below are several helpful resources that can guide you through your preparation and enhance your performance.
Online Practice Platforms
There are numerous online platforms offering free practice questions and full-length mock exams that mimic the structure of the test. These websites often include step-by-step solutions to help you learn from your mistakes. Some of the most useful sites include:
- Khan Academy: Provides video tutorials and exercises across a wide range of topics, perfect for reviewing fundamental concepts.
- Edulastic: Offers interactive practice tests that can help simulate the exam environment and provide detailed feedback.
- IXL: Features personalized practice questions tailored to your learning level and progress.
Study Guides and Books
In addition to online platforms, study guides and practice books are invaluable resources for reinforcing concepts. Many books include comprehensive review sections, practice tests, and explanations to ensure you are well-prepared. Some recommended titles are:
- The Princeton Review: Known for their targeted practice tests and detailed explanations, this resource is a great choice for exam prep.
- Kaplan Test Prep: Offers comprehensive study books with practice questions and solutions to help build your skills.
- Barron’s: A trusted name in exam preparation, Barron’s guides provide in-depth coverage of various topics with practice exams.
By utilizing these resources, you can build a solid foundation, familiarize yourself with the exam format, and approach your study sessions with confidence.
Common Math Concepts in Exams
Exams focused on mathematical reasoning often test students’ ability to understand and apply various fundamental principles. These concepts are essential for solving problems efficiently and accurately. Mastery of key areas allows students to approach the exam with confidence. Below are some of the most commonly tested mathematical topics, which provide a solid foundation for success.
One of the central areas in these exams is linear equations, where students are required to manipulate expressions and solve for unknown variables. Another significant concept is quadratic equations, which involves solving second-degree equations through various methods such as factoring, completing the square, or using the quadratic formula. Understanding functions is also crucial, especially when dealing with graphing and interpreting different types of relationships.
Additionally, inequalities and systems of equations are frequently assessed. These topics require students to work with multiple equations simultaneously or to analyze the solutions that satisfy given conditions. Mastering polynomials and their operations is another key skill, as well as solving problems related to exponents and roots.
Finally, concepts of probability and statistics are often integrated into the exam, testing students’ ability to interpret data and calculate probabilities in various scenarios. Mastering these core areas is crucial for success in the exam and lays the groundwork for further study in advanced mathematics.
Time Management Strategies for Math Exams
Effectively managing your time during a math exam is crucial for maximizing performance. With the limited time available, it’s important to allocate enough time to each section of the exam while avoiding spending too much time on any one problem. Implementing a strategy for time management ensures you can complete all sections and still have time for review. Below are key strategies to optimize your time during the test.
Prioritize Easy Questions First
Start by quickly scanning the exam to identify questions that are easy or familiar. These problems should be solved first, as they will boost your confidence and provide quick points. Make sure to answer all these simpler questions before moving on to more complex problems. This will give you a solid base and allow you to manage your time more effectively for harder questions later on.
Allocate Time to Each Section
Before starting, set a time limit for each section of the exam based on the total time and the number of questions. For example, if you have two hours for 50 questions, aim to spend no more than 2-3 minutes on each. If a problem is taking too long, mark it and move on–come back to it later if time allows. This ensures that you don’t run out of time and can still attempt every question.
Additionally, be mindful of your time during the review stage. Leave at least 10-15 minutes at the end of the exam to review your answers. Check for any mistakes, especially in calculations and question interpretation, to ensure the highest possible accuracy.
Time management is key to navigating a challenging exam efficiently. By using these strategies, you’ll be able to complete the test with a clear mind and confident answers.
Where to Find August 2025 Answers
Finding correct solutions for exam questions is essential for students preparing for tests. Whether you’re reviewing for practice or need to double-check your responses, it’s important to use reliable and trusted sources. Below are some ways you can access correct solutions and understand the reasoning behind them.
Official Educational Websites
One of the most reliable sources for verified solutions is official educational websites, such as government or school district platforms. These sites often provide:
- Past exam papers and solutions
- Official answer keys
- Step-by-step solution guides
These resources can give you a solid understanding of how answers are derived and the specific methodologies that should be used in solving questions.
Study Guides and Review Books
Many students rely on study guides and review books published by well-known educational companies. These books typically include:
- Practice questions from past exams
- Detailed solutions and explanations
- Tips and strategies for tackling common problems
By using these guides, you can not only find answers but also strengthen your understanding of key concepts.
Online Educational Communities
Another great way to find solutions is through online forums and educational communities. Websites like Khan Academy, Reddit, or StackExchange feature:
- Discussion threads with explanations for specific questions
- Video tutorials and problem-solving sessions
- Interactive quizzes to test your knowledge
These platforms provide additional learning opportunities where you can engage with others and clarify any doubts you might have.
By leveraging these resources, you’ll be able to access accurate answers and enhance your exam preparation process.
Using Past Papers for Exam Success
Utilizing previous years’ exam papers is one of the most effective methods for preparing for an upcoming test. These papers provide insight into the types of questions that may appear and help familiarize students with the format and timing of the actual exam. By practicing with real past questions, students can build confidence and improve problem-solving skills.
Working through past papers allows you to:
- Understand Question Patterns: Identifying recurring question themes helps in predicting the focus areas for the upcoming test.
- Improve Time Management: Practicing under timed conditions helps you manage your time more effectively during the actual exam.
- Identify Weak Areas: Past papers highlight areas where further revision is needed, allowing you to focus on specific topics that require attention.
How to Use Past Papers Effectively
To make the most of past papers, follow these steps:
- Start with Untimed Practice: Initially, complete past papers without a time limit to focus on understanding the concepts and methods.
- Time Yourself: As you progress, start timing yourself to simulate exam conditions. This will help you get used to working within the time constraints.
- Review Your Mistakes: After completing a paper, carefully review any mistakes. Understanding why an answer is wrong is crucial to avoiding the same errors in the future.
- Seek Help if Necessary: If you’re struggling with certain questions, seek additional resources or ask for help from a teacher or tutor.
The Benefits of Past Papers in Exam Preparation

Using past papers not only helps with exam readiness but also enhances critical thinking and problem-solving abilities. By working through a variety of questions, you can develop strategies to tackle different problem types efficiently. This practice helps boost both accuracy and speed, which are essential for achieving success in any exam.
Overall, incorporating past papers into your study routine is an invaluable tool for improving exam performance and achieving better results.