Secondary Math 1 Module 9 Answer Key

In this section, we will explore the detailed solutions to various exercises and topics covered in a specific section of your curriculum. This resource is designed to support your learning process, helping you navigate through complex problems and concepts that can often be challenging to master. By understanding the approach to solving each problem, you will gain clarity and improve your problem-solving techniques.
Each solution is broken down step-by-step, guiding you through the reasoning and methods used to reach the final result. The aim is not only to show you the correct answers but also to explain how these answers are derived, enabling you to apply similar strategies in future tasks. With this resource, you’ll strengthen your ability to tackle similar exercises on your own with greater confidence.
Practice and repetition are key to mastering any subject, and with the explanations provided here, you can reinforce your knowledge. Learning through example is one of the most effective methods for mastering new concepts, and this guide serves as a tool to help you do just that.
Solutions for Module 9 Exercises
This section provides the detailed solutions for the problems presented in the ninth unit of your coursework. It aims to clarify the thought processes and methods required to successfully solve the tasks. By following these solutions, you will gain a deeper understanding of the material, allowing you to approach similar problems with improved accuracy and confidence.
The focus is not only on presenting the correct results but also on explaining how these solutions are derived. Each problem is broken down into manageable steps, making complex concepts easier to understand and apply. This approach helps build a solid foundation for more advanced topics that build on these basic principles.
As you work through each solution, pay attention to the reasoning behind each step. This will help you strengthen your problem-solving abilities and allow you to tackle future challenges with a clearer perspective. With consistent practice, you will improve your ability to apply these techniques independently in various situations.
Understanding the Key Concepts
This section is dedicated to exploring the fundamental principles behind the problems you will encounter in this section of your coursework. Grasping these essential concepts is crucial, as they serve as the foundation for more complex tasks. By breaking down each idea, you will gain the clarity needed to apply these techniques with ease and accuracy in your exercises.
Core Principles for Success
The central ideas covered here revolve around the logical steps required to solve various challenges. These principles can be applied universally, offering a structured approach to tackling problems in different contexts. Understanding these core elements helps build your overall proficiency and provides a framework for learning more advanced topics.
Building Problem-Solving Skills
As you familiarize yourself with these concepts, you will start to develop better strategies for addressing similar challenges. A strong grasp of the basics will enable you to think critically, analyze problems efficiently, and apply the right methods when faced with new questions. Developing these skills early on ensures continued progress throughout your studies.
How to Use the Answer Guide
The guide provided for solving exercises is a valuable resource that helps you verify your results and understand the problem-solving process. It is designed to assist you in checking your work and learning the correct methods for tackling different tasks. By using this tool effectively, you can ensure that you are approaching challenges in the right way and improving your skills over time.
Step-by-Step Verification
When using the solution guide, it’s important to go through each step carefully. Start by attempting the problem on your own before checking the provided solution. This helps you identify areas where you may need further clarification. Compare your approach with the solution, paying attention to the methods used and ensuring you understand why each step is necessary.
Utilizing the Guide for Better Learning
The goal is not just to find the correct answers but to understand the reasoning behind each solution. Using the guide as a learning tool helps reinforce the concepts you have studied and improves your ability to solve similar problems independently. Take note of common patterns or strategies that can be applied to other exercises.
| Problem Number | Your Solution | Guide Solution | Differences |
|---|---|---|---|
| 1 | 20 | 20 | No Difference |
| 2 | 12 | 15 | Check Step 3 |
| 3 | 18 | 18 | No Difference |
Common Challenges in Module 9
In this section, we will address some of the typical difficulties students face when working through the problems in the ninth unit of the course. Understanding these challenges is essential to overcoming obstacles and mastering the concepts presented. By identifying common issues early, you can develop strategies to avoid mistakes and improve your overall performance.
One of the main difficulties is managing complex problem-solving steps, especially when multiple concepts are involved. Many students struggle with integrating different ideas into a single solution, leading to confusion or errors. Additionally, certain topics may require a deeper understanding of abstract concepts, which can be challenging without a solid grasp of the foundational material.
Another common challenge is applying the right methods consistently. In some cases, students may know the correct approach but fail to implement it accurately under time pressure. This can result in incorrect answers, even if the underlying understanding is strong. Developing a systematic approach to problem-solving can help mitigate these issues.
Step-by-Step Guide for Solving Problems
This guide is designed to help you approach and solve problems in a structured and methodical way. By following a clear set of steps, you can break down complex tasks into smaller, more manageable parts. This approach ensures that you address each element of the problem systematically, increasing your chances of finding the correct solution.
The first step is to carefully read the problem and identify what is being asked. Make sure you understand the variables involved and any constraints or conditions provided. Next, organize the information by writing down the known values and marking any important details that may help in the solution process.
Once you have a clear understanding of the problem, the next step is to choose the most appropriate method or formula to apply. This might involve using algebraic techniques, geometric principles, or other strategies depending on the nature of the task. After selecting the method, work through the calculations or reasoning step by step, showing all of your work.
Finally, once you have completed the calculations or reasoning, double-check your result to ensure it makes sense in the context of the problem. If necessary, review each step to catch any potential mistakes. This methodical approach helps you avoid errors and ensures that you arrive at the correct solution with confidence.
Tips for Better Performance in Math
Improving your skills in solving problems requires both practice and effective strategies. In this section, we will explore several tips that can help you boost your performance and understand the material more thoroughly. By following these suggestions, you can enhance your ability to approach tasks with confidence and achieve better results.
First, consistent practice is key. The more problems you solve, the better you will become at identifying patterns and applying the correct methods. Additionally, working through various types of problems exposes you to different strategies, helping you become more adaptable in your approach.
Another important tip is to review your mistakes carefully. Each error is an opportunity to learn and improve. By analyzing where you went wrong, you can identify areas where you need more practice or clarification. This reflective process helps you avoid repeating the same mistakes and strengthens your understanding of the subject.
| Tip | Benefit |
|---|---|
| Practice regularly | Increases familiarity and speed in solving problems |
| Review mistakes | Helps identify weak areas and improve accuracy |
| Ask for help when needed | Clarifies difficult concepts and strengthens understanding |
Finally, don’t hesitate to ask for help when you need it. Whether it’s a teacher, tutor, or classmate, discussing challenging problems with others can provide new insights and explanations that you might not have considered. Collaborating with others helps reinforce your understanding and makes learning more engaging.
Exam Preparation with Module 9
Preparing for an exam involves more than just memorizing formulas or solutions. It requires a deep understanding of the concepts and the ability to apply them in different situations. This section will guide you through effective strategies for reviewing and mastering the content covered in the ninth section of your coursework.
The first step is to focus on understanding the core concepts. Review the material thoroughly, paying attention to the methods used to solve problems. Break down complex ideas into smaller, more digestible parts, and ensure that you understand the reasoning behind each solution. Repetition is crucial–the more you practice, the more comfortable you will become with applying what you’ve learned.
Next, simulate exam conditions by practicing with timed exercises. This helps you get accustomed to solving problems under pressure and improves your time management skills. Use previous exercises and practice problems to test your knowledge and identify areas where you may need more focus before the exam.
Lastly, review any mistakes you make during practice. Understanding why an answer is incorrect is just as important as getting it right. Take the time to revisit challenging problems, analyze your mistakes, and correct any misunderstandings. This will not only help you perform better on the exam but also reinforce the material for long-term retention.
Strategies for Learning Key Formulas
Mastering important formulas is a crucial step in excelling at problem-solving tasks. Formulas are often the foundation of solving complex problems, and learning how to effectively remember and apply them can significantly improve your understanding and performance. In this section, we will discuss several strategies to help you better memorize and apply essential formulas with ease.
Active Recall and Repetition
One of the most effective techniques for memorizing formulas is active recall. This involves repeatedly testing yourself on the formulas you need to learn, without looking at the notes or textbook. Regular repetition helps reinforce the material and makes it easier to recall under exam conditions. Consider the following methods:
- Create flashcards with formulas on one side and their applications or derivations on the other side.
- Write out the formulas from memory and check for accuracy.
- Incorporate them into practice problems to see how they work in different contexts.
Understanding, Not Just Memorizing
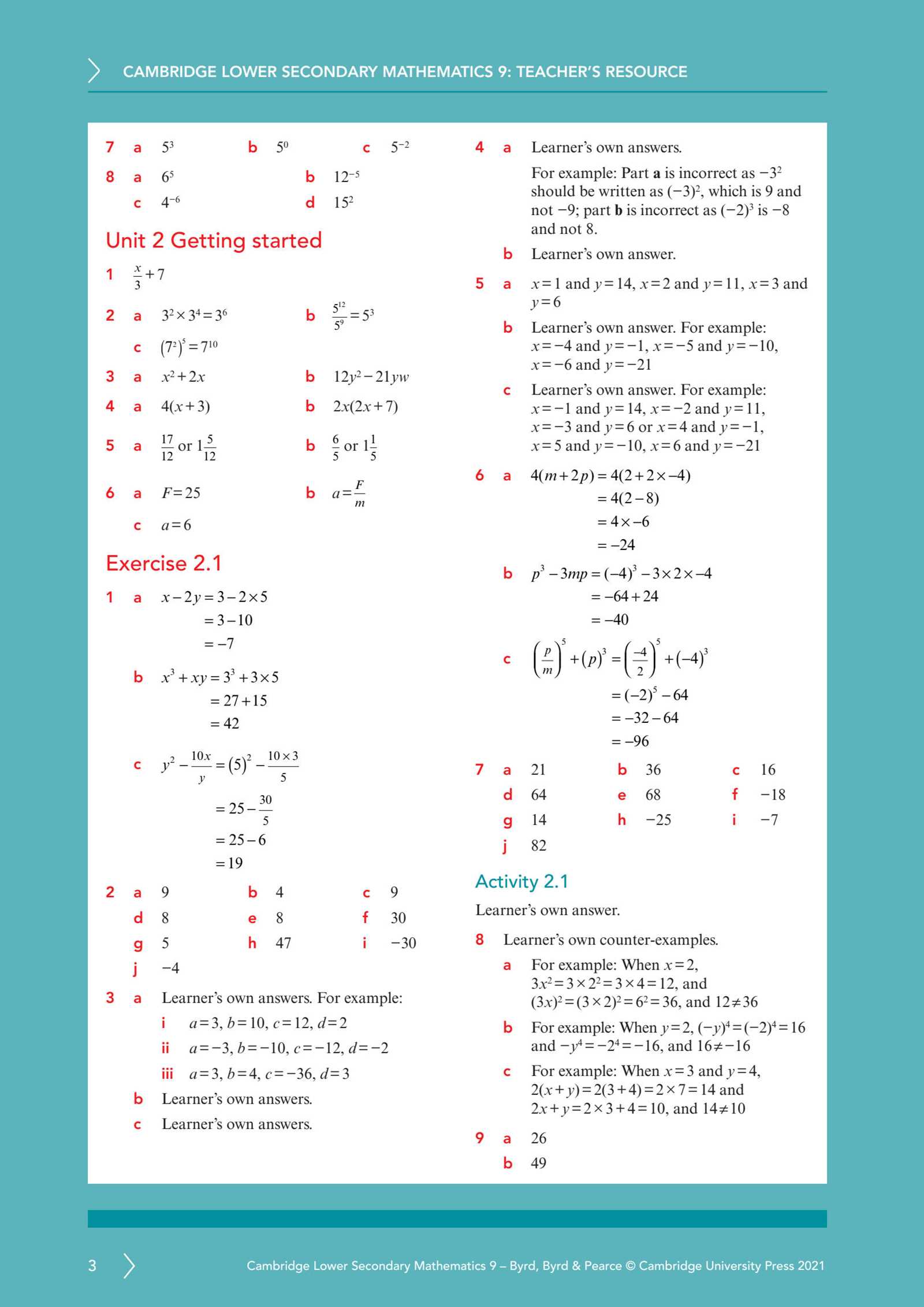
Simply memorizing formulas isn’t always enough–it’s essential to understand their meaning and how they are derived. Understanding the logic behind a formula allows you to apply it correctly and recognize when it is applicable. Consider these strategies:
- Break down the formula into its components and understand each part’s role.
- Link formulas to real-life examples or situations to make them more relatable.
- Practice explaining the formula to others, which will help solidify your understanding.
By actively recalling formulas, understanding their applications, and reinforcing them through consistent practice, you can significantly improve your ability to remember and apply them with confidence.
Reviewing Practice Questions from Module 9
Reviewing practice questions is an essential part of reinforcing your understanding and preparing for assessments. By working through problems, you can test your grasp of the concepts, identify areas for improvement, and build confidence. In this section, we will discuss effective strategies for reviewing and learning from practice questions.
Start by revisiting each question carefully. Don’t just focus on the final answer–take time to understand the steps involved in reaching the solution. Reflect on the methods used and consider alternative approaches. This helps deepen your comprehension and strengthens your problem-solving skills.
Another important step is to analyze your mistakes. If you got a question wrong, don’t just move on–look at where you went wrong. Was it a misunderstanding of the concept, a simple arithmetic error, or a misapplication of a formula? Understanding the cause of the error is crucial to avoiding similar mistakes in the future.
| Question Type | Common Challenges | Tips for Improvement |
|---|---|---|
| Algebraic Problems | Misapplying formulas, incorrect signs | Review steps carefully, practice with variations |
| Geometric Problems | Forgetting key theorems, misinterpreting diagrams | Revisit geometric properties, draw diagrams clearly |
| Word Problems | Difficulty translating text into equations | Break the problem into parts, identify key information |
By reviewing each question thoroughly, understanding your mistakes, and following targeted improvement strategies, you can enhance your skills and achieve better results in the future. This approach turns each practice session into a valuable learning experience, ultimately helping you build a stronger foundation in the subject matter.
Understanding Solutions in Detail
To fully grasp the material, it’s important to go beyond just finding the correct result–understanding the process that leads to the solution is key. This deeper comprehension allows you to approach problems with confidence and apply your knowledge in different contexts. In this section, we will break down how to examine solutions step by step and gain a better understanding of the methods involved.
Breaking Down the Steps
Each solution involves a series of logical steps. Rather than simply memorizing the steps, focus on why each action is taken. For example, consider how a formula is applied, what the intermediate steps signify, and how each part of the solution contributes to the final result. By breaking the problem into smaller components, you will gain a clearer understanding of the approach used.
Recognizing Patterns and Techniques
In many cases, solutions follow a specific pattern or technique that can be applied to similar problems. By identifying these recurring patterns, you can become more efficient in solving future problems. Look for common strategies, such as substitution, factoring, or using properties of geometric shapes. The more you recognize these methods, the quicker and more accurately you will be able to apply them in new scenarios.
Overall, thoroughly understanding each solution not only helps you solve similar problems more effectively, but also strengthens your foundational knowledge, ensuring long-term success and proficiency in the subject.
How to Check Your Work Effectively
Reviewing your work thoroughly is a critical step in ensuring that your solutions are correct and accurate. The process of verification can help you identify any errors, clarify misunderstandings, and reinforce your learning. This section outlines strategies for effectively checking your work, making sure you can approach each problem with confidence and precision.
Step-by-Step Verification
One of the most reliable ways to check your work is to review each step of your solution carefully. By retracing your steps, you can spot mistakes or miscalculations early. Consider the following methods for a thorough check:
- Review your initial setup: Make sure the problem was interpreted correctly and all given information was included.
- Check intermediate steps: Ensure that each calculation or operation was performed correctly.
- Verify the final result: Compare the outcome with your expectations based on the problem’s context.
Alternative Methods of Verification
In addition to retracing your steps, there are other techniques you can use to double-check your work:
- Work backward: Start from the solution and trace the steps in reverse to see if you arrive at the correct starting point.
- Use estimation: Check if the final result makes sense by approximating or estimating the expected outcome.
- Cross-check with similar problems: Compare your approach and solution with similar problems to identify any inconsistencies.
By taking the time to carefully review and verify your work using these strategies, you can catch errors early, strengthen your understanding, and improve the quality of your solutions.
Top Mistakes to Avoid in Module 9
As you progress through complex problems, it’s easy to make common mistakes that can hinder your understanding and performance. Recognizing these pitfalls early on can help you avoid them and strengthen your overall grasp of the subject. In this section, we’ll outline some of the most frequent errors and provide tips on how to avoid them.
Common Errors and How to Prevent Them
There are several key mistakes that students often make when working through problems. These errors can arise from misinterpreting the problem, rushing through steps, or overlooking important details. Here are a few to watch out for:
- Misreading the Problem: Always read the problem carefully. Missing key details can lead to incorrect setups or misapplication of formulas.
- Skipping Steps: Rushing through the solution can cause important intermediate steps to be skipped, leading to errors in the final result.
- Incorrect Use of Formulas: Ensure that you are using the correct formula for the situation and that you apply it properly.
- Neglecting Units: When working with measurements, always check that your units are consistent and that they are carried through correctly.
How to Avoid These Mistakes
To minimize these common errors, consider the following tips:
- Take Your Time: Don’t rush through problems. Carefully analyze each part of the problem and review your work before moving on.
- Double-Check Formulas: Before applying a formula, make sure you understand when and how it should be used.
- Work Through Each Step: Avoid skipping steps, even if they seem simple. Each step contributes to the accuracy of the solution.
- Review Units: Always check that your units are consistent and correctly accounted for throughout the solution.
By being mindful of these common mistakes and following these strategies, you can avoid errors and improve your problem-solving skills. This will ultimately lead to a stronger understanding of the material and better performance on assessments.
Improving Problem-Solving Skills
Enhancing your ability to solve problems effectively is crucial for mastering complex concepts. Building strong problem-solving skills requires practice, persistence, and the ability to approach challenges with the right mindset. In this section, we will explore strategies that can help improve your problem-solving techniques and boost your confidence in tackling various tasks.
Developing a Strategic Approach
A strategic approach to problem-solving involves breaking down the problem into manageable parts and working through each systematically. Here are some tips to strengthen this process:
- Understand the Problem: Before diving into calculations or solutions, take time to fully grasp what is being asked. Clarify any terms or concepts that seem unclear.
- Plan Your Approach: Outline the steps you will take to solve the problem. Having a plan helps to organize your thoughts and ensure that you’re not overlooking any details.
- Work Step-by-Step: Tackle the problem one step at a time. Focusing on one task at a time will prevent confusion and reduce the likelihood of mistakes.
Practicing with Varied Problems
Consistent practice with a variety of problems is key to developing flexible problem-solving abilities. By exposing yourself to different types of challenges, you can learn how to apply different methods and solutions:
- Start Simple: Begin with straightforward problems to build confidence and reinforce basic skills.
- Challenge Yourself: Gradually increase the difficulty of the problems to push your limits and develop new techniques.
- Review Mistakes: Analyze your mistakes to understand where you went wrong. This helps you learn from them and avoid repeating the same errors.
By applying these strategies and consistently practicing, you can improve your problem-solving skills and approach each new challenge with a more structured and confident mindset.
How the Answer Key Can Help
Having access to a solutions guide can significantly enhance your understanding and improve your learning process. It offers valuable insight into the methods and steps needed to solve problems correctly. In this section, we will explore the various ways a solutions reference can support your learning and boost your problem-solving abilities.
Identifying Mistakes and Understanding Solutions
One of the primary benefits of a solutions guide is its ability to help identify and correct mistakes. Reviewing the correct process step-by-step allows you to spot where you may have gone wrong. Here’s how this process can be helpful:
- Spotting Errors: By comparing your work with the correct solution, you can easily pinpoint where your approach diverged from the right method.
- Learning from Mistakes: Understanding why an error occurred helps you avoid repeating it in future exercises.
- Reinforcing Concepts: Seeing the correct steps reaffirms your understanding of the concepts involved and can deepen your knowledge.
Improving Problem-Solving Techniques
A solutions guide is not just a way to verify results but also an excellent resource for learning how to approach different types of problems. Here’s how it can refine your problem-solving skills:
- Understanding Strategies: The solution process often involves specific strategies or shortcuts that can make solving similar problems faster and easier in the future.
- Building Confidence: When you understand how the solution works, you gain the confidence to tackle new problems independently.
- Expanding Your Methods: Reviewing multiple solutions gives you a broader range of approaches to apply in future challenges.
By using a solutions reference thoughtfully, you can enhance your problem-solving skills, learn from your mistakes, and build a stronger foundation in the subject matter.
Frequently Asked Questions About Module 9
This section addresses some of the most common questions regarding the learning material and exercises from this section. Whether you are struggling with specific concepts or seeking tips to improve your understanding, the answers here will guide you through common concerns and clarify key points.
1. What topics are covered in this section?
This section focuses on a variety of fundamental concepts that build on previous knowledge. It includes topics such as problem-solving techniques, specific formulas, and methods used to approach complex exercises. Each section aims to enhance your understanding and prepare you for more advanced concepts.
2. How can I check if I am on the right track?
To verify your progress, it is crucial to compare your work with the correct solutions. If you are uncertain, break down each step of the process and see where your approach differs. Often, small mistakes can lead to larger errors, so it is helpful to ensure every step is logical and follows the right sequence.
3. Can I improve my problem-solving skills with this material?
Yes, this material is designed to help improve your problem-solving skills. By working through examples, understanding common mistakes, and reviewing solution steps, you will gain greater confidence in applying strategies to new problems.
4. Are there additional resources to help me study?
In addition to this guide, there are various textbooks, online resources, and practice tests that can help reinforce your understanding. These tools provide extra practice and offer different explanations and methods for solving problems.
5. What should I do if I get stuck on a question?
If you encounter difficulty with a problem, start by reviewing the steps carefully and break the problem into smaller, manageable parts. If necessary, seek help from peers, instructors, or online communities. Sometimes, discussing the problem can provide a new perspective and help you move forward.
Final Tips for Mastering Module 9
As you approach the end of your studies for this section, it’s important to consolidate your understanding and refine your approach. Mastering the material requires practice, attention to detail, and a clear strategy. Below are a few final tips to help ensure that you are fully prepared to succeed and tackle any challenges that may arise.
Review Key Concepts Regularly
Repetition is crucial when it comes to solidifying your grasp on new material. Regularly reviewing essential principles will help keep them fresh in your mind and improve recall during problem-solving.
- Take time each day to revisit important topics.
- Create flashcards for formulas and important steps to reinforce your memory.
- Practice solving different types of problems to enhance your versatility.
Break Down Complex Problems
When faced with more challenging exercises, take a step back and break them into smaller, more manageable parts. Often, large problems are simply a series of smaller steps that can be tackled individually.
- Identify what is being asked before beginning any calculation.
- Work through each step methodically, ensuring every calculation is correct.
- Double-check each answer to make sure it makes sense in the context of the problem.
By following these final steps, you can approach your studies with confidence, knowing that your preparation has been thorough. Whether you are preparing for assessments or working through practice problems, consistency and attention to detail are key to mastering the content in this section.